electron scattering (collisions)
Elastic Collisions: Conserve P and E
Inelastic : Only Conserve P
Definition of Momentum Transfer
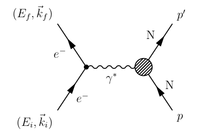
Using Ryder 4-momentum tensor convention: [math]P_{\mu} \equiv (E/c, -\vec{p}) \;\;\;\;\; P^{\mu} \equiv (E/c, \vec{p})[/math]
[math]q_\mu \equiv (\omega, \vec{q})[/math]
[math]k^i_\mu \equiv (E_i, \vec{k_i})[/math]
[math]k^f_\mu \equiv (E_f, \vec{k_f})[/math]
- Conservation of Momentum
- [math] k^i_\mu =q_\mu + k^f_\mu [/math]
- Momentum Transfer
- [math] q_\mu =k^i_\mu - k^f_\mu [/math]
- Momentum Transfer Squared
- [math] -Q^2 = q^2 = q_\mu q^{\mu} =(E_i-E_f)^2 - (\vec{k}_i - \vec{k}_f) \cdot (\vec{k}_i - \vec{k}_f) [/math]
- [math]= m_i^2 + m_f^2 -2E_iE_f + 2\left | \vec{k}_i\right | \left | \vec{k}_f\right | cos(\theta)[/math]
- [math]=-4E_iE_f \sin^2(\theta/2) \;\;\; m_i = m_f \ll E_i \mbox{ and } E \approx \left | \vec{k}_i\right |[/math]
- [math]Q^2 =4E_iE_f \sin^2(\theta/2) \gt 0[/math] A space-like scattering event
Space-like interval: Two events are separated by a space like interval then there isn't enough time passing between them to allow a cause-effect relationship because a photon can't traverse the distance. This means that there is no reference frame that may be used to describe the event as happening at the same spatial location but there is a frame that describes them happening at the same time. If the spacetime interval between the two events is defined as s[math]^2 = (\Delta r)^2 - (c\Delta t)^2[/math] then [math]s^2 \gt 0[/math] for a space-like interval
Time-like interval: Two events are separated by a time like interval if enough time passes between them to allow a cause-effect relationship. This means that there is no reference frame that may be used to describe the event as happening at the same time but there is one that describes them happening at the same spatial location. If the spacetime interval between the two events is defined as s[math]^2 = (\Delta r)^2 - (c\Delta t)^2[/math] then [math]s^2 \lt 0[/math] for a time-like interval
4-Momentum vector definition using Ryder convention
- [math]P_{\mu} \equiv \left ( \frac{E}{c} , - \vec p \right )[/math]
- [math]P^{\mu} \equiv \left ( \frac{E}{c} , \vec p \right )[/math]
- [math]P_{\mu} P^{\mu} = \left ( \frac{E}{c}\right )^2 - \vec p^2 = E^2-p^2 = m^2[/math] if you define the speed of light as unity
- Note
- Other conventions used by Perkins
- [math]P_{\mu} \equiv \left ( \vec p, -E \right )[/math]
- [math]P^{\mu} \equiv \left ( \vec p , E\right )[/math]
or Kollen
- [math]P_{\mu} \equiv \left ( \vec p, iE \right )[/math]
- [math]P^{\mu} \equiv \left ( \vec p , iE\right )[/math]
Momentum transfer is defined as
- [math]q_{\mu} \equiv ( \omega, \vec q) = P^i_{\mu} - P^f_{\mu}[/math] : conservation of momentum
- [math]q_{\mu}q^{\mu} = (E_i - E_f)^2 - (\vec {P}_i - \vec{P}_f) \cdot (\vec {P}_i - \vec{P}_f)[/math]
- [math]= m_i^2 +m_f^2 - 2E_iE_f + 2 \left | \vec {P}_i \right | \left | \vec {P}_f \right |[/math]
Definition of Missing Mass
Inelastic scattering (Energy is not conserved but absorbed from the momentum transfer)
[math]P_e^{\mu} \equiv (E_i,\vec{k}_i)[/math]
[math]\left(P_e^{\mu}\right)^{\prime} \equiv (E_f,\vec{k}_f)[/math]
[math]P_p^{\mu} \equiv (M_p,0)[/math]
[math]\left(P_p^{\mu}\right)^{\prime} \equiv (E_X,\vec{k}_X)[/math]
- 4-momentum conservation
- [math]P_e^{\mu} + P_p^{\mu} = \left(P_e^{\mu}\right)^{\prime} + \left(P_p^{\mu}\right)^{\prime}[/math]
[math]\left(P_p^{\mu}\right)^{\prime} \left(P_{\mu}^p\right)^{\prime} = [P_e^{\mu} + P_p^{\mu} - \left(P_e^{\mu}\right)^{\prime} ][P^e_{\mu} + P^p_{\mu} - \left(P^e_{\mu}\right)^{\prime} ][/math]
[math]E_X^2 - P_X^2 \equiv W^2 = (E_i^2 - k_i^2) + (E_f^2-k_f^2) + M_p^2 + 2M_p(E_i - E_f) -2(E_iE_f-\vec{k_i}\cdot \vec{k_f})[/math]
- [math]W^2=M_p^2 + 2M_p(E_i-E_f) -Q^2 \equiv [/math]Invariant Missing Mass = mass of the intermediate state that was created.
200 px
The Delta Resonance
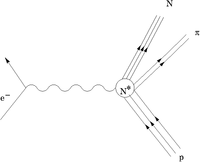
Decay Modes: [math]ep \rightarrow \;\; \left \{ {N \pi \;\;\; 99.5% \atop p \gamma \;\;\; 0.5% } \right .[/math]
[math]\sigma = \lt \Delta^+ | V_{\mu}^3| p\gt [/math]
- [math]V_{\mu}^3 =[/math] Vector current of the virtual photon exchange

Spin 1/2 and 3/2 final states

References
Past colloquia
TF_01092012_Colloq
Theory
Phenomenological
NP, B291(1987)793; NP, B346(1990)1;
Z. Phys. C56(1992)493;
Eur. Phys. J. C44 (2005)219;
hep-ph/0205123
arXiV:1310.5285
QCD inspired
NP, B483(1997)291; NP, B484(1997)265;
PRL 85(2000)3591; PRL 89(2002)162301;
JHEP 0211(2002)44; NP, A720(2003)131;
Eur. Phys. J. C30(2003)213; arXiV:09073534;
NP, A761(2005)67; PR, C81(2010)024902
Hybrids
PYTHIA + BUU simulation
PR, C70(2004)054609; NP, A801(2008)68
Experiment
keith's 2012 talk in Italy on EG1-DVCS
Unpolarized
http://link.springer.com/article/10.1007%2FJHEP04%282014%29005
File:AndyMiller 200TalkAtDESY.pdf 2002 talk by CA Miller at DESY
File:Dueren.98.055.pdf 1998 Duren talk
2013 APS talk on EG1-DVCS
July 30,2014 talk by XingLong Li at Heremes
File:XingLongLi TalkOnPACIAEmdoelForSIDIS.pdf
from
http://www.google.com/url?sa=t&rct=j&q=&esrc=s&source=web&cd=31&ved=0CB0QFjAAOB4&url=http%3A%2F%2Fhadron2014.csp.escience.cn%2Fdct%2Fattach%2FY2xiOmNsYjpwZGY6MzAwMjA%3D&ei=UPw1VNiQAdHlsATA7IHwDw&usg=AFQjCNH5yyek_zTJ22TZq0Dw_YLUeAxe2Q
Xiangs proposal for CLAS12 measurement of SIDIS Xsections see Fig. 1
Semi-inclusive deep inelastic scattering at small transverse momentum
Naomi's SIDIS Hermes talk from 2011 at NNPSS11
1986 article ob QCD effects in semi-inclusive deep inelastic scattering from a polarized target by P. Chiappetta J. -Ph. Guillet
theses
2014 Ph.D thesis of Naomi's student Sylvester Joosten
http://www.google.com/url?sa=t&rct=j&q=&esrc=s&source=web&cd=7&cad=rja&uact=8&ved=0CFYQFjAG&url=http%3A%2F%2Fwww.nikhef.nl%2Fpub%2Fservices%2Fbiblio%2Ftheses_pdf%2Fthesis_E_Garutti.pdf&ei=Osw3VJbSAY27ogSx3IDwDQ&usg=AFQjCNENlNdgPr6dgfq9OanlL8cUnYHmMQ&bvm=bv.77161500,d.cGU
TF_SIDIS_Physics





