Go Back
Four-vector Algebra

Let's do the four-vector algebra:
[math] p_{\gamma} = \left( T_{\gamma},\ T_{\gamma},\ 0,\ 0 \right) [/math]
[math] p_D = \left( m_D,\ 0,\ 0,\ 0 \right) [/math]
[math] p_{n} = \left( E_n,\ \ p_n\cos \Theta_n,\ \ p_n\sin \Theta_n,\ \ 0 \right) [/math]
[math] p_{p} = \left( E_p,\ \ p_p\cos \Theta_p,\ \ p_p\sin \Theta_p,\ \ 0 \right) [/math]
By conservation of four-momentum:
[math] p^{\mu}_{\gamma} + p^{\mu}_D = p^{\mu}_p + p^{\mu}_n\ \ \Rightarrow \ \ p^{\mu}_p = p^{\mu}_{\gamma} + p^{\mu}_D - p^{\mu}_n[/math]
Squaring both side of equation above and using the four-momentum invariants [math](p^{\mu})^2 = m^2[/math] we have:
[math] m_p^2 = m_D^2 + m_n^2 + 2T_{\gamma}\cdot m_D - 2m_D\cdot E_n - 2\left( T_{\gamma}E_n - T_{\gamma}p_n\cos\Theta_n\right) [/math]
Detectors located at [math]\Theta = 90^o[/math] case
Detector is located at [math]\Theta_n = 90^o[/math], so the formula above is simplified:
[math] m_p^2 = m_D^2 + m_n^2 + 2T_{\gamma}\cdot m_D - 2m_D\cdot E_n - 2\ T_{\gamma}E_n [/math]
We can easily solve the equation above with respect to incident photon energy:
[math]T_{\gamma} = \frac {m_p^2 - m_n^2 - m_D^2 + 2\ m_D E_n}{2\ (m_D - E_n)} [/math]
For non-relativistic neutrons [math]T_n \ll m_n = 939.5\ MeV[/math] and
[math]T_{\gamma} = \frac {m_p^2 - m_n^2 - m_D^2 + 2\ m_D m_n + 2\ m_D T_n}{2\ (m_D - m_n)} [/math]
Substituting the corresponding masses, we get finally for the case of non-relativistic neutrons detected at the angle [math]\Theta_n = 90^o[/math]:
[math]T_{\gamma}\ [MeV] = 2.003\ T_n\ [MeV] + 1.577\ [MeV][/math]
and visa versa:
[math]T_n\ [MeV] = 0.499\ T_{\gamma}\ [MeV] - 0.787\ [MeV][/math]
Using the formulas above we can:
1. Calculate the threshold for [math]^2D(n,\gamma)[/math] reaction:
[math]E_{\gamma} = 1.577\ MeV[/math]
2. Predict the incident photon energy based on the detected neutron energy.
3. Predict the detected neutron energy based on the incident photon energy.
- for the incident photons up to 25 MeV we have (0 - 11.69) MeV neutrons
- for the incident photons up to 44 MeV we have (0 - 21.17) MeV neutrons
Also note:
and visa versa
[math] T_n = \frac {2\ T_{\gamma}\ m_D + m_D^2 + m_n^2 - m_p^2} {2\left( T_{\gamma} + m_D \right)} - m_n[/math]
how it looks
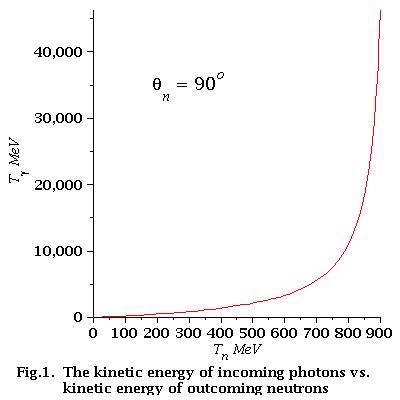
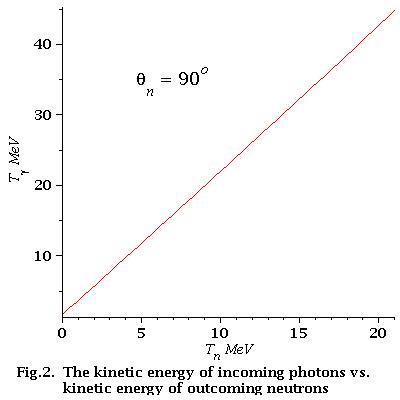
low energy approximation=
As we can see from Fig.2 for low energy neutrons (0-21 MeV)
energy dependence of incident photons is linear
Find that dependence. We have:
[math] T_{\gamma}(0\ MeV) = 1.715360792\ MeV [/math]
[math] T_{\gamma}(21\ MeV) = 44.78703086\ MeV [/math]
So, the equation of the line is:
[math] T_{\gamma}
= \frac{T_{\gamma}(21\ MeV) - T_{\gamma}(0\ MeV)}{21\ MeV - 0\ MeV}\ T_n + T_{\gamma}(0\ MeV) [/math]
Finally for low energy neutrons (0-21 MeV):
[math] T_{\gamma} = 2.051\ T_n + 1.715 [/math]
example of error calculation
example 1
Say, we have, 10 MeV neutron with uncertainty 1 MeV,
the corresponding uncertainly for photons energy is:
[math] \delta T_{\gamma} = 2.051\ \delta T_n = 2.051\times 1\ MeV = 2.051\ MeV [/math]
example 2
Say, the neutron's time of flight uncertainly is 1 ns
The neutron's kinetic energy is:
[math]T_n = m_n (\gamma - 1) = m_n\left[ \frac{1}{\sqrt{1-\left(\frac{l}{c\ t}\right)^2}} - 1 \right][/math]
And:
[math]\delta T_n \left(\delta t\right) = -\ \frac{m\ l^2 \delta t}{\left(1-\left(\frac{l}{c\ t}\right)^2\right)^{3/2}c^2 t^3}[/math]
Also we need the neutron's time of flight as function of neutron's kinetic energy:
[math]t:=\frac{l}{c\ \beta_n} = \frac{l}{c\ (p_n/E_n)} =
\frac{l\ (T_n + m_n)}{c\sqrt{T_n^2+2m_nT_n}}[/math]
Say, we have 10 MeV neutron, 1 m away detector, and neutron's time of flight uncertainty is 1 ns. Then the neutron time of flight is:
[math]t(T_n = 10\ MeV) = 23\ ns[/math]
Neutron kinetic energy errors are:
[math]\delta T_n(\delta t = 1\ ns) = 0.88\ MeV[/math]
[math]\frac{\delta T_n}{T_n} = \frac{0.88\ MeV}{10\ MeV} = 8.8\ %[/math]
And photon energy errors are:
[math]\delta T_{\gamma} = 2.051\cdot \delta T_n = 2.051\cdot 0.88\ MeV = 1.81\ MeV [/math]
[math]\frac{\delta T_{\gamma}}{T_{\gamma}} = \frac{1.80\ MeV}{(2.051\cdot 10 + 1.715)\ MeV} = 8.1\ %[/math]
Below are some examples for different detector distance and neutron kinetic energy:
| [math]\delta t_n[/math]
|
[math]l[/math]
|
[math]T_n[/math]
|
[math]\beta_n[/math]
|
[math]t_n[/math]
|
[math]\delta T_n[/math]
|
[math]\frac{\delta T_n}{T_n}[/math]
|
[math]\delta T_{\gamma}[/math]
|
[math]\frac{\delta T_{\gamma}}{T_{\gamma}}[/math]
|
| 1 ns |
1 m |
5 MeV |
0.103 |
32 ns |
0.31 MeV |
6.2 % |
0.64 MeV |
5.3 %
|
| 1 ns |
1 m |
10 MeV |
0.145 |
23 ns |
0.88 MeV |
8.8 % |
1.81 MeV |
8.1 %
|
| 1 ns |
1 m |
20 MeV |
0.203 |
16 ns |
2.51 MeV |
12.6 % |
5.16 MeV |
12.1 %
|
| 1 ns |
1 m |
0.5 MeV |
0.033 |
102 ns |
0.010 MeV |
1.9 % |
|
|
| 1 ns |
1 m |
1 MeV |
0.046 |
72 ns |
0.028 MeV |
2.8 % |
|
|
| 1 ns |
1 m |
2 MeV |
0.065 |
51 ns |
0.078 MeV |
3.9 % |
|
|
| 1 ns |
1 m |
4 MeV |
0.092 |
36 ns |
0.22 MeV |
5.5 % |
|
|
Go Back


