A W thesis old

Apparatus

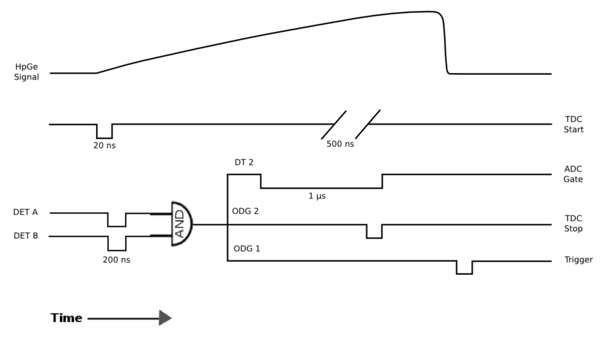
The coincidence counting set-up and modules path are as follows. A source is set atop a base constructed of hydrogen dense blocks. These were chosen to reduce the number of background energy spikes detected. The source is placed an equal distance from decorator A and detector B. Detector A is positioned directly above the source. A high-purity germanium detector is being utilized for this set-up. The one outgoing signal enters a ORTEC 673 Spectroscopy Amplifier and the outgoing signal then passes through a CANBERRA CFD 2035A. The signal can then enter an optional CAEN CFD to produce a time delay, or can pass directly into a channel (1) of the Level Translator. The second outgoing signal from the Spec Amp goes to a peak sensing CAEN V785N. The output from this module goes to the DAQ. Perpendicular from detector A and placed an equal distance from the source is detector B. This detector can either be a NaI-3 or a germanium detector depending on the source being counted. Detector B’s signal goes to a Post Amplifier and one outgoing signal goes to the above mentioned CAEN V785N and also to the DAQ. The second signal enters a CANBERRA CFD 2035A. The outgoing signal goes to a different channel (4) of the same Level Translator. A ribbon cable on the Level Translator module goes to a Time-to-Digital Converter (TDC) CAEN V775 that is operating in common start mode. Both channels (1 and 4) of the Level Translator outgoing signal pass to a 3-fold Logic Unit which is used in both OR mode and AND mode to see coincidence lines. This data is collected in the Trig DAQ.

898 keV signal table

[math]\sigma_Cut[/math]

[math]T_{1/2}[/math]	Trig	Signal	BackG Subtracted	Fit Parameters					Signal Area	Noise Area	SNR
0.44	coin			[math]\mu=[/math] 895.744 +/- 5.774e-4	[math]\sigma=[/math] 0.8189 +/- 0.0034	A= 0.0097 +/- 0.0013	B= 4.891e-9 +/- 6.707e-9	C= 2.543e-5 +/- 1.053e-4	0.0217 +/- 6.383e-4	4.643e-4 +/- 5.804e-5	46.678 +/- 0.0124
0.98	sing			[math]\mu=[/math] 897.255 +/- 0.0047	[math]\sigma=[/math] 0.7277 +/- 0.0280	A=0.7027 +/- 0.0129	B= 8.448e-4+/- 4.457e-5	C=-0.7355 +/- 0.0408	1.2938 +/- 0.0381	0.3123 +/- 0.0390	4.143 +/- 0.6977
	coin			[math]\mu=[/math] 896.818 +/- 0.0075	[math]\sigma=[/math] 0.9856 +/- 0.0276	A= 0.0020 +/- 2.036e-5	B= 1.263e-8 +/- 7.797e-10	C= 1.095e-5 +/- 5.103e-7	0.0049 +/- 1.446e-4	9.518e-5 +/- 1.190e-5	51.591 +/- 0.0036
1.46	sing			[math]\mu=[/math] 897.663 +/- 0.00987	[math]\sigma=[/math] 0.8348 +/- 0.0580	A=0.3925 +/- 0.1107	B=-2.696e-4 +/- 2.725e-7	C= 0.2633 +/- 1.939e-4	1.075 +/- 0.0316	0.0777 +/- 0.0097	13.827 +/- 0.3763
	coin			[math]\mu=[/math] 897.780 +/- 0.0025	[math]\sigma=[/math] 0.9737 +/- 0.0186	A= 1.679e-3 +/- 5.996e-7	B= 1.217e-8 +/- 7.211e-11	C= 1.098e-5 +/- 2.962e-8	3.987e-3 +/- 1.174e-4	8.732e-5 +/- 1.092e-5	45.661 +/- 0.0030
2.12	sing			[math]\mu=[/math] 894.701 +/- 0.0127	[math]\sigma=[/math] 0.6371 +/- 0.0569	A=0.3740 +/- 6.200e-5	B= 8.285e-5+/- 4.503e-8	C=-0.0482+/- 5.103e-7	0.6212 +/- 0.0183	0.0723 +/- 0.0090	8.588 +/- 0.3858
	coin			[math]\mu=[/math] 890.896 +/- 0.0144	[math]\sigma=[/math] 1.137 +/- 0.0056	A= 7.293e-4 +/- 8.083e-9	B= 8.441e-7 +/- 6.284e-11	C= -7.342e-4 +/- 2.106e-7	0.00199 +/- 5.872e-5	8.296e-5 +/- 1.037e-5	24.032 +/- 0.0015
2.27	sing			[math]\mu=[/math] 903.478 +/- 0.0064	[math]\sigma=[/math] 0.7121 +/-0.0877	A=0.2929 +/- 2.373e-4	B= -7.136e-4+/- 1.414e-9	C= 0.6715 +/- 1.777e-4	0.5609+/- 0.0165	0.0862 +/- 0.0108	6.505 +/- 0.3063
	coin			[math]\mu=[/math] 905.932 +/- 0.0050	[math]\sigma=[/math] 0.8066 +/- 0.0236	A= 5.888e-4 +/- 2.063e-7	B= 1.238e-6 +/- 4.497e-10	C= -1.106e-3 +/- 4.701e-7	1.170e-3 +/- 3.446e-5	5.310e-5 +/- 6.638e-6	22.033 +/- 8.137e-4

Re-Fit of Run 7203 without Event Cuts

[math]T_{1/2}[/math]	Trig	Signal	BackG Subtracted	Fit Parameters					Signal Area	Noise Area	SNR
2.12	sing			[math]\mu=[/math] 894.859 +/- 0.0377	[math]\sigma=[/math] 0.8294 +/- 0.0389	A= 0.1064 +/- 1.281e-4	B=4.131e-4 +/- 3e-9	C= -0.3439 +/- 1.047e-4	0.2214 +/- 0.0065	0.0900 +/- 0.0113	2.459 +/- 0.0082

Re-Fit of Run 7203 without Event Cuts and Scaled by 1.66

[math]T_{1/2}[/math]	Trig	Signal	BackG Subtracted	Fit Parameters					Signal Area	Noise Area	SNR
2.12	sing			[math]\mu=[/math] 894.901 +/- 0.0878	[math]\sigma=[/math] 0.8380 +/- 0.0389	A= 0.1774 +/- 4.505e-4	B=4.931e-5 +/- 9.842e-7	C= -2.231e-3 +/- 9.027e-4	0.3731 +/- 0.0110	0.1532 +/- 0.0192	2.435 +/- 0.1543

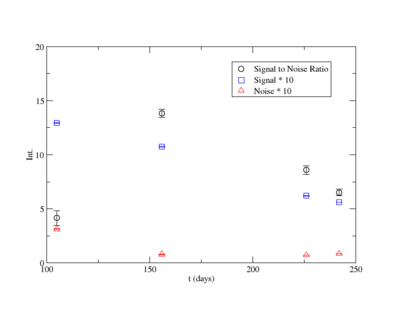
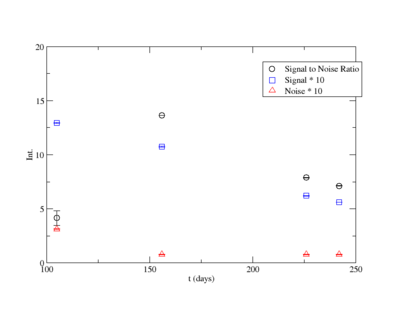
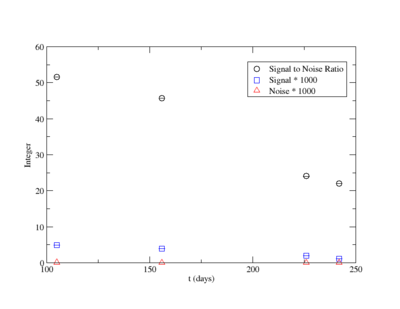
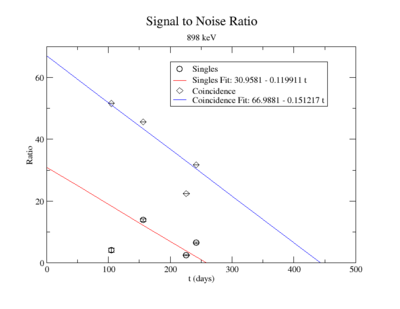
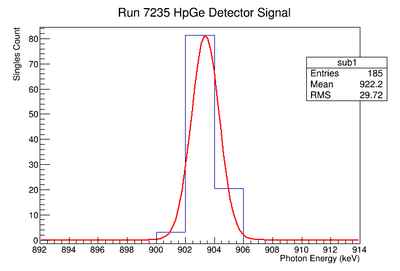
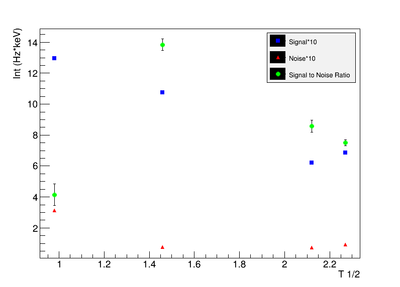
Signal, Noise, and Signal to Noise Ratio plots for 898 keV singles runs.

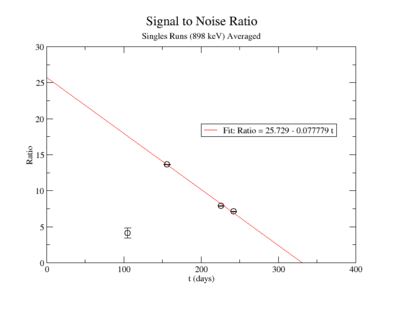
Averaging over the last three noise values:

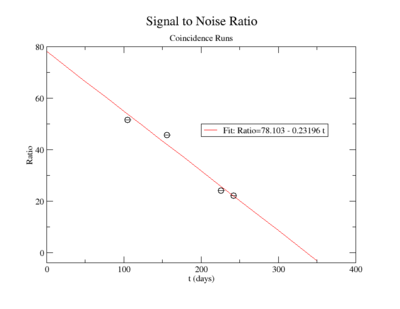
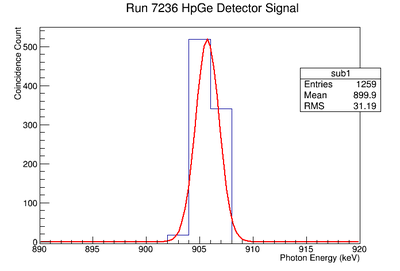
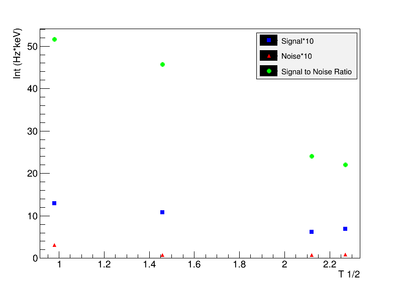
Signal, Noise, and Signal to Noise Ratio plots for 898 keV coincidence runs.

Averaging over the noise values:

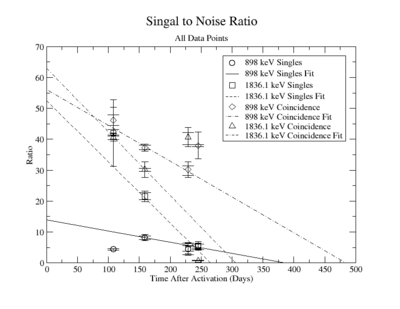
Signal to Noise Ratio (Singles and Coincidence):

1836 keV signal table

1836.1 keV Signal Table

[math]T_{1/2}[/math]	Trig	Signal	BackG Subtracted	Fit Parameters					Signal Area	Noise Area	SNR
0.44	coin			[math]\mu=[/math] 1833.43 +/- 0.0058	[math]\sigma=[/math] 1.447 +/- 0.0228	A= 0.0036 +/- 5.937e-7	B= 1.129e-6 +/- 9.991e-10	C=-2.041e-3 +/- 1.682e-6	0.0127 +/- 3.752e-4	1.937e-4 +/- 2.421e-5	65.781 +/- 0.0096
0.98	sing			[math]\mu=[/math] 1837.7 +/- 0.02	[math]\sigma=[/math] 1.126 +/- 0.0264	A=0.3115 +/- 9.318e-6	B=3.759e-5 +/- 2.793e-7	C=-6.575e-2 +/- 5.102e-4	0.8590 +/- 0.0253	0.0200 +/- 0.0025	42.920 +/- 0.6349
	coin			[math]\mu=[/math] 1835.86 +/- 0.01	[math]\sigma=[/math] 1.406 +/- 0.0201	A= 0.0018 +/- 2.541e-7	B= 4.930e-9 +/- 5.263e-11	C= 8.945e-6 +/- 8.814e-8	0.0063 +/- 1.853e-4	1.037e-4 +/- 1.297e-5	60.662 +/- 0.0047
1.46	sing			[math]\mu=[/math]1838.65 +/- 0.0436	[math]\sigma=[/math] 1.117 +/- 0.0569	A=0.1757 +/- 3.782e-5	B=2.046e-4 +/- 1.5e-9	C=-3.713e-1 +/- 4.940e-5	0.4933 +/- 0.0145	0.0234 +/- 0.0029	21.115 +/- 0.3456
	coin			[math]\mu=[/math] 1838.003 +/- 0.0115	[math]\sigma=[/math] 1.854 +/- 0.0242	A= 9.717e-4 +/- 4.374e-7	B= 7.051e-7 +/- 1.119e-10	C=-1.291e-3 +/- 1.738e-7	0.0044+/- 1.286e-4	4.248e-5 +/- 5.309e-6	102.838 +/- 0.0033
2.12	sing			[math]\mu=[/math] 1831.333 +/- 0.2887	[math]\sigma=[/math] 0.5219 +/- 0.0555	A= 0.1601+/- 1.321e-4	B=-1.429e-6 +/- 1.565e-10	C=2.260e-2 +/- 5.944e-7	0.2214 +/- 0.0065	0.0462 +/- 0.0058	4.796 +/- 0.1192
	coin			[math]\mu=[/math] 1824.26 +/- 0.0153	[math]\sigma=[/math] 1.570 +/- 0.0871	A= 5.842e-4 +/- 1.552e-5	B= -7.557e-7 +/- 6.718e-11	C= 1.405e-3 +/- 1.754e-7	0.0024 +/- 7.002e-5	1.802e-4 +/- 2.252e-5	13.198 +/- 0.0016
2.27	sing			[math]\mu=[/math] 1850.08 +/- 0.0361	[math]\sigma=[/math] 1.238 +/- 0.1486	A=0.0741 +/- 3.058e-4	B=4.389e-4 +/- 5.774e-10	C=-8.040e-1 +/- 1.270e-4	0.2471+/- 0.0073	0.0451 +/- 0.0056	5.478 +/- 0.1354
	coin			[math]\mu=[/math] 1854.58 +/- 0.1587	[math]\sigma=[/math] 1.696+/- 0.1625	A= 3.873e-4 +/- 8.569e-7	B= -9.482e-7 +/- 7.143e-11	C= 0.0018 +/-3.216e-7	0.0017 +/- 5.083e-5	1.623e-5 +/- 2.028e-6	106.360 +/- 0.0012

Re-Fit of Run 7203 without Event Cuts

[math]T_{1/2}[/math]	Trig	Signal	BackG Subtracted	Fit Parameters					Signal Area	Noise Area	SNR
2.12	sing			[math]\mu=[/math]1831.55 +/- 1.74	[math]\sigma=[/math]0.6455 +/- 0.0693	A= 0.0658 +/- 0.0007	B= 7.775e-6+/- 1.615e-7	C= -7.311e-3 +/- 2.991e-4	0.1139 +/- 0.0034	0.0216 +/- 0.0027	5.282 +/- 0.0598

Re-Fit of Run 7236 without Event Cuts

[math]T_{1/2}[/math]	Trig	Signal	BackG Subtracted	Fit Parameters					Signal Area	Noise Area	SNR
2.12	sing			[math]\mu=[/math] 1860.48 +/- 0.0874	[math]\sigma=[/math]7.160 +/- 0.5751	A= 7.067e-5 +/- 1.151e-6	B= 8.389e-9+/- 1.764e-8	C= -1.293e-5 +/- 3.280e-5	0.0013 +/- 3.914e-5	0.0021 +/- 0.0003	0.6289 +/- 0.0007

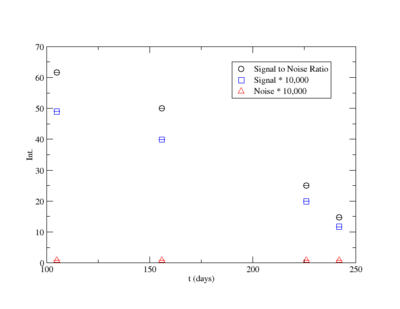
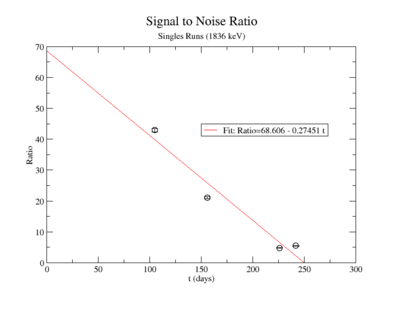
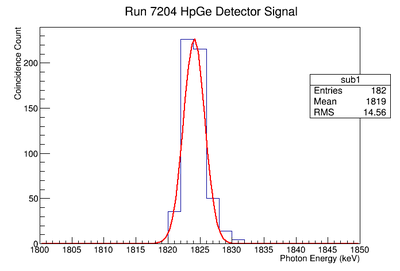
Signal, Noise, and Signal to Noise Ratio plots for 1836 keV singles runs.

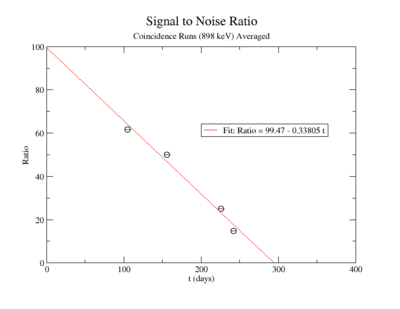
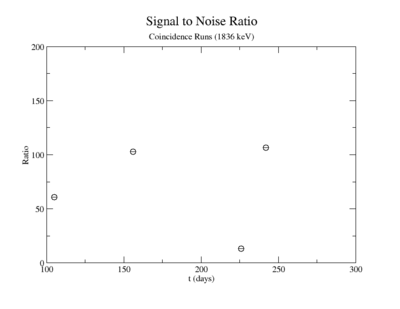
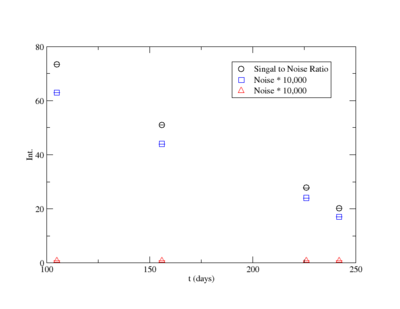
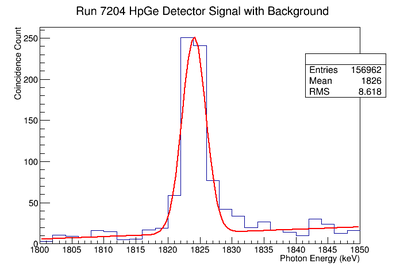
Signal, Noise, and Signal to Noise Ratio plots for 1836 keV coincidence runs.

Averaging over the noise values:

Signal to Noise Ratio (Singles and Coincidence):

Error analysis

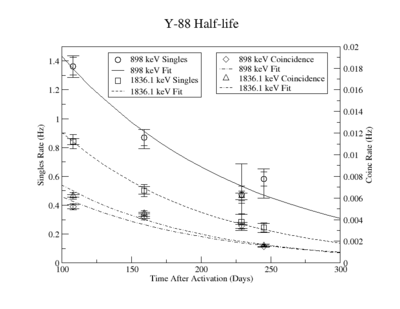
Half-life

Values

898 keV Singles

Days	[math]T_{1/2}[/math]	Trig	Energy Window	Energy Calibration	Linear Fit of Background	Gaussian Integration of Signal	Total Systematic Error
105	0.98	sing	[math] {+0.005} \atop { -0.009}[/math]	[math] {+0.014} \atop { -0.025}[/math]	[math]\pm 0.0025[/math]	[math]\pm 0.07[/math]	[math] {+0.073} \atop { -0.078}[/math]
156	1.46	sing	[math] {+0.0062} \atop { -0.032}[/math]	[math] {+0.0071} \atop { -0.0212}[/math]	[math] {+0.0065} \atop { -0.0063}[/math]	[math]\pm 0.0547[/math]	[math] {+0.057} \atop { -0.077}[/math]
226	2.12	sing	[math] {+0.1554} \atop { -0.1128}[/math]	[math] {+0.0562} \atop { -0.0774}[/math]	[math] {+0.0128} \atop { -0.0122}[/math]	[math]\pm 0.0174[/math]	[math] {+0.213} \atop { -0.191}[/math]
242	2.27	sing	[math] {+0.0069} \atop { -0.0481}[/math]	[math] {+0.0246} \atop { -0.0697}[/math]	[math]\pm 0.01[/math]	[math]\pm 0.062[/math]	[math] {+0.07} \atop { -0.133}[/math]

898 keV Coincidence

Days	[math]T_{1/2}[/math]	Trig	Energy Window	Energy Calibration	Linear Fit of Background	Gaussian Integration of Signal	Coincidence Energy	Total Systematic Error
105	0.98	coin	[math] {+2.9e-5} \atop { -2.1e-5}[/math]	[math] \pm 1.6e-5[/math]	[math] {+7.358e-6} \atop { -7.092e-6}[/math]	[math]\pm 0.00018[/math]	[math] {+5.68e-4} \atop { -1.75e-3}[/math]	[math] {+6.39e-4} \atop { -1.8e-3}[/math]
156	1.46	coin	[math] {+1.7e-5} \atop { -2.0e-6}[/math]	[math] \pm 1.6e-5[/math]	[math] {+2.004e-6} \atop { -1.990e-6}[/math]	[math] {+7.611e-5} \atop { -7.605e-5}[/math]	[math] {+4.03e-4} \atop { -6.22e-4}[/math]	[math] {+4.43e-4} \atop { -6.45e-4}[/math]
226	2.21	coin	[math] {+2.0e-5} \atop { -1.06e-4}[/math]	[math] {+3.5e-5} \atop { -6.8e-5}[/math]	[math] {+8.041e-6} \atop { -7.859e-6}[/math]	[math]\pm 0.00013[/math]	[math] {+4.92e-4} \atop { -1.06e-3}[/math]	[math] {+5.62e-4} \atop { -1.24e-3}[/math]
242	2.27	coin	[math] {+3.0e-6} \atop { -1.0e-6}[/math]	[math] {+2.0e-6} \atop { -8e-6}[/math]	[math] {+4.436e-6} \atop { -4.365e-6}[/math]	[math]\pm 1.078e-5[/math]	[math] {+3.63e-4} \atop { -1.52e-3}[/math]	[math] {+3.68e-4} \atop { -1.53e-3}[/math]

1836.1 keV Singles

Days	[math]T_{1/2}[/math]	Trig	Energy Window	Energy Calibration	Linear Fit of Background	Gaussian Integration of Signal	Total Systematic Error
105	0.98	sing	[math] {+0.001} \atop { -8.0e-4}[/math]	[math]\pm 0.0028[/math]	[math] {+0.0051} \atop { -0.0049}[/math]	[math]\pm 0.0197[/math]	[math]\pm 0.021[/math]
156	1.46	sing	[math] {+0.001} \atop { -0.004}[/math]	[math] {+0.0141} \atop { -0.0071}[/math]	[math]\pm 0.0014[/math]	[math]\pm 0.0240[/math]	[math] {+0.028} \atop { -0.026}[/math]
226	2.12	sing	[math] {+0.0288} \atop { -0.0211}[/math]	[math] {+0.0122} \atop { -0.0244}[/math]	[math] {+0.0092} \atop { -0.0085}[/math]	[math] {+0.0321} \atop { -0.0320}[/math]	[math] {+0.053} \atop { -0.056}[/math]
242	2.27	sing	[math] {+6.0e-4} \atop { -0.013}[/math]	[math]\pm 0.0041[/math]	[math] {+0.0055} \atop { -0.0054}[/math]	[math] {+0.027} \atop { -0.0272}[/math]	[math] {+0.028} \atop { -0.033}[/math]

1836.1 keV Coincidence

Days	[math]T_{1/2}[/math]	Trig	Energy Window	Energy Calibration	Linear Fit of Background	Gaussian Integration of Signal	Coincidence Energy Cut	Total Systematic Error
105	0.98	coin	[math] {+3.8e-5} \atop { -3.4e-5}[/math]	[math] {+3.2e-5} \atop { -3.1e-5}[/math]	[math] {+2.505e-6} \atop { -2.475e-6}[/math]	[math]\pm 8.97e-5[/math]	[math] {+3.11e-4} \atop { -2.57e-3}[/math]	[math] {+3.91e-4} \atop { -2.64e-3}[/math]
156	1.46	coin	[math] {+2.0e-5} \atop { -9.0e-6}[/math]	[math] {+1.2e-5} \atop { -3.7e-5}[/math]	[math] {+3.422e-6} \atop { -3.347e-6 }[/math]	[math]\pm 5.83e-5[/math]	[math] {+4.63e-4} \atop { -2.82e-3}[/math]	[math] {+4.98e-4} \atop { -2.87e-3}[/math]
226	2.12	coin	[math] {+3.9e-5} \atop { -1.58e-4}[/math]	[math] {+6.9e-5} \atop { -13.6e-5}[/math]	[math] {+1.515e-5} \atop { -1.481e-5}[/math]	[math]\pm 2.13e-4[/math]	[math] {+1.16e-3} \atop { -5.77e-3}[/math]	[math] {+1.29e-3} \atop { -6.07e-3}[/math]
242	2.27	coin	[math] {+2.0e-6} \atop { -7.0e-6}[/math]	[math] \pm 3.0e-6[/math]	[math]\pm 6.18e-6[/math]	[math]\pm 0.00012 [/math]	[math] {+4.51e-4} \atop { -1.21e-3}[/math]	[math] {4.72e-4} \atop { -1.23e-3}[/math]

Percent Error

898 keV Singles

Days	[math]T_{1/2}[/math]	Trig	Energy Window	Energy Calibration	Linear Fit of Background	Gaussian Integration of Signal	Total Systematic Error
105	0.98	sing	[math] {+0.367%} \atop { -0.660%}[/math]	[math] {+1.026%} \atop { -1.83%}[/math]	[math]\pm 0.183%[/math]	[math]\pm 5.132%[/math]	[math] {+5.32%} \atop { -5.71%}[/math]
156	1.46	sing	[math] {+0.713%} \atop { -3.68%}[/math]	[math] {+0.816%} \atop { -2.438%}[/math]	[math] {+0.747%} \atop { -0.724%}[/math]	[math]\pm 6.29%[/math]	[math] {+6.52%} \atop { -8.80%}[/math]
226	2.12	sing	[math] {+32.83%} \atop { -23.83%}[/math]	[math] {+11.87%} \atop { -16.35%}[/math]	[math] {+2.7%} \atop { -2.58%}[/math]	[math]\pm 3.68%[/math]	[math] {+44.94%} \atop { -40.44%}[/math]
242	2.27	sing	[math] {+1.19%} \atop { -8.28%}[/math]	[math] {+4.24%} \atop { -12.0%}[/math]	[math]\pm 1.72%[/math]	[math]\pm 10.68%[/math]	[math] {+12.1%} \atop { -22.99%}[/math]

898 keV Coincidence

Days	[math]T_{1/2}[/math]	Trig	Energy Window	Energy Calibration	Linear Fit of Background	Gaussian Integration of Signal	Coincidence Energy	Total Systematic Error
105	0.98	coin	[math] {+0.56%} \atop { -0.404%}[/math]	[math] {+0.31%} \atop { -1.21%}[/math]	[math] {+0.142%} \atop { -0.136%}[/math]	[math]\pm 3.46%[/math]	[math] {+10.92%} \atop { -33.65%}[/math]	[math] {+12.29%} \atop { -35.44%}[/math]
156	1.46	coin	[math] {+0.405%} \atop { -0.048%}[/math]	[math] {+0.36%} \atop { -0.31%}[/math]	[math] {+0.048%} \atop { -0.047%}[/math]	[math]\pm 1.81%[/math]	[math] {+9.6%} \atop { -14.8%}[/math]	[math] {+10.51%} \atop { -12.27%}[/math]
226	2.21	coin	[math] {+0.588%} \atop { -3.12%}[/math]	[math] {+0.59%} \atop { -1.74%}[/math]	[math] {+0.237%} \atop { -0.231%}[/math]	[math]\pm 3.82%[/math]	[math] {+14.5%} \atop { -31.2%}[/math]	[math] {+16.11%} \atop { -36.23%}[/math]
242	2.27	coin	[math] {+0.2%} \atop { -0.067%}[/math]	[math] {+0.13%} \atop { -0.267%}[/math]	[math] {+0.296%} \atop { -0.291%}[/math]	[math]\pm 0.719%[/math]	[math] {+24.2%} \atop { -101.3%}[/math]	[math] {+24.55%} \atop { -101.67%}[/math]

1836.1 keV Singles

Days	[math]T_{1/2}[/math]	Trig	Energy Window	Energy Calibration	Linear Fit of Background	Gaussian Integration of Signal	Total Systematic Error
105	0.98	sing	[math] {+0.119%} \atop { -0.095%}[/math]	[math]\pm 0.33%[/math]	[math] {+0.606%} \atop { -0.582%}[/math]	[math]\pm 2.34%[/math]	[math] {+2.46%} \atop { -2.45%}[/math]
156	1.46	sing	[math] {+0.199%} \atop { -0.798%}[/math]	[math] {+2.81%} \atop { -1.42%}[/math]	[math]\pm 0.279%[/math]	[math]\pm 4.79%[/math]	[math] {+5.66%} \atop { -5.28%}[/math]
226	2.12	sing	[math] {+10.21%} \atop { -7.48%}[/math]	[math] {+4.32%} \atop { -8.65%}[/math]	[math] {+3.26%} \atop { -3.01%}[/math]	[math] {+11.37%} \atop { -11.34%}[/math]	[math] {+18.74%} \atop { -19.94%}[/math]
242	2.27	sing	[math] {+0.245%} \atop { -5.31%}[/math]	[math]\pm 1.674%[/math]	[math] {+2.246%} \atop { -2.205%}[/math]	[math] {+11.025%} \atop { -11.107%}[/math]	[math] {+11.414%} \atop { -13.303%}[/math]

1836.1 keV Coincidence

Days	[math]T_{1/2}[/math]	Trig	Energy Window	Energy Calibration	Linear Fit of Background	Gaussian Integration of Signal	Coincidence Energy Cut	Total Systematic Error
105	0.98	coin	[math] {+0.613%} \atop { -0.55%}[/math]	[math] {+1.27%} \atop { -0.5%}[/math]	[math]\pm 0.04%[/math]	[math]\pm 1.45%[/math]	[math] {+5.02%} \atop { -41.45%}[/math]	[math] {+7.05%} \atop { -42.52%}[/math]
156	1.46	coin	[math] {+0.435%} \atop { -0.196%}[/math]	[math] {+0.522%} \atop { -0.804%}[/math]	[math] {+0.074%} \atop { -0.073% }[/math]	[math]\pm 1.267%[/math]	[math] {+10.07%} \atop { -61.3%}[/math]	[math] {+11.09%} \atop { -62.31%}[/math]
226	2.12	coin	[math] {+0.629%} \atop { -2.55%}[/math]	[math] {+1.11%} \atop { -1.10%}[/math]	[math] {+0.244%} \atop { -0.239%}[/math]	[math]\pm 3.44%[/math]	[math] {+18.71%} \atop { -93.06%}[/math]	[math] {+20.74%} \atop { -96.77%}[/math]
242	2.27	coin	[math] {+0.125%} \atop { -0.438%}[/math]	[math] {+0.125%} \atop { -0.188%}[/math]	[math]\pm 0.386% [/math]	[math]\pm 7.5% [/math]	[math] {+28.2%} \atop { -75.6%}[/math]	[math] {29.41%} \atop { -76.62%}[/math]

SNR

The signal to noise ratio is

Days	[math]T_{1/2}[/math]	Trig	SNR	Statistical Error	Systematic Error
105	0.98	sing	42.07	[math]\pm 0.0483[/math]	[math]\pm 0.0197[/math]
		coin	9.65	[math]\pm 1.04e-3 [/math]	[math] {+1.47e-4} \atop { -1.11e-4}[/math]
156	1.46	sing	21.52	[math]\pm 0.0420 [/math]	[math] {+0.028} \atop { -0.026}[/math]
		coin	7.16	[math]\pm 1.02e-3 [/math]	[math] {+7.31e-5} \atop { -7.43e-5}[/math]
226	2.12	sing	4.64	[math]\pm 0.0597 [/math]	[math] {+0.053} \atop { -0.056}[/math]
		coin	9.65	[math]\pm 1.09e-3 [/math]	[math] {+2.39e-4} \atop { -3.11e-4}[/math]
242	2.27	sing	5.43	[math]\pm 0.0325 [/math]	[math] {+0.028} \atop { -0.033}[/math]
		coin	2.49	[math]\pm 9.89e-4 [/math]	[math] {+1.20e-4} \atop { -1.21e-4}[/math]

=other

Energy	Trig	[math]T_{1/2}[/math] (days)	[math]N_0[/math] (before efficiency)	[math]N_0[/math] (after efficiency)	[math]T_{final}[/math]	[math] N(t_{final})[/math]	[math]\frac{N(t_{final})}{N_0}[/math]
898	sing	130.39 +/- 26.26	1.281e-8 +/- 2.236e-9	1.636e-6 +/- 2.957e-7	383.63 [math] {+202.83} \atop { -119.44}[/math]	1.351e-7 +/- 7.551e-8	0.0826 +/- 0.0485
	coin	108.18 +/- 4.89	6.411e-11 +/- 1.314e-12	3.794e-8 +/- 2.633e-9	483.60 [math] {+111.97} \atop { -83.40}[/math]	1.636e-9 +/- 3.954e-10	0.0431 +/- 0.0108
1836.1	sing	106.16 +/- 8.63	9.634e-9 +/- 1.089e-9	3.089e-6 +/- 3.619e-7	264.94 [math] {+29.37} \atop { -26.24}[/math]	5.519e-7 +/- 8.705e-8	0.1787 +/- 0.0351
	coin	100.10 +/- 4.0	8.147e-11 +/- 1.670e-12	4.171e-8 +/- 1.860e-9	305.63 [math] {+64.72} \atop { -49.63}[/math]	5.721e-9 +/- 1.238e-9	0.1371 +/- 0.0303

898 keV Signal and Background Noise Table

Run #	Area of Signal	Area of Background	Signal to Noise Ratio
7203	130.17	120.59	1.08
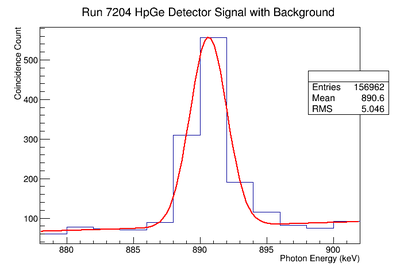
7204	825.34	558.66	1.48
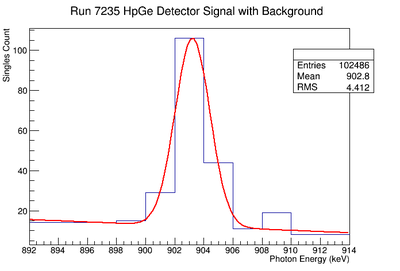
7235	194.38	60.23	3.23
7236	1429.75	436.93	3.27

A_W_thesis

1836.1 keV Signal and Background Noise Table

Run #	Area of Signal	Area of Background	Signal to Noise Ratio
7203	83.29	24.73	3.37
7204	944.58	101.68	9.29
7235	82.25	20.90	3.94
7236	1849.86	97.64	18.95

HpGe Detector

Run	Source	Energy	Expected rate (Hz)	HpGe Det B Rate (ADC 7) (Hz)	HpGe Eff (%)
7027	Na-22	511	1589.218 +/- 49.425	18.07 +/- 0.111	1.137 +/- 0.0317
7025	Cs-137	661.657	2548.03 +/- 80.008	25.468+/- 0.172	0.9995 +/- 0.0321
7029	Mn-54	834.848	41.405 +/- 1.300	0.485 +/- 0.014	1.171 +/- 0.0427
7026	Co-60	1173.228	1876.619 +/- 58.926	11.03 +/- 0.106	0.588 +/- 0.0328
7027	Na-22	1274.537	888.264 +/- 27.625	4.857 +/- 0.034	0.5468 +/- 0.0319
7026	Co-60	1332.492	1878.167 +/- 58.974	9.584 +/- 0.023	0.510 +/- 0.0315

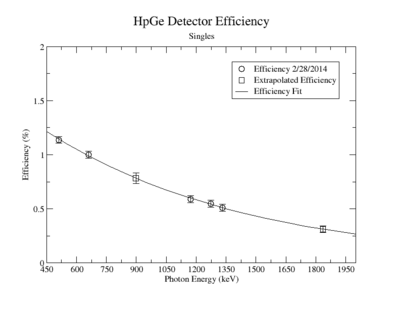
Extrapolated Efficiency

Source	Energy	HpGe Eff (%)
Y-88	898	1.036 +/- 0.0226
Y-88	1836.1	0.1983 +/- 0.0178

Exp Fit including Cs-137

  1  Constant     7.96246e-01   6.78458e-02   6.83891e-05  -2.33206e-03
  2  Slope       -1.07699e-03   7.10063e-05   7.15739e-08  -2.09517e+00

f(x) = exp(p0+p1*x).

Extrapolated Efficiency

Source	Energy	HpGe Eff (%)
Y-88	898	0.8429 +/- 0.0034
Y-88	1836.1	0.3069 +/- 0.0192

Energy	Trig	[math]N_0[/math] (before efficiency)
898	sing	1.281e-8 +/- 2.254e-9
	coin	6.411e-11 +/- 3.572e-12
1836.1	sing	9.634e-9 +/- 1.102e-9
	coin	8.147e-11 +/- 2.987e-12

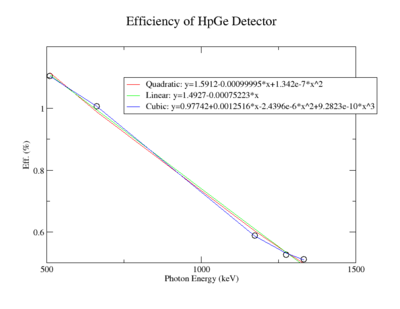
Exp Fit including all Na-22 points and excluding Mn-54

  1  Constant     6.36491e-01   4.70444e-02   1.00271e-05  -3.28621e-03
  2  Slope       -9.81232e-04   5.68236e-05   1.21109e-08  -1.59453e+00

Extrapolated Efficiency

Source	Energy	HpGe Eff (%)
Y-88	898	0.7830 +/- 0.0471
Y-88	1836.1	0.3119 +/- 0.0310

Energy	Trig	[math]N_0[/math] (before efficiency)
898	sing	1.281e-8 +/- 2.254e-9
	coin	6.411e-11 +/- 3.572e-12
1836.1	sing	9.634e-9 +/- 1.102e-9
	coin	8.147e-11 +/- 2.987e-12

Efficiency on 2/28/2014

Run	Source	Energy	Expected rate (Hz)	HpGe Det B Rate (ADC 7) (Hz)	HpGe Eff (%)
7028	Ba-133	80.9971	1067.468 +/- 1.131	14.483 +/- 1.442	1.357
7028	Ba-133	276.3997	221.297 +/- 0.2345	4.239 +/- 0.9671	1.916
7028	Ba-133	302.8510	574.310 +/- 0.6085	9.523 +/- 0.5539	1.658
7028	Ba-133	356.0134	1941.418 +/- 2.057	27.130 +/- 1.316	1.397
7028	Ba-133	383.8480	278.416 +/- 0.2950	3.982 +/- 0.2440	1.430
7027	Na-22	511	1589.218 +/- 1.842	17.545 +/- 0.4560	1.104
7025	Cs-137	661.657	2548.022 +/- 2.222	25.622+/- 0.3286	1.006
7029	Mn-54	834.848	4032.782 +/- 0.0114	0.2911 +/- 0.1667	0.0072
7026	Co-60	1173.228	1876.619 +/- 0.2687	11.062 +/- 0.1215	0.589
7027	Na-22	1274.537	888.264 +/- 1.029	4.685 +/- 0.0825	0.527
7026	Co-60	1332.492	1878.167 +/- 0.2690	9.616 +/- 0.1181	0.512

Run	Source	Energy	Expected rate (Hz)	NaI Det B Rate (ADC 7) (Hz)	NaI Eff (%)
7027	Na-22	511	1589.218 +/- 49.425	14.86 +/- 0.0945	0.9351 +/- 0.0317
7025	Cs-137	661.657	2548.03 +/- 80.008	23.45 +/- 0.9729	0.9203 +/- 0.0520
7026	Co-60	1173.228	1876.619 +/- 58.926	8.001 +/- 0.0953	0.4264 +/- 0.0336
7027	Na-22	1274.537	888.264 +/- 27.625	4.05 +/- 0.0247	0.4559 +/- 0.0317
7026	Co-60	1332.492	1878.167 +/- 58.974	7.234 +/- 0.1675	0.3852 +/- 0.0390

Signal, Noise, and Signal to Noise Ratio plots for 898 keV singles runs.

Signal, Noise, and Signal to Noise Ratio plots for 898 keV coincidence runs.

[math]\sigma_Fit[/math]

[math]T_{1/2}[/math]	Trig	Fit Parameters					Signal Area	Noise Area	SNR
0.98	sing	[math]\mu=[/math] 897.260 +/- 0.0047	[math]\sigma=[/math] 0.7074 +/- 0.0280	A=0.725 +/- 0.0129	B= 8.448e-4+/- 4.457e-5	C=-0.7355 +/- 0.0408	0.9938 +/- 0.0054	0.3039 +/- 0.0380	3.270 +/- 0.0243
	coin	[math]\mu=[/math] +/-	[math]\sigma=[/math] +/-	A= +/-	B= +/-	C= +/-	+/-	+/-	+/-
2.27	sing	[math]\mu=[/math] 903.482 +/- 0.0064	[math]\sigma=[/math] 0.6595 +/- 0.0877	A= 0.3199 +/- 0.0156	B= -7.248e-4+/- 9.968e-7	C= 6.816e-1 +/- 8.138e-4	0.5146 +/- 0.0073	0.0852 +/- 0.0107	6.037 +/- 0.0082
	coin	[math]\mu=[/math] +/-	[math]\sigma=[/math] +/-	A= +/-	B= +/-	C= +/-	+/-	+/-	+/-

Fit method

Do the fit:

get parameters for line

Then fill 1 histogram with line

Then subtract

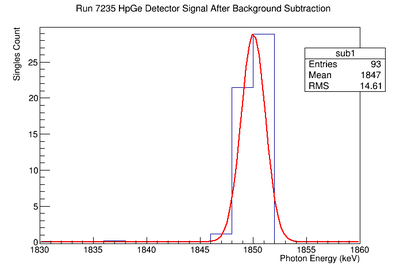
TH1F *coin1=new TH1F("coin1","coin1",30,1800,1860);

ntuple->Draw("ADC7*0.604963-49.7001 >>coin1")

TH1F *lin1=new TH1F("lin1","line1",30,1800,1860);

for(int i=1800;i<1861;i++){
lin1->Fill(i,-2028+1.12*i)
}

TH1F *sub1=new TH1F("sub1","sub1",30,1800,1860);

sub1->Add(coin1,1);
sub1->Add(lin1,-1);

sub1->Draw();

A pdf of the Mathematica notebook used to calculate background area, gaussian area, and plot signal/noise vs. activity.

File:AW Background Noise custom4.pdf

All the ROOT fit parameters used to find the background and the resulting peaks.

File:Y-88 Fit Log Scaled&Cut.pdf

Integrating the gaussian of the HpGe detector signal.

File:AW Gaussian Integral2.pdf

[math] f(x)=A \textstyle \int_{\mu-2\sigma}^{\mu+2\sigma} e^{-\frac{1}{2}\left(\frac{x-\mu}{\sigma}\right)^2} \operatorname{d}\!x +B x +C[/math]

Old Analysis Section

Run6980_Y88

Run7023_Y88

Run7108_Y88

Run7161_Y88

Run7204_Y88

Run7236_Y88

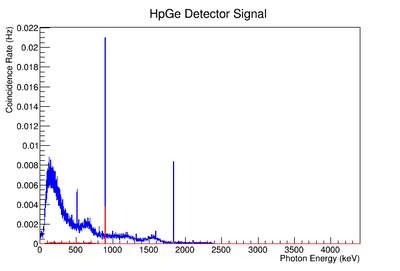
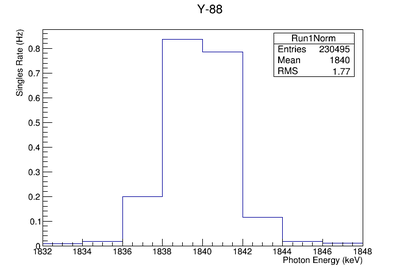
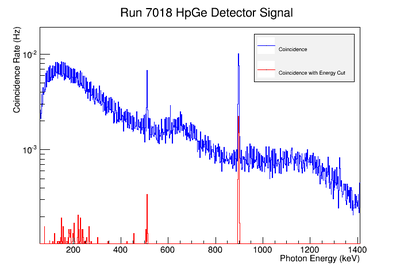
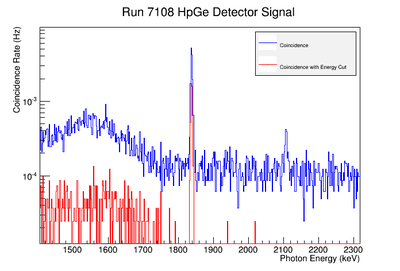
Using our Y-88 source, our set-up allows us to perform runs with the detectors in coincidence (AND mode) or singles (OR mode). The figure on the left shows the HpGe detector's coincidence events (red) that occurred within a 200 ns timing window. This graph has been overlaid with the same detector’s single events (blue). We can see that by having the detectors in coincidence, the noise is reduced and several peaks, which do not have multiple photons in coincidence, are removed.

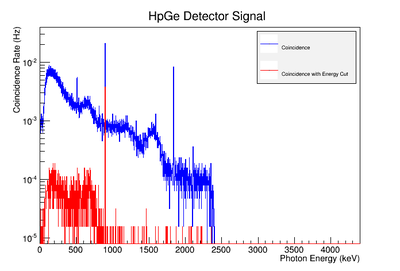
The coincidence run does not show the two energy peaks associated with Y-88 decay alone. Even though we require coincidence for the system to trigger, there is still noise. These peaks could be Compton events contaminating the coincidence and accidentals. Photons can loss a portion of their energy traveling to the detector. They do not necessarily have to deposit all of their energy into the detector either. The photon can Compton scatter out. It is not possible to tell the difference between a photon that deposited all of its energy from a scattered photon, since the speed of light is so fast and the resolution of the detector so poor. The photons can, however, be distinguished if an energy cut is applied. In order to remove the Compton events and the accidentals, we can require the photon energy to be completely deposited into the detector. This can be achieved by placing an energy cut on the NaI detector around the high energy Y-88 peak. This will remove other unwanted photon energies. The graph on the right is an overlay of the coincidence signal before (blue) and after (red) an energy cut is applied to the companion detector (NaI detector). The noise seen at low energies is greatly reduced after including the cut and improved the signal.

898 keV analysis

The raw Histograms and fits may be found here A_W_thesis_old#898_keV_signal_table

The Measured half life is

Y-88 Half-life

Days	[math]T_{1/2}[/math]	Trig	Integral	Statistical Error	Systematic Error	Systematic Error without Coincidence Energy Cut
108	1.01	sing	1.364	[math]\pm 0.062 [/math]	[math] {+0.073} \atop { -0.078}[/math]	N/A
		coin	0.0052	[math]\pm 2.864e-4 [/math]	[math] {+6.39e-4} \atop { -1.8e-3}[/math]	[math] {+1.86e-4} \atop { -1.84e-4}[/math]
159	1.49	sing	0.8697	[math]\pm 0.055 [/math]	[math] {+0.057} \atop { -0.077}[/math]	N/A
		coin	0.0042	[math]\pm 2.263e-4 [/math]	[math] {+4.43e-4} \atop { -6.45e-4}[/math]	[math] {+8.3e-5} \atop { -7.82e-5}[/math]
229	2.15	sing	0.4733	[math]\pm 0.058 [/math]	[math] {+0.213} \atop { -0.191}[/math]	N/A
		coin	0.0034	[math]\pm 2.587e-4 [/math]	[math] {+5.62e-4} \atop { -1.24e-3}[/math]	[math] {+1.41e-4} \atop { -2.17e-4}[/math]
245	2.30	sing	0.5807	[math]\pm 0.049 [/math]	[math] {+0.07} \atop { -0.133}[/math]	N/A
		coin	0.0015	[math]\pm 5.454e-5 [/math]	[math] {+3.68e-4} \atop { -1.53e-3}[/math]	[math] {+1.27e-5} \atop { -1.47-5}[/math]

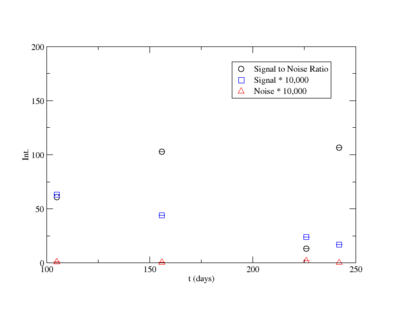
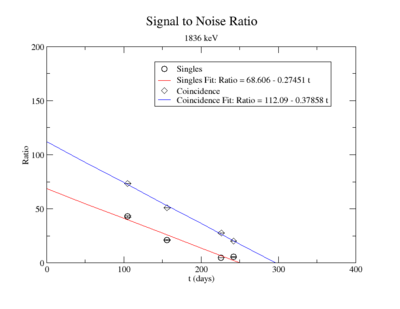
The signal to noise ratio is

Days	[math]T_{1/2}[/math]	Trig	SNR	Statistical Error	Systematic Error
108	1.01	sing	4.368	[math]\pm 0.252 (5.77%) [/math]	[math] {+0.232} \atop { -0.249}[/math]
		coin	46.181	[math]\pm 4.204 (9.10%)[/math]	[math] {+1.653} \atop { -1.635}[/math]
159	1.49	sing	8.228	[math]\pm 0.999 (12.14%)[/math]	[math] {+0.536} \atop { -0.724}[/math]
		coin	37.30	[math]\pm 1.132 (3.03%)[/math]	[math] {+0.739} \atop { -0.694}[/math]
229	2.15	sing	4.478	[math]\pm 2.047 (45.71%) [/math]	[math] {+2.008} \atop { -1.811}[/math]
		coin	30.195	[math]\pm 2.480 (8.21%)[/math]	[math] {+1.253} \atop { -1.926}[/math]
245	2.30	sing	5.494	[math]\pm 1.415 (25.75%)[/math]	[math] {+0.665} \atop { -1.263}[/math]
		coin	37.994	[math]\pm 4.287 (11.28%)[/math]	[math] {+0.322} \atop { -0.372}[/math]

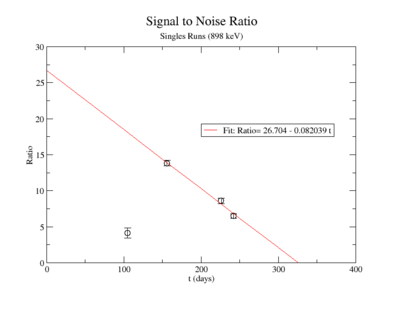
The first data point for the singles 898 keV was not included in the signal to noise fit. At the time of measurement, the noise was larger. This could be from contaminates with short half-lives in the sample that decayed away. Only elements with longer half-lives, such as Y-88, remained. The background of the remaining three data points were averaged.

For the 898 keV coincidence signal to noise fit, the last data point was not included. The length of the run and activities in the room, such as the nitrogen tank refilling, during counting caused the peak to be spread over a larger energy range. This also meant that the background noise would be larger. The background of the first three data points were averaged.

what about third data point

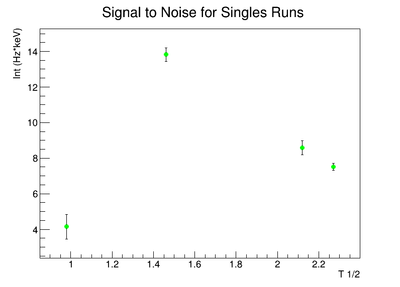
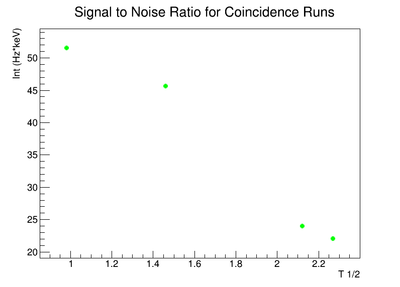
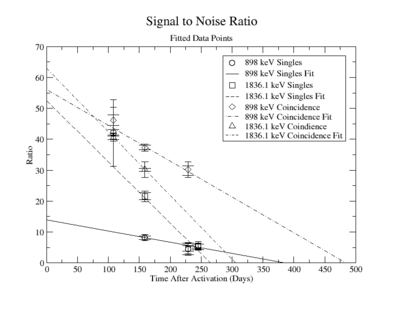
The following plot is the signal to noise ratio with only the data points used to obtain a fit.

Energy	Trig	[math]T_{1/2}[/math] (days)	[math]A_0[/math] (before efficiency) (Hz)	[math]N_0[/math] (before efficiency) (nuclei)	[math]A_0[/math] (after efficiency) (Hz)	[math]N_0[/math] (after efficiency) (nuclei)	[math]T_{final}[/math] (days)	[math]A(t_{final})[/math] (Hz)	[math] N(t_{final})[/math] (nuclei)	[math]\frac{N(t_{final})}{N_0}[/math]
898	sing	130.39 +/- 26.26 115.05 +/- 27.87	3.081 +/- 0.538 3.464 +/- 0.604	4.096e7 +/- 7.147e6 4.604e7 +/- 8.034e6	393.544 +/- 71.131 422.377 +/- 79.957	5.231e9 +/- 9.454e8 5.88e9 +/-1.063e9	383.63 [math] {+202.83} \atop { -119.44}[/math]	32.506 +/- 18.162 36.539 +/- 20.416	4.320e8 +/- 2.414e8 4.857e8 +/- 2.714e8	0.0826 +/- 0.0485
	coin	108.18 +/- 4.89	0.0154 +/- 0.0003	204958.085 +/- 4201.641	9.125 +/- 0.633	1.213e8 +/- 8.416e6	483.60 [math] {+111.97} \atop { -83.40}[/math]	0.3935 +/- 0.0951	5.230e6 +/- 1.264e6	0.0431 +/- 0.0108
1836.1	sing	106.16 +/- 8.63	2.317 +/- 0.262	3.080e7 +/- 3.480e6	742.929 +/- 87.053	9.874e9 +/- 1.157e9	264.94 [math] {+29.37} \atop { -26.24}[/math]	132.74 +/- 20.938	1.764e9 +/- 2.783e8	0.1787 +/- 0.0351
	coin	100.10 +/- 4.0	0.0196 +/- 0.0004	260440.778 +/- 5339.036	10.033 +/- 0.447	1.334e8 +/- 5.946e6	305.63 [math] {+64.72} \atop { -49.63}[/math]	1.376 +/- 0.298	1.829e7 +/- 3.958e6	0.1371 +/- 0.0303

March 2015

Energy	Trig	[math]T_{1/2}[/math] (days)	[math]A_0[/math] (before efficiency) (Hz)	[math]N_0[/math] (before efficiency) (nuclei)	[math]A_0[/math] (after efficiency) (Hz)	[math]N_0[/math] (after efficiency) (nuclei)	[math]T_{final}[/math] (days)	[math]A(t_{final})[/math] (Hz)	[math] N(t_{final})[/math] (nuclei)	[math]\frac{N(t_{final})}{N_0}[/math]
898	sing	152.83 +/- 26.99	1.371 +/- 0.239	1.822e7 +/- 3.180e6	175.093 +/- 31.647	2.327e9 +/- 4.206e8 4.057e9 +/- 7.333e8	[math] {+} \atop { -}[/math]
	coin	108.18 +/- 4.89	0.0154 +/- 0.0003	204958.085 +/- 4201.641	9.125 +/- 0.633	1.213e8 +/- 8.416e6	483.60 [math] {+111.97} \atop { -83.40}[/math]	0.3935 +/- 0.0951	5.230e6 +/- 1.264e6	0.0431 +/- 0.0108
1836.1	sing	101.099 +/- 14.473	0.904 +/- 0.102	1.202e7 +/- 1.358e6	289.993 +/- 33.98	3.854e9 +/- 4.516e8 5.471e9 +/- 6.410e8	[math] {+} \atop { -}[/math]
	coin	100.10 +/- 4.0	0.0196 +/- 0.0004	260440.778 +/- 5339.036	10.033 +/- 0.447	1.334e8 +/- 5.946e6	305.63 [math] {+64.72} \atop { -49.63}[/math]	1.376 +/- 0.298	1.829e7 +/- 3.958e6	0.1371 +/- 0.0303

Average [math] N_0[/math]: [math](38.4 \pm 5.29) \times 10^{8}[/math]

[math]N = \frac{A}{\sigma \Phi \left ( 1 - e^{- \lambda t_i} \right ) e^{- \lambda t_d}}[/math]

[math]N[/math] = number of atoms in sample that were activated

[math]A[/math]= activity measure at time [math]t_d[/math]

[math]\sigma[/math] = photo nuclear cross section

[math]\Phi[/math] = photon flux

[math]\lambda[/math] = decay constant

[math]t_i[/math] = irradiation time

[math]t_d[/math] = decay time that has expired until A is measured

Using nickel target you can take ratios to try and approximate [math]\Phi[/math]

Media:ContHealtPhys_Bevelacqua_2004APPIII.pdf

http://www.nist.gov/pml/data/xcom/

Sample	[math]E_{\gamma}[/math] (keV)	Activity (Hz)	Parent Nuclei [math]\sigma_{\gamma}[/math] (barn)	[math]\lambda \left (\frac{1}{s} \right)[/math]
Y-88	898	[math] 3.148 \pm 0.012[/math]	[math]0.7645 \pm 0.0035[/math]	7.53e-8
	1836.1	[math]1.921 \pm 0.0006[/math]
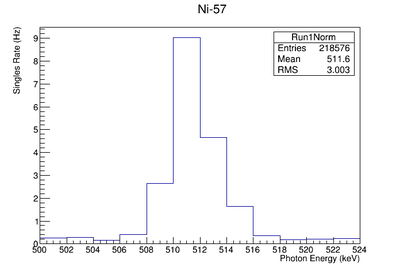
Ni-57	1377.63	[math]0.4542 \pm 0.015[/math]	1.188	5.41e-6
	511	[math]17.5 \pm 0.19[/math]
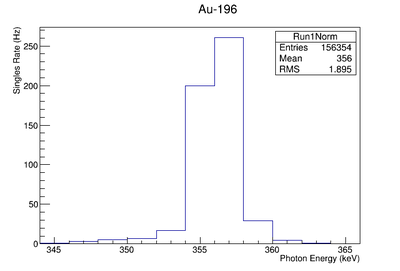
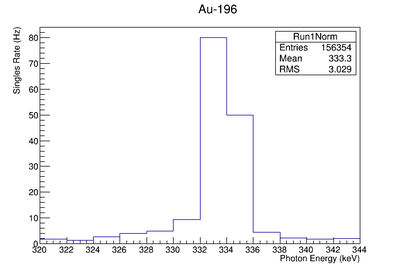
Au-196	355.73	[math]503.6 \pm 3.95[/math]	5.886	1.30e-6
	333.03	[math]138.3 \pm 2.18[/math]

[math]t_i[/math] = 5 hr =18000 s

[math]t_d[/math] = 5 days = 432000 s

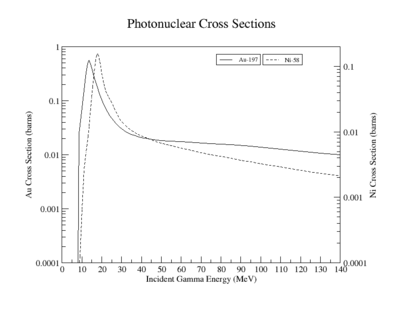
File:Photonuclear xsections.pdf

Nickel normalization

Reaction

Ni-08-22-13

Run list

PAA_11-11-13

Photon absorption cross sections:

Ni-58 plot data table

Ni-60 http://www.nndc.bnl.gov/sigma/getPlot.jsp?evalid=15652&mf=3&mt=5&nsub=0

Ni-61 http://www.nndc.bnl.gov/sigma/getPlot.jsp?evalid=15653&mf=3&mt=5&nsub=0

Ni-62 http://www.nndc.bnl.gov/sigma/getPlot.jsp?evalid=15654&mf=3&mt=5&nsub=0

Ni-64 http://www.nndc.bnl.gov/sigma/getPlot.jsp?evalid=15655&mf=3&mt=5&nsub=0

according to pg 13.3 on gets the total cross section by adding up the cross section in the tables up to the desired energy.

http://www.bnl.gov/isd/documents/70393.pdf

Gold normalization

Reaction:

Naturally occurring Isotopes of Gold are Au-197 ( 100% )

Neutron knockout:

Au-197 ->Au-196 has the following lines in order of decreasing intensity: 355.73, 333.03 , half life =9.6 hours, 6.167 days

Proton knockout:

Goes to a stable isotope

NP knockout:

Au-197->Pt-195 no high intensity lines above 100 keV , half life = 4.010 days

Photon absorption cross sections:

Au-197

plot data table

1836.1 Analysis

The raw Histograms and fits may be found here A_W_thesis_old#1836_keV_signal_table

The Measured half life is

Y-88 Half-life

Days	[math]T_{1/2}[/math]	Trig	Integral	Statistical Error	Systematic Error	Systematic Error without Coincidence Energy Cut
108	1.01	sing	0.8414	[math]\pm 0.048 [/math]	[math]\pm 0.021[/math]	N/A
		coin	0.0062	[math]\pm 3.13e-4 [/math]	[math] {+3.91e-4} \atop { -2.64e-3}[/math]	[math] {+1.14e-4} \atop { -1.11e-4}[/math]
159	1.49	sing	0.5015	[math]\pm 0.042 [/math]	[math] {+0.028} \atop { -0.026}[/math]	N/A
		coin	0.0046	[math]\pm 2.37e-4 [/math]	[math] {+4.98e-4} \atop { -2.87e-3}[/math]	[math] {+6.66e-5} \atop { -7.43e-5}[/math]
229	2.15	sing	0.2822	[math]\pm 0.059[/math]	[math] {+0.053} \atop { -0.056}[/math]	N/A
		coin	0.0062	[math]\pm 4.60e-4[/math]	[math] {+1.29e-3} \atop { -6.07e-3}[/math]	[math] {+2.39e-4} \atop { -3.63e-4}[/math]
245	2.30	sing	0.2449	[math]\pm 0.032 [/math]	[math] {+0.028} \atop { -0.033}[/math]	N/A
		coin	0.0016	[math]\pm 5.63e-5 [/math]	[math] {4.72e-4} \atop { -1.23e-3}[/math]	[math] {+1.20e-4} \atop { -1.21e-4}[/math]

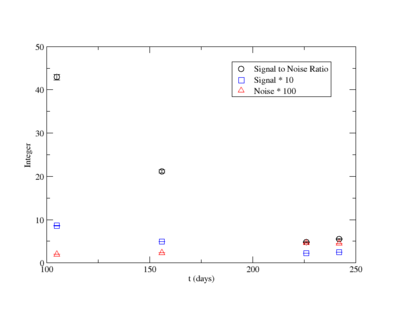
The signal to noise ratio is

Days	[math]T_{1/2}[/math]	Trig	SNR	Statistical Error	Systematic Error
108	1.01	sing	42.07	[math]\pm 10.78 (25.62%)[/math]	[math] {+1.035} \atop { -1.031}[/math]
		coin	40.666	[math]\pm 1.236 (3.04%) [/math]	[math] {+0.748} \atop { -0.728}[/math]
159	1.49	sing	21.52	[math]\pm 1.722 (8.23%) [/math]	[math] {+1.218} \atop { -1.136}[/math]
		coin	30.172	[math]\pm 2.480 (8.22%) [/math]	[math] {+0.437} \atop { -0.489}[/math]
229	2.15	sing	4.64	[math]\pm 1.161 (25.03%) [/math]	[math] {+0.870} \atop { -0.925}[/math]
		coin	40.666	[math]\pm 3.095 (7.61%) [/math]	[math] {+1.566} \atop { -2.379}[/math]
245	2.30	sing	5.43	[math]\pm 0.980 (18.05%) [/math]	[math] {+0.620} \atop { -0.722}[/math]
		coin	0.7569	[math]\pm 0.057 (7.57%) [/math]	[math] {+0.0568} \atop { -0.0572}[/math]

For the 1836 keV coincidence signal to noise fit, the last data point was not included. The length of the run and activities in the room, such as the nitrogen tank refilling, during counting caused the peak to be spread over a larger energy range. This also meant that the background noise would be large. The background of the first three data points were averaged.

The following plot is the signal to noise ratio with only the data points used to obtain a fit.

Energy	Trig	[math]T_{1/2}[/math] (days)	[math]A_0[/math] (before efficiency) (Hz)	[math]N_0[/math] (before efficiency) (nuclei)	[math]A_0[/math] (after efficiency) (Hz)	[math]N_0[/math] (after efficiency) (nuclei)	[math]T_{final}[/math] (days)	[math]A(t_{final})[/math] (Hz)	[math] N(t_{final})[/math] (nuclei)	[math]\frac{N(t_{final})}{N_0}[/math]
898	sing	130.39 +/- 26.26	3.081 +/- 0.538	4.096e7 +/- 7.147e6	393.544 +/- 71.131	5.231e9 +/- 9.454e8	383.63 [math] {+202.83} \atop { -119.44}[/math]	32.506 +/- 18.162	4.320e8 +/- 2.414e8	0.0826 +/- 0.0485
	coin	108.18 +/- 4.89	0.0154 +/- 0.0003	204958.085 +/- 4201.641	9.125 +/- 0.633	1.213e8 +/- 8.416e6	483.60 [math] {+111.97} \atop { -83.40}[/math]	0.3935 +/- 0.0951	5.230e6 +/- 1.264e6	0.0431 +/- 0.0108
1836.1	sing	106.16 +/- 8.63	2.317 +/- 0.262	3.080e7 +/- 3.480e6	742.929 +/- 87.053	9.874e9 +/- 1.157e9	264.94 [math] {+29.37} \atop { -26.24}[/math]	132.74 +/- 20.938	1.764e9 +/- 2.783e8	0.1787 +/- 0.0351
	coin	100.10 +/- 4.0	0.0196 +/- 0.0004	260440.778 +/- 5339.036	10.033 +/- 0.447	1.334e8 +/- 5.946e6	305.63 [math] {+64.72} \atop { -49.63}[/math]	1.376 +/- 0.298	1.829e7 +/- 3.958e6	0.1371 +/- 0.0303

Average [math] N_0[/math]: [math](38.4 \pm 5.29) \times 10^{8}[/math]

Error analysis

1.) rate energy cuts

2.) Energy calibration error

3.) Linear background fit error

4.) Gaussian integration of Signal - background peak

5.) ignorable error from run length time

6.) Coincidence energy cut.

7.) dividing by average background for SNR

8.) correction ratio for 3rd data point

9.) error in half life to find N0 for the detection limit extrapolation

10.) error extrapolation efficiency to higher energy lines for N0 calculation

Energy Window systematic error

The signal is measured by subtracting a count rate that is summed over an energy interval. The choice of this integration interval, energy window, is a source of systematic error. In order to calculate the error associated with the energy window, its range was adjusted based on the statistical variance of the background subtracted count rate fit to a gaussian. The integral was measured for window ranges chosen according to an integer interval of the standard deviation [math] \mu \pm n \sigma [/math], where n = 3,4,5. The difference between the largest and mid-value was taken as the positive standard deviation, and the lowest was used for the standard deviation negative fluctuation. The resulting errors were divided by the signal to obtain a percent error as shown in column 1 of TableXXX.

Energy calibration systematic error

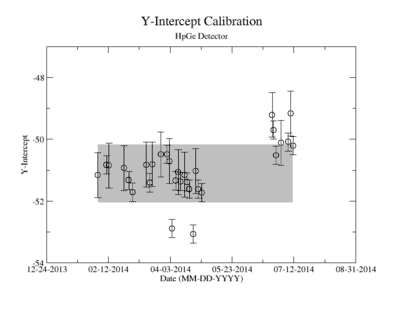
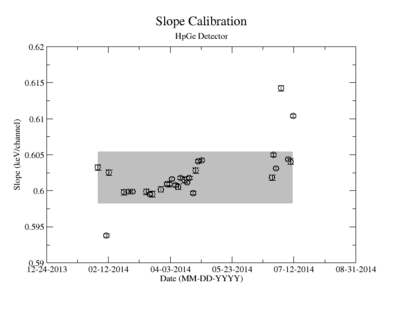
Prior to each measurement of the Y-88 sample's activity, the detectors where calibrated using several standard sources with known activity and dominant energy lines. This was accomplished by counting certified samples of Cs-137, Co-60, and Na-22 as well as Ba-133 and Mn-54. The observed mean ADC channel number was correlated with the expected energy line from each of the above sources. The correlation was measured using a standard linear fit algorithm. The linear fit algorithm determines the uncertainty of each fit parameter, the slope and the y-intercept. The effects of this uncertainty on the signal was analysed and is referred to as the energy calibration error.

The uncertainty in the energy calibration just before a singles measurement was used to investigate the impact of the calibration on the measured signal for a given energy window of integration. While the singles measurements took less than 10 minutes, the coincidence measurement required more than 24 hours. The long term variance of the energy calibration shown in Figure ZZ.XXX was used to estimate the impact of the energy calibration drift during the coincidence measurements. The fit's y-intercept has a temporal variance corresponding to a relative uncertainty of about 1.865%; the slope is about 0.608%. The effects of this error on the coincidence signal were analysed. In both cases, the range of the energy widow is held constant while the slope and the y-intercept where adjusted to account for all possible combinations with and without their respective uncertainty. The integral value was recorded after every fit parameter change and the max, min, and mean values were obtained. The difference between the largest and mean value was taken as the positive standard deviation, and the lowest was used for the standard deviation's negative fluctuation. The resulting errors were divided by the signal to obtain a percent error as shown in column 2 of TableXXX.

Linear Fit Systematic error

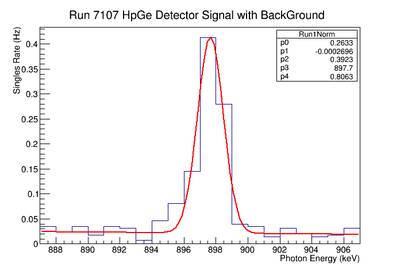
The signal is determine by subtracting the underlying background. A estimate of this background is made by fitting the observed count rate with the function below :

[math] f(x)=A \textstyle \int_{\mu-2\sigma}^{\mu+2\sigma} e^{-\frac{1}{2}\left(\frac{x-\mu}{\sigma}\right)^2} \operatorname{d}\!x +B x +C[/math].

The above function contains a Gaussian representing the measured signal and a linear function for the background. The above figure XX.YY shows the fit applied. The linear fit parameters are used to create a histogram that is subtracted from the raw signal histogram, resulting in a histogram of just a signal. The energy window range used for the fit was adjusted and several fits performed to determine the uncertainty of the fit's slope and y-intercept. This systematic error, based on the fits dependence on the energy window is shown in the table under the column labeled "Linear Fit of Background". The fit parameters where adjusted to determine the maximum and minimum area of the background. For minimum background area, it was required that the area be positive. The difference between the maximum and unadjusted area value was taken as the positive standard deviation's fluctuation, and the minimum was used for the standard deviation's negative fluctuation. The resulting errors were divided by the signal to obtain a percent error as shown in column 3 of TableXXX.

Systematic error of the Coincidence Energy Cut

Figure AAA.XXX below show the coincidence measurements made before(blue) and after(red) energy cuts are applied on the NaI signal to reduce the accidental coincidence events and photons that fail to deposit all their energy in the NaI detector due to compton scattering.

To analyze the error association with coincidence counting only, the signal on the NaI detector was fit to a gaussian distribution. The [math]\sigma[/math] of the gaussian fit was used to adjust the energy cut limits. The energy window was set at [math]\text{lower/upper limit} \pm \sigma [/math]. The intergal values were recorded for each instance and the difference between the maximum and unadjusted integral values were taken as the positive standard deviation's fluctuation while the minimums were used for the standard deviation's negative fluctuation. The resulting errors were divided by the coincidence signal to obtain a percent error as shown in column 5 of TableXXX. The limited energy resolution of the NaI detector makes this uncertainty the dominant systematic error. If two HpGe detectors are used, then this uncertainty would decrease substantially will be far less than the remaining dominant systematic error; the energy Gaussian integral uncertainty. We report this systematic uncertainty but it is not propagated for the final result in order consider the case of a coincidence apparatus using two HpGe detectors.

Half-life

898 keV Singles

Days	[math]T_{1/2}[/math]	Trig	Energy Window	Energy Calibration	Linear Fit of Background	Gaussian Integration of Signal	Total Systematic Error
108	1.01	sing	[math] {+0.367%} \atop { -0.660%}[/math]	[math] {+1.026%} \atop { -1.83%}[/math]	[math]\pm 0.183%[/math]	[math]\pm 5.132%[/math]	[math] {+5.32%} \atop { -5.71%}[/math]
159	1.49	sing	[math] {+0.713%} \atop { -3.68%}[/math]	[math] {+0.816%} \atop { -2.438%}[/math]	[math] {+0.747%} \atop { -0.724%}[/math]	[math]\pm 6.29%[/math]	[math] {+6.52%} \atop { -8.80%}[/math]
229	2.15	sing	[math] {+32.83%} \atop { -23.83%}[/math]	[math] {+11.87%} \atop { -16.35%}[/math]	[math] {+2.7%} \atop { -2.58%}[/math]	[math]\pm 3.68%[/math]	[math] {+44.94%} \atop { -40.44%}[/math]
245	2.30	sing	[math] {+1.19%} \atop { -8.28%}[/math]	[math] {+4.24%} \atop { -12.0%}[/math]	[math]\pm 1.72%[/math]	[math]\pm 10.68%[/math]	[math] {+12.1%} \atop { -22.99%}[/math]

898 keV Coincidence

Days	[math]T_{1/2}[/math]	Trig	Energy Window	Energy Calibration	Linear Fit of Background	Gaussian Integration of Signal	Coincidence Energy	Total Systematic Error	Systematic Error without Coincidence Energy Cut
108	1.01	coin	[math] {+0.56%} \atop { -0.404%}[/math]	[math] \pm 0.31%[/math]	[math] {+0.142%} \atop { -0.136%}[/math]	[math]\pm 3.46%[/math]	[math] {+10.92%} \atop { -33.65%}[/math]	[math] {+12.29%} \atop { -34.62%}[/math]	[math] {+3.58%} \atop { -3.54%}[/math]
159	1.49	coin	[math] {+0.405%} \atop { -0.048%}[/math]	[math] \pm 0.38%[/math]	[math] {+0.048%} \atop { -0.047%}[/math]	[math]\pm 1.81%[/math]	[math] {+9.6%} \atop { -14.8%}[/math]	[math] {+10.55%} \atop { -15.36%}[/math]	[math] {+1.98%} \atop { -1.86%}[/math]
229	2.25	coin	[math] {+0.588%} \atop { -3.12%}[/math]	[math] {+1.03%} \atop { -2.0%}[/math]	[math] {+0.237%} \atop { -0.231%}[/math]	[math]\pm 3.82%[/math]	[math] {+14.5%} \atop { -31.2%}[/math]	[math] {+16.53%} \atop { -36.47%}[/math]	[math] {+4.15%} \atop { -6.38%}[/math]
245	2.30	coin	[math] {+0.2%} \atop { -0.067%}[/math]	[math] {+0.13%} \atop { -0.53%}[/math]	[math] {+0.296%} \atop { -0.291%}[/math]	[math]\pm 0.719%[/math]	[math] {+24.2%} \atop { -101.3%}[/math]	[math] {+24.53%} \atop { -102.0%}[/math]	[math] {+0.847%} \atop { -0.98%}[/math]

1836.1 keV Singles

Days	[math]T_{1/2}[/math]	Trig	Energy Window	Energy Calibration	Linear Fit of Background	Gaussian Integration of Signal	Total Systematic Error
108	1.01	sing	[math] {+0.119%} \atop { -0.095%}[/math]	[math]\pm 0.33%[/math]	[math] {+0.606%} \atop { -0.582%}[/math]	[math]\pm 2.34%[/math]	[math] {+2.46%} \atop { -2.45%}[/math]
159	1.49	sing	[math] {+0.199%} \atop { -0.798%}[/math]	[math] {+2.81%} \atop { -1.42%}[/math]	[math]\pm 0.279%[/math]	[math]\pm 4.79%[/math]	[math] {+5.66%} \atop { -5.28%}[/math]
229	2.15	sing	[math] {+10.21%} \atop { -7.48%}[/math]	[math] {+4.32%} \atop { -8.65%}[/math]	[math] {+3.26%} \atop { -3.01%}[/math]	[math] {+11.37%} \atop { -11.34%}[/math]	[math] {+18.74%} \atop { -19.94%}[/math]
245	2.30	sing	[math] {+0.245%} \atop { -5.31%}[/math]	[math]\pm 1.674%[/math]	[math] {+2.246%} \atop { -2.205%}[/math]	[math] {+11.025%} \atop { -11.107%}[/math]	[math] {+11.414%} \atop { -13.303%}[/math]

1836.1 keV Coincidence

Days	[math]T_{1/2}[/math]	Trig	Energy Window	Energy Calibration	Linear Fit of Background	Gaussian Integration of Signal	Coincidence Energy Cut	Total Systematic Error	Systematic Error without Coincidence Energy Cut
108	1.01	coin	[math] {+0.613%} \atop { -0.55%}[/math]	[math] {+0.52%} \atop { -0.5%}[/math]	[math]\pm 0.04%[/math]	[math]\pm 1.45%[/math]	[math] {+5.02%} \atop { -41.45%}[/math]	[math] {+6.31%} \atop { -42.58%}[/math]	[math] {+1.84%} \atop { -1.79%}[/math]
159	1.49	coin	[math] {+0.435%} \atop { -0.196%}[/math]	[math] {+0.261%} \atop { -0.804%}[/math]	[math] {+0.074%} \atop { -0.073% }[/math]	[math]\pm 1.267%[/math]	[math] {+10.07%} \atop { -61.3%}[/math]	[math] {+10.83%} \atop { -62.39%}[/math]	[math] {+1.45%} \atop { -1.62%}[/math]
229	2.15	coin	[math] {+0.629%} \atop { -2.55%}[/math]	[math] {+1.11%} \atop { -2.19%}[/math]	[math] {+0.244%} \atop { -0.239%}[/math]	[math]\pm 3.44%[/math]	[math] {+18.71%} \atop { -93.06%}[/math]	[math] {+20.81%} \atop { -97.90%}[/math]	[math] {+3.85%} \atop { -5.85%}[/math]
245	2.30	coin	[math] {+0.125%} \atop { -0.438%}[/math]	[math] \pm 0.188%[/math]	[math]\pm 0.386% [/math]	[math]\pm 7.5% [/math]	[math] {+28.2%} \atop { -75.6%}[/math]	[math] {29.5%} \atop { -76.88%}[/math]	[math] {+7.5%} \atop { -7.56%}[/math]

Systematic error in the SNR

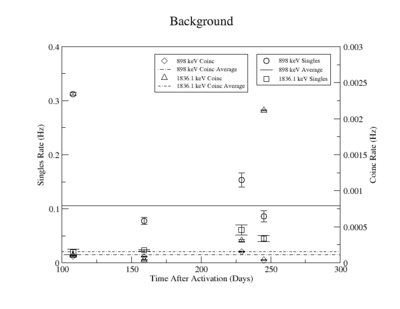
The singles measurement of the 898 keV peak had an initial high background that decays quickly and was more or less constant for singles measurements take 159 days after irradiation. We assumed that this reflected the presence of activated material with half lives longer that 245 days. As a result the background for the last three measurements was averaged and used in the denominator for the SNR. The first singles measurement has a large background due to activated nuclei with short half lives. This background decreased substantially after about 51 days when the next measurement was taken (the half-life of Y-88 is 106.6 days). The singles background near the 1836 keV line was measured to be around 0.5 Hz and fluctuated less over the experiment compared to the background around the 898 peak. The coincidence measurements had such little background, due to the nature of the apparatus, that the background was averaged over the first three measurements for both the 898 and the 1836 peaks separately. The background for the final coincidence measurements was ignored because of the increased noise produced when the detectors nitrogen dewar was filled during the 5 day background measurement.

The systematic uncertainty of the background was not analysed and propagated into the final SNR uncertainty. The signal's total systematic error, described above, was used for the final uncertainty.

Signal to Noise Ratio

Energy (keV)	Trig	Variance of Averaged Background
898	sing	[math]\pm 39.16%[/math]
	coin	[math]\pm 50.48%[/math]
1836.1	sing	N/A
	coin	[math]\pm 153.50%[/math]

The background's systematic error is being ignored.

Background contribution to the SNR error

The analysis of the background uncertainty is described in the above section, "Linear Fit Systematic error." The background's relative statistical uncertainty shown in column 5 of TableXXX is calculated by varying the integral of the background by the uncertainties from the fit and dividing by the background used for the SNR ratio calculation. Column 6 presents the uncertainty of the averaged background as mentioned in the previous section. The variance the coincidence measurement is considerable larger than the individual coincidence measurements. The background in each singles measurement is smaller than the average variance indicating that the individual background measurements could have been made longer to improve their statistical uncertainty.

898 keV Background

Days	[math]T_{1/2}[/math]	Trig	Background	Linear Fit of Background Error	Statistical Error of Averaged Background
108	1.01	sing	0.3123	[math]\pm 0.801%[/math]	N/A
		coin	9.518e-5	[math] {+7.73%} \atop { -7.45%}[/math]	[math]\pm 50.01%[/math]
159	1.49	sing	0.0777	[math] {+8.37%} \atop { -8.11%}[/math]	[math]\pm 5.9%[/math]
		coin	8.732e-5	[math] {+2.30%} \atop { -2.28%}[/math]	[math]\pm 54.51%[/math]
229	2.15	sing	0.1532	[math] {+8.36%} \atop { -7.96%}[/math]	[math]\pm 3.0%[/math]
		coin	1.554e-4	[math] {+5.17%} \atop { -5.06%}[/math]	[math]\pm 30.63%[/math]
245	2.30	sing	0.0862	[math]\pm 11.60%[/math]	[math]\pm 5.3%[/math]
		coin	3.948e-5	[math] {+11.24%} \atop { -11.06%}[/math]	[math]\pm 120.57%[/math]

1836 keV Background

Days	[math]T_{1/2}[/math]	Trig	Background	Linear Fit of Background Error	Statistical Error
108	1.01	coin	1.037e-4	[math] {+2.42%} \atop { -2.39% }[/math]	[math]\pm 951.78%[/math]
159	1.49	coin	4.248e-5	[math] {+8.06%} \atop { -7.88% }[/math]	[math]\pm 232.34%[/math]
229	2.15	coin	3.112e-4	[math] {+4.87%} \atop { -4.76%}[/math]	[math]\pm 317.16%[/math]
245	2.30	coin	2.114e-3	[math]\pm 0.29% [/math]	[math]\pm 46.69%[/math]

Singles Efficiency on 2/28/2014

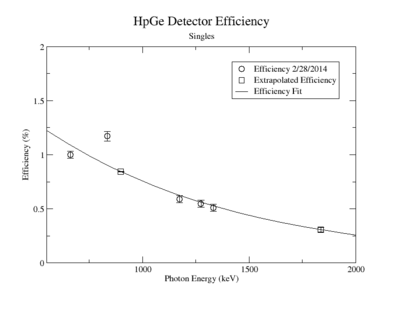
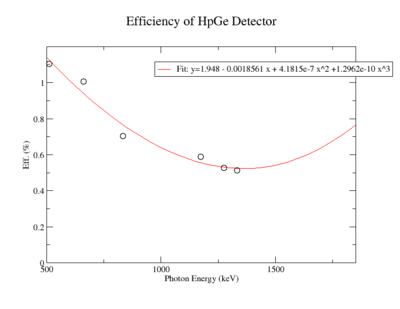
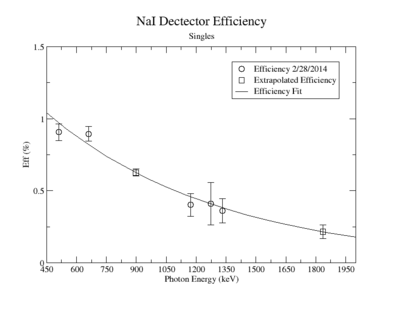
A measurement of the HpGe detectors geometric efficiency for detecting the Y-88 energy lines at 898 keV and 1836.1 keV was performed in order to determine the absolute activity of the sample. The count rate for several sources of known activity was measured by the data acquisition system. Since each sample has decayed since the original calibration by the source vendor, column 4 of tableXX.YY. reports the expected rates from each calibrated sample that were calculated using the half life and the known activity of the standard sources. The characteristic energy peaks from each source was fit to a gaussian distribution. The variance from that fit was used to determine the range of integration for the count rate measurement. The integration energy range was selected to include counts over the energy interval from [math]\bar{E} - (2\sigma+\Delta \sigma)[/math] to [math]\bar{E} + (2\sigma+\Delta \sigma)[/math]. The measured rates for each calibrated source using the HpGe detector are listed in column 5. The efficiency given by the ratio of the rates in column 5 to the expected rates in column 4 are shown in column 6 of the same table. The percent efficiency of the standard sources was fit to a exponential curve shown in figureXX.YY. The singles efficiency for 898 keV and 1836.1 keV were extrapolated from the exponential equation and are recorded in tableXX.YY. This process was repeated for the NaI detector and the results can be seen in figureXX.YY and tablesXX.YY and XX.YY.

HpGe Detector

Run	Source	Energy	Expected rate (Hz)	HpGe Det B Rate (ADC 7) (Hz)	HpGe Eff (%)
7027	Na-22	511	1589.218 +/- 49.425	18.07 +/- 0.111	1.137 +/- 0.0317
7025	Cs-137	661.657	2548.03 +/- 80.008	25.468+/- 0.172	0.9995 +/- 0.0321
7029	Mn-54	834.848	41.405 +/- 1.300	0.485 +/- 0.014	1.171 +/- 0.0427
7026	Co-60	1173.228	1876.619 +/- 58.926	11.03 +/- 0.106	0.588 +/- 0.0328
7027	Na-22	1274.537	888.264 +/- 27.625	4.857 +/- 0.034	0.5468 +/- 0.0319
7026	Co-60	1332.492	1878.167 +/- 58.974	9.584 +/- 0.023	0.510 +/- 0.0315

  1  Constant     6.36491e-01   4.70444e-02   1.00271e-05  -3.28621e-03
  2  Slope       -9.81232e-04   5.68236e-05   1.21109e-08  -1.59453e+00

Extrapolated Efficiency

Source	Energy	HpGe Eff (%)
Y-88	898	0.7830 +/- 0.0471
Y-88	1836.1	0.3119 +/- 0.0310

NaI Detector

Run	Source	Energy	Expected rate (Hz)	NaI Det B Rate (ADC 7) (Hz)	NaI Eff (%)
7027	Na-22	511	1589.218 +/- 49.425	14.393 +/- 0.715	0.9057 +/- 0.0586
7025	Cs-137	661.657	2548.03 +/- 80.008	22.8 +/- 0.9154	0.8948 +/- 0.0510
7026	Co-60	1173.228	1876.619 +/- 58.926	7.529 +/- 0.5503	0.4012 +/- 0.0796
7027	Na-22	1274.537	888.264 +/- 27.625	3.654 +/- 0.5266	0.4114 +/- 0.1474
7026	Co-60	1332.492	1878.167 +/- 58.974	6.784 +/- 0.5345	0.3612 +/- 0.0848

Source	Energy	NaI Eff (%)
Y-88	898	0.6262 +/- 0.0246
Y-88	1836.1	0.2158 +/- 0.0466

Coincidence Efficiency

Unlike the singles efficiency found above, the efficiency for a coincidence measurement relies on the combined efficiency of both detectors. A known Y-88 source was not available to measure the coincidence activity directly. Instead, the HpGe's geometric efficiency for detecting the Y-88 energy lines at 898 keV and 1836.1 keV was combined with the NaI detectors efficiency for the 1836.1 and 898 keV lines respectively. For example, to obtain the 898 keV coincidence efficiency, the HpGe singles efficiency at this energy line is multiplied by the NaI singles efficiency at the 1836.1 keV line. The uncertainties of the singles efficiency are added in quadrature and the resulting values recorded in column 4 of tableXX.YY. The reverse case was applied to find the coincidence efficiency at the 1836.1 keV line and the values are listed in column 4 of tableXX.YY.

Source	HpGe Singles 898 keV Eff (%)	NaI Singles 1836.1 keV Eff (%)	HpGe Coincidence Eff (%)
Y-88	0.7830 +/- 0.0471	0.2158 +/- 0.0466	0.1690 +/- 0.0663

Source	HpGe Singles 1836.1 keV Eff (%)	NaI Singles 898 keV Eff (%)	HpGe Coincidence Eff (%)
Y-88	0.3119 +/- 0.0310	0.6262 +/- 0.0246	0.1953 +/- 0.0396

A_W_thesis