TD Ddoverd 2010

2/10/2010

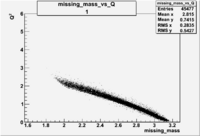
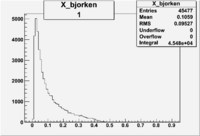
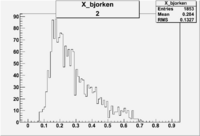
root file	reaction	[math]Q^2[/math]	W vs [math]Q^2[/math]	[math]F_{cup}[/math]	[math]X_b[/math]	[math]X_b\gt 0.3[/math]	# events for <1.232
1	B<0, pi^-=27 && e^-=11		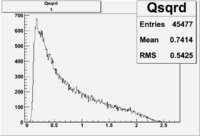				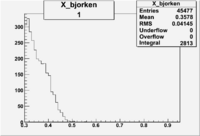9868
2	B>0, pi^+=27 && e^-=11		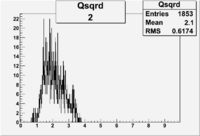		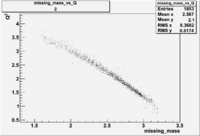		412
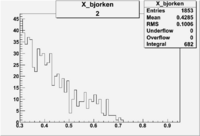
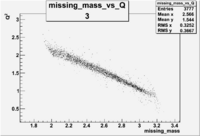
3	B>0, pi^+=27 && e^-=7		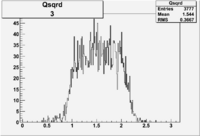				793
4	B>0, pi^-=7 && e^-=11						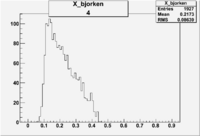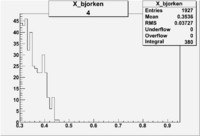400
5	B<0, pi^+=7 && e-=11		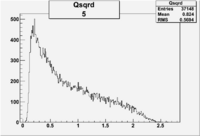		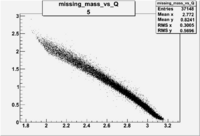		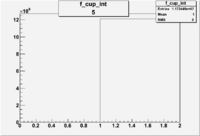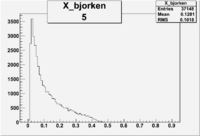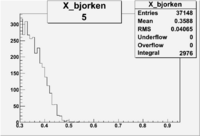9406

Rate differences

[math]R_{ep \rightarrow \pi^-X} \equiv \frac{B\lt 0, \pi^- = 27, e^- = 11}{B\gt 0, \pi^- = 7, e^- = 11} [/math]

Which paddle do we expect the Pion to hit if we flip the direction of the B-Field?

Only using Certain Paddles

[math]Ratio_1 = \frac{1(B\lt 0*\pi^-=27*e^-=11)}{2(B\gt 0*\pi^+=27*e^-=11)} = 24[/math]

[math]Ratio_2 = \frac{5(B\lt 0*\pi^+=7*e^-=11)}{4(B\gt 0*\pi^-=7*e^-=11)} = 19[/math]

[math]\frac{Ratio_1}{Ratio_2} = 1.2[/math]

or

[math]Ratio_3 = \frac{1(B\lt 0*\pi^-=27*e^-=11)}{5(B\lt 0*\pi^+=7*e^-=11)} = 1.2[/math]

[math]Ratio_4 = \frac{4(B\gt 0*\pi^-=7*e^-=11)}{2(B\gt 0*\pi^+=27*e^-=11)} = 1.03[/math]

Looking at Ratio_3 and Ratio_4 one can make a conclusion that we are detecting ~[math](11 \pm 8)[/math]% more [math]\pi^-[/math] type hadrons.

Choosing events Below 1.232 GeV && Certain paddle numbers

[math]Ratio_3 = \frac{1(B\lt 0*\pi^-=27*e^-=11)}{5(B\lt 0*\pi^+=7*e^-=11)} = \frac{9868}{9406} = 1.05 [/math]

[math]Ratio_4 = \frac{4(B\gt 0*\pi^-=7*e^-=11)}{2(B\gt 0*\pi^+=27*e^-=11)} = \frac{400}{412} = 0.97 [/math]

22-02-2010

• NH3 Target, two file lists: NH3Bn.list (B<0, 26994-26983) && NH3Bp.list (B>0, 27074-27079)

no paddle cuts

• A.) B>0

1.) B>0, [math]e^-_{PaddleNumber} = 7[/math] && [math]\pi^{+}_{PaddleNumber} = 27[/math], NH3Bp1_1.root

2.) B>0, [math]e^-_{PaddleNumber} = 7[/math] && [math]\pi^{-}_{PaddleNumber} = 7[/math] , NH3Bp2_1.root

• B.) B<0

1.) B<0, [math]e^-_{PaddleNumber} = 11[/math] && [math]\pi^{+}_{PaddleNumber} = 7[/math], NH3Bn1_1.root

2.) B<0, [math]e^-_{PaddleNumber} = 11[/math] && [math]\pi^{-}_{PaddleNumber} = 27[/math] , NH3Bn2_1.root

Paddle Cuts

Again, choosing events below 1.232 GeV, applying cuts, and plotting Histograms for certain electron and pion paddles.

[math]W[/math]_vs_[math]Q^2[/math], [math]Q^2[/math], Fcup && [math]x_B[/math].

• A.) B>0

1.) B>0, [math]e^-_{PaddleNumber} = 7[/math] && [math]\pi^{+}_{PaddleNumber} = 27[/math], NH3Bp1.root

2.) B>0, [math]e^-_{PaddleNumber} = 7[/math] && [math]\pi^{-}_{PaddleNumber} = 7[/math] , NH3Bp2.root

• B.) B<0

1.) B<0, [math]e^-_{PaddleNumber} = 11[/math] && [math]\pi^{+}_{PaddleNumber} = 7[/math], NH3Bn1.root

2.) B<0, [math]e^-_{PaddleNumber} = 11[/math] && [math]\pi^{-}_{PaddleNumber} = 27[/math] , NH3Bn2.root

File	[math]Q^2[/math]	W vs [math]Q^2[/math]	[math]F_{cup}[/math]	[math]X_b[/math]
NH3Bp1.root				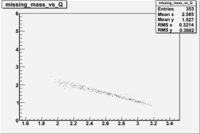200px
NH3Bp2.root		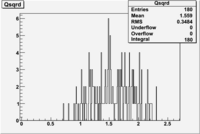		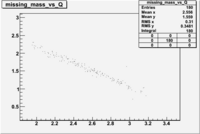200px
NH3Bn1.root		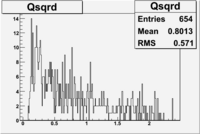		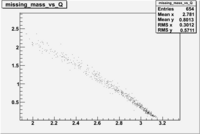200px
NH3Bn2.root		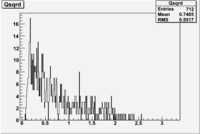		200px

Now you need to cut on [math]Q^2[/math].  The above suggests that looking at 1 < [math]Q^2[/math] < 2 GeV/c^2 may be a good starting point.

The idea is to compare the outbending (B<0) [math] \pi^-[/math] rate in paddle 27 to the inbending(B>0) [math]\pi^-[/math] rate in paddle 7 when 1 < [math]Q^2[/math] < 2. For the same kinematics the rates should be the same because the reaction is the same. Do the same for [math]\pi^+[/math] to see if it is consistent. This will show much flipping the magnet polarity impacts the rate measurement. Are the differences due to the B-field change or the scintillator efficiency, or to the track reconstruction? Our goal is to argue that the detector has the same efficiency for detecting [math]\pi^-[/math] and [math]\pi^+[/math] in the same scintillator when the Torus B-field direction is flipped.

1 < [math]Q^2[/math] < 2

File	W vs [math]Q^2[/math]	[math]F_{cupint}[/math]	[math]X_b[/math]
NH3Bp1.root	200px
NH3Bp2.root	200px
NH3Bn1.root	200px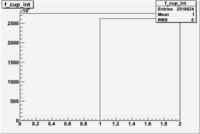
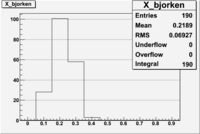
NH3Bn2.root	200px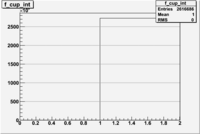

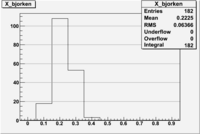
[math]X_{bj}[/math] bin	Bp1/Bn1	Bp2/Bn2
0.1	2.38 [math]\pm[/math] 0.299	1.09 [math]\pm[/math] 0.405
0.2	1.29 [math]\pm[/math] 0.188	3.59 [math]\pm[/math] 0.215
0.3	1.38 [math]\pm[/math] 0.242	4.6 [math]\pm[/math] 0.284
0.4	1.62 [math]\pm[/math] 1.02	3.76 [math]\pm[/math] 1.28

[math]\frac{B\gt 0 * \pi^+}{B\lt 0 * \pi^+} = \frac{0.0001}{0.00008} = 1.25 \pm 0.13[/math]

[math]\frac{B\gt 0 * \pi^-}{B\lt 0 * \pi^-} = \frac{0.0003255}{0.00006955} = 4.68 \pm 0.156 [/math]

Angle vs Paddle Number Distribution

#	[math]\theta[/math] angle vs paddle number	[math]\phi[/math] angle vs paddle number
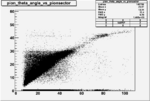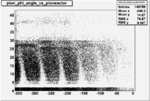
B>0, [math]\pi^+[/math] && [math]e^-[/math]
B>0, 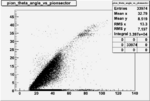[math]\pi^-[/math] && [math]e^-[/math]
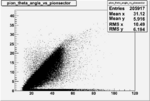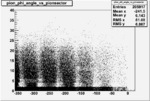
B<0, [math]\pi^+[/math] && [math]e^-[/math]
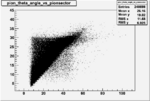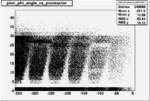
B<0, [math]\pi^-[/math] && [math]e^-[/math]

26/04/2010

NH3Bn positive pion runs

7.root - 27.root

pion paddle number	x_bj=0.1	x_bj=0.2	x_bj=0.3	x_bj=0.4
1	10 [math]\pm[/math] 3.16	38 [math]\pm[/math] 6.16	20 [math]\pm[/math] 4.47	2 [math]\pm[/math] 1.4
2	14 [math]\pm[/math] 3.7	60 [math]\pm[/math] 7.7	28 [math]\pm[/math] 5.3	0
3	20 [math]\pm[/math] 4.5	93 [math]\pm[/math] 9.6	48 [math]\pm[/math] 6.9	3 [math]\pm[/math] 1.7
4	26 [math]\pm[/math] 5.1	87 [math]\pm[/math] 9.3	53 [math]\pm[/math] 7.3	5 [math]\pm[/math] 2.2
5	24 [math]\pm[/math] 4.9	94 [math]\pm[/math] 9.7	42 [math]\pm[/math] 6.5	6 [math]\pm[/math] 2.4
6	30 [math]\pm[/math] 5.5	125 [math]\pm[/math] 1.1	79 [math]\pm[/math] 8.9	7[math]\pm[/math] 2.6
8	16 [math]\pm[/math] 4	102 [math]\pm[/math] 10	65 [math]\pm[/math] 8.1	4 [math]\pm[/math] 2
9	19 [math]\pm[/math] 4.3	96 [math]\pm[/math] 9.8	48 [math]\pm[/math] 6.9	3 [math]\pm[/math] 1.7
10	23 [math]\pm[/math] 4.8	98 [math]\pm[/math] 9.9	57 [math]\pm[/math] 7.5	2 [math]\pm[/math] 1.4
11	16 [math]\pm[/math] 4	71 [math]\pm[/math] 8.4	38 [math]\pm[/math] 6.2	2 [math]\pm[/math] 1.4
12	20 [math]\pm[/math] 4.5	85 [math]\pm[/math] 9.2	53 [math]\pm[/math] 7.3	6 [math]\pm[/math] 2.5
13	13 [math]\pm[/math] 3.6	73 [math]\pm[/math] 8.5	42 [math]\pm[/math] 6.5	5 [math]\pm[/math] 2.2
14	19 [math]\pm[/math] 4.3	75 [math]\pm[/math] 8.7	38 [math]\pm[/math] 6.2	3 [math]\pm[/math] 1.7
15	15 [math]\pm[/math] 3.9	62 [math]\pm[/math] 7.9	25 [math]\pm[/math] 5	2 [math]\pm[/math] 1.4
16	22 [math]\pm[/math] 4.7	58 [math]\pm[/math] 7.6	42 [math]\pm[/math] 6.5	0
17	5 [math]\pm[/math] 2.2	40 [math]\pm[/math] 6.3	23 [math]\pm[/math] 4.8	4 [math]\pm[/math] 2
18	10 [math]\pm[/math] 3.2	33 [math]\pm[/math] 5.7	18 [math]\pm[/math] 4.2	2 [math]\pm[/math] 1.4
19	7 [math]\pm[/math] 2.6	27 [math]\pm[/math] 5.2	21 [math]\pm[/math] 4.5	1 [math]\pm[/math] 1
20	3 [math]\pm[/math] 1.7	34 [math]\pm[/math] 5.8	13 [math]\pm[/math] 3.6	0
21	3 [math]\pm[/math] 1.7	16 [math]\pm[/math] 4	9 [math]\pm[/math] 3	0
22	2 [math]\pm[/math] 1.4	10 [math]\pm[/math] 3.2	2 [math]\pm[/math] 1.4	0
23	0	2 [math]\pm[/math] 1.4	3 [math]\pm[/math] 1.7	1 [math]\pm[/math] 1
24	0	1 [math]\pm[/math] 1	1 [math]\pm[/math] 1	0

NH3Bp positive pion runs

#p.root

pion paddle number	x_bj=0.1	x_bj=0.2	x_bj=0.3	x_bj=0.4
4	21 [math]\pm[/math]4.6	15 [math]\pm[/math] 3.9	2 [math]\pm[/math][math]1.4[/math]
5	39 [math]\pm[/math][math]6.2[/math]	58 [math]\pm[/math][math]7.6[/math]	18 [math]\pm[/math][math]4.2[/math]
6	56[math]\pm[/math] [math]7.5[/math]	91 [math]\pm[/math][math]9.5[/math]	49 [math]\pm[/math][math]7[/math]
7	90 [math]\pm[/math][math]9.5[/math]	110[math]\pm[/math] [math]11[/math]	82 [math]\pm[/math][math]9.2[/math]	5 [math]\pm[/math][math]2.2[/math]
8	81[math]\pm[/math] [math]9[/math]	155[math]\pm[/math] [math]12[/math]	78[math]\pm[/math] [math]8.8[/math]	3 [math]\pm[/math][math]1.7[/math]
9	68 [math]\pm[/math]8.1	139[math]\pm[/math] 12	85[math]\pm[/math] 9.2	4[math]\pm[/math] 2
10	83[math]\pm[/math] 9.1	181[math]\pm[/math] 13	88[math]\pm[/math] 9.4	8[math]\pm[/math] 2.8
11	102[math]\pm[/math] 10	164 [math]\pm[/math]13	96[math]\pm[/math] 9.8	8 [math]\pm[/math]2.8
12	103 [math]\pm[/math]10.1	188 [math]\pm[/math]13.7	122[math]\pm[/math] 11	8[math]\pm[/math] 2.8
13	85 [math]\pm[/math]9.2	203 [math]\pm[/math]14.2	132 [math]\pm[/math]11.5	16[math]\pm[/math] 4
14	105[math]\pm[/math] 10.2	219 [math]\pm[/math]14.8	120[math]\pm[/math] 10.9	11 [math]\pm[/math]3.3
15	116[math]\pm[/math] 10.8	192[math]\pm[/math] 13.8	113 [math]\pm[/math]10.6	8 [math]\pm[/math]2.8
16	91 [math]\pm[/math]9.5	208[math]\pm[/math] 14.4	134[math]\pm[/math] 11.6	9[math]\pm[/math] 3
17	98 [math]\pm[/math]9.9	187[math]\pm[/math] 13.7	112[math]\pm[/math] 10.6	9[math]\pm[/math] 3
18	106 [math]\pm[/math]10.3	159 [math]\pm[/math]12.6	121[math]\pm[/math] 11	7 [math]\pm[/math]2.6
19	91 [math]\pm[/math]9.5	166 [math]\pm[/math]12.9	107[math]\pm[/math] 10.3	9[math]\pm[/math] 3
20	83 [math]\pm[/math]9.1	138 [math]\pm[/math]11.7	90 [math]\pm[/math]9.5	7[math]\pm[/math] 2.6
21	81[math]\pm[/math] 9	167 [math]\pm[/math]12.9	110[math]\pm[/math] 10.5	11 [math]\pm[/math]3.3
22	87 [math]\pm[/math]9.3	164 [math]\pm[/math]12.8	84[math]\pm[/math] 9.2	5[math]\pm[/math] 2.2
23	77 [math]\pm[/math]8.8	98[math]\pm[/math] 9.9	73[math]\pm[/math] 8.5	6 [math]\pm[/math]2.4
24	86[math]\pm[/math] 9.3	131[math]\pm[/math] 11.4	81[math]\pm[/math] 9	6[math]\pm[/math] 2.4
25	96 [math]\pm[/math]9.8	185[math]\pm[/math] 13.6	92 [math]\pm[/math]9.6	14[math]\pm[/math] 3.7
26	87 [math]\pm[/math]9.3	147 [math]\pm[/math]12.1	97 [math]\pm[/math]9.8	8 [math]\pm[/math]2.8
27	72[math]\pm[/math] 8.5	132 [math]\pm[/math]11.5	82[math]\pm[/math] 9.1	6 [math]\pm[/math]2.4
28	64[math]\pm[/math] 8	131 [math]\pm[/math]11.4	73 [math]\pm[/math]8.5	10[math]\pm[/math] 3.2
29	46[math]\pm[/math] 6.9	101[math]\pm[/math] 10	53 [math]\pm[/math]7.5	2[math]\pm[/math] 1
30	13[math]\pm[/math] 3.5	14 [math]\pm[/math]3.8	18 [math]\pm[/math]4.8	1 [math]\pm[/math]1

06-02-2010

Electron Efficiency

Chosen electron paddles are following for the positive and negative paddles respectively: 7 and 11.

electroneffbp.root &&  electroneffbn.root

B>0

TH1.Print Name  = Qsqrd, Entries= 323638, Total sum= 323634
fSumw[0]=0, x=-0.2
fSumw[1]=0, x=-0.1
fSumw[2]=0, x=9.71039e-18
fSumw[3]=0, x=0.1
fSumw[4]=3, x=0.2
fSumw[5]=21, x=0.3
fSumw[6]=93, x=0.4
fSumw[7]=285, x=0.5
fSumw[8]=575, x=0.6
fSumw[9]=1148, x=0.7
fSumw[10]=2478, x=0.8
fSumw[11]=9402, x=0.9
fSumw[12]=24380, x=1
fSumw[13]=31648, x=1.1
fSumw[14]=29967, x=1.2
fSumw[15]=27864, x=1.3
fSumw[16]=27157, x=1.4
fSumw[17]=26820, x=1.5
fSumw[18]=25603, x=1.6
fSumw[19]=25027, x=1.7
fSumw[20]=24470, x=1.8
fSumw[21]=23512, x=1.9
fSumw[22]=20320, x=2
fSumw[23]=13453, x=2.1
fSumw[24]=6738, x=2.2
fSumw[25]=2043, x=2.3
fSumw[26]=449, x=2.4
fSumw[27]=119, x=2.5
fSumw[28]=41, x=2.6
fSumw[29]=13, x=2.7
fSumw[30]=5, x=2.8
fSumw[31]=4, x=2.9

B<0

TH1.Print Name = Qsqrd, Entries= 716018, Total sum= 716011

fSumw[0]=0, x=-0.2
fSumw[1]=0, x=-0.1
fSumw[2]=180, x=9.71039e-18
fSumw[3]=112762, x=0.1
fSumw[4]=160348, x=0.2
fSumw[5]=91665, x=0.3
fSumw[6]=62692, x=0.4
fSumw[7]=46135, x=0.5
fSumw[8]=35169, x=0.6
fSumw[9]=28473, x=0.7
fSumw[10]=23810, x=0.8
fSumw[11]=20763, x=0.9
fSumw[12]=18452, x=1
fSumw[13]=16021, x=1.1
fSumw[14]=14494, x=1.2
fSumw[15]=12731, x=1.3
fSumw[16]=11610, x=1.4
fSumw[17]=10422, x=1.5
fSumw[18]=9437, x=1.6
fSumw[19]=8929, x=1.7
fSumw[20]=8149, x=1.8
fSumw[21]=7504, x=1.9
fSumw[22]=6209, x=2
fSumw[23]=4557, x=2.1
fSumw[24]=2948, x=2.2
fSumw[25]=1625, x=2.3
fSumw[26]=659, x=2.4
fSumw[27]=195, x=2.5
fSumw[28]=50, x=2.6
fSumw[29]=18, x=2.7
fSumw[30]=4, x=2.8
fSumw[31]=7, x=2.9

Electron Eff Result

Inclusive detected electrons -vs- Q-squared	Inclusive Missing Mass (W) for 1.0 Q^2 <1.2
The ratio of inclusive electrons detected in scintillator paddle #7 when Btorus >0 (B_p)to inclusive electrons detected by paddle 11 when B<0(B_n)	The inclusive missing mass W for each torus setting. Dashed line is B>0 and solid line is B<0

Media:electronefficiencyratioBp7Bn11.txt

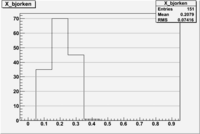
Now plot efficiency as function of X_{BJ} and W < 1232 and require pion.

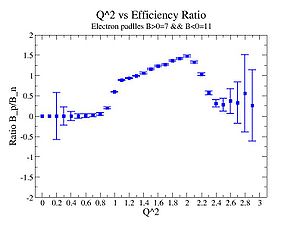
Positive Pion Efficiency dependence on [math]x_bj[/math]

Pion and electron both required.

W<1232 and [math]Q^2=1.1 GeV^2[/math]

Now cut on Q^2 where the inclusive electron rates are the same with both torus settings and then require at least one positive pion.

X_bj	B_n/B_p Rates
0.14	0.25 [math]\pm[/math] 0.55
0.15	0.74 [math]\pm[/math] 0.27
0.17	1.07 [math]\pm[/math] 0.18
0.19	1.3 [math]\pm[/math] 0.13
0.2	1.4 [math]\pm[/math] 0.14

There appears to be a region around X_{Bj}= 0.2 which has the same number of pions detected for both torus settings.

Negative Pion Efficiency dependence on [math]Q^2[/math]

Pion and electron both required(e_sector=7 for B>0 && e_sector=11 for B<11).

Now cut on Q^2 where the inclusive electron rates are the same with both torus settings and then require at least one positive pion.

[math]Q^2[/math]	B_p/B_n Rates
0.2	0.004 [math]\pm[/math]0.55
0.3	0.017 [math]\pm[/math] 0.5
0.4	0.069[math]\pm[/math] 0.6
0.5	0.0262[math]\pm[/math] 0.6
0.6	0.039[math]\pm[/math] 0.5
0.7	0.1 [math]\pm[/math]0.64
0.8	0.055[math]\pm[/math] 0.52
0.9	0.259[math]\pm[/math] 0.52
1	1.232[math]\pm[/math] 0.52
1.1	3.96[math]\pm[/math] 0.8

There DOES NOT appears to be a region which has the same number of negative pions detected for both torus settings.

What is wrong?

8/13/10

1.) Change Osipenko cuts to maximize electrons when B <0 but still minimize impact of negative pion contamination. Look at effects on Npe distribution.

Insert current number of electron events that are removed by the Osipenko cut for B<0. Compare it to event removed by other cuts.

2.) Schedule Prelim exam.

Shropshire has replaced cole.

Members are: Forest, Fisher, Shropshire, Dale, Tatar(?)

Ask Dustin McNulty

1.) [1]

"Original cut parameters generated by Osipenko et.al. were not very efficient for especially outbending data of eg1b experiment. The loss of electrons was substantial. For inbending data, loss of electrons were at acceptable level. To gain some electrons back we generated new cut parametes that will specifically work better for outbending data. Also we slightly adjusted the cut parameters for inbending data for some sectors and segments."

2.) Upgraded Proposal defense presentation(includes event display) File:TamarProposalP 1.pdf

11/10/10

DTS files used for analysis.

Media:ND3Bn.txt
Media:ND3Bp.txt
Media:NH3Bn.txt
Media:NH3Bp.txt

positive

negative

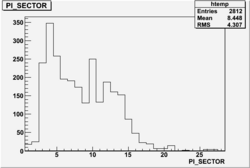
What are the cuts for the above histogram?

 pion, OSI, EC

11/30/10

DST ntuple suggestions

1. Event number and run number should be recorded. Run number is in RUNINFO. Event number should be in the event packet.
2. Create variable called helicity and fill it with absolute helicity.

GEM detector

1. order mylar and copper tape., 1" wide, $20 worth of each
2. check DPO 4104 see if working properly
3. find 10 frames for Qweak GEM foils (or order more)
4. check gas supply

12/6/10

DST ntuple

Run number looks good.

PbPt values are all non-zero now.

1) Some of the TORUS values are zero when they should be positive?

Doesnt matter. There are totally four files: ND3_target+Torus_positive, ND3_target+Torus_negative, NH3_target+Torus_positive and NH3_target+Torus_negative. So not an issue.

2.) Electrons have less than 0.25 GeV energy?

Still dont know.

3.) ASYM=HWP*LINAC*P_T is in the root file.

4.) Where are the FC normalization histograms

Delta_D_over_D

12/20/10

DST

1.) Looks like a particle ID problem for outbending (B<0) negative pions.

Calculate the missing mass for the and stor it in M_X. (included and corrected in NTUPLE)

Redo all the ntuples.

All ntuples are done.

2.) is run 27048 OK (no target run(empty)), corrected for both target runs

3.) Current quarks(the core of the constituent quark without the gluons and sea quarks(covering). The mass of the current quarks(up and down) is 5-10 MeV), light cone?

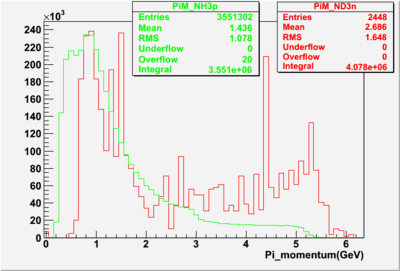
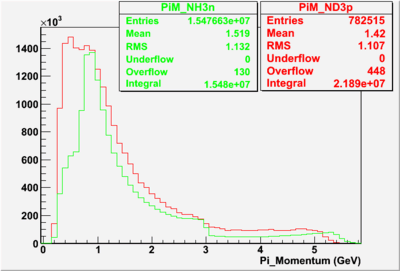
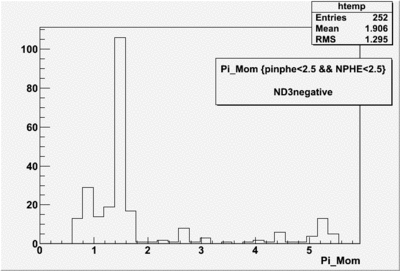
pion momentum for different torus settings and targets

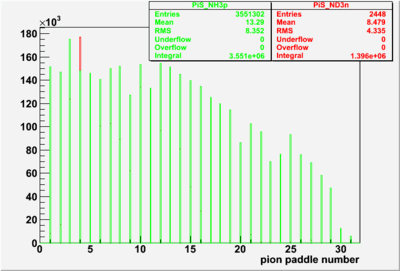
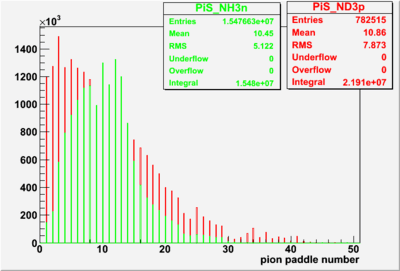
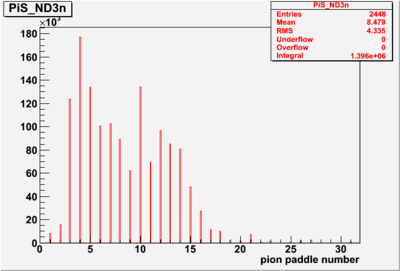
pion paddle number for different torus settings

Need a before and after cuts histogram with stats to see number of events dropped

DeltaDoverD_Progress