Difference between revisions of "Forest Relativity Notes"
Jump to navigation
Jump to search
| Line 62: | Line 62: | ||
== Proper Length== | == Proper Length== | ||
| − | ; Proper Length<math> (c\Tau)</math>: | + | ; Proper Length<math> (c\Tau)</math>: The length of an object in the object's rest frame. |
=Invariant Length= | =Invariant Length= | ||
Revision as of 17:06, 30 October 2007
Lorentz Transformations
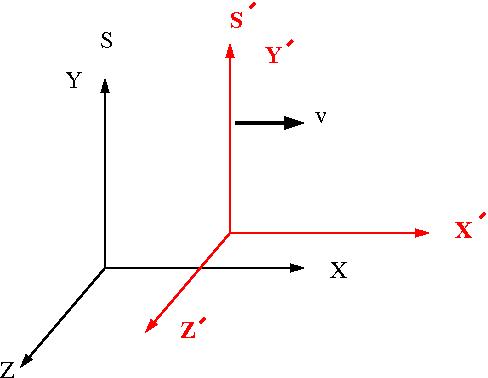
The picture below represents the relative orientation of two different coordinate systems . is at rest (Lab Frame) and is moving at a velocity v to the right with respect to frame .
The relationship between the coordinate of an object in frame to the same object described using the coordinates in frame is geven by the Lorentz transformation:
where
- example
- Or in matrix form the tranformation looks like
- Note
- Einstein's summation convention drops the symbols and assumes it to exist whenever there is a repeated subscript and uperscript
- ie;
- in the example above the symbol is repeated thereby indicating a summation over .
Trig Method
Another way to represent the lorentz transformation is by using the substitution
- The Matrix form pf the tranformation looks like
- Or the reverse transformation
- Notice that you just needed to change the signs for the inverse matrix
Proper Time and Length
Proper Time
- Proper Time
- The time measured in the rest frame of the clock. The time interval is measured at the same x,y,z coordinates because the clock chose is in a frame which is not moving (rest frame).
The time given in any frame (t) =
- Note
- since you expect the Proper time interval to be the smallest
Proper Length
- Proper Length
- The length of an object in the object's rest frame.