Determing Elliptical Equations

$\textbf{\underline{Navigation}}$

$\vartriangleleft$ $\triangle$ $\vartriangleright$

Determining Elliptical Equation

As found earlier

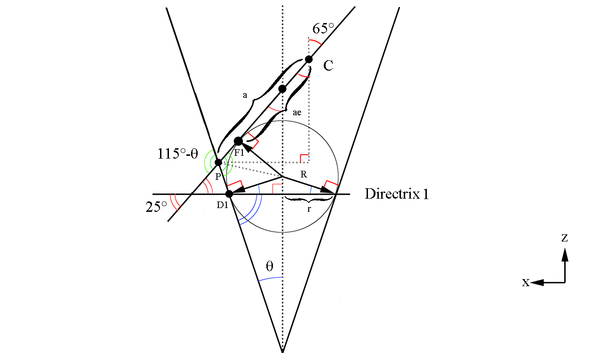
Figure 5: A cone of constant Theta with varying Phi.

$CP_x=a\ sin(65^{\circ})$

$CP_z=a\ cos(65^{\circ})$

Given the center point coordinates found earlier:

$c \equiv (-\Delta x, 0,z+\Delta z)$

$c \equiv (-\Delta a\ sin(65^{\circ}), 0,z+\Delta a\ cos(65^{\circ}))$

This gives the location of P($\phi=0$) at

$P(\phi=0) \equiv (-\Delta a\ sin(65^{\circ})+a\ sin((65^{\circ}), 0,z+\Delta a\ cos(65^{\circ})-a\ cos(65^{\circ}))$

$P(\phi=0) \equiv (-\frac{a_2-a_1}{2} sin(65^{\circ})+\frac{a_1+a_2}{2} sin((65^{\circ}), 0,2.53+\frac{a_2-a_1}{2} cos(65^{\circ})-\frac{a_1+a_2}{2} cos(65^{\circ}))$

The height in z can be viewed as a linear function of x and expressed in slope intercept form for a plane:

$z_{Plane\ of\ sector}=m_{plane}x+b_{plane}$

where

$m_{plane}=\frac{\Delta z}{\Delta x}=\frac{a\ cos(65^{\circ})}{-a\ sin(65^{\circ})}=-cot(65^{\circ})$

$b_{plane}\equiv (0,0,2.53)$

The D1 and D2 components can be obtained by finding the x and y components of the two directrix circles, which will be a function of their respective radii and the angle phi around the z axis.

$x_{Lower\ Directrix}^2+y_{Lower\ Directrix}^2=r_{Lower\ Directrix}^2\equiv r_{Lower\ Directrix}^2 cos^2(\phi)+r_{Lower\ Directrix}^2 sin^2(\phi)$

Solving for the cartesian components,

$x_{Lower\ Directrix}=r_{Lower\ Directrix}\ cos(\phi)\qquad \qquad \qquad y_{Lower\ Directrix}=r_{Lower\ Directrix}\ sin(\phi)$

Similarly,

$x_{Upper\ Directrix}=r_{Upper\ Directrix}\ cos(\phi)\qquad \qquad \qquad y_{Upper\ Directrix}=r_{Upper\ Directrix}\ sin(\phi)$

Using the radius information found earlier for the upper and lower Dandelin spheres and directrix,

$R_{Lower\ Dandelin}=(ae-\Delta a) tan(65^{\circ})\qquad \qquad \qquad R_{Upper\ Dandelin}=(ae+\Delta a) tan(65^{\circ})$

$r_{D1}=R_{Lower\ Dandelin}cos(\theta)=(ae-\Delta a) tan(65^{\circ})sec(\theta)\qquad \qquad\ \qquad r_{D2}=R_{Upper\ Dandelin}cos(\theta)=(ae+\Delta a) tan(65^{\circ})cos(\theta)$

$r_{D1}=(\frac{a_1+a_2}{2}\frac{sin(25^{\circ})}{cos(\theta)}-\frac{a_2-a_1}{2}) tan(65^{\circ})cos(\theta)\qquad \qquad \qquad r_{D2}=(\frac{a_1+a_2}{2}\frac{sin(25^{\circ})}{sec(\theta)}+\frac{a_2-a_1}{2}) tan(65^{\circ})cos(\theta)$

The height to the first directrix circle is

$z_{D1}=r_{D1}\ cot(\theta)$

The height to the second directrix circle is

$z_{D2}=r_{D2}\ cot(\theta)$

This gives the components for a point on the directrix circles as

$x_{D1}=(\frac{a_1+a_2}{2}\frac{sin(25^{\circ})}{cos(\theta)}-\frac{a_2-a_1}{2}) tan(65^{\circ})cos(\theta) cos(\phi)\qquad \qquad y_{D1}=(\frac{a_1+a_2}{2}\frac{sin(25^{\circ})}{cos(\theta)}-\frac{a_2-a_1}{2}) tan(65^{\circ})cos(\theta) sin(\phi)\qquad \qquad z_{D1}=r_{D1}\ cot(\theta)$

$x_{D2}=(\frac{a_1+a_2}{2}\frac{sin(25^{\circ})}{cos(\theta)}+\frac{a_2-a_1}{2}) tan(65^{\circ})cos(\theta) cos(\phi)\qquad \qquad y_{D2}=(\frac{a_1+a_2}{2}\frac{sin(25^{\circ})}{cos(\theta)}+\frac{a_2-a_1}{2}) tan(65^{\circ})cos(\theta) sin(\phi)\qquad \qquad z_{D2}=r_{D2}\ cot(\theta)$

Using these two points, which will be at the same angle phi around the z axis, a line can be created

$\vec d=r_{D2}cos(\phi)-r_{D1}cos(\phi)\ \hat x+r_{D2}sin(\phi)-r_{D1}sin(\phi)\ \hat y+r_{D2}cot(\theta)-r_{D1}cot(\theta)\ \hat z$

Since this line is composed of the difference in the cartesian components of the two points it acts as a direction vector pointing from one point to the next. We can use the fact that a line in space is simply the intersection of two planes which in this situation results in the direction vector. Finding the parametric form equation of this line

$(x,y,z)=(r_{D1}cos(\phi),\ r_{D1}sin(\phi),\ r_{D1}cot(\theta))+t(r_{D2}cos(\phi)-r_{D1}cos(\phi),\ r_{D2}sin(\phi)-r_{D1}sin(\phi),\ r_{D2}cot(\theta)-r_{D1}cot(\theta))$

Solving for the individual cartesian components

$x=r_{D1}cos(\phi)+t(r_{D2}cos(\phi)-r_{D1}cos(\phi))\qquad \qquad y=r_{D1}sin(\phi)+t(r_{D2}sin(\phi)-r_{D1}sin(\phi))\qquad \qquad z=r_{D1}cot(\theta)+t(r_{D2}cot(\theta)-r_{D1}cot(\theta))$

$\Rightarrow t=\frac{x-r_{D1}cos(\phi)}{r_{D2}cos(\phi)-r_{D1}cos(\phi)}=\frac{y-r_{D1}sin(\phi)}{r_{D2}sin(\phi)-r_{D1}sin(\phi)}=\frac{z-r_{D1}cot(\theta)}{r_{D2}cot(\theta)-r_{D1}cot(\theta)}$

Since this condition implies that the 3 equations listed above for all conditions, we can solve for the $\phi=0$ situation which must then hold for all other instances of $\phi$

$\frac{x-r_{D1}cos(\phi)}{r_{D2}cos(\phi)-r_{D1}cos(\phi)}=\frac{z-r_{D1}cot(\theta)}{r_{D2}cot(\theta)-r_{D1}cot(\theta)}$

$\frac{x-r_{D1}cos(\phi)}{(r_{D2}-r_{D1})cos(\phi)}=\frac{z-r_{D1}cot(\theta)}{(r_{D2}-r_{D1})cot(\theta)}$

$\frac{(x-r_{D1}cos(\phi))(r_{D2}-r_{D1})cot(\theta)}{(r_{D2}-r_{D1})cos(\phi)}+r_{D1}cot(\theta)=z$

Using the condition that on the plane

$z_{plane}=-cot(65^{\circ})x+2.53$

We can substitute for z to find the point of intersection for the plane and cone at a given $\theta$ and $\phi$

$\frac{ \left( x_P-r_{D1}cos(\phi) \right) cot(\theta)}{cos(\phi)}+r_{D1}cot(\theta)=-cot(65^{\circ})x_P+2.53$

$\left ( \frac{x_P}{cos(\phi)}+r_{D1}-r_{D1} \right )cot(\theta )=-cot(65^{\circ})x_P+2.53$

$\left ( \frac{x_P}{cos(\phi)} \right )cot(\theta )+cot(65^{\circ})x_P=2.53$

$\frac{x_P\ cot(\theta )}{cos(\phi)}+cot(65^{\circ})x_P=2.53$

$x_P \left (\frac{ cot(\theta)+cos(\phi)cot(65^{\circ})}{cos(\phi)}\right )=2.53$

$x_P(cot(\theta)+cos(\phi)cot(65^{\circ})=2.53cos(\phi)$

$x_P=\frac{2.53cos(\phi)}{(cot(\theta)+cos(\phi)cot(65^{\circ})}$

$\Rightarrow t=\frac{x-r_{D1}cos(\phi)}{r_{D2}cos(\phi)-r_{D1}cos(\phi)}=\frac{y-r_{D1}sin(\phi)}{r_{D2}sin(\phi)-r_{D1}sin(\phi)}=\frac{z-r_{D1}cot(\theta)}{r_{D2}cot(\theta)-r_{D1}cot(\theta)}$

$\frac{x_P-r_{D1}cos(\phi)}{r_{D2}cos(\phi)-r_{D1}cos(\phi)}=\frac{y-r_{D1}sin(\phi)}{r_{D2}sin(\phi)-r_{D1}sin(\phi)}$

$(r_{D2}sin(\phi)-r_{D1}sin(\phi))\frac{(x_P-r_{D1}cos(\phi))}{r_{D2}cos(\phi)-r_{D1}cos(\phi)}=y_P-r_{D1}sin(\phi)$

$(r_{D2}-r_{D1})sin(\phi)\frac{(x_P-r_{D1}cos(\phi))}{(r_{D2}-r_{D1})cos(\phi)}=y_P-r_{D1}sin(\phi)$

$sin(\phi)\frac{(x_P-r_{D1}cos(\phi))}{cos(\phi)}=y_P-r_{D1}sin(\phi)$

$y_P=sin(\phi)\frac{(x_P-r_{D1}cos(\phi))}{cos(\phi)}+r_{D1}sin(\phi)$

$y_P=tan(\phi)x_P-r_{D1}sin(\phi)+r_{D1}sin(\phi)$

$y_P=tan(\phi)x_P-r_{D1}sin(\phi)+r_{D1}sin(\phi)$

$y_P=tan(\phi)\left (\frac{2.53cos(\phi)}{(cot(\theta)+cos(\phi)cot(65^{\circ})}\right )$

$y_P=\frac{2.53sin(\phi)}{(cot(\theta)+cos(\phi)cot(65^{\circ})}$

$z_P=\frac{(x_P-r_{D1}cos(\phi))(r_{D2}-r_{D1})cot(\theta)}{(r_{D2}-r_{D1})cos(\phi)}+r_{D1}cot(\theta)$

$z_P=\frac{(x_P)cot(\theta)}{cos(\phi)}$

$z_P=\frac{(\frac{2.53cos(\phi)}{(cot(\theta)+cos(\phi)cot(65^{\circ})})cot(\theta)}{cos(\phi)}$

$z_P=\frac{2.53cot(\theta)}{(cot(\theta)+cos(\phi)cot(65^{\circ})}$

---

$\textbf{\underline{Navigation}}$

$\vartriangleleft$ $\triangle$ $\vartriangleright$