Difference between revisions of "A W thesis"

From New IAC Wiki
Jump to navigation Jump to search
Line 201: Line 201:
 
| 105 || 0.98 ||coin ||<math>\pm  7.042e-4</math>|| <math> {+1.6e-5} \atop { -6.3e-5}</math>  ||<math> {+7.358e-6} \atop { -7.092e-6}</math>||<math>\pm  0.00018</math> ||<math> {+5.68e-4} \atop { -1.75e-3}</math> ||<math> {+0.0013} \atop { -0.0025}</math>
 
| 105 || 0.98 ||coin ||<math>\pm  7.042e-4</math>|| <math> {+1.6e-5} \atop { -6.3e-5}</math>  ||<math> {+7.358e-6} \atop { -7.092e-6}</math>||<math>\pm  0.00018</math> ||<math> {+5.68e-4} \atop { -1.75e-3}</math> ||<math> {+0.0013} \atop { -0.0025}</math>
 
|-
 
|-
−
| 156 || 1.46 ||coin ||<math>\pm  6.383e-4</math>|| <math> {+1.5e-5} \atop { -1.3e-5}</math> || +2.004e-6, -1.990e-6 || + 7.611e-5, -7.605e-5 || <math> {+4.03e-4} \atop { -6.22e-4}</math> ||
+
| 156 || 1.46 ||coin ||<math>\pm  6.383e-4</math>|| <math> {+1.5e-5} \atop { -1.3e-5}</math> ||<math> {+2.004e-6} \atop { -1.990e-6}</math> || <math> {+7.611e-5} \atop { -7.605e-5}</math>|| <math> {+4.03e-4} \atop { -6.22e-4}</math> ||<math> {+0.0011} \atop { -0.0013}</math>
 
|-
 
|-
−
| 226 || 2.21 ||coin ||<math>\pm  6.912e-4</math>|| <math> {+2.0e-5} \atop { -5.9e-5}</math> || +8.041e-6, -7.859e-6 || +/- 0.00013 || <math> {+4.92e-4} \atop { -1.06e-3}</math> ||
+
| 226 || 2.21 ||coin ||<math>\pm  6.912e-4</math>|| <math> {+2.0e-5} \atop { -5.9e-5}</math> ||<math> {+8.041e-6} \atop { -7.859e-6}</math>|| <math>\pm  0.00013</math>|| <math> {+4.92e-4} \atop { -1.06e-3}</math> ||<math> {+0.0012} \atop { -0.0018}</math>
 
|-
 
|-
−
| 242 || 2.27 ||coin ||<math>\pm  2.179e-4</math> || <math> {+2.0e-6} \atop { -4e-6}</math> || +4.436e-6, -4.365e-6 || +/- 1.078e-5 || <math> {+3.63e-4} \atop { -1.52e-3}</math> ||
+
| 242 || 2.27 ||coin ||<math>\pm  2.179e-4</math> || <math> {+2.0e-6} \atop { -4e-6}</math> ||<math> {+4.436e-6} \atop { -4.365e-6}</math>|| <math>\pm  1.078e-5</math>|| <math> {+3.63e-4} \atop { -1.52e-3}</math> ||<math> {+5.8e-4} \atop { -0.0017}</math>
 
|-
 
|-
 
|}
 
|}

Revision as of 18:38, 10 September 2014

Introduction

Methods of determining atomic concentration in Material

Neutron Activation Analysis (NAA)

Neutron activation analysis (NAA) is a high-sensitivity and non-destructive multi-elemental analytical technique used for analysis of major, minor, and trace elements in samples. NAA is significantly different from other spectroscopic analytical techniques in that it is based on nuclear transitions rather than electronic transitions. The basic essentials to carry out an analysis of samples by NAA are a source of neutrons to bombard the sample, instruments suitable for detecting gamma rays, and knowledge of the reactions that occur when neutrons interact with target nuclei.

The sequence of events taking place during the most common type of nuclear reaction for NAA, namely neutron capture, is illustrated in the figure. (make figure) Upon irradiation, a neuron interacts with the target nucleus via a non-elastic collision. A compound nucleus forms in an excited and usually unstable state. This unfavourable state will almost instantaneously de-excite into a more stable configuration by emitting one or more characteristic prompt gamma rays. In most cases, this new and stable configuration yields a radioactive nucleus. The newly formed radioactive nucleus now decays by the emission of one of more characteristic delayed gamma rays. This decay process is at a much slower rate according to unique half-life of the radioactive nucleus. Measurement, in principle, falls into two categories: (1) prompt gamma-ray neutron activation analysis (PGNAA), where measurements are taken during the irradiation of a sample, or (2) the more common delayed gamma-ray neutron activation analysis (DGNAA), where measurements follow radioactive decay.

A range of different neutron sources can be used for NAA. These include reactors, accelerators, fusors, and radioisotopic neutron emitters. Since they have high fluxes of neutrons from uranium fission, nuclear reactors offer the highest available sensitivities for most elements.

There are several types of detectors and configurations employed in NAA. Most are designed to detect the emitted gamma radiation. The instrumentation most commonly used consists of scintillation type or semiconductor type detector(s), associated electronics, and a computer-based, multi-channel analyser. Scintillation type detectors use a radiation-sensitive crystal, usually thallium-doped sodium iodide (NaI(Tl)). Hyperpure or intrinsic germanium (HpGe) detectors that operate at liquid nitrogen temperatures (77 degrees K) are the semiconductor type most commonly operated for NAA.

There are a few drawbacks to the use of NAA. Even though the technique is non-destructive, the irradiated sample will remain radioactive for great lengths of time depending on the half-lives, requiring handling and disposal protocols. Also, not all labs have convenient access to a suitable reactor for a neutron source to irradiate a sample. As with any other analytical method, NAA is also not universal. For instance, the determination of low-Z elements, such as C, N, O, F, or several other elements such as Mg, Si, Ca, Ti, Ni, Sr, Y, Zr, Nb, Sn, and Tl, is not sufficiently sensitive or impossible.

Inductively coupled plasma mass spectrometry(ICP-MS)

Inductively coupled plasma atomic emission spectroscopy(ICP-OES)

Atomic Absorption Spectrometry (multi-element AAS)

Particle-induced X-ray Emission (PIXE)

Another method, uses X-ray from a synchrotron light source and look at the de-excitation of atomic electrons to measure the atomic number. Reports are that they can measure pico-gram quantities.

table of detection limits -vs- Method

Coincidence Counting Setup

A_W_CAA_apparatus

Y-88 CAA

Run6980_Y88

Run7023_Y88

Run7108_Y88

Run7161_Y88

Run7204_Y88

Run7236_Y88



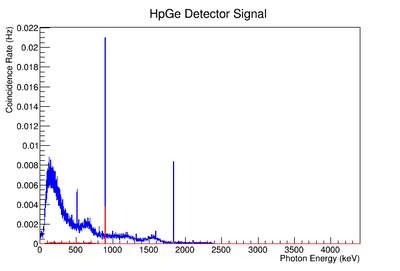
Run7023 Y88 scaled.png Run7023 OverlayCoin Cuts.png

Run7023 OverlayCoin Cuts log.png

Using our Y-88 source, our set-up allows us to perform runs with the detectors in coincidence (AND mode) or singles (OR mode). The figure on the left shows the HpGe detector's coincidence events (red) that occurred within a 200 ns timing window. This graph has been overlaid with the same detector’s single events (blue). We can see that by having the detectors in coincidence, the noise is reduced and several peaks, which do not have multiple photons in coincidence, are removed.

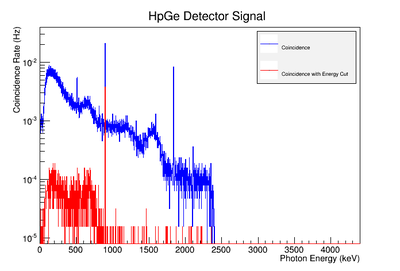
The coincidence run does not show the two energy peaks associated with Y-88 decay alone. Even though we require coincidence for the system to trigger, there is still noise. These peaks could be Compton events contaminating the coincidence and accidentals. Photons can loss a portion of their energy traveling to the detector. They do not necessarily have to deposit all of their energy into the detector either. The photon can Compton scatter out. It is not possible to tell the difference between a photon that deposited all of its energy from a scattered photon, since the speed of light is so fast and the resolution of the detector so poor. The photons can, however, be distinguished if an energy cut is applied. In order to remove the Compton events and the accidentals, we can require the photon energy to be completely deposited into the detector. This can be achieved by placing an energy cut on the NaI detector around the high energy Y-88 peak. This will remove other unwanted photon energies. The graph on the right is an overlay of the coincidence signal before (blue) and after (red) an energy cut is applied to the companion detector (NaI detector). The noise seen at low energies is greatly reduced after including the cut and improved the signal.



Background subtraction

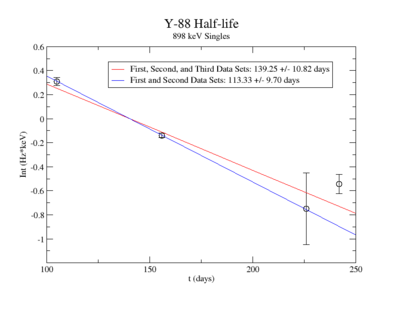
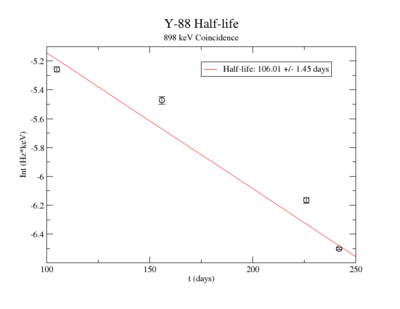
898 keV analysis

The raw Histograms and fits may be found here A_W_thesis_old#898_keV_signal_table

The Measured half life is

Y-88 Half-life

Days [math]T_{1/2}[/math] Trig Integral Statistical Error Systematic Error
105 0.98 sing 1.364 [math]\pm [/math] [math] {+0.2974} \atop { -0.1759}[/math]
coin 0.8414 [math]\pm [/math] [math]\pm 0.1039[/math]
156 1.46 sing 0.8697 [math]\pm [/math] [math] {+0.3758} \atop { -0.3622}[/math]
coin 0.5015 [math]\pm [/math] [math] {+0.0548} \atop { -0.0586}[/math]
226 2.12 sing 0.4733 [math]\pm [/math] [math] {+0.2335} \atop { -0.2546}[/math]
coin 0.2822 [math]\pm [/math] [math] {+0.0769} \atop { -0.0880}[/math]
242 2.27 sing 0.5807 [math]\pm [/math] [math] {+0.1693} \atop { -0.2118}[/math]
coin 0.2449 [math]\pm [/math] [math]\pm 0.059[/math]

Y-88 Halflife 898 custom.pngY-88 Halflife 898 coin nocuts.png

The signal to noise ratio is

SNR 898.png


N_0 = xxxx

When SNR = 0 then t= 301.1 days (s) and 343.3 days (c)

N_{sing} = ZZZZ = lowest measurable activity using singles

N_{conic} = AAAA = lowest measurable activity using conic

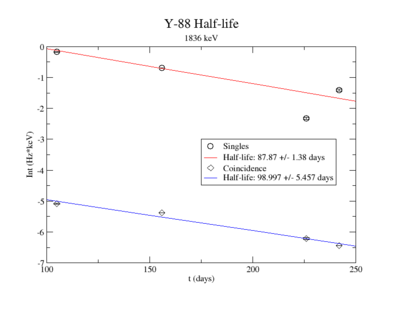
1836.1 Analysis

The raw Histograms and fits may be found here A_W_thesis_old#1836_keV_signal_table

The Measured half life is

Y-88 Half-life

Days [math]T_{1/2}[/math] Trig Integral Statistical Error Systematic Error
105 0.98 sing
coin
156 1.46 sing
coin
226 2.12 sing
coin
242 2.27 sing
coin

Y-88 Halflife 1836.png

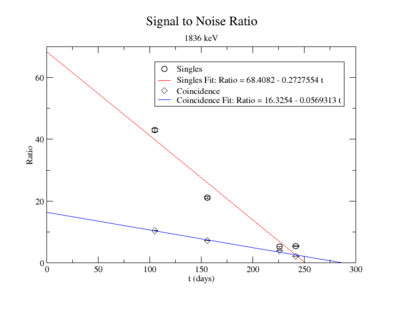
The signal to noise ratio is

SNR 1836.png


N_0 = xxxx

When SNR = 0 then t= 250.8 days (s) and 286.8 days (c)

N_{sing} = ZZZZ = lowest measurable activity using singles

N_{conic} = AAAA = lowest measurable activity using conic


Error analysis

1.) rate energy cuts

2.) Energy calibration error

3.) Linear background fit error

4.) Gaussian integration of Signal - background peak

5.) ignorable error from run length time

6.) Coincidence energy cut.

7.) dividing by average background for SNR

8.) correction ratio for 3rd data point

9.) error in half life to find N0 for the detection limit extrapolation

10.) error extrapolation efficiency to higher energy lines for N0 calculation


Half-life

898 keV Singles

Days [math]T_{1/2}[/math] Trig Energy Window Energy Calibration Linear Fit of Background Gaussian Integration of Signal Total Systematic Error
105 0.98 sing [math]\pm 10%[/math] [math] {+1.026%} \atop { -1.833%}[/math] [math] {+18.094%} \atop { -16.767%}[/math] [math]\pm 5.132%[/math] [math] {+21.802%} \atop { -12.899%}[/math]
156 1.46 sing [math]\pm 26.538%[/math] [math] {+0.816%} \atop { -2.438%}[/math] [math] {+0.747%} \atop { -0.724%}[/math] [math] {+33.437%} \atop { -29.907%}[/math] [math] {+43.207%} \atop { 41.648%}[/math]
226 2.12 sing [math]\pm 37.249%[/math] [math] {+11.874%} \atop { -16.353%}[/math] [math] {+2.704%} \atop { -2.578%}[/math] [math]\pm 3.676%[/math] [math] {+49.335%} \atop { -53.790%}[/math]
242 2.27 sing [math]\pm 22.835%[/math] [math] {+4.236%} \atop { -12.003%}[/math] [math]\pm 1.722%[/math] [math]\pm 10.677%[/math] [math] {+29.151%} \atop { -36.477%}[/math]

898 keV Coincidence

Days [math]T_{1/2}[/math] Trig Energy Window Energy Calibration Linear Fit of Background Gaussian Integration of Signal Coincidence Energy Total Systematic Error
105 0.98 coin [math]\pm 7.042e-4[/math] [math] {+1.6e-5} \atop { -6.3e-5}[/math] [math] {+7.358e-6} \atop { -7.092e-6}[/math] [math]\pm 0.00018[/math] [math] {+5.68e-4} \atop { -1.75e-3}[/math] [math] {+0.0013} \atop { -0.0025}[/math]
156 1.46 coin [math]\pm 6.383e-4[/math] [math] {+1.5e-5} \atop { -1.3e-5}[/math] [math] {+2.004e-6} \atop { -1.990e-6}[/math] [math] {+7.611e-5} \atop { -7.605e-5}[/math] [math] {+4.03e-4} \atop { -6.22e-4}[/math] [math] {+0.0011} \atop { -0.0013}[/math]
226 2.21 coin [math]\pm 6.912e-4[/math] [math] {+2.0e-5} \atop { -5.9e-5}[/math] [math] {+8.041e-6} \atop { -7.859e-6}[/math] [math]\pm 0.00013[/math] [math] {+4.92e-4} \atop { -1.06e-3}[/math] [math] {+0.0012} \atop { -0.0018}[/math]
242 2.27 coin [math]\pm 2.179e-4[/math] [math] {+2.0e-6} \atop { -4e-6}[/math] [math] {+4.436e-6} \atop { -4.365e-6}[/math] [math]\pm 1.078e-5[/math] [math] {+3.63e-4} \atop { -1.52e-3}[/math] [math] {+5.8e-4} \atop { -0.0017}[/math]


1836.1 keV Singles

Days [math]T_{1/2}[/math] Trig Energy Window Energy Calibration Linear Fit of Background Gaussian Integration of Signal Total Systematic Error
105 0.98 sing [math]\pm 11.778%[/math] [math]\pm 0.33%[/math] [math] {+0.606%} \atop { -0.582%}[/math] [math]\pm 2.241%[/math] [math]\pm 12.35%[/math]
156 1.46 sing [math]\pm 7.019%[/math] [math] {+2.812%} \atop { -1.416%}[/math] [math] {+5e-4%} \atop { -4.9e-4%}[/math] [math]\pm 4.786%[/math] [math] {+10.933%} \atop { -9.698%}[/math]
226 2.12 sing [math]\pm 20.234%[/math] [math] {+4.323%} \atop { -8.646%}[/math] [math] {+3.26%} \atop { -3.012%}[/math] [math] {+11.375%} \atop { -11.339%}[/math] [math] {+27.259%} \atop { -31.172%}[/math]
242 2.27 sing [math]\pm 19.6%[/math] [math]\pm 1.674%[/math] [math] {+2.246%} \atop { -2.205%}[/math] [math] {+11.025%} \atop { -11.107%}[/math] [math] {+24.066%} \atop { -24.1%}[/math]


1836.1 keV Coincidence

Days [math]T_{1/2}[/math] Trig Energy Window Energy Calibration Linear Fit of Background Gaussian Integration of Signal Coincidence Energy Cut Total Systematic Error
105 0.98 coin +/- 5.105e-4 [math] {+7.9e-5} \atop { -3.1e-5}[/math] +2.505e-6, -2.475e-6 +/- 8.97e-5 [math] {+3.11e-4} \atop { -2.57e-3}[/math]
156 1.46 coin +/- 6.325e-4 [math] {+2.4e-5} \atop { -3.7e-5}[/math] +3.422e-6, -3.347e-6 +/-5.83e-5 [math] {+4.63e-4} \atop { -2.82e-3}[/math]
226 2.12 coin +/- 7.285e-4 [math] {+6.9e-5} \atop { -6.8e-5}[/math] [math] {+1.515e-5} \atop { -1.481e-5}[/math] [math]\pm 2.13e-4[/math] [math] {+1.16e-3} \atop { -5.77e-3}[/math]
242 2.27 coin +/- 2.166e-4 [math] {+2.0e-6} \atop { -3e-6}[/math] +0.0020, -6.179e-6 +/-0.00012 [math] {+4.51e-4} \atop { -1.21e-3}[/math]


Signal to Noise Ratio

Days [math]T_{1/2}[/math] Trig Averaged Background
105 0.98 sing
coin
156 1.46 sing
coin
226 2.12 sing
coin
242 2.27 sing
coin


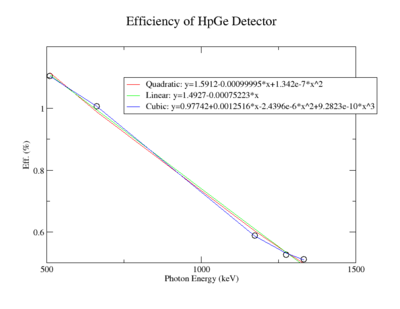
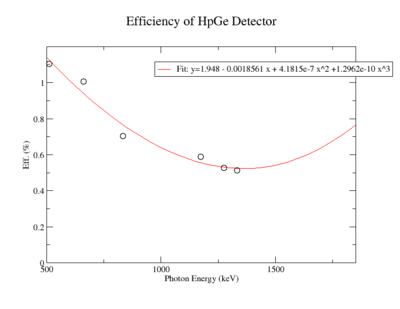
Efficiency

Days [math]T_{1/2}[/math] Trig Extrapolation of Efficiency to High Energy
105 0.98 sing
coin
156 1.46 sing
coin
226 2.12 sing
coin
242 2.27 sing
coin


Detection Limit Extrapolation

Days [math]T_{1/2}[/math] Trig Half-life Error to Find N0
105 0.98 sing
coin
156 1.46 sing
coin
226 2.12 sing
coin
242 2.27 sing
coin

Efficiency on 2/28/2014

Run Source Energy Expected rate (Hz) HpGe Det B Rate (ADC 7) (Hz) HpGe Eff (%)
7027 Na-22 511 1589.218 +/- 1.842 17.545 +/- 0.4560 1.104 +/- 1.251
7025 Cs-137 661.657 2548.022 +/- 2.222 25.622+/- 0.3286 1.006 +/- 1.443
7029 Mn-54 834.848 41.405 +/- 0.0426 0.2911 +/- 0.1667 0.703 +/- 0.1688
7026 Co-60 1173.228 1876.619 +/- 0.2687 11.238 +/- 0.3205 0.599 +/- 0.3627
7027 Na-22 1274.537 888.264 +/- 1.029 4.685 +/- 0.0825 0.527 +/- 0.6563
7026 Co-60 1332.492 1878.167 +/- 0.2690 9.616 +/- 0.1181 0.512 +/- 0.2071

HpGeEffPlot 2 28.pngHpGeEffPlot 2 28 2014.png

Efficiency on 7/11/2014

Run Source Energy Expected rate (Hz) HpGe Det B Rate (ADC 7) (Hz) HpGe Eff (%)
7027 Na-22 511 1440.050 +/- 1.778 15.465 +/- 0.5032 1.104 +/- 1.204
7025 Cs-137 661.657 2526.332 +/- 2.347 21.877 +/- 0.5463 0.8659 +/- 1.581
7029 Mn-54 834.848 30.818 +/- 0.0338 0.1711 +/- 0.2022 0.5551 +/- 0.2034
7026 Co-60 1173.228 1787.496 +/- 0.2725 9.9523 +/- 0.1973 0.557 +/- 0.2620
7027 Na-22 1274.537 804.890 +/- 0.9938 4.582 +/- 0.1409 0.586 +/- 0.6272
7026 Co-60 1332.492 1788.971 +/- 0.2727 9.092 +/- 0.0810 0.508 +/- 0.1905

HpGeEffPlot 7 11.png

Ba-133 CAA

BO-08-22-13


Useful commands

Converting CODA data file to ROOT

make sure the CODA and ROOT environmental variables are setup by source the following scripts 

source ~/CODA/setup

source ~/ROOT/root/bin/thisroot.csh

Now change to the data subdirectory and execute the program to convert the data file to root 

cd /data

~/CODA/CODAreader/ROOT_V5.30/V785V792/evio2nt -fr6994.dat > /dev/null

rename the file so it has the .root extension allowing ROOT to identify it in the browser 

mv r6994 r6994.root


Calibration work

AW_ADC_7_Histogram

AW_ADC_3_Histogram


System's intrinsic err

Plot calibration parameters as a function of time

determine the variance of the parameters using several (>20) fits

Impact of higher order fits

Plot [math]E_{expected} - E_{fit} -vs- E_{expected}[/math]

Variance comes from several fits,.

Compare uncertainty when fit is E-vs-Channe to Channel-vs-E

Probably should use %error for the weighting

Concentration measurement

A comparison of the measure concentrations using singles and coincidence counting


PAA_Research

A_W_thesis_old

Retrieved from "https://wiki.iac.isu.edu/index.php?title=A_W_thesis&oldid=94481"