TF EIM Chapt1

Fundamentals

Charge

Every stable and independent object (particle) that has charge has been observed to contain a quantized unit of charge which is a multiple of [math]1.6 \times 10^{-19} Coulombs[/math]

What are the obervations/experiments?

Experiment 1: Matter is composed of Atoms with a positively charged nucleus surround by negatively charged electrons. If we now the charge of one mole of electrons ([math]F[/math]= Faradays constant) and the number of electrons in a mole ([math]N_A[/math] = Avagadros number)then the charge of a single electron is given by

[math]e = \frac{F}{N_A}[/math]

Experiment 2: Oil drop experiment

Experiment 3: The Hall Effect and the Josephson Effect

Coulomb

The amount of charge that flows through any cross section of a wire in 1 second if there is a steady current of 1 ampere in the wire.

Coulomb Force

Two charged object separated by a distance (d) will feel a force between them known as the coulomb force. The magnitude of this force has been experimentally shown to be

[math]\left | \vec{F}_{coul} \right | = \frac{1}{4 \pi \epsilon_0} \frac{q_1 q_2}{r^2}[/math]

where

[math] \varepsilon_0 =\frac {1}{\mu_0 c_0^2}=8.854187817 \times 10^{-12}[/math]F/m = a experimentally measured quantity satisfying the above relationship know as the permittivity of free space.

[math] q_1 =[/math] charge of first object

[math]q_2 =[/math] charge of second object

[math]r =[/math] distance between the charges

(Charles Augustin Coulomb first measured the attract and repulsive force between two charged objects using a torsion balance around 1785)

This force may be described in terms of an electric field E such that

[math]\vec{E} = \frac{\vec{F_q}}{q}[/math]

Where

F= force between the objects

Electric Field

Vector Field

What is a vector field?

A vector field is the association of each point in space with a direction (vector).

Example: Gravity. On the surface of the earth you observe that an apple will fall down to the ground. You can measure the magnitude of this force by observing the attraction much like how coulomb observed how two charges attract or repel each other. You can define a vector ([math]\vec{g})[/math] which points directly down and tell you the direction that an apple will accelerate if it is released. If you construct [math]\vec{g}[/math] such that

[math]\vec{g} = \frac{F_g}{m} = \frac{\frac{GMm\vec{r}}{r^3}}{m} = \frac{GM\hat{r}}{r^2}[/math] = acceleration of an apple towards the earth's surface when close to the earth's surface.

Where G= gravitational constant = [math]6.67 \times 10^{-11} \frac{N m^2}{kg^2}[/math]

Electric Field Vector

Electric Field	Grass seeds in Insulating Liquid
Electric Vector Field for an infinitely long sheet of negative charge	The orientation of Grass seeds immersed in an insulating liquid with a charged plate.

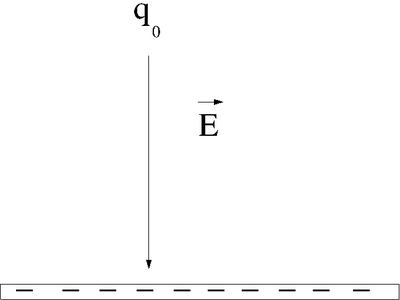
Instead of an apple of mass [math](m)[/math] we can use a test charge of charge [math](q_0)[/math].

Instead an earth of mass [math](M)[/math] we can use an object of charge [math](Q)[/math].

We now define an Electric field Vector [math](\vec{E})[/math] such that

[math]\vec{E} = \frac{\vec{F}_q}{q_0} [/math]

Note

The definition of [math]\vec{E}[/math] requires that the magnitude of the test charge approach zero, because the test change can disturb the electric field we are actually trying to determine.

[math]\vec{E} = \lim_{q_0 \rightarrow 0} \frac{\vec{F}_q}{q_0} = \lim_{q_0 \rightarrow 0} \frac{\frac{1}{4 \pi \varepsilon_o} \frac{Q q_0 \vec{r}}{r^3}}{q_0} = \frac{1}{4 \pi \varepsilon_o} \frac{Q \hat{r}}{r^2}[/math]

Lines of Coulomb Force

Once you know the Electric Vector Field you know the force on any charge placed in that field simply by

[math]\vec{F} = q \vec{E}[/math]

In other words, the tangent to the direction of the Force vector indicates the direction of the vector field [math]\vec{E}[/math].

Electric potential

The Electric potential (V) is a scalar which may be used to describe Electric Fields as well.

A charge which moves along an electric field line is having work done on it by an electric field.

From the work energy theorem, Work is equal to the change in energy of a system

[math]W = \Delta[/math] Energy

The work done moving a change along an electric field line is

[math]W = \int \vec{F} \cdot d\vec{r} = \int q \vec{E} \cdot d\vec{r} = q \int E dr = q V = U[/math] = Potential Energy

Potential Difference [math] \equiv \Delta V = V_b - V_a = \int_a^b \vec{E} \cdot d\vec{r}[/math]

Ohm's Law

resistance is a constant

[math]R = \frac{\Delta V}{I}[/math]= constant

Voltage

The MKS unit for Voltage is a Joule per Coulomb [math]\left ( \frac{\mbox{J}}{\mbox{C}}\right )[/math]

Voltage in circuits is typically defined as the electric potential energy per unit charge relative to ground.