Difference between revisions of "Forest ModernPhysics"
| (16 intermediate revisions by the same user not shown) | |||
| Line 33: | Line 33: | ||
==Davisson and Germer== | ==Davisson and Germer== | ||
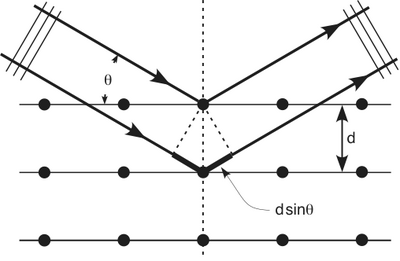
| − | We know that X-rays having a wavelength of <math>\lambda_{X-rays} = 7.1 \times 10^{-11} \mbox{m}</math> make | + | We know that X-rays having a wavelength of <math>\lambda_{X-rays} = 7.1 \times 10^{-11} \mbox{m}</math> make a diffraction pattern on an aluminum foil. The waves diffract around the obstacle and then constructively interfere according to |
| + | |||
| + | : <math>2 d sin \theta = n \lambda</math> | ||
| + | |||
| + | where <math>d</math> is the spacing between crystal planes, <math>\theta</math> is the reflected angle, and <math>\lambda</math> is the X-ray wavelength. The order of the diffraction maxima is given by <math>n</math>. The picture below should have a max in the center but the intensity is so high that it has been blocked in order to avoid washing out the picture. | ||
[[File:X-rayInterferencePattern.gif | 100px]] | [[File:X-rayInterferencePattern.gif | 100px]] | ||
| Line 48: | Line 52: | ||
;What would be the energy of an electron with the same wavelength as the above X-ray? | ;What would be the energy of an electron with the same wavelength as the above X-ray? | ||
| − | relativistic energy relation | + | relativistic total energy relation |
| − | : <math> | + | : <math>E_{tot} = \sqrt{(pc)^2 + (mc^2)^2}</math> |
| + | : <math>= \sqrt{(17.5 keV)^2 + (511 keV)^2}</math> | ||
| + | : = 511.3 keV | ||
| + | |||
| + | relativistic kinetic energy | ||
| + | :<math>K = E - mc^2 = (\gamma-1)mc^2 = 0.3 \mbox{keV} = 300 \mbox{eV}</math> | ||
| + | |||
| + | ; Note <math>\beta = \frac{pc}{E_{tot}} = \frac{17.5 \mbox{keV}}{511 \mbox{keV}} = 0.03 \Rightarrow</math> classical physics may be used for electrons below 50 keV | ||
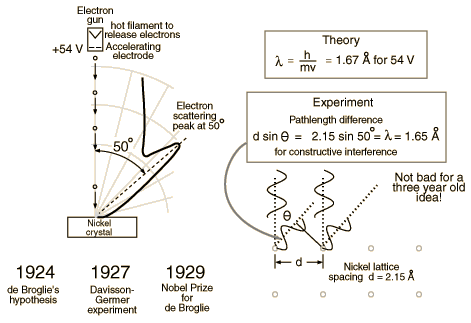
| − | + | Clinton Davisson and Lester Germer in 1927 published conclusive evidence for the diffraction of electron waves using 54 eV electrons impinging a crystal made of nickel. | |
| + | |||
| + | One problem to overcome for the experiment was that such a low energy electron scatters in air. The had to do the experiment in a vacuum. | ||
| − | |||
From hyperphysics: | From hyperphysics: | ||
| Line 63: | Line 75: | ||
[[File:Bragg_Diffraction_Illusstration.png | 400 px]] | [[File:Bragg_Diffraction_Illusstration.png | 400 px]] | ||
| + | |||
| + | = Quantization of Atomic Energy Levels= | ||
| + | |||
| + | == Absorption== | ||
| + | |||
| + | Shine white light on a gas then send the transmitted light through a prism you will see some wavelengths are mission. | ||
| + | |||
| + | |||
| + | |||
| + | == Emission == | ||
| + | |||
| + | Heat up a gas and light of specific wavelengths will be emitted. | ||
| + | |||
| + | == Balmer-Rydberg Formula== | ||
| + | |||
| + | Balmer found that the specific wavelengths oberved using Hydrogen obeyed the relation | ||
| + | |||
| + | <math>\frac{1}{\lambda} = R \left ( \frac{1}{4} - \frac{1}{n^2}\right )</math> | ||
| + | |||
| + | where | ||
| + | : <math>R = 0.0110 \mbox{nm}^{-1}</math> | ||
| + | |||
| + | Rydberg generalized the eqation to | ||
| + | |||
| + | <math>\frac{1}{\lambda} = R \left ( \frac{1}{n^{\prime^2}} - \frac{1}{n^2}\right )</math> | ||
| + | |||
| + | where <math>n > n^\prime</math> and both are integers | ||
| + | |||
| + | |||
| + | == Bohr Model of the Atom== | ||
| + | |||
[http://wiki.iac.isu.edu/index.php/Forest_Classes] [[Forest_Classes]] | [http://wiki.iac.isu.edu/index.php/Forest_Classes] [[Forest_Classes]] | ||
Latest revision as of 15:59, 30 September 2009
Matter Waves (Wave Particle Duality)
Special relativity said that
if m=0
Plank said he could fit the Black Body radiation data assuming that that
where = Plank's constant
Combining the two we have
photons have momentum like a particle (mv)
Do particles reciprocate and behave like photons?
De Broglie's Hypothesis
If photons can behave like particles by having momentum
Then can a particle behave like a wave by having wavelength
or
de Broglie Hypothesis
Davisson and Germer
We know that X-rays having a wavelength of make a diffraction pattern on an aluminum foil. The waves diffract around the obstacle and then constructively interfere according to
where is the spacing between crystal planes, is the reflected angle, and is the X-ray wavelength. The order of the diffraction maxima is given by . The picture below should have a max in the center but the intensity is so high that it has been blocked in order to avoid washing out the picture.
Another way to calculate
- What would be the energy of an electron with the same wavelength as the above X-ray?
relativistic total energy relation
- = 511.3 keV
relativistic kinetic energy
- Note classical physics may be used for electrons below 50 keV
Clinton Davisson and Lester Germer in 1927 published conclusive evidence for the diffraction of electron waves using 54 eV electrons impinging a crystal made of nickel.
One problem to overcome for the experiment was that such a low energy electron scatters in air. The had to do the experiment in a vacuum.
From hyperphysics:
Bragg Diffraction
Quantization of Atomic Energy Levels
Absorption
Shine white light on a gas then send the transmitted light through a prism you will see some wavelengths are mission.
Emission
Heat up a gas and light of specific wavelengths will be emitted.
Balmer-Rydberg Formula
Balmer found that the specific wavelengths oberved using Hydrogen obeyed the relation
where
Rydberg generalized the eqation to
where and both are integers