Difference between revisions of "Forest Bhabha Scattering"
| Line 15: | Line 15: | ||
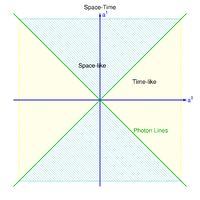
A particle which travels only along the horizontal time axis is not moving in space while a particle traveling only along the vertical axis is not moving in time (within the uncertainty principle). | A particle which travels only along the horizontal time axis is not moving in space while a particle traveling only along the vertical axis is not moving in time (within the uncertainty principle). | ||
| + | |||
| + | ==e+e- scattering== | ||
| + | |||
| + | If the electron and positron simply scatter off of one another via a coulomb interaction, then they exchange a virtual photon along the space axis. You start with an external line from the left to represent the electron | ||
=Step 2 identify 4-Momentum conservation= | =Step 2 identify 4-Momentum conservation= | ||
Revision as of 17:42, 14 April 2012
Bhabha (electron -positron) Scattering
Bhabha scattering identifies the scatterng of an electron and positron (particle and anti-particle). There are two processes that can occur
1.) scattering via the exchange of a virtual photon
2.) annihilation in which the e+ and e- spend some time as a photon which then reconverts back to an e+e- pair
Step 1 Draw the Feynman Diagram
The Feynman diagram is a space-time description of the interaction where the horizontal axis (abscissa) is used to denote time and the vertical axis (ordinate) is 3-D space.
A particle which travels only along the horizontal time axis is not moving in space while a particle traveling only along the vertical axis is not moving in time (within the uncertainty principle).
e+e- scattering
If the electron and positron simply scatter off of one another via a coulomb interaction, then they exchange a virtual photon along the space axis. You start with an external line from the left to represent the electron
Step 2 identify 4-Momentum conservation
Let:
- initial electron 4-momentum
- initial electron spinor
- final electron 4-momentum
- final electron spinor
- initial positron 4-momentum
- initial positron spinor
- finial positron 4-momentum
- finial positron spinor
Step 3 Determine Matrix element for each vertex
Step 4 Find total amplitude
Matrix element for scattering
According to the Feynman RUles for QED:
the term
is used at the vertex to describe the Quantum electrodynamic (electromagneticc) interaction between the two fermion spinor states entering the vertex and forming a photon which will "connect" this vertex with the next one.
- The QED interaction Lagrangian is
Matrix element for annihilation