|
|
| Line 52: |
Line 52: |
| | <math>z=z'-L</math> | | <math>z=z'-L</math> |
| | | | |
| − | <math> B_z = \frac {μ_0 }{2} \frac {R^2 \cdot I}{(z_n^{'2} + R^{'2})^{\frac{3}{2}} </math> | + | <math> B_z = \frac {μ_0}{2} \frac {R^2 \cdot I}{(z^{'2} + R^{'2})^{\frac{3}{2}} </math> |
| | | | |
| | [http://wiki.iac.isu.edu/index.php/PhotoFission_with_Polarized_Photons_from_HRRL Go Back] | | [http://wiki.iac.isu.edu/index.php/PhotoFission_with_Polarized_Photons_from_HRRL Go Back] |
Revision as of 15:10, 10 June 2010
Field needed to bend 44 MeV electrons 0.47 degrees
[math]1 MeV = 1.6\cdot 10^{-13} J = 1.6\cdot 10^{-13} \frac{m^2\cdot kg}{s^2}[/math]
[math]c = 2.998 \cdot 10^8 \frac{m}{s}[/math]
[math]\frac{MeV}{C} = 0.534 \cdot 10^{-21} \frac{m\cdot kg}{s}[/math]
[math]p_e = 44 \frac{MeV}{c} = 23.5 \cdot 10^{-21} \frac{m\cdot kg}{s}[/math]
[math]B = \frac{p_e}{q_e \cdot R}[/math]
[math]1T=\frac{kg}{C\cdot s}[/math], [math]q_e = 1.6\cdot 10^{-19} C[/math], [math]1T=10^{-4}G[/math]
[math]B(T) = \frac{p_e (\frac{MeV}{c})\cdot 0.33\cdot 10^{-2}}{R(m)}[/math]
[math]B(T) = \frac{4.67\cdot 10^{-2}}{R(m)}[/math]
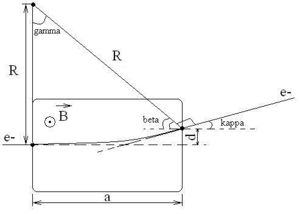
[math]180^0 = \kappa + 90^0 + \beta[/math]
[math]180^0 = \gamma + 90^0+ \beta[/math]
[math]\kappa = \gamma[/math]
[math]R = \frac{a}{cos(\beta)} = \frac{a}{cos(90^0 - \kappa)} = \frac{a}{sin(\kappa)}[/math]
[math]d = R \cdot (1 - cos(\kappa)) = \frac{a \cdot (1 - cos(\kappa))}{sin(\kappa)}[/math]
[math]B(T) = \frac{p_e (\frac{MeV}{c})\cdot 0.33\cdot 10^{-2}\cdot sin(\kappa)}{a(m)}[/math] - general expression for B-field.
[math]B(T) = \frac{7.83\cdot 10^{-2}\cdot sin(\kappa)}{a(m)}[/math]
If [math]\kappa = 0.47^0[/math] then [math]sin(\kappa) = 0.0082[/math] and our B-field becomes:
[math]B(T) = \frac{1.2\cdot 10^{-3}}{a(m)}[/math]
[math]a \simeq 0.125 m[/math] for the coils under consideration. Hence, the B-field needed is:
[math]B = 0.00964 T = 96.4 G[/math]
Magnetic Field Produced by Coils
300px
[math]z'=L+z[/math]
[math]z=z'-L[/math]
[math] B_z = \frac {μ_0}{2} \frac {R^2 \cdot I}{(z^{'2} + R^{'2})^{\frac{3}{2}} [/math]
Go Back
