Difference between revisions of "Relativistic Frames of Reference"
| Line 32: | Line 32: | ||
| − | <center><math>speed=\frac{ | + | <center><math>speed=\frac{Distance}{Time}</math></center> |
| − | <center><math>c=\frac{ | + | <center><math>c=\frac{d}{t}</math></center> |
| Line 45: | Line 45: | ||
| − | <center><math>c=\frac{\sqrt{ | + | <center><math>c=\frac{\sqrt{x^2+y^2+z^2}}{t}</math></center> |
| Line 51: | Line 51: | ||
| − | <center><math>c=\frac{\sqrt{ | + | <center><math>c=\frac{\sqrt{x^{'2}+y^{'2}+z^{'2}}}{t}</math></center> |
| Line 60: | Line 60: | ||
| − | <center><math>\frac{ | + | <center><math>\frac{x^{'2}+y^{'2}+z^{'2}}{t^{'2}}= c^2=\frac{x^2+y^2+z^2}{t^2}</math></center> |
| Line 66: | Line 66: | ||
| − | <center><math>c^2 | + | <center><math>c^2 t^{'2}=x^{'2}+y^{'2}+z^{'2}\ \ \ \ \ c^2 t^{2}=x^2+y^2+ z^2</math></center> |
| − | <center><math>\Rightarrow c^2 | + | <center><math>\Rightarrow c^2 t^{'2}- x^{'2}-y^{'2}-z^{'2}= c^2 t^{2}-x^2-y^2-z^2</math></center> |
| − | This quantity is known as the time space interval <math> | + | This quantity is known as the time space interval <math>s^2</math> |
| − | <center><math> | + | <center><math>s^2\equiv c^2 t^{'2}-x^{'2}-y^{'2}-z^{'2}= c^2 t^{2}-x^2-y^2-z^2</math></center> |
| − | Since the speed of light is a constant for all frames of reference, this allows the space time interval to also be invariant. | + | Since the speed of light is a constant for all frames of reference, this allows the space time interval to also be invariant for inertial frames. |
---- | ---- | ||
Revision as of 01:19, 4 June 2017
Relativistic Frames of Reference
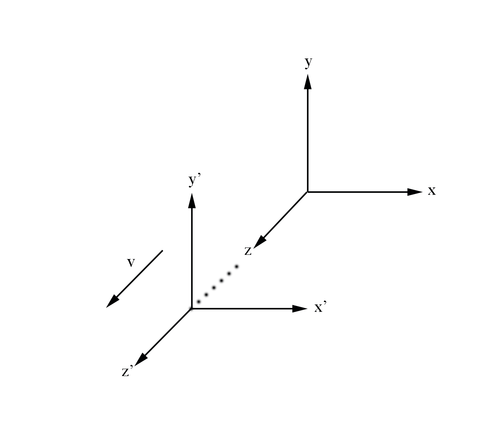
From the Galilean description of motion for a frame of reference moving relative to another frame considered stationary we know that
Using Einstein's Theory of Relativity, we know that the speed of light is a constant, c, for all reference frames. In the unprimed frame, from the definition of speed:
where
Using the distance equation in a Cartesian coordinate system, the equation for the speed of light becomes
Following the postulate of Special Relativity, this implies for the primed frame
We can rewrite this as
This is possible since the ratios of distance to time are multiples of the same base, i.e. the square of the speed of light . Therefore for the relative change in the time in one frame, the distance must change by the same factor to maintain the same constant. With this we can write
This quantity is known as the time space interval
Since the speed of light is a constant for all frames of reference, this allows the space time interval to also be invariant for inertial frames.