LB Thesis Post Defense
This is a recent copy of my thesis to be workshopped.
Introduction
\chapter{Introduction} \hspace{0.5in} Photon Activation Analysis (PAA) exploits nuclear reactions to quantify the isotopes within a sample. If the isotope of interest is embedded in some matrix, then a natural question arises: "What concentration of the isotope must be present within the matrix to detect it?". The answer can be quantified by studying the signal to noise ratio of characteristic gamma decay lines of the isotope of interest. The concentration at which the signal to noise ratio is unity will be defined as the minimum detectable concentration.
\hspace{0.5in} The purpose of this experiment was to quantify the minimum detectable concentrations of Se-82 and Se-76 in soil. Se-82 and Se-76 are naturally occurring isotopes with abundances of 8.73\% and 9.37\% respectively. Using high energy photons to eject a neutron from either isotope leaves the isotope in an excited state that will eventually de-excite by emitted characteristic gamma radiation that can be detected by a High Purity Germanium (HpGe) detector. There is a 0.4\% deviation in the ratio of $\frac{Se-82}{Se-76}$ in soil due to environmental factors \cite{Reference6}. The goal of this work is to determine if the minimum detectable concentration of Se in soil will allow measurements of this small deviation.
Theory
\chapter{Theory}
\hspace{0.5in} Photon activation analysis (PAA) is a method used to determine the elemental composition of a sample based on the characteristic photon energies emitted by gamma decays from the nucleus of radioactive isotopes that have been activated by bremsstrahlung photons. bremsstrahlung photons are produced with sufficient energy to free nucleons from the target nuclei in the sample of interest. The resulting nucleus tends to be in an excited state after the interaction. Photons with characteristic energies corresponding to the nucleus are emitted when the nucleus de-excites. The energy of these photons can be measured using a high purity germanium (HpGe) detector. Isotopes of interest are uniquely identified by measuring the decay rates of their characteristic energy lines over time and comparing them to their expected values. A theoretical description of each of the above steps is given below.
\section{Bremsstrahlung Radiation}
\hspace{0.5in} A charged particle emits bremsstrahlung (braking radiation) if it decelerates due to its interaction with the electric field of an atom in a material. Consider an electron from an accelerator that has travelled into a tungsten/aluminum radiator. As the electron travels, there is a probability that it will be deflected by the electric field of either electrons or nuclei within the material and emit a photon. The cross section for the nuclear process is given by the Bethe-Heitler equation \cite{Reference2}: $$d\sigma_{Brem} = 4Z^2r_{e}^2\frac{d\nu}{\nu}[(1+(\frac{E}{E_0})^2)[\frac{\phi_1(\gamma)}{4}-\frac{1}{3}\ln{Z} - f(Z)]-\frac{2E}{3E_0}[\frac{\phi_2(\gamma)}{4}-\frac{1}{3}\ln{Z}-f(Z)]]$$
where
$E_0 = $ initial energy of the electron
$E = $ final energy of the electron
$\nu = \frac{E_0 - E}{h} = $ energy of the Bremsstrahlung photon
$Z = $ number of protons in the target material
$\gamma = \frac{100m_ec^2h\nu}{E_0EZ^{\frac{1}{3}}}$
$f(Z) = (Z\alpha)^2\Sigma_1^\infty\frac{1}{n[n^2 + (Z\alpha)^2]}$
%$\alpha = \frac{1}{137}$
It is important to note that $\phi_1(\gamma)$ and $\phi_2(\gamma)$ are screening functions that change based on the value of Z. Including the case where the incident electron is deflected by another electron, the total cross section for the Bethe-Heitler formula becomes
$$d\sigma_{e^-} = \frac{Z(Z+1)}{Z^2}d\sigma_{Brem}$$
\section{Minimum Neutron Knockout Energy in Selenium}
\hspace{0.5in} Determining the most efficient irradiation of a target sample for PAA relies on knowing both the minimum nucleon knockout energy of the isotope of interest and the cross section for the reaction. The cross section expresses the probability of liberating a nucleon from the target. If a photon has insufficient energy to liberate a nucleon from a target, then the nucleus could still be put in an excited state, but this experiment focused on neutron removal. Both the incident photon energy and the cross section of the reaction of interest are used to determine the optimal irradiation conditions for the sample.
\hspace{0.5in} There are three important aspects for the efficient irradiation by the bremsstrahlung photons that escape the accelerator's radiator. The photons must have sufficient energy to overcome the binding energy and liberate nucleons from within the target. The energy of the photons impinging upon the target must also include as much of the cross section as possible to increase the probability of the reaction occurring. Finally, saturation requires 5 half lives worth of irradiation time. The samples in this experiment were not saturated.
%\hspace{0.5in}After the Bremsstrahlung photons leave the accelerator's radiator, they will be incident on the target, but there are three important aspects to ensure an effective irradiation: The photons must have enough energy to liberate nucleons from the target, the energy must include as much of the cross section as possible, and the sample must not completely decay while in the beam.
\hspace{0.5in}The minimum photon energy that may induce nuclear reactions of interest is calculated using the binding energy of a nucleus. In this case, the reactions of interest are ${76 \atop 34\; }Se (\gamma,n){75 \atop 34\;}Se$ and ${82 \atop 34\; }Se (\gamma,n){81 \atop 34\;}Se$. The minimum neutron knockout energy for both reactions can be found by
$$E_{knockout}^{neutron} = |M_{Se-76} - M_{Se-75} - M_{neutron}| = |70718.2992-69789.8882 - 939.57| = 11.2 MeV$$ $$E_{knockout}^{neutron} = |M_{Se-82} - M_{Se-81} - M_{neutron}| = |76304.9218 - 75374.6322 - 939.57 |= 9.3 MeV$$
Both reactions can be studied if the bremsstrahlung photon energy is at least 11.2 MeV.
%\section{Cross Sections} \hspace{0.5in} Another important aspect to take into consideration when choosing the beam energy is the cross section for the specific reactions \cite{Reference4}\cite{Reference5}.
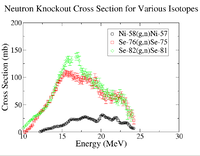
\begin{figure}[h]
\centering
\includegraphics[width = 9cm]{Appendices/LB_Thesis_BigFont_XSect.png}
\caption{Neutron knockout cross section for selenium isotopes and nickel used in this work}
\label{XSect}
\end{figure}
The maximum value of the cross section occurs between 15 MeV and 20 MeV. This means that photons of that energy will have the highest probability of liberating a neutron from a selenium nucleus.
\hspace{0.5in}The production rate ($\frac{dN}{dt}$) is given by \cite{Reference1} \begin{equation}
\frac{dN}{dt} = N^+ - \lambda N(t)
\end{equation}
\begin{equation}
N(t) = \frac{N^+}{\lambda}(1-e^{-\lambda t})
\end{equation}
where $N^+$ is given by
\begin{equation}
N^+ = n_T V_T \int \sigma(E) \phi(E) dE
\end{equation}
Where $n_T$ is the number of nucleons per unit volume within the target, $V_T$ is the volume of the target, $\sigma(E)$ is the reaction cross section, $\phi(E)$ is the photon flux through the target, dE represents the energy range in which the cross section is non-zero, and $\lambda$ is the decay constant for the isotope ($\ln2/t_{1/2}$). The dependence of this equation on the half life means the net production will be lower for isotopes with shorter half lives because the isotopes with longer half lives will not reach saturation as quickly, meaning the levelling off of the capacitive production rate curve would take longer to become apparent. Due to the large difference in half lives (57.28 minutes for Se-81 and 120 days for Se-75), the net production rate will be higher for Se-75 than Se-81 if they are irradiated at the same time.
\section{HpGe Detector Physics} \hspace{0.5in} The class of detectors used to measure photon energies with high resolution are high purity Germanium (HpGe) detectors. Germanium is a semiconductor, thus current flows if electrons are moved from the valence band to the conduction band. Unfortunately the "band gap" for Germanium is such that, at room temperature, thermal excitations can move electrons from the valence band into the conduction band, creating a high amount of noise that could potentially make a signal ambiguous. The band gap energy in a material is given by
$$E_g(T) = E_g(0)-\frac{\alpha T^2}{T+\beta}$$
where $ \alpha = \frac{\sqrt{2m(V_0 - E)}}{\hbar}$ and $\beta = \frac{\sqrt{2mE}}{\hbar}$ \cite{Reference3} . It is clear that as the temperature decreases, the band gap energy increases. The large amount of noise at room temperature can be remedied by cryogenically cooling the detector thereby increasing the band gap energy. This makes a signal produced by an incoming photon very obvious in the detector. A high voltage is applied across the semiconductor to accelerate electron-hole pairs to opposite sides of the semiconductor and increase the depletion region improving detection efficiency.
\hspace{0.5in}There are three main types of interactions that can create a signal within the detector. The first interaction is the photoelectric effect. This means that the incoming radiation has sufficient energy to liberate an electron within the material creating an electron-hole pair. The electric field created by the applied high voltage will drift the electrons to one terminal and the holes to another producing a signal.
\hspace{0.5in} The second type of interaction that can occur is Compton scattering in which a photon scatters off of an electron losing energy. For the photon's remaining energy to contribute to a signal that is proportional to the original energy of the incoming photon, all Compton scattering events must occur inside the sensitive volume of the detector and liberate an electron via the photoelectric effect. This ensures that all of the energy of the incoming photon has been measured by the detector. It is possible that the radiated photon Compton scatters depositing a fraction of its energy inside the sensitive volume of the detector. If this happens then the detected signal does not accurately represent the total energy of the radiated photon, but instead will contribute to the Compton continuum. Inspecting the Compton scattering formula for the energy of the outgoing photon, it can be seen that there is a maximum energy that the photon can deposit. $$E' = \frac{E}{1+\frac{E}{m_ec^2}(1-\cos{\theta})}$$ where
E' is the final energy of the photon
E is the initial energy of the photon
%$m_e$ is the mass of the electron
%c is the speed of light
$\theta$ is the deflection angle for the photon
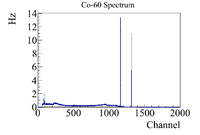
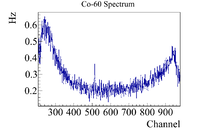
It can be seen that the maximum energy loss occurs when the photon backscatters off of the electron ($\theta = 180^{\circ}$), resulting in a Compton edge. An example of the Compton edge from a 1.1 MeV photon is shown in Figure \ref{Compton Continuum}.
\begin{figure}[h]
\centering
\begin{minipage}[b]{0.4\textwidth}
\includegraphics[width=\textwidth]{Chapters/Co60_Thesis_UnzoomedSpec.png}
\caption{Co-60 Spectrum}
\label{Co-60Spect}
\end{minipage}
\hfill
\begin{minipage}[b]{0.4\textwidth}
\includegraphics[width=\textwidth]{Chapters/Co_60_GammaSpec_ComptonEdge_Zoomed.png}
\caption{Compton Continuum Ranging From 294-906 keV }
\label{Compton Continuum}
\end{minipage}
\end{figure}
\hspace{0.5in}The final interaction that can occur is pair production. If the incident photon has high enough energy (1.02 MeV), then the photon can fluctuate into an electron-positron pair. These can produce pulses within the detector. The size of the pulse is proportional to the energy of the electron and the positron. If the positron annihilates with an electron within the semiconductor, then two 511 keV photons are produced that can also create signals within the detector as outlined above.
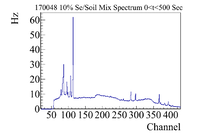
\hspace{0.5in} Once the signals are produced, they are sent through a pre-amplifier, located close to the semiconductor, and then a post amplifier with two analog outputs. One is sent to a constant fraction discriminator (CFD), while the other signal is sent to an analog-to-digital converter (ADC) which converts the analog signal from the HpGe detector into a channel number. More information can be found in section 2.2. A sample histogram is shown in Figure \ref{Sample Gamma Spectrum}
\begin{figure}[h]
\centering
\includegraphics[width = 6cm]{Chapters/LB_SampleSeSoilSpec.png}
\caption{Sample Energy Spectrum}
\label{Sample Gamma Spectrum}
\end{figure}
It is easy to see that there could be lines produced by the selenium radioisotopes with competing energy lines produced by the matrix the selenium was within, or from other radioactive materials within the lab.
\section{Identifying Isotopes of Interest in a Gamma Spectrum} \hspace{0.5in} Identifying energy lines characteristic of an isotope of interest and measuring the decay rate of those energy lines to calculate a half life are the two steps taken to identify an isotope of interest. Isotopes can have several characteristic gamma decay lines. Consider an isotope of interest that has three prominent decay lines. If all of those lines are present in the spectrum, then that would be an indication that the isotope of interest is in the sample. The more characteristic decay lines an isotope has, the easier it is to infer that it may be present in the sample. If an isotope of interest only has one characteristic decay line, then that could increase the chances for a false positive. Unfortunately, the presence of energy lines alone are not enough information to prove the energy lines were produced by the isotope of interest.
\hspace{0.5in}To strengthen the argument further, the lifetime of the energy line of interest is measured. The activity (A) of decaying isotopes will follow the standard half life equation $$A(t) = A_0e^{-\lambda t}$$ where $\lambda$ is the decay constant for the isotope and $t$ is the time that has passed since the irradiation. The decay constant is related to the half life via \begin{equation}
\lambda = \frac{\ln{2}}{t_{\frac{1}{2}}}.
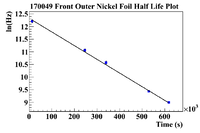
\end{equation} By measuring the activity and plotting it as a function of time, the decay constant, and thus the half life can be found. A sample nickel spectrum which has been fitted with an exponential function is shown in Figure \ref{Ni Energy Decay Sample}.
\begin{figure}[h]
\centering
\includegraphics[width = 8cm]{Chapters/LB_Thesis_FrontNiFoil_LinHLExample.png}
\caption{Example of a decaying Ni-57 1337 keV energy line with a half life of 35.60 hours.}
\label{Ni Energy Decay Sample}
\end{figure}
\hspace{0.5in} It can be seen above that the activity will decrease as time goes on due to decay. If both the characteristic energy lines of the isotopes and the half life are in agreement with the attributes of the isotope of interest, then the argument vastly strengthens as there aren't many isotopes with similar half lives and energies. Usually these two pieces of information are sufficient to identify an isotope of interest within the irradiated sample. The argument may be strengthened further, for isotopes with several lines, by quantifying the branching ratios for the different energy lines. The activity of the energy lines should match the branching ratio, but an accurate measurement of the detector efficiency needs to be taken for each energy line.
\section{Signal to Noise Ratio} \hspace{0.5in}The previous sections discussed how to produce and identify isotopes of interest within a certain sample, but these steps do not ensure that characteristic energy lines will be visible at all. One problem that may arise is the background signal from the matrix could be much more prominent than the characteristic energy lines of interest. If this is the case, then the signals will not be visible for data analysis. The signal to noise ratio is a quantity that may be used to quantify the minimum detectable concentration of selenium in soil. Irradiating the selenium and the soil in the same container and measuring them as a single target begs the question:``At what concentration of selenium in soil will the characteristic energy lines of Selenium not be visible?". The signal to noise ratio (SNR) is given by
\begin{equation}
SNR = |\frac{I-B}{B}|
\end{equation}
The signal to noise ratio can be plotted as a function of concentration to determine the concentration that corresponds to the ratio of unity, making the signal immeasurable. It can also be plotted for a single measurement as a function of time to see how the background matrix signal decays during the measurement. This can be used to investigate an optimum measurement time delay after the beam has been turned off to determine an optimal time to measure.
Experimental Setup
\chapter{Experimental Setup}
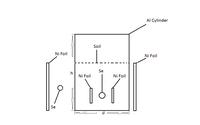
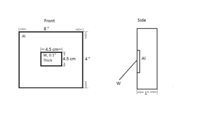
\section{Target Information} \hspace{0.5in} On May 23, 2017 four targets were irradiated using 32 MeV electrons impinging on a 25.4 mm thick radiator. Aluminium cylinders were used to contain the samples. The cylinder had a 4.5 cm diameter, a height of 12 cm, and a thickness of 1 mm. The beam conditions were set to a frequency of 180Hz, a pulse width of 2.2 $\mu$s, and a current of 0.15 $\mu$A. Figure \ref{Targ Setup} is a schematic of the sample configuration, while Figure \ref{W,Al converter} is a schematic of the Tungsten/Aluminum converter. \begin{figure}[h]
\centering
\includegraphics[width = 14cm]{Chapters/LB_Thesis_TargSetup.png}
\caption{Target schematic, d = 4.5 cm, h = 12 cm, 1 mm thick.}
\label{Targ Setup}
\end{figure}
\begin{figure}
\centering
\includegraphics[width = 12cm]{Chapters/LB_Thesis_Radiator_Front.png}
\caption{Schematic of tungsten aluminum converter.}
\label{W,Al converter}
\end{figure}
\begin{table}[]
\centering
\begin{tabular}{|c|c|c|c|} \hline
\% Concentration of Se doping by Mass & Beam On & Beam Off & $\Delta t_{Measure}$ (s) \\ \hline
50\% & 08:47 & 09:19 & 1476 \\ \hline
10\% & 09:55 & 10:55 & 1504 \\ \hline
1\% & 11:07 & 12:07 & 1214 \\ \hline
0.1\% & 16:09 & 16:39 & 2037 \\ \hline
\end{tabular}
\caption{Beam times for each sample.}
\label{Table 2.1}
\end{table}
\begin{table}[h]
\centering
\begin{tabular}{|c|c|c|c|c|c|c|c|}\hline
Sample & $m_s$ &$m_i^{Se}$ & $m_o^{Se}$ &$M_F^{Ni}$ &$M_R^{Ni}$ & $m_F^{Ni}$ &$m_R^{Ni}$\\ \hline
50\% & 0.4921 & 0.5145 & 0.0900 & 1.5535 & 1.6140 & 0.3956 & 0.3050 \\ \hline
10\% & 4.9921 & 0.5142 & 0.1245 & 1.3310 & 1.2196 & 0.3592 & 0.2768 \\ \hline
1\% & 10.2403 & 0.0954 & 0.0914 & 3.4274 & 3.4887 & 1.8672 & 1.7220 \\ \hline
0.1\% & 100.1242 & 0.1074 & 0.1042 & 1.3911 & 1.3804 & 0.4467 & 0.2548 \\ \hline
\end{tabular}
\caption{Masses of target components in grams, each mass has a reading error of 5 $\times 10^{-4}$ g. $m_s$ = soil mass, $m_i^{Se}$ = inner Se mass, $m_o^{Se}$ = outer Se mass, $M_F^{Ni}$ = mass of front outer foil,
$M_R^{Ni}$ = mass of rear outer foil,\\$m_F^{Ni}$ = front inner foil mass, $m_R^{Ni}$ = mass of rear inner foil.}
\label{TargMass}
\end{table}
\begin{table}[h]
\centering
\rotatebox{90}{
\begin{tabular}{|c|c|c|c|c|c|c|c|}\hline
Sample & $V_s$ &$V_i^{Se}$ & $V_o^{Se}$ &$V_F^{Ni}$ &$V_R^{Ni}$ & $v_F^{Ni}$ &$v_R^{Ni}$\\ \hline
50\% & 0.57 $\pm$ 0.1\% & 0.11 $\pm$ 0.09\% & 0.02 $\pm$ 0.52\% & 0.20 $\pm$ 0.03\% & 0.21 $\pm$ 0.03\% & 0.05 $\pm$ 0.11\% & 0.04 $\pm$ 0.14\% \\ \hline
10\% & 5.82 $\pm$ 0.01\% & 0.11 $\pm$ 0.09\% & 0.03 $\pm$ 0.35\% & 0.17 $\pm$ 0.03\% & 0.16 $\pm$ 0.04\% & 0.05 $\pm$ 0.11\% & 0.04 $\pm$ 0.14\% \\ \hline
1\% & 11.94 $\pm$ 0.01 \% & 0.02 $\pm$ 0.52\% & 0.02 $\pm$ 0.35\% & 0.44 $\pm$ 0.01\% & 0.45 $\pm$ 0.01\% & 0.24 $\pm$ 0.02\% & 0.22 $\pm$ 0.03\% \\ \hline
0.1\% & 116.79 $\pm$ 0.01 \% & 0.02 $\pm$ 0.52\% & 0.02 $\pm$ 0.35\% & 0.18 $\pm$ 0.03\% & 0.18 $\pm$ 0.03\% & 0.06 $\pm$ 0.09\% & 0.03 $\pm$ 0.19\% \\ \hline
\end{tabular}
}
\caption{Volumes of target components in cubic centimeters, $V_s$ = soil volume, $V_i^{Se}$ = inner Se volume, $V_o^{Se}$ = outer Se volume, $V_F^{Ni}$ = volume of front outer foil, $V_R^{Ni}$ = volume of rear outer foil, $v_F^{Ni}$ = volume of front inner foil, $v_R^{Ni}$ = volume of rear inner foil}
\label{TargVol}
\end{table}
Table 2.3 lists the masses used for each sample, while Table 2.4 lists the volumes of each sample. \clearpage
\section{Data Acquisition System} \hspace{0.5in} Signals produced by the radioactive target within the sensitive volume of the HpGe detector (as outlined in Section 1.3) were sent through a series of electronic units commonly referred to as a data acquisition system (DAQ). Specifically, after the signal was produced it was amplified by a pre-amplifier within the HpGe detector. The final two pieces of the DAQ were an ORTEC model 672 Spectroscopy Amplifier and a Canberra model 8701 peak sensing analog to digital converter (ADC) with an internal constant fraction discriminator (CFD). The high voltage source used was an ORTEC 659 5 kV power supply set to 4.5 kV in a positive bias.
\hspace{0.5in} The constant fraction discriminator's purpose was to filter unwanted signals created within the HpGe detector's sensitive volume. The CFD duplicated the analog signal, inverted it, and scaled it by a constant value. Once this has been done the duplicate signal is time-delayed by an amount of time that is less than the rise time of the analog signal. The CFD then searched for a zero crossing point. If such a point was found then the signal was allowed to pass through the CFD. If not, then the signal was rejected. For the purpose of this experiment the lower limit setting resulted in measurements of 40 keV photons or lower being rejected.
\hspace{0.5in} The peak sensing analog to digital converter is a Wilkinson apparatus which operates by opening a gate for a select amount of time. While the gate is open, the conversion takes place. Assuming the gate is open, the analog pulse is sent through a differentiating circuit, which has an output proportional to the rate of change of the signal. Once the circuit finds a maximum, the voltage is held constant by a capacitor. If a larger signal were to appear, then the capacitor would maintain the larger voltage as opposed to the smaller one. A comparitor was used to decide if a higher voltage appeared while the gate was open. After the gate closes, the capacitor discharges and voltage information is assigned a unique numerical channel.
Data Analysis
%\usepackage{adjustbox}
\chapter{Data Analysis} \section{Split Run Measurement Technique} \hspace{0.5in} The measured activity of a sample and its normalization target (outer witness Se pellet) were stored in the same data file along with a calibration source used as a marker to separate the measurements. Each sample was measured at the same position from the detector by switching samples after five minutes. This allows multiple measured samples to be written to the same file. Calibrated sources were used as a marker to indicate a change in the sample being measured by the HpGe detector. Several samples were measured during a single Multiparameter Data Acquisition System (MPA) run.
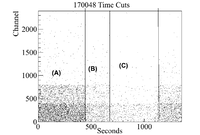
\hspace{0.5in} It is very important that the marker's energy spectrum be clearly distinguishable from the sample's energy spectrum. A Co-60 source was used as a marker because it has very strong energy lines at 1.173 MeV and 1.332 MeV, whereas the selenium/soil samples had no strong energy lines greater than 1 MeV. After a five minute measurement, the first sample was removed and the Co-60 flag was placed in front of the HpGe detector for one minute. This ensured that the signal produced by the flag was strong enough to adequately separate the runs. After the one minute measurement using the Co-60 flag, it was removed and the second selenium sample was placed in front of the detector and measured for five minutes. This process was repeated for the length of the entire run. It can be quite cumbersome to record all of the transition times while switching the samples, but this can be remedied by plotting the channel number vs. time on a 2D histogram as shown in Figure \ref{Sample Split Time vs Counts}.
\begin{figure}[!tbp]
\centering
\begin{minipage}[b]{0.4\textwidth}
\includegraphics[width=\textwidth]{Chapters/LB_Thesis_170048TimeCutSample.png}
\caption{Sample split run where (A) is the Se/soil Mix, (B) is the Co-60 marker, and (C) is the pure selenium.}
\label{Sample Split Time vs Counts}
\end{minipage}
\hfill
\begin{minipage}[b]{0.4\textwidth}
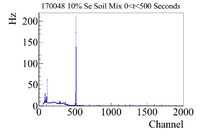
\includegraphics[width=\textwidth]{Pictures/170048_SeSoilMixSpec_0_t_500_TimeCutSample.png}
\caption{(A) Se/soil mixture for 0$<$t$<$500 s.}
\end{minipage}
\end{figure}
\begin{figure}[!tbp]
\centering
\begin{minipage}[b]{0.4\textwidth}
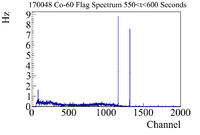
\includegraphics[width=\textwidth]{Pictures/170048_Co60FlagSpec_550_t_600Sec.png}
\caption{(B) Co-60 flag 550 $<$t$<$600 s}
\end{minipage}
\hfill
\begin{minipage}[b]{0.4\textwidth}
\includegraphics[width=\textwidth]{Pictures/170048_PureSeSpec_615_t_1115_TimeCutSample.png}
\caption{ (C) Pure Se 615$<$t$<$1115 s.}
\end{minipage}
\end{figure}
Note the different runs are distinct and can be identified as long as one measurement is known. The larger peaks in Figure 3.3 are from the Co-60's 1.173 and 1.332 MeV lines.
\section{Energy Spectrum Analysis Method}
\hspace{0.5in} A detailed explanation of how to trace the isotope of interest's activity back to its original value is given below. The sample of interest here is \#170048, which was a 10\% mixture of selenium and soil. The measurement was made on Detector $A$ at the Idaho Accelerator Center. A full description of Detector $A$ is given in Appendix A. The measurement was a split run using a Co-60 flag to identify the change between a pure selenium sample and the Se/soil mixture. The target information can be found below:
\begin{center}
\begin{tabular}{|c|c|c|c|c|} \hline
Soil Mass & Mixed Se Mass & Outer Se Mass &Front Ni Foil Mass & Rear Ni Foil\\ \hline
4.9921 g & 0.5142 g & 0.1245 g & 1.3310 g & 1.2196 g \\ \hline
\end{tabular}
\end{center}
\subsection{Multi-Channel Histograms} "Multi-Channel Histograms" were named such because the analysis was performed in a one, three, or ten channel, spectrum window. Each histogram had its number of counts weighted by the mass of the sample. Since the resolution of Detector $A$ is about 1 keV, the window in which the signal is viewed can't be less than 1 keV. The function used to fit the energy lines was of the form \begin{equation}
f(x) = C_1 + C_2e^{\frac{(x-\overline{x})^2}{2\sigma^2}}
\end{equation}
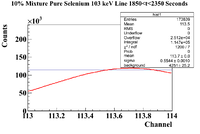
Here, the constant value ($C_1$) added to the Gaussian measures the value for the background that will be subtracted. Figure \ref{1 Chan Sample Hist} shows a typical "multi-channel" histogram for Sample #170048 using one channel
\begin{figure}[h]
\centering
\includegraphics[width = 10cm]{Pictures/LB_Thesis_DataAnalysis_Sample_1Chan.png}
\caption{\#170048 Pure Se thin window histogram}
\label{1 Chan Sample Hist}
\end{figure}
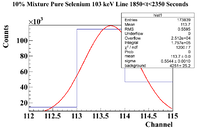
Figure \ref{1 Chan Sample Hist} is a histogram that corresponds to the 103 keV Line of Se-81. By looking at the statistics box in the thin window, the total number of counts can be found by the line labeled "Integral". The intrinsic error in the signal was found by expanding the channel axis by half of the standard deviation of the fit on each side to see how the number of counts varied as the size of the window was varied. A sample of a three channel window with counts $I_{Expanded}$ is shown in Figure \ref{3 Chan Sample Hist}. The purpose of the expansion was to see how inclusion of more channels affected the final result. \begin{figure}[h]
\centering
\includegraphics[width = 10cm]{Pictures/LB_Thesis_DataAnalysis_Sample_1Chan_Expwindow.png}
\caption{Pure selenium three channel window histogram}
\label{3 Chan Sample Hist}
\end{figure}
Unfortunately in this situation the keV resolution is too small, so the window can only be expanded by 1 on both sides. The systematic error in the signal can be estimated by \begin{equation}
e_I =| I_{Expanded} - I |
\end{equation}
while the statistical error is given by
\begin{equation}
\sigma_I = \sqrt{I}
\end{equation}
\subsection{Background Subtraction}
\hspace{0.5in} In the statistics box, the value labeled "background" corresponds to the constant value from Eq. 4.1 in the fitting function ($C_1$). To remove the background from the number of counts in the statistics box, the constant value must be integrated across the window of interest. In this case
\begin{equation}
Background = B =\int_{113}^{115} C_1 d(Chan) = n C_1
\end{equation}
where n is the width of the window (one, three, or ten). Since this reduces to multiplying the statistics box background value by a constant, the error can also be found by multiplying it by the same constant. In other words
\begin{equation}
\sigma_B = n\sigma_{C_1}
\end{equation}
Now that the value for the signal and the background have been found, they can be subtracted from one another. \begin{equation}
N = I - B
\end{equation} \begin{equation}
\sigma_N = \sqrt{I + \sigma_B^2}
\end{equation}
This value can be divided by the runtime (t) of the measurement to find the activity.
\begin{equation}
A_{Measured} = \frac{N}{t}
\end{equation}
Since the time interval for these measurements was recorded using a 20MHz clock, the error in the time is negligible compared to the error in the signal so it was not accounted for in this analysis. This means the error in the activity can be found by \begin{equation}
\sigma_{A_{Measured}} = \frac{\sigma_N}{t}
\end{equation}
\subsection{Decay During Measurements}
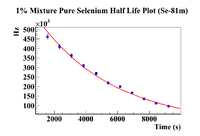
\hspace{0.5in} It is worth noting that the half life for Se-81 is rather short (57.28 min). For a 5 minute measurement, the activity decreases by
\begin{equation}
A(t) = A_0 e^{-\lambda_{Se-81 }300} = 0.94 A_0
\end{equation}
the activity decreases by roughly 6\% during the measurement. To remedy this, one can use the fact that the measurement being made is actually integrating the true value of the activity over the time of the measurement. In other words \begin{equation}
A_{Measure} = \frac{\int_0^t A_{True}e^{-\lambda t}dt}{t}
\end{equation}
By integrating this function and solving for the activity \begin{equation}
A_{True} = \frac{e^{\lambda t}A_{Measure}t \lambda}{e^{\lambda t} -1}
\end{equation}
The error can be found through standard error propagation methods to be \begin{equation}
\sigma_{A_{True}} = \sqrt{\left(\frac{\lambda t e^{\lambda t}\sigma_{A_{Measured}}}{e^{\lambda t}-1}\right)^2 + \left(\frac{A_{Measured}te^{\lambda t}(e^{\lambda t}-1-t\lambda)}{(e^{\lambda t}-1)^2}\right)^2\sigma_{\lambda}^2}
\end{equation}
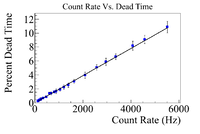
\subsection{Dead Time} \hspace{0.5in} Dead time is used to estimate the number of counts that may have been lost because the DAQ system was too busy recording the previous event. A count rate vs. dead time plot is shown below:
\begin{figure}[h]
\centering
\includegraphics[width = 6cm]{Pictures/LB_DeadTime_Thesis.png}
\caption{Detector $A$ Dead Time, \%D.T. = (0.01 $\pm$ 0.05) + (2.0 $\pm$ 0.1)$\times$(Count Rate)}
\label{fig:my_label}
\end{figure}
To account for the dead time, the total number of entries in the histogram was divided by the run time to get an average count rate. The plot was used to estimate the dead time. Once the dead time has been estimated, the correction is
\begin{equation}
A_{DTC} = \frac{A_{True}}{1-DT}
\end{equation}
Where DT is the dead time of the measurement. The error is given by
\begin{equation}
\sigma_{A_{DTC}} = \sqrt{\left(\frac{A_{True}\sigma_{DT}}{(1-DT)^2}\right)^2 + \left(\frac{\sigma_{A_{True}}}{(1-DT)}\right)^2}
\end{equation}
\subsection{Efficiency Corrections} \hspace{0.5in}The HpGe detector has two types of efficiency: geometric and energy. Mathematically, the efficiency corrections can be made by dividing $A_{DTC}$ by the efficiency ($\epsilon$). \begin{equation}
A_{\epsilon} = \frac{A_{DTC}}{\epsilon}
\end{equation}
\begin{equation}
\sigma_{A_{\epsilon}} = A_{\epsilon} \sqrt{\left(\frac{\sigma_{A_{DTC}}}{A_{DTC}}\right)^2 + \left(\frac{\sigma_{\epsilon}}{\epsilon}\right)^2}
\end{equation}
\subsection{Consistency Check} \hspace{0.5in} In order to ensure the calculations outlined above were applied correctly, measured activities were corrected back to the time of the previous post-irradiation activity measurement using a known half life. Once all the corrections have been made, the radioactive decay equation was used to trace the rate back to the previous measurement. For example, consider a measurement taken 500 seconds after the first measurement of the sample is taken. After the corrections have been made, the signal was corrected back using a 500 second decay time and compared to the previous activity measurement. \begin{equation}
A_{TC} = A_{\epsilon}e^{\lambda t}
\end{equation} \begin{equation}
\sigma_{A_{TC}} = \sqrt{\sigma_{A_{\epsilon}}^2e^{2\lambda t} + \sigma_{\lambda}^2A_{\epsilon}^2 t^2 e^{2\lambda t}}
\end{equation} Finally, take the ratio of the first measurement and the time corrected measurement to see if this number statistically agrees with unity.
\subsection{Multi-Channel Method Applied} \hspace{0.5in}The multi-channel window method was applied to attempt to trace the rate of the second measurement of the pure selenium sample #170048-001 back to the first and determine if they are in agreement with each other. The tables ~\ref{tabl:FirstMeasuredCorrection} and \ref{SecondMeasuredCorrection} show the values of the correction equations outlined above used on the first and second measurements of pure selenium respectively. The runtime was t=500 seconds for both measurements. The dead time correction used was $DT \pm \sigma_{DT}$ = $(0.84 \pm 0.16)\%$ for the first measurement and $DT \pm \sigma_{DT}$ = $(0.65 \pm 0.15) \%$ for the second measurement. The efficiency used was $\epsilon \pm \sigma_{\epsilon}$ = 0.08 $\pm$ 0.003 for both corrections because it was the same energy line at the same distance away from the detector.
\begin{table}[h]
\centering
\begin{tabular}{|c|c|c|} \hline
Value & Equations Used & Result \\ \hline
I $\pm \sqrt{I}$ & - & 1.1 $\times 10^5$ \\ \hline
$I_{Expanded}$ & - & 1.8 $\times 10^5$ \\ \hline
$e_I$ & 3.2 & 6.1 $\times 10^4$ \\ \hline
B & 3.4 &4.3 $\times 10^3$ \\ \hline
$\sigma_B$ & 3.5 & 25 \\ \hline
N & 3.6 & 1.1 $\times 10^5$ \\ \hline
$\sigma_N$ & 3.7 & 340\\ \hline
A &3.8 & 220\\ \hline
$\sigma_A$ &3.9 & 0.68 \\ \hline
$A_{True}$ & 3.12 & 230\\ \hline
$\sigma_{A_{True}}$ &3.13 &0.71 \\ \hline
$A_{DTC}$ & 3.14 & 230\\ \hline
$\sigma_{A_{DTC}}$ & 3.15 & 0.80\\ \hline
$A_{\epsilon}$ &3.16 & 2.9 $\times 10^5$ \\ \hline
$\sigma_{A_{\epsilon}}$ &3.17 & 9.5 $\times 10^3$ \\ \hline
\end{tabular}
\caption{Table of first measurement corrections}
\label{tabl:FirstMeasuredCorrection}
\end{table}
\begin{table}[h]
\centering
\begin{tabular}{|c|c|c|} \hline
Value & Equations Used & Result \\ \hline
I $\pm \sqrt{I}$ & -& 7.9 $\times 10^4$ \\ \hline
$I_{Expanded}$ & - & 1.3 $\times 10^5$\\ \hline
$e_I$ & 3.2 & 5.1 $\times 10^4$\\ \hline
B & 3.4 & 3.6 $\times 10^3$ \\ \hline
$\sigma_B$ &3.5 & 24 \\ \hline
N & 3.6 & 7.5 $\times 10^4$ \\ \hline
$\sigma_N$ & 3.7 & 2.8 $\times 10^2$\\ \hline
A & 3.8 & 150\\ \hline
$\sigma_A$ & 3.9 & 0.56 \\ \hline
$A_{True}$ & 3.12 & 160\\ \hline
$\sigma_{A_{True}}$ &3.13 & 0.59\\ \hline
$A_{DTC}$ &3.14 & 160 \\ \hline
$\sigma_{A_{DTC}}$ & 3.15 &0.64 \\ \hline
$A_{\epsilon}$ & 3.16 & 2.0 $\times 10^5$ \\ \hline
$\sigma_{A_{\epsilon}}$ & 3.17 & 6.5 $\times 10^3$ \\ \hline
\end{tabular}
\caption{Table of second measurement corrections}
\label{SecondMeasuredCorrection}
\end{table}
Now using equation 4.18, the second measurement can be time corrected back by 1235 seconds to find the rate at the time of the first measurement. Doing this gives $A_{TC} \pm \sigma_{A_{TC}} = 2.5 \pm 0.8 \times 10^5$. The ratio of the efficiency corrected activities gives a value of $0.90 \pm 0.05$, which is within two standard deviations of unity, hence they are in agreement.
\hspace{0.5 in} Once the corrections have been applied to all runs for a sample, a fit was plotted using the function below \begin{equation}
\ln{A_{\epsilon}(t)} = \ln{A_{\epsilon_0}} - \lambda t.
\end{equation} A linear fit was performed and the half life and the activity at the end of the irradiation of the sample was found using the fit parameters ($\lambda$ and $\ln{A_{\epsilon_0}}$). Note that $A_{\epsilon_0}$ is the activity at the beginning of the measurement. This can be corrected back to $t_{off}^{Beam}$ with equations 4.17 and 4.18.
\section{Signal to Noise Ratio Calculations} \hspace{0.5in} To find the signal to noise ratio at the time the beam was turned off, the background was analyzed to see the decay behavior. The background rate dropped by 62\% between the first and second measurement of the 1\% Se/Soil sample. This implied the background rate could not be considered constant and needed to be traced back to $t_{Beam Off}$.
\begin{figure}[h]
\centering
\includegraphics[width=8cm]{Chapters/LB_170049_Background_Overlay_xmgrace.png}
\caption{Background Decay}
\label{3 Chan Sample Hist}
\end{figure}
The background rate for the pure sample remained relatively constant when compared to the background rate for the mixture. Table \ref{Background Initial Activity} shows the initial activity of the background and the decay constants.
\begin{table}[h]
\centering
\begin{tabular}{|c|c|c|} \hline
Sample & Initial Background Activity (Hz) & Decay Constant ($s^{-1}$) \\ \hline
50\% Se/Soil & 8.25 $\pm$ 1.00 & 1.99 $\times 10^{-4} \pm$ 0.10\% \\ \hline
10\% Se/Soil & 25.03 $\pm$ 1.01 & 3.67 $\times 10^{-4} \pm$ 0.08\% \\ \hline
1\% Se/Soil & 108.31 $\pm$ 1.00 & 4.30 $\times 10^{-4} \pm$ 0.02\% \\ \hline
\end{tabular}
\caption{Background Initial Activity}
\label{Background Initial Activity}
\end{table}
These corrections were only applied to the signal to noise ratio calculation for Se-81m. Se-75 had a signal to noise ratio of less than one for all measurements corrected to $t_{Beam Off}$, so the signal to noise ratio was calculated based on measurements made in June 2017 by using equation 1.5. Both measurements of the background used in the signal to noise ratio were efficiency corrected as outlined in section 3.2.5. The SNR plot was fit with a line and solved for the point at which the fit function intersects with unity, which represents the minimum detectable concentration.
\begin{equation}
1 = mx + b
\end{equation} The error in this calculation was found by using the standard error propagation method.
\begin{equation}
\sigma_{SNR} = \sqrt{\frac{\sigma_m^2}{m^4} + \sigma_b^2}
\end{equation}
\newpage
\section{Graphs}
\newpage
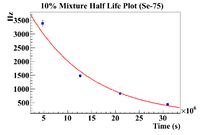
\begin{figure}[!tbp]
\centering
\begin{minipage}[b]{0.8\textwidth}
\includegraphics[width=\textwidth]{Pictures/LB_Thesis_170048_002_Se75_HLPlot.png}
\caption{10 \% Se/soil mix half life plot Se-75}
\end{minipage}
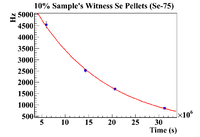
\hfill
\begin{minipage}[b]{0.8\textwidth}
\includegraphics[width=\textwidth]{Appendices/LB_Thesis_170048_001_Se75_HLPlot.png}
\caption{10\% mixture pure selenium witness pellet half life plot Se-75}
\end{minipage}
\end{figure}
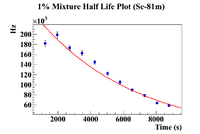
\newpage \begin{figure}[!tbp]
\centering
\begin{minipage}[b]{0.8\textwidth}
\includegraphics[width=\textwidth]{Chapters/LB_Thesis_170049_002_HLPlot_Se81m.png}
\caption{1 \% Se/soil mix half life plot Se-81m}
\end{minipage}
\hfill
\begin{minipage}[b]{0.8\textwidth}
\includegraphics[width=\textwidth]{Appendices/LB_Thesis_170049_001_HLPlot_Se81m.png}
\caption{1\% mixture pure selenium witness pellet half life plot Se-81m}
\end{minipage}
\end{figure}
\newpage \begin{figure}[!tbp]
\centering
\begin{minipage}[b]{0.8\textwidth}
\includegraphics[width=\textwidth]{Chapters/Se81m_HalfLives_AllChan_xmgrace.png}
\caption{Se-81m mixture half lives}
\end{minipage}
\hfill
\begin{minipage}[b]{0.8\textwidth}
\includegraphics[width=\textwidth]{Chapters/Se81m_PureSe_AllChan_xmgrace.png}
\caption{Se-81m pure witness Se half lives}
\end{minipage}
\end{figure}
\newpage \begin{figure}[!tbp]
\centering
\begin{minipage}[b]{0.8\textwidth}
\includegraphics[width=\textwidth]{Chapters/Se75MixtureHL_xmgrace_AllChan.png}
\caption{Se-75 mixture half lives}
\end{minipage}
\hfill
\begin{minipage}[b]{0.8\textwidth}
\includegraphics[width=\textwidth]{Chapters/Se75_PureSe_HalfLives_AllChan_xmgrace.png}
\caption{Se-75 pure witness Se half lives}
\end{minipage}
\end{figure}
(Should I increase these to 2 standard deviations for Chebychev's Rule?)
\clearpage
\section{Discussion of Errors}
\hspace{0.5in}There were two sets of errors within this experiment. The first set was produced by errors from irradiation which include beam energy, beam divergence, attenuation, and the relative position of the pure outer Selenium to the Selenium embedded within the soil matrix. The second set of errors were produced during the measurements using the HpGe detector, including unseen energy lines near the decay lines of Selenium originated from within the soil matrix and other noise from within the counting room.
\hspace{0.5in}For the first set, the simplest error was that Segebade used a beam energy of 30 MeV \cite{Reference1}, while this experiment used 32 MeV. This resulted in a change in expected energy of the Bremsstrahlung photons, which in turn changed the portion of the cross section included. The next source of error can be attributed the the divergence and attenuation of the beam. Since a pure Selenium sample was irradiated within the same target as the mixture, assuming the flux was the same, equations 1.4 simply becomes
\begin{equation}
\frac{N_{Se}^{Mix}}{N_{Se}^{Pure}} = \frac{n_T^{Se Mix} V_T^{Se Mix} }{n_T^{Se Pure} V_T^{Se Pure}}
\end{equation}
One would believe that ratio of the activity of the pure sample and the mixture would be unity if the flux through the target was the same. Unfortunately this was not the case, which would imply that the flux through the samples was not the same. An increase in the divergence and attenuation of the beam could also be attributed to the presence of other targets in front of mine. Another source of error was produced by the relative position of the pure outer Selenium and the Selenium within the soil matrix. Since the level of soil varied, it is highly possible that the Selenium within the mixture was higher or lower (relative to the beam axis) than the pure Selenium. This would impact the flux seen by both sets of Selenium.
\hspace{0.5in}During the HpGe measurement, one source of error could be the presence of lines produced from the soil matrix competing with the characteristic energy lines from Selenium. As a result the measurement would be higher than expected which would impact the number of counts seen within the chosen window width. Another source of error is simply the noise from within the hall. Many sources and samples were brought into and out of the hall which produced more noise to muddle the measurement.
Conclusion
\chapter{Conclusion}
\hspace{0.5in}The purpose of this experiment was to determine the minimum detectable concentration of Se-82 and Se-76 in soil. Photon activation analysis was used to measure this concentration by creating the unstable isotopes of Se-81m and Se-75. The observed minimum detectable concentration for Se-81m was 0.13 $\pm$ 0.06\% $\left ( 1300 \pm$ 600 \frac{\mu g}{g} \right )$, which is consistent with the inability to observe the Se-81m energy lines in the 0.1\% Se/Soil sample. The minimum detectable concentration for Se-75, as measured 56 days after irradiation, was a 0.005 $\pm$ 0.003\% ( 50 $\pm$ 30 $\frac{\mu g}{g}$). The Se-75 signal was still visible as of May of 2018.
Appendix A
\chapter{Detector Specifications}
\section{Energy Calibrations} \hspace{0.5in}The data acquisition system converts the analog signal from the HpGe detector's post amp into a channel number, but it is left to the experimenter to find out how to find which channel number corresponds to which energy. By using several calibrated sources with several characteristic energy lines spanning from 80-2000 keV, calibration constants can be found. Obviously, the more energy lines used will result with better fit parameters. Once the calibrated source has been measured by the HpGe detector, the energy lines of interest were fitted with a Gaussian function and the mean and its error were recorded. Plot the expected energy vs. the channel number with its error and fit the plot with a line. The equation of the line can be applied when plotting histograms to convert from channel number to energy via $$E = b + m(Channel)$$ where m and b are the linear fit parameters.
\hspace{0.5 in} It is worth noting that there could be some problems with binning when applying the energy calibrations, which is referred to as "picket fencing". This was due to the histogram bins being smaller than the hardware resolution.
\begin{figure}
\centering
\includegraphics[width = 10cm]{Pictures/170013_PicketFence_Thesis.png}
\caption{Picket fencing due to binning incompatibility}
\label{Picket Fence}
\end{figure}
This can be overcome by simply fitting the energy peak of interest to a Gaussian function, then applying the calibration to the mean of the fit.
\section{Efficiency} \hspace{0.5in} There are two types of efficiency for the HpGe detector: geometric and energy. Geometric efficiency deals with the detector's acceptance of radiation from a source as defined by the solid angle subtended by the detector. Energy efficiency deals with the detector's ability to accurately count photons of varying energies. To find the efficiency of the HpGe detector, the radiation was measured from calibrated sources with known activity and characteristic energy lines near the sample's characteristic energy lines (to account for the energy portion of the efficiency). The HpGe detectors have a track system set up near them. Samples can be placed on the track and the distance from the face of the detector to the sample can be varied up to one meter. Using this, make measurements at points of interest defined by where the irradiated samples were measured to account for the geometric efficiency. After the measurements, the experimenter can use the activation dates of the calibrated sources to find the activity of the source on the day of the efficiency measurement and take the ratio of the activity of the source seen by the HpGe detector and the actual activity of the calibrated source. A sample calculation is described below. Consider a Ba-133 source with an activity of 10.54 $\pm$ 3\% $\mu$Ci on its date of activation, which was July 1, 2008. The first step is to correct the activity to the date the efficiency measurement was taken, which in this case was May 27, 2017. To correct the activity to the day of the efficiency measurement, use equations
\begin{equation}
A_{TC} = A e^{- \lambda t}
\end{equation}
\begin{equation}
\sigma_{A_{TC}} = \sqrt{\sigma_A^2 e^{-2 \lambda t} + A^2t^2e^{-2 \lambda t} \sigma_{\lambda}^2}
\end{equation} . The correction yielded an activity of 5.87 $\pm$ 0.96 $\mu$Ci. Once the activity has been found, the theoretical value of the number of counts of an energy line given off by the source was calculated via \begin{equation}
A_{TC} \times BR \times (3.7 \times 10^{10} \frac{Hz}{Ci})
\end{equation} Where $A_{TC}$ is the time corrected activity and BR is the branching ratio of the decay. Next, the activity of a line of interest in a 10 channel window was measured and take the ratio between the theoretical and the actual activities to find the efficiency, or \begin{equation}
\epsilon = \frac{A_E}{A_T}
\end{equation} Where $A_E$ is the experimental activity measured by the HpGe detector and $A_T$ is the theoretical activity of the source. The error may be quantified by \begin{equation}
\sigma_{\epsilon} = A_{\epsilon}\sqrt{(\frac{\sigma_{A_{E}}}{A_E})^2 + (\frac{\sigma_{A_T}^2}{A_T^2})}
\end{equation} The table below shows the information of the sources used. The parentheses in the energy column represent the branching ratio for the decay.
\begin{table}[h]
\centering
\begin{tabular}{|c|c|c|c|c|} \hline
Source & Inventory Number & Activity ($\mu$Ci)& Irradiation Date & Energy (keV)\\ \hline
Ba-133 & 129791 & 10.54 $\pm$ 3\% & 07/01/08 & 81.00 (32.9\%) \\ \hline
Co-60 & 129790 & 10.42 $\pm$ 3\% & 07/01/08 & 1332.49 (99.98\%) \\ \hline
Co-60 & 129739 & 1.082 $\pm$ 3\% & 07/01/08 & 1332.49 (99.98\%) \\ \hline
\end{tabular}
\caption{Efficiency Sources}
\label{tab:my_label}
\end{table}
\begin{table}[h]
\centering
\rotatebox{90}{
\begin{tabular}{|c|c|c|c|c|c|c|} \hline
Serial &Date Measured & Corrected Activity ($\mu$Ci) & Position (cm) &$A_T$ (Hz) & $A_E$ (Hz) & \% $\epsilon$ \\ \hline
\hline
129791 &05/27/17 & 5.87 $\pm$ 0.18 & 90 & 71455.51 $\pm$2143.66 & 13.81 $\pm$ 0.26 & 0.02 $\pm$ 3.60 \% \\ \hline
129791 & 05/27/17 & 5.87 $\pm$ 0.18 & 70 & 71455.51 $\pm$ 2143.66 & 23.18 $\pm$ 0.29 & 0.03 $\pm$ 3.25 \% \\ \hline
129791 & 05/27/17 & 5.87 $\pm$ 0.18 & 50 & 71455.51 $\pm$ 2143.66 & 42.14 $\pm$ 0.49 & 0.06 $\pm$ 3.22 \% \\ \hline
129791 & 05/27/17 & 5.87 $\pm$ 0.18 & 44 & 71455.51 $\pm$ 2143.66 & 56.14 $\pm$ 0.67 & 0.08 $\pm$ 3.23 \%\\ \hline
129791 & 05/27/17 & 5.87 $\pm$ 0.18 & 30 & 71455.51 $\pm$ 2143.66 & 112.86 $\pm$ 1.41 & 0.16 $\pm$ 3.21 \% \\ \hline
129791 & 05/27/17 & 5.87 $\pm$ 0.18 & 20 & 71455.51 $\pm$ 2143.66 & 230.39 $\pm$ 1.53 & 0.32 $\pm$ 3.07\%\\ \hline
129791 & 09/06/17 & 5.75 $\pm$ 0.17 & 10 & 69994.75 $\pm$ 2069.41 & 642.33 $\pm$ 5.15 & 0.92 $\pm$ 3.10\%\\ \hline
129790 & 09/14/17 & 2.72 $\pm$ 0.15 & 70 & 100622.49 $\pm$ 5782.52 & 8.95 $\pm$ 0.18 & 0.0089 $\pm$ 6 \% \\ \hline
129790 & 09/14/17 & 2.72 $\pm$ 0.15 & 50 & 100622.49 $\pm$ 5782.52 & 17.18 $\pm$ 0.20 & 0.0170 $\pm$ 6\% \\ \hline
129790 & 09/14/17 & 2.72 $\pm$ 0.15 & 30 & 100622.49 $\pm$ 5782.52 & 42.45 $\pm$ 0.47 & 0.0422 $\pm$ 6\% \\ \hline
129790 & 09/14/17 & 2.72 $\pm$ 0.15 & 20 & 100622.49 $\pm$ 5782.52 & 85.20 $\pm$ 0.71 & 0.0847 $\pm$ 6\% \\ \hline
129739 & 09/15/17 & 0.283 $\pm$ 0.016 & 10 & 10469.187 $\pm$ 314.08 & 32.76 $\pm$ 0.338 & 0.31 $\pm$ 3\% \\ \hline
\end{tabular}
}
\caption{Table of Efficiencies}
\label{Efficiency table}
\end{table}
\section{Detector A Specifications} \hspace{0.5in} Below is a table showing the intrinsic properties of Detector A.
\begin{table}[h]
\centering
\begin{tabular}{|c|c|}
\hline
Serial No. & TP43076A \\ \hline
Relative $\epsilon$ @ 1.33MeV & 44\\ \hline
FWHM @ 1.33MeV & 1.93 \\ \hline
Ratio FW.1M FWHM & 2.0 \\ \hline
Ratio FW.02M FWHM & 2.9 \\ \hline
Peak to Peak Compton & 60\\ \hline
FWHM@122keV & 700 \\ \hline
Crystal Diameter (mm) & 66.7\\ \hline
Crystal Length (mm) & 49.2\\ \hline
\end{tabular}
\caption{Detector A specifications}
\label{Table A.3}
\end{table}
New SNR Section (8/18)
\section{Signal to Noise Ratio Calculations} \hspace{0.5in} Finding the correct value for the signal to noise ratio was done in three steps. Due to the fact that the specific activities were not all the same for the different normalization reference materials (in this case, the pure Se witness pellet), the first step was to take the weighted average of the specific activities and get normalization factors to force the specific activities to agree with the weighted average. The second step was to construct plots of $\frac{A_{Se Mix}}{a_{Se Pure}}$ to find the conccentration of Se that would need to be removed to have an activity of zero Hz at zero percent concentration of Se. Finally, $\frac{N}{B}$ was plotted as a function of the amount of Se spikant added plus the initial amount of Se that was present in the soil to begin with.
\hspace{0.5in} Table \ref{Se-75 Normalization Table} and Table \ref{Se-81m Normalization Table} outlines the normalization corrections that were made to the specific activity of the pure reference Se to force the denominator of $\frac{A_{Mix Se}}{a_{Pure Se}}$ to be the same across the board.
\begin{table}[h]
\centering
\begin{tabular}{|c|c|c|c|} \hline
Sample & Uncorrected $a_{Pure Se} (\frac{Hz}{g})$ & Normalization Factor & Corrected $\frac{A_Mix}{a_{Pure Se}}$ (g) \\ \hline
10\% & 6200 $\pm$ 190 & 0.25 $\pm$ 0.01 & 1.4 $\pm$ 0.08 \\ \hline
1\% & 5400 $\pm$ 170 & 0.29 $\pm$ 0.01 & 0.16 $\pm$ 0.01 \\ \hline
0.1\% & 1200 $\pm$ 37 & 1.3 $\pm$ 0.05 & 0.07 $\pm$ 0.004 \\ \hline
\end{tabular}
\caption{Table of Se-75 normalization corrections.}
\label{Se-75 Normalization Table}
\end{table}
\begin{table}[h]
\centering
\begin{tabular}{|c|c|c|c|} \hline
Sample & Uncorrected $a_{Pure Se} (\frac{Hz}{g})$ & Normalization Factor & Corrected $\frac{A_Mix}{a_{Pure Se}}$ (g) \\ \hline
10\% & (8.0 $\pm$ 0.3) $\times 10^5$ & 1.1 $\pm$ 0.04 & 0.17 $\pm$ 0.01 \\ \hline
1\% & (9.2 $\pm$ 0.3) $\times 10^5$ & 0.93 $\pm$ 0.04 & 0.04 $\pm$ 0.001 \\ \hline
\end{tabular}
\caption{Table of Se-81m normalization corrections.}
\label{Se-81m Normalization Table}
\end{table}
The 50\% sample was not included because the results were not physical. The signal to noise ratio in both cases was lower than the 10\% SNR. Due to the fact that the sample was very small when compared to the volume of the aluminum cylinder it was contained in, the lab technician may have placed the middle of the sample at the center of the beamline instead of the bottom. This would result in the sample not receiving as much flux as if it were correctly on axis. The 0.1\% was not analyzed for the Se-81m sample because the 103 keV line was not visible due to having to wait roughly two hours for the matrix to cool down enough to reach below 20\% dead time.
\hspace{0.5in} The next step was to plot the activity ratio as a function of concentration to identify the initial concentration of Se in the soil sample. The negative x-intercept represented this value.
\begin{figure}[h]
\centering
\includegraphics[width=10cm]{Chapters/A_Mix_Div_a_Pure_NormCorrected_Se75_NoFitRestrict_XYErrors_EqnDisplay.png}
\caption{Activity ratio for Se-75 (no fit restrictions)}
\label{Se75 Activity Ratio No Fit Restrict}
\end{figure}
\begin{figure}[h]
\centering
\includegraphics[width=10cm]{Chapters/A_Mix_Div_a_Pure_NormCorrected_Se81m_NoFitRestrict_XYErrors_EqnDisplay.png}
\caption{Activity ratio for Se-81m (no fit restrictions)}
\label{Se81m Activity Ratio No Fit Restrict}
\end{figure}
The negative x-intercepts here were too large to be physical. The Se-75 had a negative x intercept of (-0.44 $\pm$ 0.04)\%, while the Se-81m had a negative x intercept of (-1.75 $\pm$ 0.17)\%. A pure soil sample was studied and found neither the 265 keV Se-75 line nor the 103 keV Se-81m line, but according to this result, they should have been visible. The next step that was taken was do a bounded fit to ensure that $\frac{b}{m} < 0.1\%$. Below are the plots with the fit restriction
\begin{figure}[h]
\centering
\includegraphics[width=10cm]{Chapters/A_Mix_Div_a_Pure_NormCorrected_Se75_YintRestrict_XYErrors_EqnDisplay.png}
\caption{Activity ratio for Se-75 (fit restrictions).}
\label{Se75 Activity Ratio Fit Restrict}
\end{figure}
\begin{figure}[h]
\centering
\includegraphics[width=10cm]{Chapters/A_Mix_Div_a_Pure_NormCorrected_Se81m_Yint_FitRestrict_XYErrors_EqnDisplay.png}
\caption{Activity ratio for Se-81m (fit restrictions).}
\label{Se81m Activity Ratio Fit Restrict}
\end{figure}
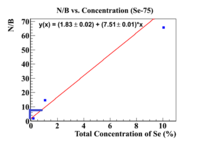
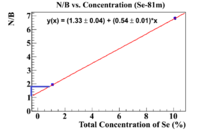
The new intercepts are (0.081 $\pm$ 0.003)\% for the Se-75 and (0.099 $\pm$ 0.005)\% for the Se-81m samples. The amount of spikant was added to the initial concentration of of Se in the soil and plotted against $\frac{N}{B}$
\begin{figure}[h]
\centering
\includegraphics[width=10cm]{Chapters/N_Div_B_ConcCorrectedbyFix_Se75_XYErrors_YintFix_EqnDisplay.png}
\caption{SNR vs. total concentration of Se-75.}
\label{SNR vs. total concentration of Se-75}
\end{figure}
\begin{figure}[h]
\centering
\includegraphics[width=10cm]{Chapters/N_Div_B_ConcCorrectedbyFix_Se81m_XYErrors_YintFix_EqnDisplay.png}
\caption{SNR vs. total concentration of Se-81m.}
\label{Se81m Activity Ratio Fit Restrict}
\end{figure}
The final step is to increase the y-intercept by two standard deviations and find the corresponding value on the x-axis via
\begin{equation}
x = \frac{b+2 \sigma - b}{m}
\end{equation}
The detection limit for Se-75 in soil is (0.005 $\pm$ 0.003)\% and the detection limit for Se-81m in soil is (0.13 $\pm$ 0.06)\%.