Difference between revisions of "Forest UCM Osc 2-DOsc"
| Line 16: | Line 16: | ||
you could define a relative phase between the two oscillators as | you could define a relative phase between the two oscillators as | ||
| − | :\delta = \delta_y - \delta_x | + | :<math>\delta = \delta_y - \delta_x</math> |
it can be substituted into the above equations by shifting the time origin (problem 5.15) | it can be substituted into the above equations by shifting the time origin (problem 5.15) | ||
| Line 38: | Line 38: | ||
::<math> =A_y \cos(\omega t^{\prime} - \omega \frac{-\delta_x}{\omega} - \delta_x)</math> | ::<math> =A_y \cos(\omega t^{\prime} - \omega \frac{-\delta_x}{\omega} - \delta_x)</math> | ||
::<math>= A_y \cos(\omega t^{\prime} +\delta_x - \delta_y)</math> | ::<math>= A_y \cos(\omega t^{\prime} +\delta_x - \delta_y)</math> | ||
| − | ::<math>= A_y \cos(\omega t^{\prime} | + | ::<math>= A_y \cos(\omega t^{\prime} -\delta)</math> |
==Interpretation== | ==Interpretation== | ||
Revision as of 15:45, 4 October 2014
Two Dimensional Oscillators
Isotropic Oscillator
The simplest 2-D oscillator that is composed of identical springs (same spring constant).
The equations of motion are separable two equations, one for each direction
you could define a relative phase between the two oscillators as
it can be substituted into the above equations by shifting the time origin (problem 5.15)
let
then
let
similarly
Interpretation
There are several modes of motion for the above system of equation
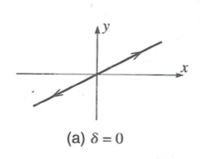
No phase difference
If there is no phase difference then (\delta =0 ) and you have oscillating motion along a line.
90 degree phase difference
If the x and y motions are completely out of phase ( phase difference of 90 degrees or )
then the motion is an ellipse (unless their amplitudes are equal in which case the mass moves in a circle).
If the phase shift is less than 90 then the ellipse is slanted towards the x-axis otherwise it is toward the y-axis.