Sadiq Proposal Defense

Abstract

Change "we" into "I" where needed to indicate that this is your Ph.D. proposal.

I am constructing an achromatic electron beam line to test positron production using the High Rep Rate Linac (HRRL) at Idaho State University's (ISU) Idaho Accelerator Center (IAC). I have studied the radiation impact of moving the cavity to new location with GEANT4 simulation and direct measurements. After new beamline was ready, I performed emittance measurements, and calculated emittance and twiss parameters. I acquired 4 NaI crystals from IAC. I built PMT bases for them and tested them, and they worked properly. I want place a tungsten target in a vacuum chamber between the 1st and 2nd quadrupole triplet sets. A dipole magnet was mapped and used to measure the beam emittance for different electron energies. I am expecting to collect positrons from the downstream side of the Tungsten target and transport them to a positron annihilation target at the end of the 90 degree beamline. Two NaI detectors at 90 degrees to the anihilation target are going to be used to detect 511 keV photons from positron annihilation, which will indicate the efficiency of generating positrons.

Introduction

I propose a measurement of the positron production efficiency using the High Repetition Rate Linac (HRRL) located at the Beam Lab of the Physics Department, at Idaho State University (ISU). The HRRL is an S-band (xx GHz) electron linear accelerator with the capabilities shown in Table 1.

Put these in Table 1  beam energy of 16 MeV, a maximum peak current of 80 mA,  a maximum  repetition rate of 1 kHz, and max pulse length of 250 ns (FWHM). 

Beam Energy	Max Peak Current	Repetition Rate	Wednesday	Thursday	Friday
16 MeV	80 mA	1 kHz	Max Pulse Length	250 ns (FWHM)

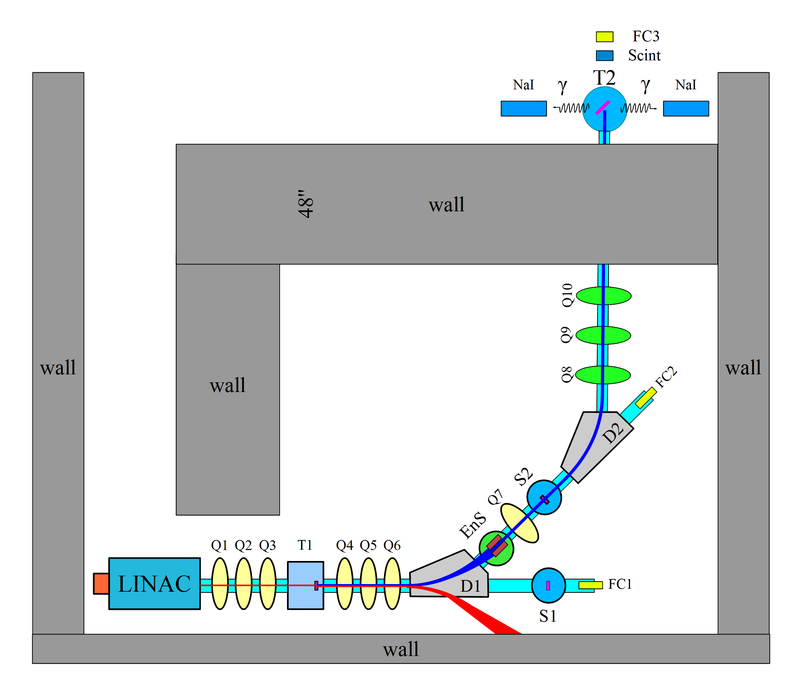
As shown in FIgure 1, the Beam Lab, located in the basement of ISU physical sciences complex, is divided into two parts by a L-shaped cement wall. The accelerator cell houses the cavity and magntic elements needed to transport electrons to an experimental cell. The experimental cell is located in an adjacent room to the accelerator cell. The HRRL beamline was reconfigured into an achromat by moving the accelerator cavity to accomdate two dipoles and a system of quadrupole magnets optimized for collecting positrons.

In the new beamline, the electron beam exits the HRRL cavity and passes through first the set of quadruple triplet magnets used to focus the electron beam onto the positron target. Positrons exiting the downstream side of the positron target will be collected by second set of quadruple triplet optimized to collect positrons. A dipole magnet selects the positrons or electrons exiting the quadrupole triplet bending them by 45 degrees towards a second dipole magnet. The polarity of the first dipole is used to select either positrons or electrons. The second dipole will bend the beam another 45 degrees, thus completing a 90 degree bend. A third quadruple triplet will be used focus the e-/e+ beam profile as needed.

Fig. HRRL beamline for positron generation.

This is the setup and apparatus that I want run for positron creation and collection.

Item	Description
T1	Positron target
T2	Annihilation target
EnS	Energy Slit
F1, F2, F3	Flang
FC1, FC2	Faraday Cup
Q1,...Q10	Quadrupole
D1, D2	Dipole
NaI	NaI Detecotrs
OTR	OTR screen
YAG	YAG screen

Earlier measurements were conducted at Idaho Accelerator Center of ISU, May of 2008. Setup are shown in figure below. 511 keV photons from annihilation were detected.

Item	Description
Tantalum Foil	6 mm thick 20 mm x 20 mm area
Tungsten Foil	2 mm thick 20 mm x 20 mm area
Phosphorus Flag	1 mil aluminum backing
Media:HpGe_Crystal_GEM-60195-Plus-P.pdf	81.3mm Diameter, 55.5mm Length
NaI detector

Fig. Setup for 2009 run.

Fig. Spectrum from HpGe Detector and NaI detecotrs.

Fig.

Experiments

HRRL Emittance measurements

Emittance is an important parameter in accelerator physics. If emittance with twiss parameters are given at the exit of the gun, we will be able to calculate beam size and divergence any point after the exit of the gun. Knowing the beam size and beam divergence on the positron target will greatly help us study the process of creating positron. Emittance with twiss parameters are also key parameters for any accelerator simulations. Also, energy and energy spread of the beam will be measured in the emittance measurement.

What is Emittance

Fig.1 Phase space ellipse <ref name="MConte08"></ref>.

In accelerator physics, Cartesian coordinate system was used to describe motion of the accelerated particles. Usually the z-axis of Cartesian coordinate system is set to be along the electron beam line as longitudinal beam direction. X-axis is set to be horizontal and perpendicular to the longitudinal direction, as one of the transverse beam direction. Y-axis is set to be vertical and perpendicular to the longitudinal direction, as another transverse beam direction.

For the convenience of representation, we use [math]z[/math] to represent our transverse coordinates, while discussing emittance. And we would like to express longitudinal beam direction with [math]s[/math]. Our transverse beam profile changes along the beam line, it makes [math]z[/math] is function of [math]s[/math], [math]z~(s)[/math]. The angle of a accelerated charge regarding the designed orbit can be defined as:

[math]z'=\frac{dz}{ds}[/math]

If we plot [math]z[/math] vs. [math]z'[/math], we will get an ellipse. The area of the ellipse is an invariant, which is called Courant-Snyder invariant. The transverse emittance [math]\epsilon[/math] of the beam is defined to be the area of the ellipse, which contains 90% of the particles <ref name="MConte08"> M. Conte and W. W. MacKay, “An Introduction To The Physics Of Particle Accelera tors”, World Scientifc, Singapore, 2008, 2nd Edition, pp. 257-330. </ref>.

By changing quadrupole magnetic field strength [math]k[/math], we can change beam sizes [math]\sigma_{x,y}[/math] on the screen. We make projection to the x, y axes, then fit them with Gaussian fittings to extract rms beam sizes, then plot vs [math]\sigma_{x,y}[/math] vs [math]k_{1}L[/math]. By Fitting a parabola we can find constants [math]A[/math],[math]B[/math], and [math]C[/math], and get emittances.

Emittance Measurement Using a YaG crystal

In July 2010y, Emittance measurement of HRRL was conducted at Beam Lab, at Physics Department of ISU. I installed a YAG crystal on the HRRL beam line to see electron beam shape. A quadrupole magnet was installed between HRRL gun and the YAG screen. I changed current on the quadrupole to control magnetic field strength of the quadrupole magnet, this changed electron beam shape on the YAG screen.

Experimental Setup

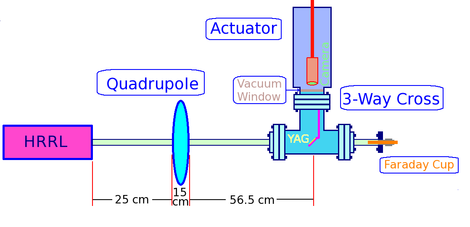
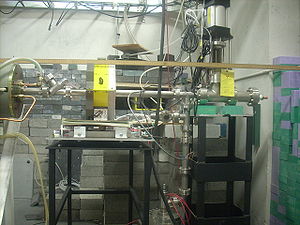
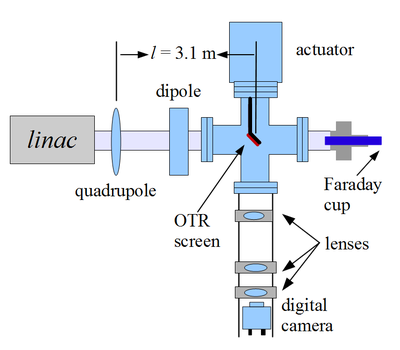
I did quadrupole scan to measure emittance of the electron beam in HRRL. In quadrupole scan method, the strength of the quadrupole magnet was changed by changing the current go through quadrupole coils. The electron beam were coming out of the gun went through quadrupole, then beam would enter a 3-way cross. Two end of the 3-way cross was installed on the beam line. The third end of the 3-way cross was placed upward and there was a actuator installed to it. The YAG crystal was mounted in the actuator, which can put the YAG in the beam line or take it out of the beam line. A camera was placed inside the actuator to look through vacuum a window and to capture the image on the YAG crystal created by electron beam. A Faraday cup was mounted at the end of the beam line to measure the transmission of the charge.

Setup and beam line and are shown in figures 1.2 and 1.3:

Fig.2 Experiment set up of HRRL 2010 July emittance test.

Fig.3 Beam Line of HRRL 2010 July emittance test.

Figures 4, 5, and 6 show Faraday cup, Quadrupole Magnet, and YAG Chrystal used in the test:

Fig.4 Faraday cup used for HRRL 2010 July emittance test.

Fig.5 Quadrupole Magnet used for HRRL 2010 July emittance test.

Fig.6 YAG Christal used for HRRL 2010 July emittance test.

Emittance measurement was carried out on HRRL on July of 2010 under the experimental setup discussed in previous section. In this measurement I used analog camera to take images.

When relativistic electron beam pass through YAG target, Opitcal Transition Radiation (OTR) is produced. OTR are taken for different quadrupole coil current (0-20 A).

Fig. OTR image of 0 Amp quadrupole coil current.	Fig. OTR image of 5 Amp quadrupole coil current.	Fig. OTR image of 10 Amp quadrupole coil current.
Fig. OTR image of 15 Amp quadrupole coil current.	Fig. OTR image of 20 Amp quadrupole coil current.	Fig. OTR image of -10 Amp quadrupole coil current.

Data Analysis

Fig. Gaussian fits for OTR images.

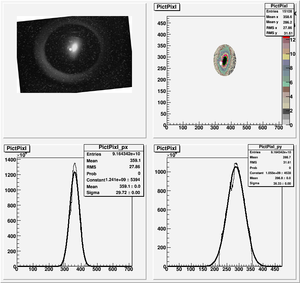
In the mages we can see a bright spot at the center. This spot did not change by changing quad coil current. So, this is image of hot filament. The bigger spot at the right side of the filament was changing by changing quad coil current, hence it is OTR. We also see 10 mm circle mounted on the OTR target, as well as beam hallow.

I did Guassian fits to beam image to extract x, y RMS values for different quad currents. I found out that the camera was rotated slightly. To compensate for it, images were rotated back, and I had beam image upright. To reduce back ground, I just focused on OTR beam image and took out the filament spot from data, as shown in image below.

Results

Fig. Square of RMS beam size [math] \sigma_x^2 [/math] vs. quad strength times quad pole length [math] k_1L [/math] for x projection of electron beam profile

Fig. Square of RMS beam size [math] \sigma_x^2 [/math] vs. quad strength times quad pole length [math] k_1L [/math] for y projection of electron beam profile

[math] \sigma_x^2= (8.05 \pm 0.25) + (4.18 \pm 0.19)k_1L + (0.64 \pm 0.034)(k_1L)^2 [/math]

[math] \epsilon_x = 2.2 \pm 1.3~mm*mrad ~\Rightarrow~ \epsilon_{n,x} = 42.4 \pm 25.4~mm*mrad[/math]

[math] \beta_x=0.72 \pm 0.31, \alpha_x=-1.23 \pm 0.56 [/math]

[math]\sigma_y^2 = (8.52 \pm 0.40) + (-3.88 \pm 0.28)k_1L + (0.57 \pm 0.048)(k_1L)^2 [/math]

[math] \epsilon_y = 2.6 \pm 2.0~mm*mrad ~\Rightarrow~ \epsilon_{n,y} = 50.5 \pm 38.3~mm*mrad[/math]

[math] \beta_y=0.54 \pm 0.22, \alpha_y=2.68 \pm 1.13 [/math]

Emittance Measurements with an OTR

During first emittance measurement, we see our electron beam image at YAG crystal is much bigger than expected. Comparison study shows that for same beam YAG screen shows bigger beam size than Optical Transition Radiation (OTR) screen. Yag is good for low charge beam. Electron beam from HRRL has a big charge in a single pulse and beam size is big.

I did second emittance measurement with 10 [math]\mu m[/math] thick aluminium OTR screen. I also improved our optical imaging system by using better digital camera that can be triggered by the same pulse trigger electron gun. Also I used three 2-inch diameter lenses to focus the lights from OTR to the CCD of the camera.

Experimental Setup

Fig. Simplified setup configuration for HRRL beamline at 2011 March measurement.

The cavity was moved the new location and beam line was built by the design of Dr. Yujong Kim, as shown in figure

Experimental setup is shown in the following figure

I chose one of the quad at a time to do the scan and turned off all the other quads. Optical transition radiation was observed at OTR target. At the end of 0 degree beamline I had a Faraday cup to measure the charge of the beam. Camera cage system was located below the OTR target. There are 3 lenses used to focus lights from target to the CCD camera. Target can be pushed into or taken out of the beamline by the actuator at the top.

OTR Target can be pushed into or taken out of the beamline vertically by the actuator at the top, which is attached to the 6-way cross. This actuator controlled remotely at the control desk.

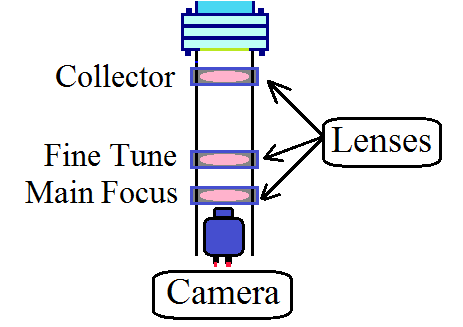
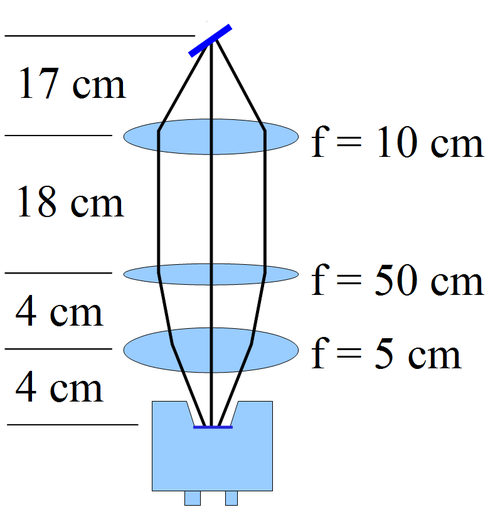
Camera cage system was located below the OTR target. Cage system attached to the bottom of the 6-way cross. Lower end of cross is a transparent window. There are 3 lenses used to focus lights from target to the CCD camera. They have focal length of 100 mm, 500 mm, and 50 mm.

The lens closest to the OTR target is 10 cm away from the target, an it has 100 mm focal length. This lens was located as close to the target as possible, so that we might collect as much OTR light as possible, and it was thus called collector lens. The lens in the middle has focal length of 500 mm. Moving this lens will change total focal length in a small amount, and this allow us to do fine tuning. Thus, we called this lens fine tuning lens. The last lens, which is furthest from target, and closed to the CCD camera has the shortest focal length of 5o mm. Its short focal length allow us to focus the light on the very small sensing area of the CCD camera.

Fig. HRRL beamline: Optical cage system to focus OTR lights on CCD camera.

Fig. HRRL beamline: Optical lenses lay out for optical cage system.

Experiment with OTR

Optical Transition Radiation (OTR): Transition radiation is emitted when a charge moving at a constant velocity cross a boundary between two materials with different dielectric constant.

Emittance measurement was carried out on HRRL on March of 2011 under the experimental setup discussed in previous section. In this measurement I used JAI digital camera.

When relativistic electron beam pass through Aluminum target OTR is produced. OTR are taken for different quadrupole coil currents.

Fig. OTR image of 0 Amp Q1 coil current.

Fig. OTR image of +1 Amp Q1 coil current.

Fig. OTR image of +2 Amp Q1 coil current.

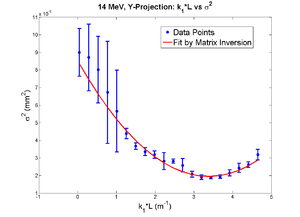
Data Analysis and Results

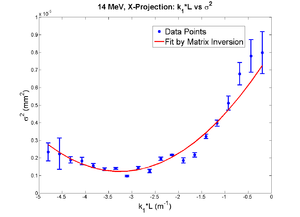
The projection of the beam is not Gaussian distribution. So, I fit Super Gaussian fitting <ref name="BeamDisBeyondRMS"> F.-J Decker, “Beam Distributions Beyond RMS”, BIW94, Vancouver,CA,Sep 1994, . </ref>.

I used the MATLAB to analyze the data. The results shows that:

[math] \sigma_x^2= (3.678 \pm 0.022) + (-4.17 \pm 0.22)k_1L + (5.55 \pm 0.42)(k_1L)^2 [/math]

[math] \epsilon_x = 0.417 \pm 0.023~mm*mrad ~\Rightarrow~ \epsilon_{n,x} = 11.43 \pm 0.64~mm*mrad[/math]

[math] \beta_x=1.385 \pm 0.065, \alpha_x=0.97 \pm 0.07 [/math]

[math]\sigma_y^2 = (2.843 \pm 0.044) + (1.02 \pm 0.52)k_1L + (3.8 \pm 1.2)(k_1L)^2 [/math]

[math] \epsilon_y = 0.338 \pm 0.065~mm*mrad ~\Rightarrow~ \epsilon_{n,y} = 9.3 \pm 1.8~mm*mrad[/math]

[math] \beta_y=1.17 \pm 0.19, \alpha_y=0.22 \pm 0.10 [/math]

Positron Detection using NaI crystal

Fig. NaI detector base circuit.

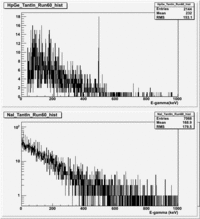
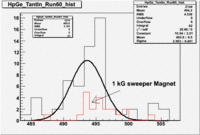
To detect positrons created, I want a put Tungsten target at the end of 90 degree beamline. When positrons hit W-target, 511 keV photons will be created. I want to use NaI detectors to detect these 511 keV photons, so I might have an estimate on the numbers of the positrons I collect at the end of the 90 degree beamline.

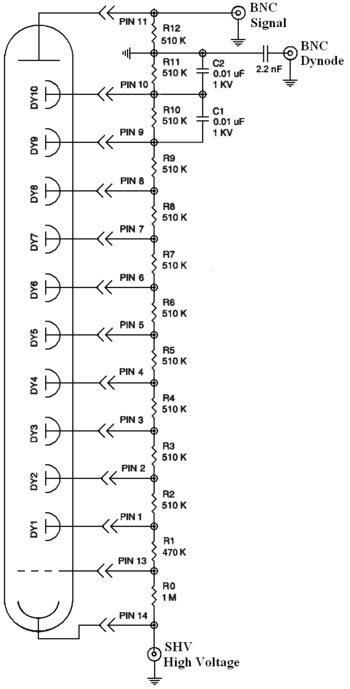
I acquired some NaI crystals from Idaho Accelerator Center (IAC). I built our own PMT bases for them, since their own bases not working properly. I modified the design of model PA-14 from Saint-Gobain crystals & detectors ltd. Following image is the design.

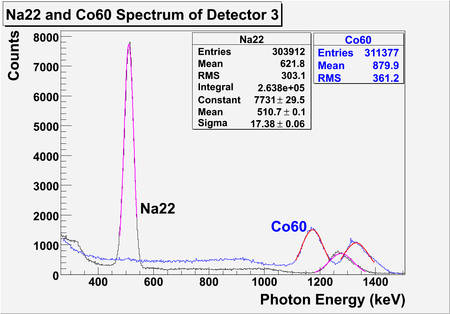
Even though now the 511 keV peak seems to be very wide and our resolution is low, I are expecting to improve these by doing coincidence in the future experiments.

Future Plan

Positron Target

A tungsten target will be placed in the space between 1st and 2nd triplet. The tungsten target will be placed inside a big chamber.

Emittance Tests with Energy Scan

I want to do emittance test with precise energy scan. I remapped dipole magnet for more precise energy scan. This will also improve our emittance measurement.

Positron Yield

I will insert the first tungsten target and create positrons. I am expecting to collect part these positrons and transport them down the second tungsten target at the end of the 90 degree beamline. By doing these, 511 keV photons will be created and I want to detect them by our NaI detectors.

References

<references/>

File:Emittance.tex