Collimation geometry for different beam energies

From New IAC Wiki
Jump to navigation Jump to search

Go Back


90[math]\displaystyle{ ^o }[/math] exit port measurements



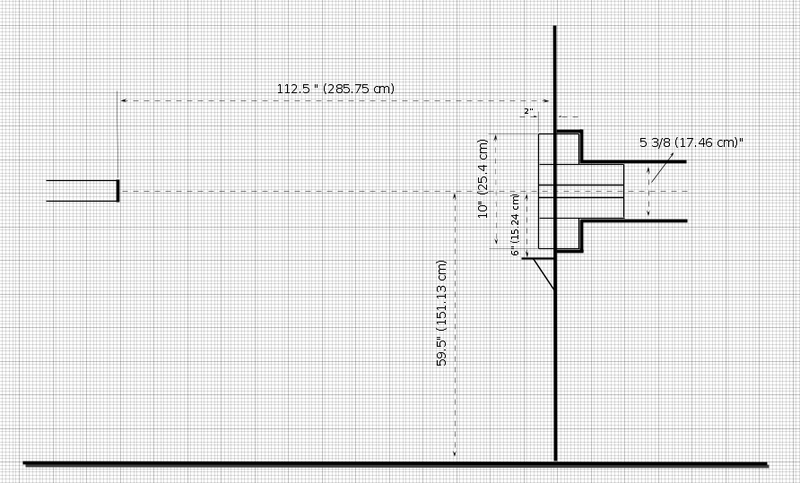
Critical and Kicker angles


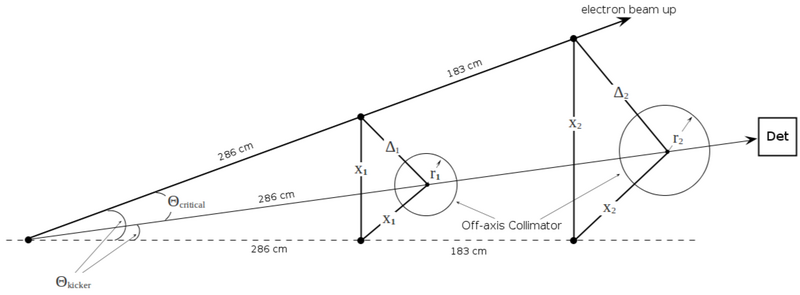
[math]\displaystyle{ \Theta_C = \frac{m_ec^2}{E} }[/math]

[math]\displaystyle{ \Theta_K = tan^{-1}\left(\frac{x_1}{286}\right)
                = tan^{-1}\left(\frac{1}{\sqrt{2}}\ \frac{\Delta_1}{286}\right)
                = tan^{-1}\left(\frac{1}{\sqrt{2}}\ tan(\Theta_C)\right) }[/math]

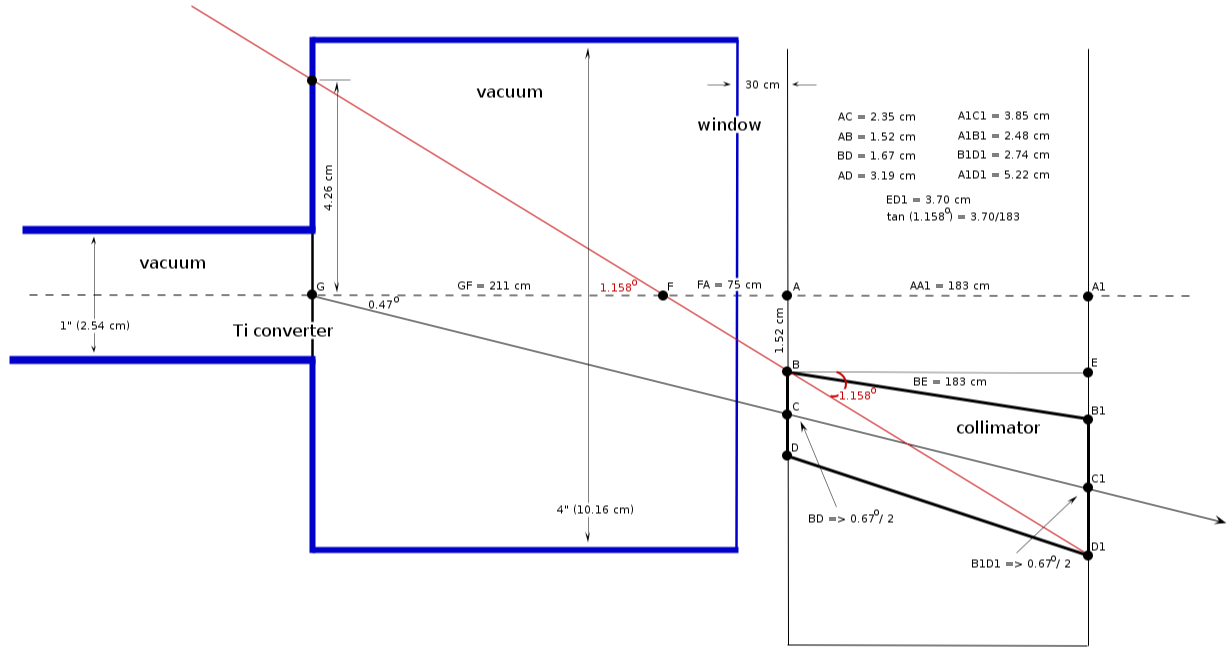
Geometry calculation


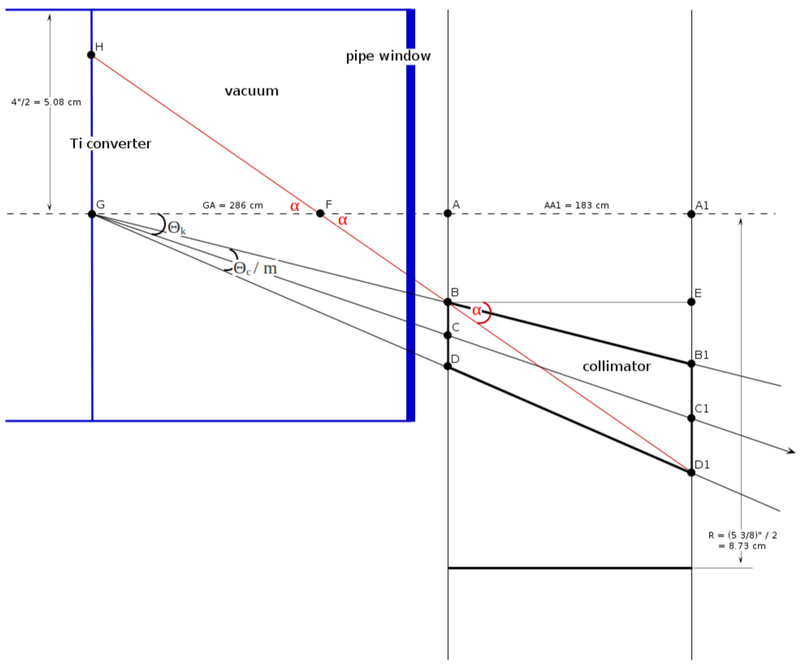
beam energy [math]\displaystyle{ \Theta_C / m }[/math] [math]\displaystyle{ \Theta_C }[/math] [math]\displaystyle{ \Theta_K }[/math] [math]\displaystyle{ \alpha }[/math] [math]\displaystyle{ AC }[/math] [math]\displaystyle{ A_1C_1 }[/math] [math]\displaystyle{ BD }[/math] [math]\displaystyle{ B_1D_1 }[/math] [math]\displaystyle{ A_1D_1 }[/math] [math]\displaystyle{ FA }[/math] [math]\displaystyle{ GH }[/math]
25 MeV [math]\displaystyle{ \Theta_C / 2 }[/math] [math]\displaystyle{ 1.17^o }[/math] [math]\displaystyle{ 0.83^o }[/math] [math]\displaystyle{ 2.03^o }[/math] 4.13 cm 6.78 cm 2.92 cm 4.79 cm 9.18 cm > 8.73 cm 75 cm 7.49 cm > 5.08 cm
25 MeV [math]\displaystyle{ \Theta_C / 3 }[/math] [math]\displaystyle{ 1.17^o }[/math] [math]\displaystyle{ 0.83^o }[/math] [math]\displaystyle{ 1.63^o }[/math] 4.13 cm 6.78 cm 1.95 cm 3.20 cm 8.37 cm < 8.73 cm 111 cm 4.99 cm < 5.08 cm
25 MeV [math]\displaystyle{ \Theta_C / 4 }[/math] [math]\displaystyle{ 1.17^o }[/math] [math]\displaystyle{ 0.83^o }[/math] [math]\displaystyle{ 1.43^o }[/math] 4.13 cm 6.78 cm 1.46 cm 2.40 cm 7.98 cm < 8.73 cm 136 cm 3.74 cm < 5.08 cm
44 MeV [math]\displaystyle{ \Theta_C / 2 }[/math] [math]\displaystyle{ 0.67^o }[/math] [math]\displaystyle{ 0.47^o }[/math] [math]\displaystyle{ 1.16^o }[/math] 2.35 cm 3.85 cm 1.66 cm 2.72 cm 5.21 cm < 8.73 cm 75 cm 4.26 cm < 5.08 cm
44 MeV [math]\displaystyle{ \Theta_C / 3 }[/math] [math]\displaystyle{ 0.67^o }[/math] [math]\displaystyle{ 0.47^o }[/math] [math]\displaystyle{ 0.93^o }[/math] 2.35 cm 3.85 cm 1.11 cm 1.82 cm 4.76 cm < 8.73 cm 111 cm 2.84 cm < 5.08 cm
44 MeV [math]\displaystyle{ \Theta_C / 4 }[/math] [math]\displaystyle{ 0.67^o }[/math] [math]\displaystyle{ 0.47^o }[/math] [math]\displaystyle{ 0.81^o }[/math] 2.35 cm 3.85 cm 0.83 cm 1.36 cm 4.53 cm < 8.73 cm 136 cm 2.13 cm < 5.08 cm


25 MeV geometry

[math]\displaystyle{ \Theta_c/4 }[/math], pipe 3"


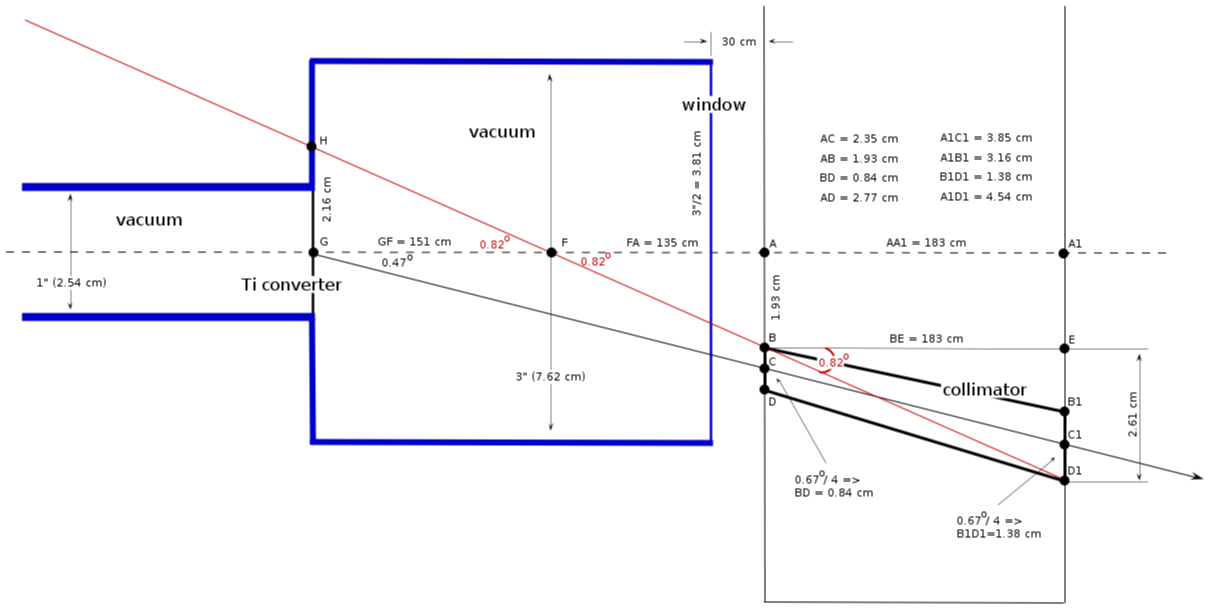
[math]\displaystyle{ \Theta_c/2 }[/math], box 3"x4" and then pipe 4"

need to adjust to converter position



Go Back

Retrieved from "https://wiki.iac.isu.edu/index.php?title=Collimation_geometry_for_different_beam_energies&oldid=52530"