Counts Rate (44 MeV LINAC)

Go Back

Counts Rate for U238

LINAC parameters used in calculations

1) pulse width 50 ns
2) pulse current 50 A
3) repetition rate 300 Hz
4) energy 44 MeV

Number of electrons/sec on radiator

[math] 50\ \frac{Coulomb}{sec} \times \frac{1\cdot e^-}{1.6\cdot 10^{-19}C} \times 50ps \times 300Hz = 0.47 \cdot 10^{13} \frac{e^-}{sec}[/math]

Number of photons/sec from radiator

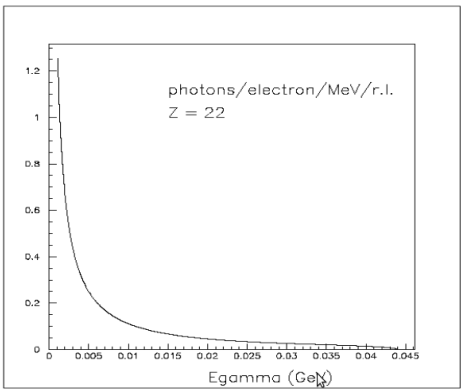
bremsstrahlung

in (10,20) MeV region we have about

    0.1 photons/electrons/MeV/r.l

radiation length

r.l.(Ti) = 3.59 cm

radiator thickness = 12.5 [math]\mu m[/math]

[math]12.5\mu m/3.59 cm = 3.48 \cdot 10^{-4} \ r.l.[/math]

steps together...

[math]0.1\ \frac{\gamma 's}{(e^- \cdot MeV \cdot r.l.)} \times 3.48 \cdot 10^{-4} r.l. \times 10\ MeV \times 0.47 \cdot 10^{13} \frac{e^-}{sec}=1.64 \cdot 10^{9} \frac{\gamma}{sec}[/math]

Collimation factor

Collimation factor is

    4-6 % of total # of photons (Alex, GEANT calculation)

then, incident flux on target is

[math]1.64 \cdot 10^{9} \frac{\gamma}{sec} \cdot 5% = 8.2 \cdot 10^{7} \frac{\gamma}{sec}[/math]

Number of neutrons/sec (yields)

photonuclear cross section for [math]^{238}U(\gamma , n)[/math] reaction

From the paper "Giant resonance for the actinide nuclei: Photoneutron and photofission cross sections for 235U, 236U, 238U, and 232Th", J. T. Caldwell and E. J. Dowdy, B. L. Berman, R. A. Alvarez, and P. Meyer. Physical Review C, (21), 1215, April 1980:

in (10,20) MeV region the average cross section, say, is:

    130 mb

target thickness, [math]^{238}U[/math]

[math]\frac{19.1\ g/cm^3}{238.02\ g/mol} = 0.08\ \frac{mol}{cm^3} = 0.08\ \frac{mol}{cm^3} \times \frac{6.02\cdot 10^{23}\ atoms}{mol} = 0.48\cdot 10^{23}\ \frac{atoms}{cm^3}[/math]

Target thickness = 1 cm:

[math]0.48\cdot 10^{23}\ \frac{atoms}{cm^3} \times 1\ cm = 0.48\cdot 10^{23}\ \frac{atoms}{cm^2}[/math]

neutrons per fission

   2.4 neutrons/fission

steps together...yeild

[math] Y = \frac{\gamma}{sec} \times t \times \sigma \times 2.4 = [/math]

[math] = 8.2 \cdot 10^{7} \frac{\gamma}{sec} \times 130\ mb \times 0.48\cdot 10^{23}\ \frac{atoms}{cm^2} \times 2.4 = 1.2 \cdot 10^{6}\ \frac{neutrons}{sec}[/math]

Worst Case Isotropic Neutrons

Let's say we have:

radius detector = 1 cm

1 meter away

fractional solid angle = [math]\frac{\pi * (1 cm)^{2}}{4 \pi (100cm)^{2}} = \frac{1}{4} \cdot 10^{-4}[/math] <= geometrical acceptance

Yield

the yield per second:

[math]1.2 \cdot 10^{6}\ \frac{neutrons}{sec} \times \frac{1}{4} \cdot 10^{-4} = 30\ \frac{neutrons}{sec} [/math]

the yield per pulse:

[math] 30\ \frac{neutrons}{sec} \times \frac{1\ sec}{300\ pulses} = 0.1\ \frac{pulses}{sec}/ [/math] <= good for stopping pulse

Therefore, this experiment is doable.

Counts Rate for Deuteron

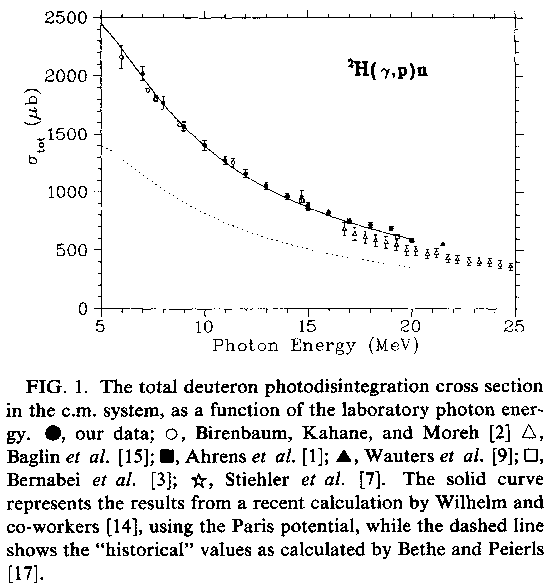
photonuclear cross section for [math]^2H(\gamma , n)[/math] reaction=

From the paper "absolute total cross sections for deuteron photodisintegration between 7 and 19 MeV", A. De Graeva and other. Physical Review C, (45), 860, February 1992:

in (10,20) MeV region the average cross section, say, is:

    1000 mb

Calibration factor

The only difference from calculations above is the cross section:

     1000 mb / 130 mb = 7.7

Yield

Let's say all other factors is the same =>

the yield is :

[math] 30\ \frac{neutrons}{sec} \times 7.7 = 231\ \frac{neutrons}{sec} [/math]

Go Back