Lab 4 RS
- RC High-pass filter
1-50 kHz filter (20 pnts)
1. Design a high-pass RC filter with a break point between 1-50 kHz. The break point is the frequency at which the filter's attenuation of the AC signal goes to 0(not passed). For a High pass filter, AC signals with a frequency below the 1-50 kHz range will be attenuated .
- To design low-pass RC filter I had:
So
2. Now construct the circuit using a non-polar capacitor.
3. Use a sinusoidal variable frequency oscillator to provide an input voltage to your filter.
4. Measure the input and output voltages for at least 8 different frequencies which span the frequency range from 1 Hz to 1 MHz.
| 0.5 | 12.4 | 0.48 | 0.039 |
| 1.0 | 11.0 | 0.88 | 0.080 |
| 2.0 | 8.0 | 1.3 | 0.162 |
| 3.0 | 6.0 | 1.5 | 0.250 |
| 4.0 | 4.9 | 1.6 | 0.326 |
| 5.0 | 4.2 | 1.7 | 0.405 |
| 6.0 | 3.7 | 1.7 | 0.459 |
| 7.0 | 3.2 | 1.7 | 0.531 |
| 8.0 | 3.0 | 1.75 | 0.583 |
| 9.0 | 2.85 | 1.70 | 0.596 |
| 10.0 | 2.60 | 1.70 | 0.654 |
| 11.0 | 2.50 | 1.70 | 0.680 |
| 12.0 | 2.35 | 1.70 | 0.723 |
| 13.0 | 2.25 | 1.70 | 0.755 |
| 14.0 | 2.20 | 1.70 | 0.772 |
| 15.0 | 2.10 | 1.70 | 0.809 |
| 20.0 | 2.00 | 1.75 | 0.875 |
| 30.0 | 1.85 | 1.75 | 0.946 |
| 50.0 | 1.82 | 1.78 | 0.978 |
| 100.0 | 1.80 | 1.80 | 1.00 |
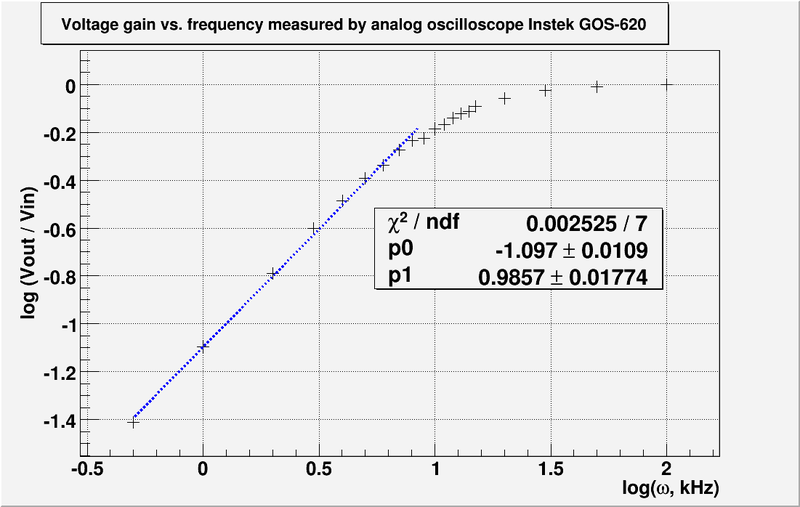
5. Graph the -vs-
phase shift (10 pnts)
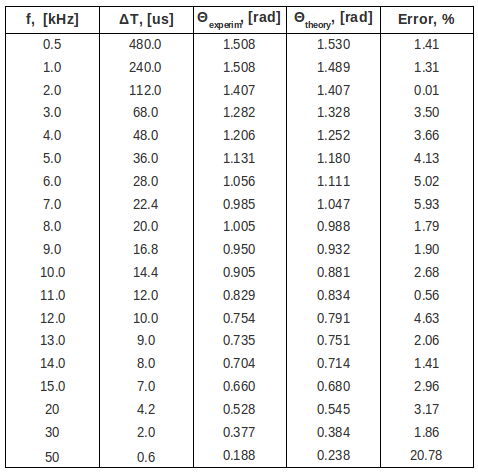
- measure the phase shift between and as a function of frequency . Hint: you could use as an external trigger and measure the time until reaches a max on the scope .
See question 3 about my phase shift measurements
Questions
1. Compare the theoretical and experimentally measured break frequencies. (5 pnts)
Theoretical break frequency:
The fit line equation from the plot above is . From intersection point of line with x-axis we find:
The error is:
2. Calculate and expression for as a function of , , and .(5 pnts)
We have:
Dividing second equation into first one we get the voltage gain:
And we are need the real part:
3. Compare the theoretical and experimental value for the phase shift . (5 pnts)
The experimental phase shift is
The theoretical phase shift is
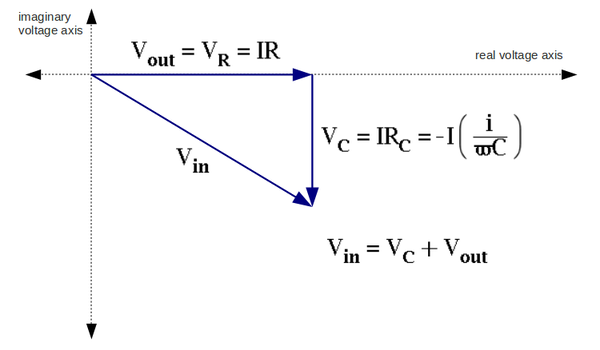
4. Sketch the phasor diagram for ,, , and . Put the current along the real voltage axis. (30 pnts)
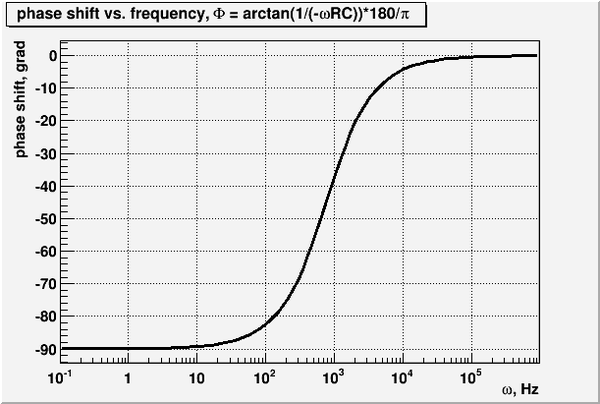
5. What is the phase shift for a DC input and a very-high frequency input?(5 pnts)
Because a DC circuit doesn't have any oscillation there are no any phase shift.
For a very high frequency input the phase shift is 0 (see plot in question 6)
6. Calculate and expression for the phase shift as a function of , , and graph -vs . (20 pnts)
From the phasor diagram above (question 4) the angle between vectors and given by
Forest_Electronic_Instrumentation_and_Measurement Go Back to All Lab Reports