Limits based on Mandelstam Variables
s Channel
[math]s \equiv \left({\mathbf P_1^*}+ {\mathbf P_2^{*}}\right)^2=\left({\mathbf P_1^{'*}}+ {\mathbf P_2^{'*}}\right)^2[/math]
In the center of mass frame, the momentum of the particles interacting are equal and opposite, i.e. [math]p_1^*=-p_2^*[/math]. However, the 4-momentum still retains an energy component, which as a scalar quantity, can not be countered by another particle's direction of motion.
[math]{\mathbf P_1^*}\equiv \left(\begin{matrix} E_1\\ p_{x_1} \\ p_{y_1} \\ p_{z_1} \end{matrix} \right) \ \ \ \ {\mathbf P_2^*}\equiv \left(\begin{matrix} E_2\\ p_{x_2} \\ p_{y_2} \\ p_{z_2} \end{matrix} \right)[/math]
[math] \left({\mathbf P_1^*}+ {\mathbf P_2^{*}}\right)^2=\left( \left(\begin{matrix} E_1\\ p_{x_1} \\ p_{y_1} \\ p_{z_1} \end{matrix} \right)+\left(\begin{matrix} E_2\\ p_{x_2} \\ p_{y_2} \\ p_{z_2} \end{matrix} \right) \right)^2[/math]
[math] \left({\mathbf P_1^*}+ {\mathbf P_2^{*}}\right)^2=\left( \left(\begin{matrix} E_1\\ p_{x_1} \\ p_{y_1} \\ p_{z_1} \end{matrix} \right)+\left(\begin{matrix} E_2\\ -p_{x_1} \\ -p_{y_1} \\ -p_{z_1} \end{matrix} \right) \right)^2[/math]
[math] \left({\mathbf P_1^*}+ {\mathbf P_2^{*}}\right)^2= \left(\begin{matrix} E_1+E_2\\0 \\ 0 \\ 0 \end{matrix} \right)^2= \left(\begin{matrix}E_{CM}\\0 \\ 0 \\ 0 \end{matrix} \right)^2[/math]
Similarly, by the relativistic definition of energy
[math]E^2 \equiv p^2+m^2[/math]
where both particles have the same mass, this implies
[math] \left({\mathbf P_1^*}+ {\mathbf P_2^{*}}\right)^2=4E_{CM}^2=4(m_{CM}^2+p_{CM}^2)=s[/math]
[math]s=4(m_{CM}^2+p_{CM}^2)[/math]
t Channel
[math]s \equiv \left({\mathbf P_1^*}- {\mathbf P_1^{'*}}\right)^2=\left({\mathbf P_2^{*}}+ {\mathbf P_2^{'*}}\right)^2[/math]
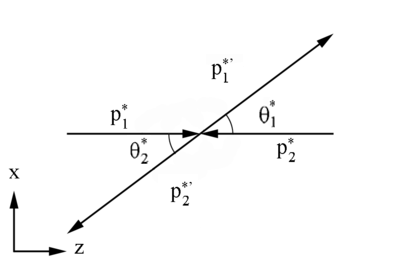
[math]{\mathbf P_1^*}\equiv \left(\begin{matrix} E_1^*\\ p_{x_1^*} \\ p_{y_1^*} \\ p_{z_1^*} \end{matrix} \right) \ \ \ \ {\mathbf P_1^{'*}}\equiv \left(\begin{matrix} E_1^{'*}\\ p_{x_2^{'*}} \\ p_{y_2^{'*}} \\ p_{z_2^{'*}} \end{matrix} \right)[/math]
The energy is conserved after the collision,
[math]E_1^*=E_1^{'*}[/math]
The momentum is also conserved in the center of mass frame in magnitude, but not direction,
[math]|p_1^*|=|p_1^{'*}|[/math]
u Channel
