CrossTalk
Go back MCNP simulations
Geometry
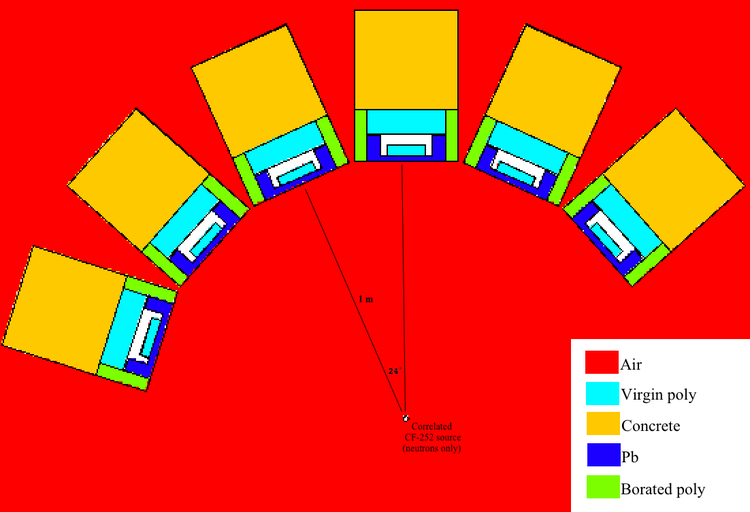
An array of 6 detectors are placed radially at a distance of 1 meter from an uncorrelated 252-CF source. The image below shows a top down view of the simulation geometry. The detector setups have a vertical extent of 30".
Detector physics
The detector physics used in the simulation is MCNP-POLIMI's default treatment for plastic organic scintillators. POLIMI uses electron equivalent light output (MeVee) for simulating detector response. Assuming the detectors have a energy deposition threshold of 0.2 MeV, the corresponding threshold, in electron equivalent light output, becomes 0.03 MeVee.
Summary
Neutron energy deposition from collisions with hydrogen and carbon are converted to electron equivalent light output (MeVee) by MCNP-POLIMI. Neutron collisions that all occur within a pulse collection time of 10 ns of each other are converted to MeVee and cumulated. If the cumulative light output exceeds 0.03 MeVee then it is considered a detection. The value 0.03 MeVee was chosen because it corresponds to a mean neutron energy deposit of 0.2 MeV.
Detector response
The electron equivalent (MeVee) conversion functions for neutrons were measured for plastic (BC 420) scintillators as a function of neutron energy deposit, . For deposit on hydrogen the measurements fit the following quadratic function:
and for deposit on carbon:
Statistics
n-n correlation was not simulated. Source particles were emitted isotropically, one at a time. An estimation of the uncorrelated n-n coincidence rate can provide a quantity that may be meaningfully compared with the simulated crosstalk rate. This rate can be described as the rate of measured n-n events if the fission neutrons were isotropically and uncorrelated from one another. The uncorrelated n-n rate, , is estimated from the simulation as follows:
- is the fission rate,
- mean neutron multiplicity,
- in the number of detectors, and
- is the probability that a single neutrons registers a hit in a single given detector. This value can be calculated directly from the simulation:
The crosstalk rate, , will simply scale proportionally with neutron multiplicity, fission rate, and the number of detectors. The estimated crosstalk rate is:
where is the probability of crosstalk per source neutron, which is calculated directly from the simulation result:
In order to quantify the amount of crosstalk contamination, I define a 'signal-to-noise ratio' equal to the ratio between the estimated signal and crosstalk rates.
I'm uncertain about the exact value for neutron multiplicity, so I assume it's equal to 2, since this is reasonable and serves as a worst case scenario. Go back MCNP simulations