Moller events No Solenoid
LUND file with Moller events (with origin of coordinates occurring at each event)
2 1 1 1 1 0 0.000563654 3.53715 0 6.2002
1 -1 1 11 0 0 0.69 -2.4999 10993.7998 10993.80 0.000511 0 0 0
2 -1 1 11 0 0 -0.69 2.4999 6.5852 7.08 0.000511 0 0 0
2 1 1 1 1 0.650745 0.00159988 3.52285 21.4844 17.5986
1 -1 1 11 0 0 -4.20 0.3862 10982.4004 10982.40 0.000511 -0.000456454 0.00077441 0
2 -1 1 11 0 0 3.45 0.8800 12.4191 12.92 0.000511 -0.000456454 0.00077441 0
2 1 1 1 1 0.650745 0.00159988 3.52285 21.4844 17.5986
1 -1 1 11 0 0 -4.20 0.3862 10982.4004 10982.40 0.000511 -0.000456454 0.00077441 0
2 -1 1 11 0 0 0.75 -1.2666 2.1155 2.58 0.000511 0 0 0

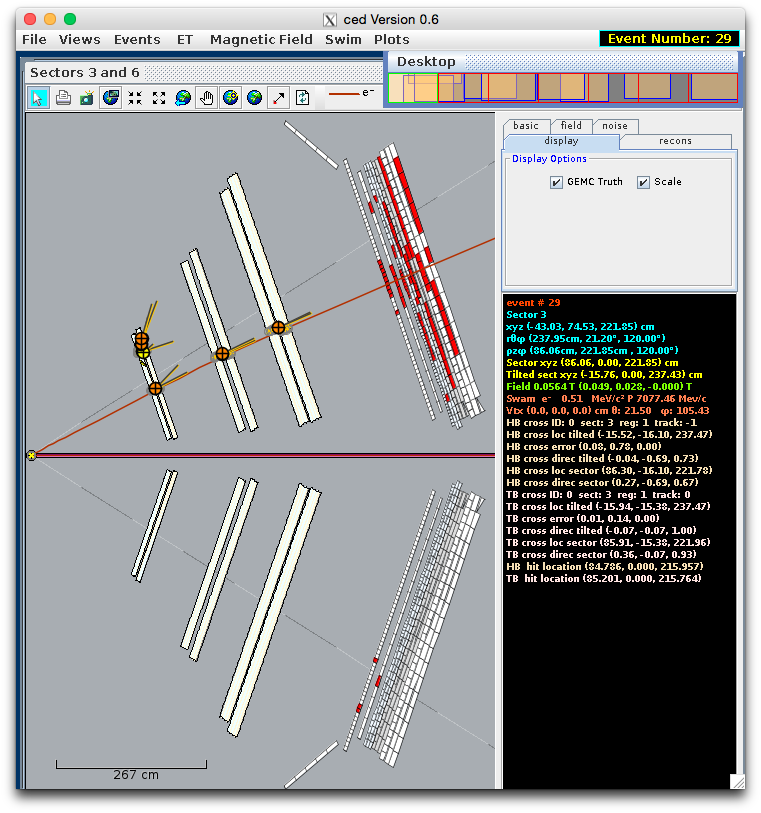
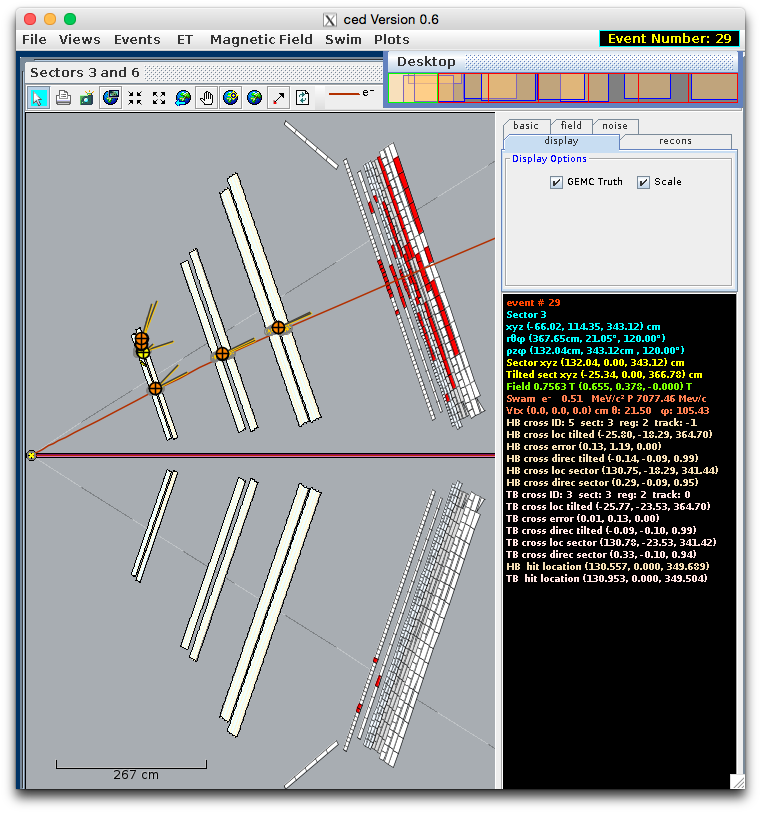
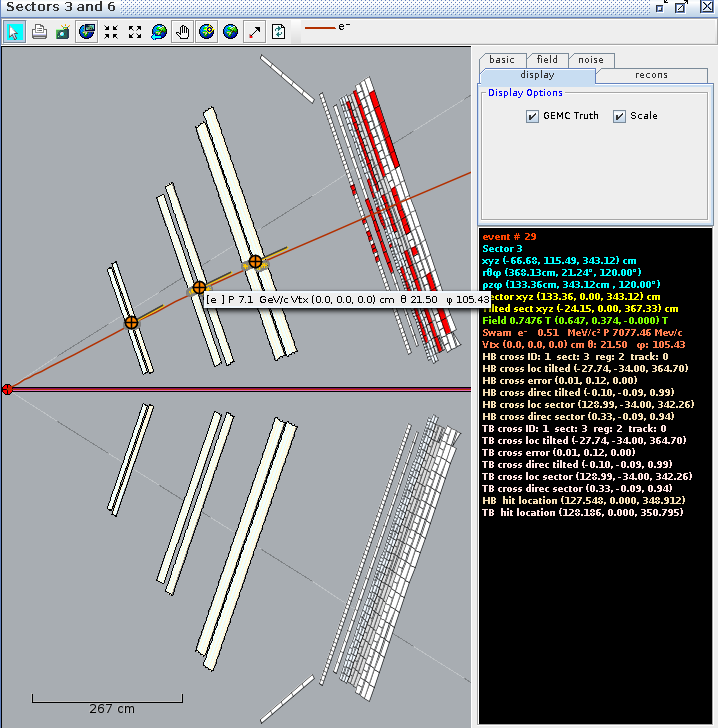
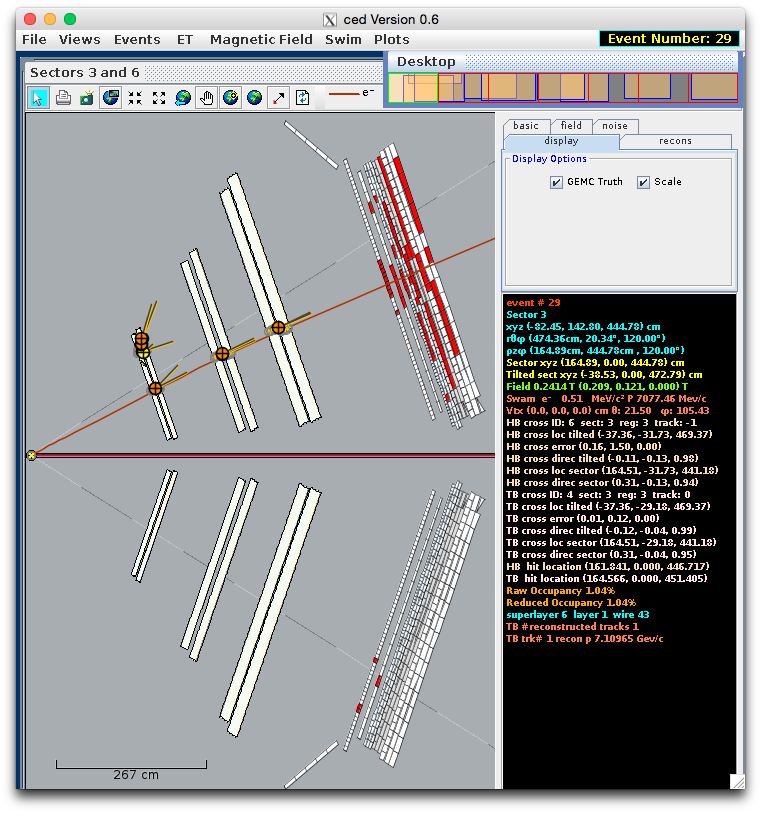
Picture of ced output for event 29
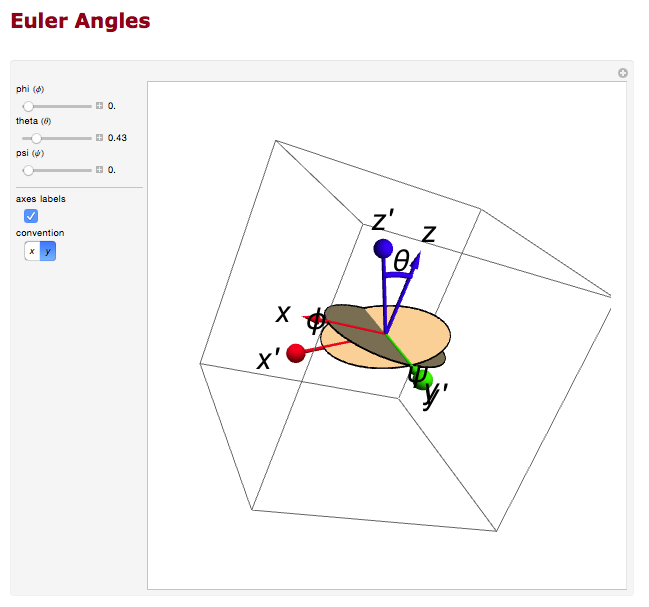
Transformation Matrix
[math]\left(
\begin{array}{ccc}
\cos (\theta ) & 0 & -\sin (\theta ) \\
0 & 1 & 0 \\
\sin (\theta ) & 0 & \cos (\theta ) \\
\end{array}
\right).\left(
\begin{array}{c}
x \\
y \\
z \\
\end{array}
\right)[/math]
[math]=\left(
\begin{array}{c}
x \cos (\theta )-z \sin (\theta ) \\
y \\
z \cos (\theta )+x \sin (\theta ) \\
\end{array}
\right)[/math]
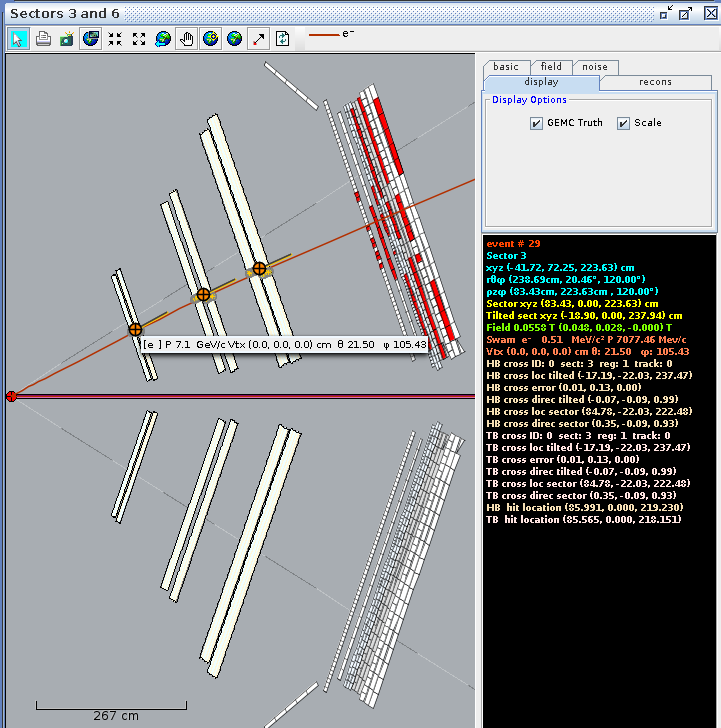
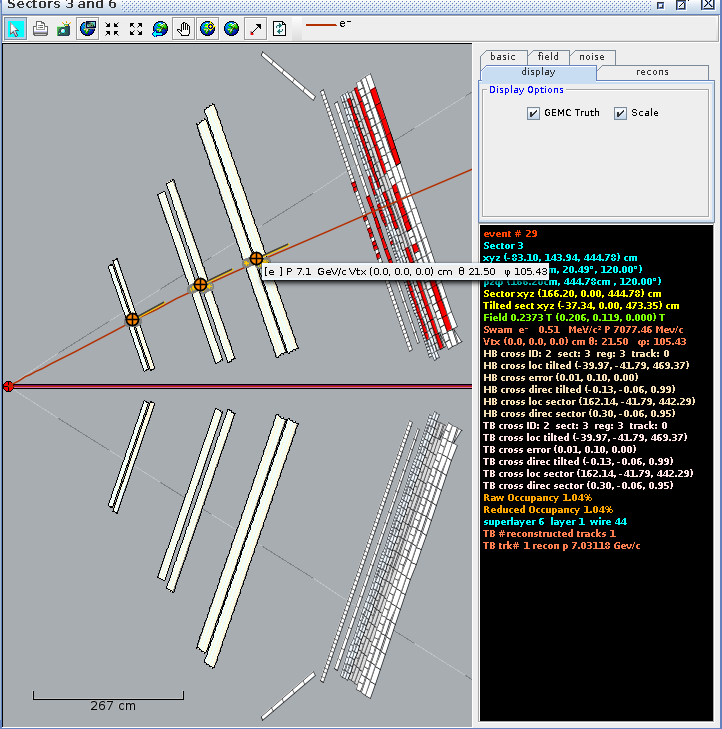
For event #29, in sector 3, the location of the first interaction is given by
Converting -25 degrees to radians,
[math]\theta =-0.436332[/math]
[math]\left(
\begin{array}{ccc}
\cos (\theta ) & 0 & -\sin (\theta ) \\
0 & 1 & 0 \\
\sin (\theta ) & 0 & \cos (\theta ) \\
\end{array}
\right).\left(
\begin{array}{c}
-15.76 \\
0 \\
237.43 \\
\end{array}
\right)[/math]
[math]=\left(
\begin{array}{c}
86.0588 \\
0. \\
221.845 \\
\end{array}
\right)[/math]
Finding [math]\phi =\frac{120\ 2 \pi }{360};[/math] since sector -1 =3-1=2*60=120 degrees
[math]\left(
\begin{array}{ccc}
\cos (\phi ) & -\sin (\phi ) & 0 \\
\sin (\phi ) & \cos (\phi ) & 0 \\
0 & 0 & 1 \\
\end{array}
\right).\left(
\begin{array}{c}
86.0588 \\
0. \\
221.845 \\
\end{array}
\right)[/math]
[math]\left(
\begin{array}{c}
-43.0294 \\
74.5291 \\
221.845 \\
\end{array}
\right)[/math]
DV_RunGroupC_Moller#Moller_Track_Reconstruction








