Difference between revisions of "Minimum accelerator energy to run experiment"
Jump to navigation
Jump to search
| Line 17: | Line 17: | ||
<math>A_1D_1(E,\ \Theta_C/m) = 8.73\ cm</math> | <math>A_1D_1(E,\ \Theta_C/m) = 8.73\ cm</math> | ||
| − | |||
| − | |||
| − | |||
1) Assuming the collimator diameter is <math>\Theta_C</math>: | 1) Assuming the collimator diameter is <math>\Theta_C</math>: | ||
| Line 36: | Line 33: | ||
\frac{1}{2}\ (286+183)\ \tan\left(\frac{1}{4}\ \frac{0.511}{E_{min}}\right) = 8.73 \Rightarrow E_{min} = 22.8\ MeV </math> | \frac{1}{2}\ (286+183)\ \tan\left(\frac{1}{4}\ \frac{0.511}{E_{min}}\right) = 8.73 \Rightarrow E_{min} = 22.8\ MeV </math> | ||
| − | 4) for arbitrary collimator size <math>\Theta_C/ | + | 4) for arbitrary collimator size <math>\Theta_C/m</math>: |
[[File:plot_energy_collimatorsize.jpeg]] | [[File:plot_energy_collimatorsize.jpeg]] | ||
Revision as of 22:09, 14 June 2010
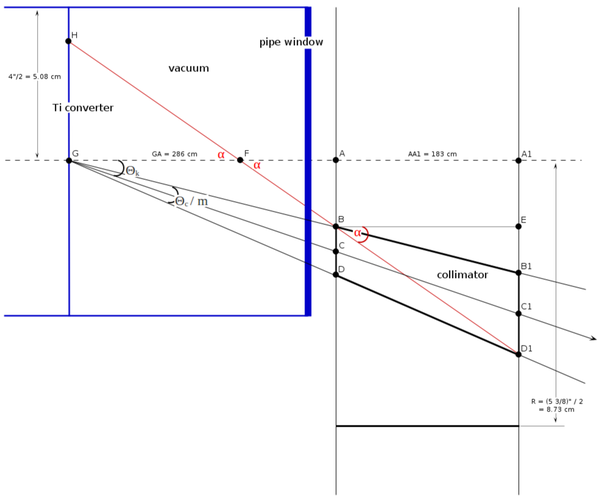
general setup
fitting the collimator size into the hole in the concrete wall
I can express the distance as function of collimator size and electron beam energy E:
To fit the collimator size into the hole in the concrete wall with radius R = 8.73 cm we need to solve equation:
1) Assuming the collimator diameter is :
2) Assuming the collimator diameter is :
3) Assuming the collimator diameter is :
4) for arbitrary collimator size :
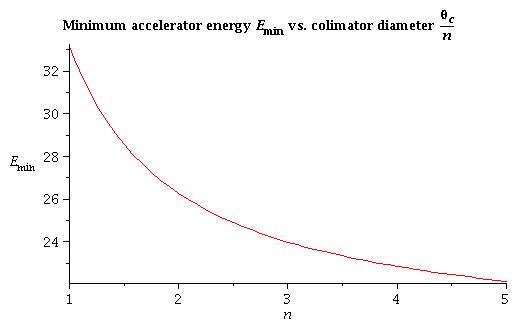
All energy under this line is good to run experiment for condition above
GH = 5.08 cm condition
1) assuming the collimator diameter is
2) assuming the collimator diameter is
3) assuming the collimator diameter is
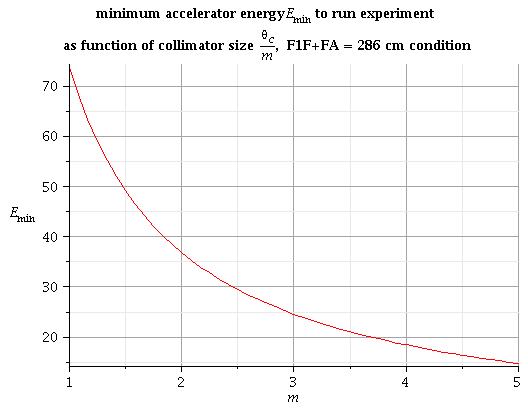
4) for arbitrary collimator size :
All energy under this line is good to run experiment for condition above
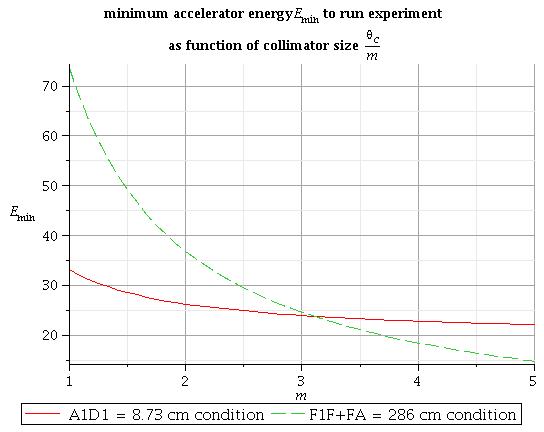
both conditions above are together
All energy under this linse is good to run experiment for both conditions above