Difference between revisions of "Geometry (44 MeV LINAC exit port)"
Jump to navigation
Jump to search
| Line 15: | Line 15: | ||
= tan^{-1}\left(\frac{1}{\sqrt{2}}\ tan(\Theta_C)\right)</math><br> | = tan^{-1}\left(\frac{1}{\sqrt{2}}\ tan(\Theta_C)\right)</math><br> | ||
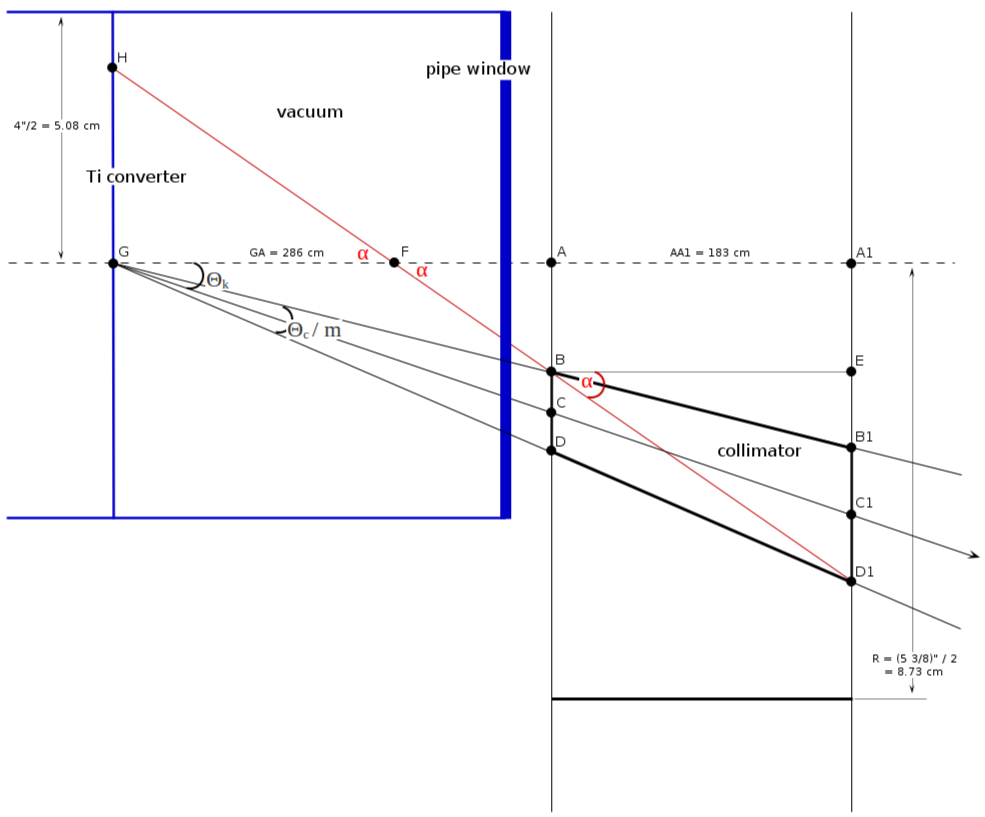
| − | = | + | =general collimator setup= |
| − | + | [[File:minimum_energy_condition.png]] | |
| − | |||
| − | + | <math>AC = 286\cdot\tan(\Theta_K)\ cm</math><br> | |
| − | + | <math>A_1C_1 = (286 +183)\cdot\tan(\Theta_K)\ cm</math> | |
| − | == | + | <math>BD = 286\cdot\tan(\frac{\Theta_C}{m})\ cm</math><br> |
| + | <math>B_1D_1 = (286 + 183)\cdot\tan(\frac{\Theta_C}{m})\ cm</math><br> | ||
| − | + | <math>\alpha = \frac{A_1D_1 - AB}{183} = \frac{(A_1C_1 + C_1D_1/2) - (AC - BD/2)}{183}</math> | |
=Vacuum pipe location (<math> \Theta_c/2</math>)= | =Vacuum pipe location (<math> \Theta_c/2</math>)= | ||
Revision as of 22:40, 20 June 2010
90 exit port measurements
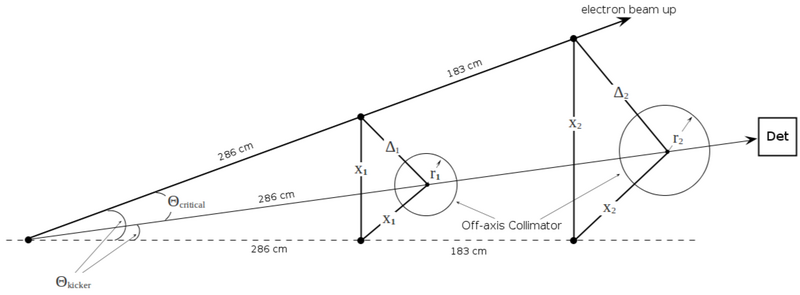
Critical and Kicker angles
general collimator setup
Vacuum pipe location ()
collimator location
1) center position:
(wall 1)
(wall 2)
2) collimator diameter:
(wall 1)
(wall 2)
collimator critical angle
from triangle :
minimal distance from the wall
from triangle FAB:
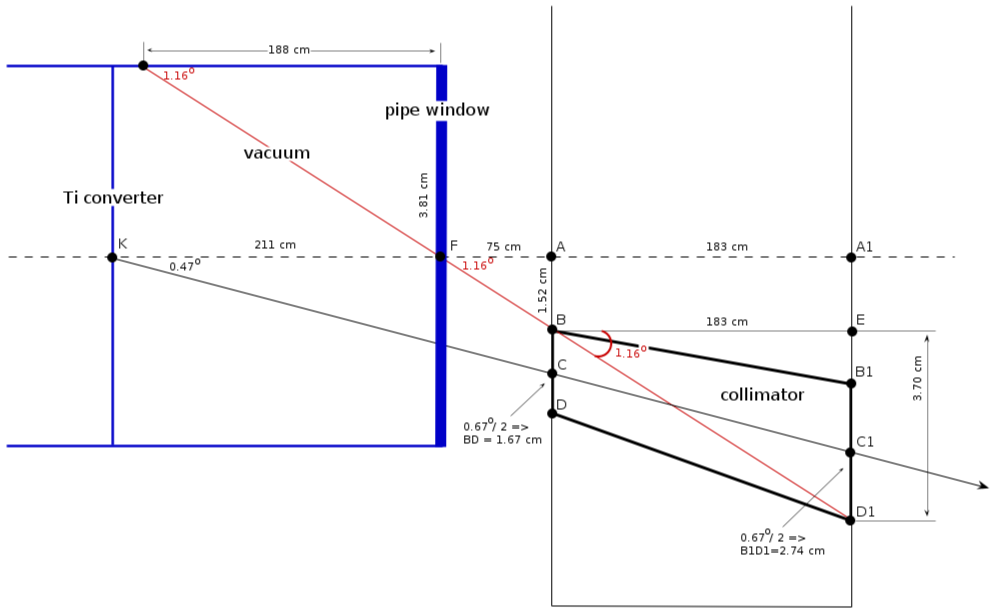
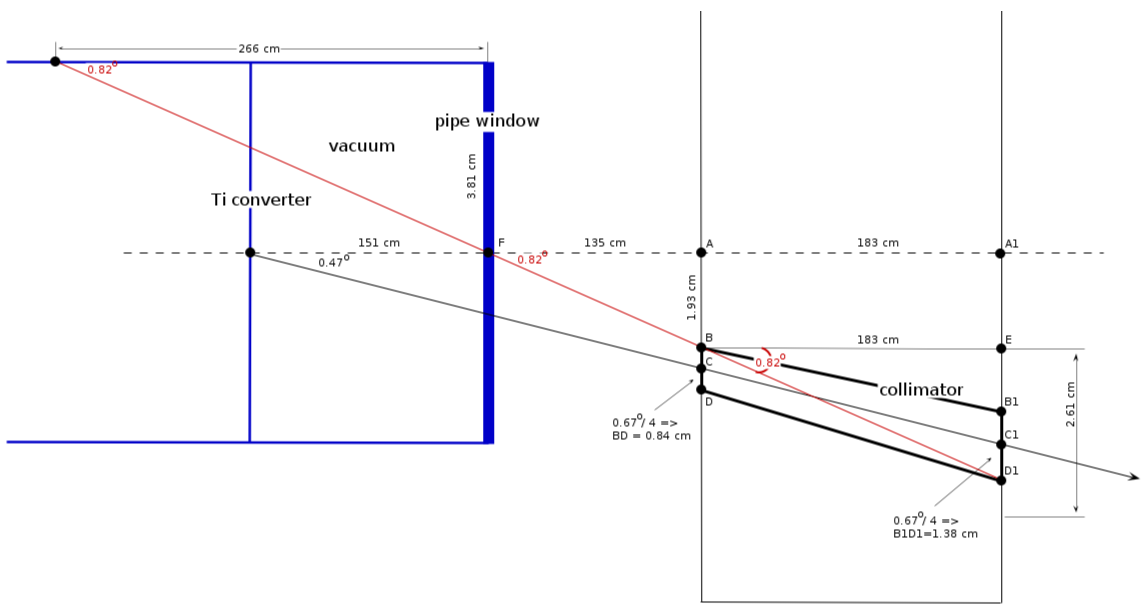
Vacuum pipe location ()
collimator location
1) center position:
(wall 1)
(wall 2)
2) collimator diameter:
(wall 1)
(wall 2)
collimator critical angle
from triangle :
minimal distance from the wall
from triangle FAB:
Funny pictures...
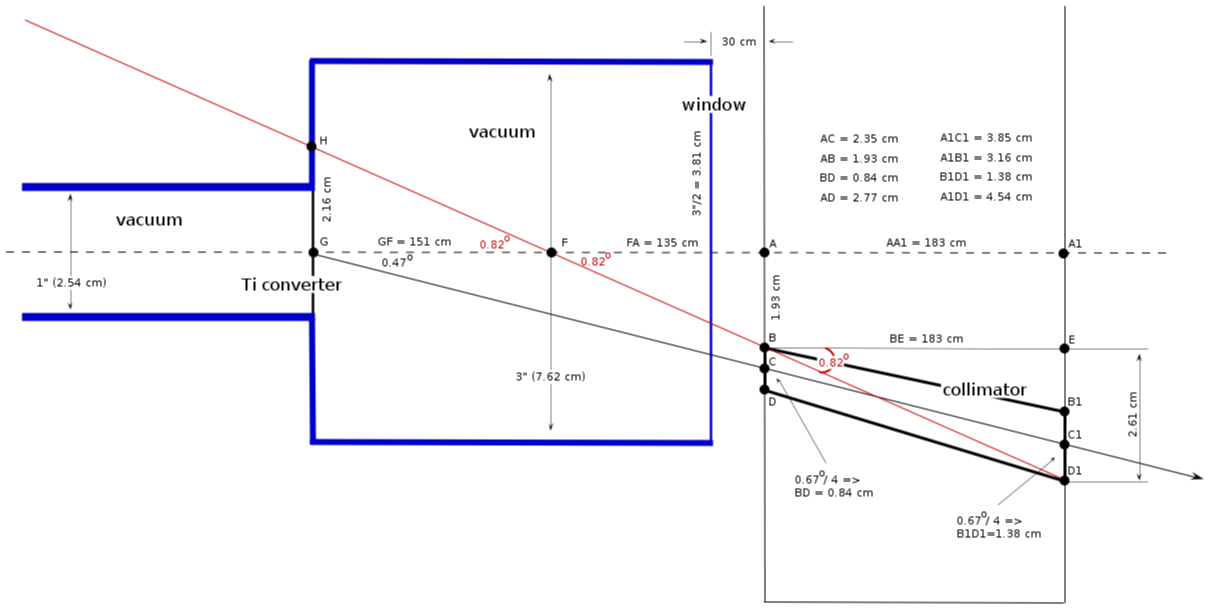
how it looks (, pipe 3")
how it looks 1 (, pipe 3")
how it looks 2 (, pipe 3")
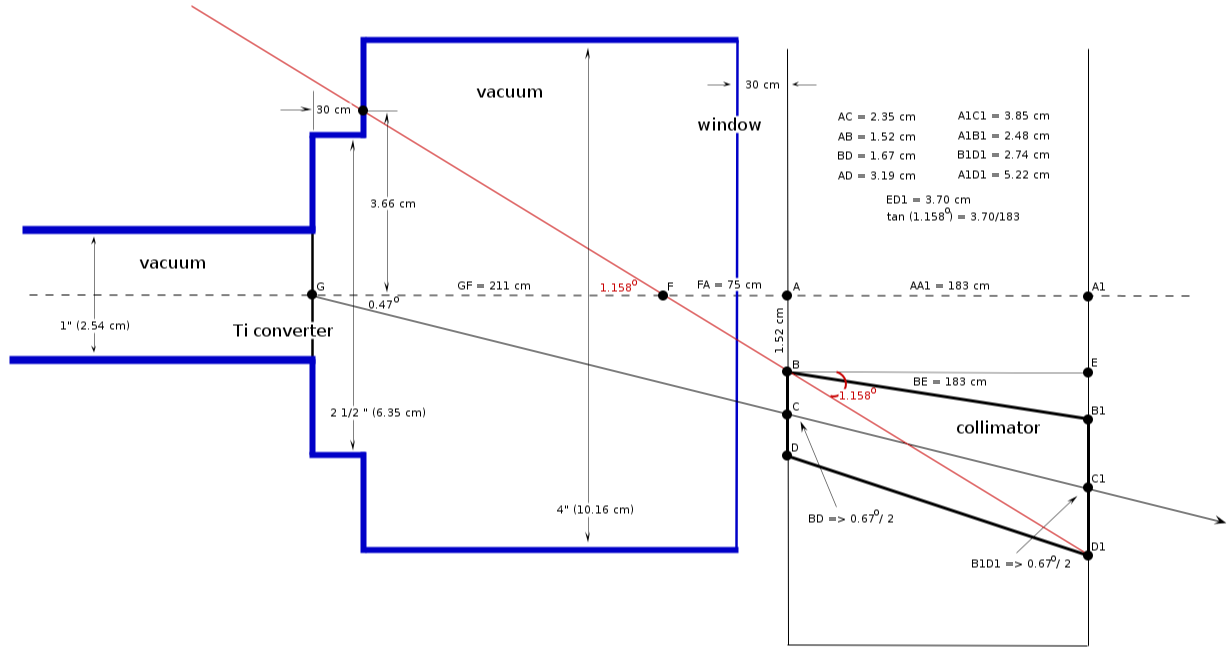
how it looks 4 (, pipe (2 1/2)" and then pipe 4")
need to adjust to converter position
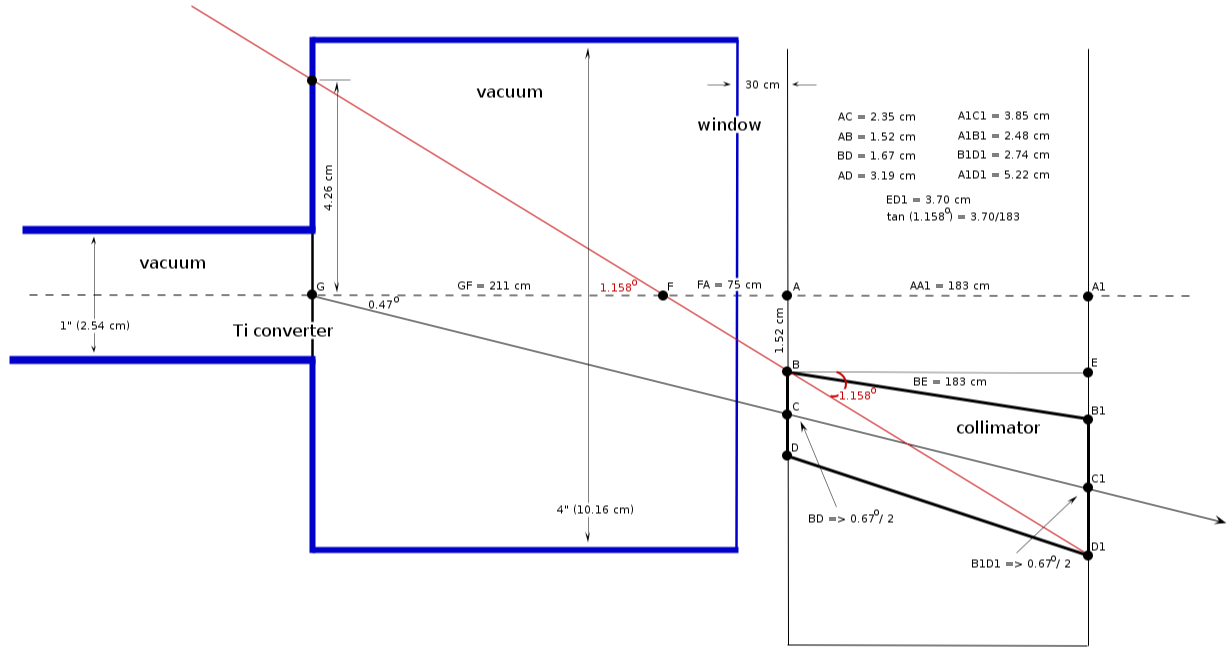
how it looks 5 (, box 3"x4" and then pipe 4")
need to adjust to converter position