Difference between revisions of "Effects Due to Target Length"
Jump to navigation
Jump to search
| Line 1: | Line 1: | ||
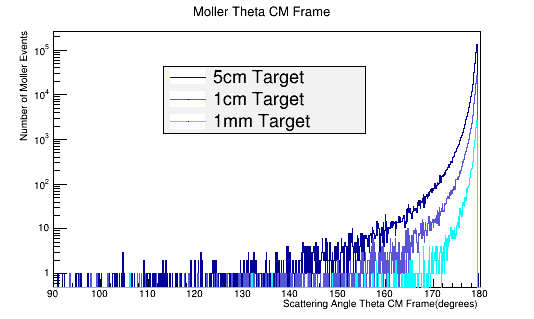
As the target material length is increased, the amount of multiple scattering increases. Since the majority of particles created in the CM frame are at large angles, this implies | As the target material length is increased, the amount of multiple scattering increases. Since the majority of particles created in the CM frame are at large angles, this implies | ||
| − | [[File:CompareTargetLength.png]] | + | [[File:CompareTargetLength.png]][[File:MolThetaLabTargetLength.png]] |
This gives, for LH2 in a 5cm long target: | This gives, for LH2 in a 5cm long target: | ||
Revision as of 04:40, 28 August 2018
As the target material length is increased, the amount of multiple scattering increases. Since the majority of particles created in the CM frame are at large angles, this implies
This gives, for LH2 in a 5cm long target:
Using the number of incident electrons, for 1 Moller electron:
This gives, for LH2 in a 1cm long target:
Using the number of incident electrons, for 1 Moller electron:
This gives, for LH2 in a 1mm long target:
Using the number of incident electrons, for 1 Moller electron: