U-Channel

[math]\textbf{\underline{Navigation}}[/math]

[math]\vartriangleleft [/math] [math]\triangle [/math] [math]\vartriangleright [/math]

u-Channel

The u quantity is does not have a clear cut interpretation like the s and t channels

[math]u \equiv \left({\mathbf P_1^*}- {\mathbf P_2^{'*}}\right)^2=\left({\mathbf P_2^*}- {\mathbf P_1^{'*}}\right)^2[/math]

[math]u \equiv\left({\mathbf P_1^*}- {\mathbf P_2^{'*}}\right)^2[/math]

[math]u \equiv \mathbf P_1^{*2}-2 \mathbf P_1^* \mathbf P_2^{'*}+ \mathbf P_2^{'*2}[/math]

[math]u \equiv 2m_1^2-2E_1^*E_2^{'*}+2 \vec p \ _1^* \vec p \ _2^{'*}[/math]

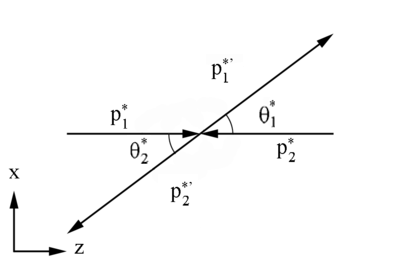
In the center of mass frame of reference,

[math] E^* \equiv E_1^*=E_1^{'*} = E_2^*=E_2^{'*} = E_1^*=E_2^*[/math]

and

[math]|p^*| \equiv | \vec p \ _1^*|=| \vec p \ _1^{'*}| =| \vec p \ _2^*|=| \vec p \ _2^{'*}|[/math]

and [math]\theta_1=-\theta_2[/math] is the angle between [math]\vec p \ _1^* [/math] and [math] \vec p \ _2^{'*}[/math]

[math]t \equiv 2m_1^*-2E_1^{*2}-2 |p |^{*2}cos\ \theta[/math]

Using the relativistic term for Energy

[math]E^2=\vec p \ ^2+m^2[/math]

[math]t \equiv -2 p \ _1^{*2}(1+cos\ \theta)[/math]

---

[math]\textbf{\underline{Navigation}}[/math]

[math]\vartriangleleft [/math] [math]\triangle [/math] [math]\vartriangleright [/math]