Difference between revisions of "Variables Used in Elastic Scattering"
Jump to navigation
Jump to search
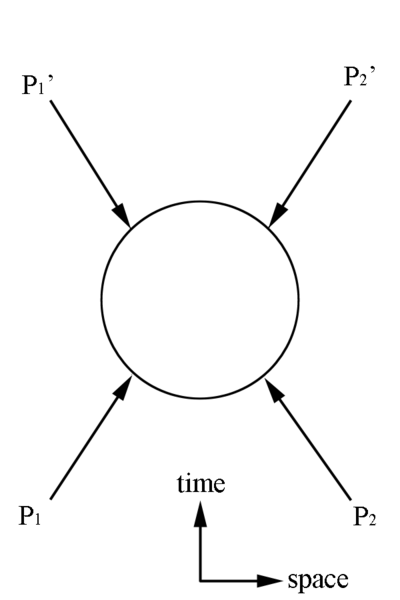
where represents the 4-Momentum Vector in the CM frame
and represents the 4-Momentum Vector in the initial Lab frame
where represents the 4-Momentum Vector in the final Lab frame
| Line 104: | Line 104: | ||
<center><math>\left({\mathbf P_1}- {\mathbf P_1^'}\right)^2=\left( m_1^2-2\left( \sqrt{p_1^2+m_1^2} \sqrt{p_1^{'2}+m_1^{'2}}-\vec p_1\cdot \vec p_1^'\right)+ m_1^{'2}\right)=\left({\mathbf P_a}\right)^2=s</math></center> | <center><math>\left({\mathbf P_1}- {\mathbf P_1^'}\right)^2=\left( m_1^2-2\left( \sqrt{p_1^2+m_1^2} \sqrt{p_1^{'2}+m_1^{'2}}-\vec p_1\cdot \vec p_1^'\right)+ m_1^{'2}\right)=\left({\mathbf P_a}\right)^2=s</math></center> | ||
| + | |||
| + | |||
| + | <center><math>\left({\mathbf P_1}- {\mathbf P_2^'}\right)^2=\left( m_1^2-2\left( \sqrt{p_1^2+m_1^2} \sqrt{p_2^{'2}+m_2^{'2}}-\vec p_1\cdot \vec p_2^'\right)+ m_2^{'2}\right)=\left({\mathbf P_b}\right)^2=s</math></center> | ||
| + | |||
| + | |||
| + | <center><math>\left({\mathbf P_2}- {\mathbf P_1^'}\right)^2=\left( m_2^2-2\left( \sqrt{p_2^2+m_2^2} \sqrt{p_1^{'2}+m_1^{'2}}-\vec p_2\cdot \vec p_1^'\right)+ m_1^{'2}\right)=\left({\mathbf P_c}\right)^2=s</math></center> | ||
| + | |||
| + | |||
| + | <center><math>\left({\mathbf P_2}- {\mathbf P_2^'}\right)^2=\left( m_2^2-2\left( \sqrt{p_2^2+m_2^2} \sqrt{p_2^{'2}+m_2^{'2}}-\vec p_2\cdot \vec p_2^'\right)+ m_2^{'2}\right)=\left({\mathbf P_d}\right)^2=s</math></center> | ||
| + | |||
| + | |||
| + | |||
| + | |||
| + | <center><math>\left({\mathbf P_1}- {\mathbf P_1^'}\right)^2=\left( m_1^2-2\left( \sqrt{p_1^2p_1^{'2}+m_1^2p_1^{'2}+m_1^{'2}p_1^2+m_1^{'2}m_1^2}-\vec p_1\cdot \vec p_1^'\right)+ m_1^{'2}\right)=\left({\mathbf P_a}\right)^2=s</math></center> | ||
Revision as of 22:05, 31 January 2016
Lorentz Invariant Quantities
Total 4-Momentums
As was shown earlier the scalar product of a 4-Momentum vector with itself ,
,
and the length of a 4-Momentum vector composed of 4-Momentum vectors,
,
are invariant quantities.
It was further shown that
which can be expanded to
New 4-Momentum Quantities
Working in just the Lab frame, we can form new 4-Momentum Vectors comprised of 4-Momenta in this frame, with
Using the algebraic fact
and the fact that the length of these 4-Momentum Vectors are invariant,
Using the fact that the scalar product of a 4-momenta with itself is invariant,
We can simiplify the expressions
Finding the cross terms,
This gives
Using the relativistic expression for total energy