Difference between revisions of "Niowave Report 11-30-2015"
| Line 16: | Line 16: | ||
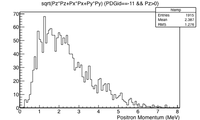
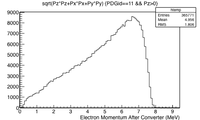
| − | The momentum distribution of positrons escaping the downstream side of the LBE target is shown below along the momentum distribution of electrons that traverse the LBE target. Postrons leaving the downstream side of the target are predicted to have a mean momentum of 2.4 MeV whereas the electrons that penetrate the | + | The momentum distribution of positrons escaping the downstream side of the LBE target is shown below along the momentum distribution of electrons that traverse the LBE target. Postrons leaving the downstream side of the target are predicted to have a mean momentum of 2.4 MeV whereas the electrons that penetrate the LBE target will have lost approximately 3 MeV. Most of the positrons have a momentum beetween one and three MeV. A system to transport these electrons would need to cover this momentum range to avoid appreciable loss. A solenoidal magnetic field was chosen that surround the LBD target. |
Revision as of 13:35, 30 November 2015
Overview
This report investigates the impact of target thickness and the use of an external solenoid for the production of positrons using a 10 MeV electron beam. A electron beam energy of 10 MeV is chosen as a compromise between maximizing the production of bremstrahlung photons and minimizing the production of neutrons that occur when an MeV energy electron beam interects with a dense metal. A converter target of liquid Lead-Bismuth Eutectic (LBE) is chosen with a concentration of 44.5% lead and 55.5% bismuth in order to provide a high atomic number (Z) material to increase bremstrahlung production that will also absorb the heat deposited when the material is traversed by an MeV electron beam. This work used the simulation package GEANT4 to predict the impact of target thickness and the use of a solenoid magnetic field on positron production efficiency as well as the amount of energy that will be deposited in the apparatus when using a 3.48 cm diameter beam pipe.
Target optimization
Optimal Thickness
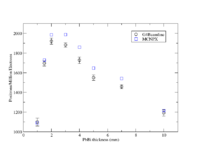
One method to produce positrons relies on the creation of positron-electron pairs from the bremsstrahlung photons that are produced when electrons traverse material with a high atomic number (Z). While thicker material encourages the production of these photons, the increased thickness will also cause the produced positrons to annihilate within the material before they can escape. As a result, there is an optimal thickness which will balance the two processes to maximize positron production. A simulation was performed to determine the optimal thickness using a 10 MeV electron beam with a 1cm radius uniform cylindrical spatial distribution impinging a windowless PbBi target. The target thickness was changed to determine the optimal thickness for emmitt positrons from the downstream surface of the LBE target. The simulation was performed by two separate investigators using separate simulation packages. The results, shown below, indicate that a LBE target thicknes of about 2mm will produce about two positrons for every thousand electrons impinging the LBE target.
|
The momentum distribution of positrons escaping the downstream side of the LBE target is shown below along the momentum distribution of electrons that traverse the LBE target. Postrons leaving the downstream side of the target are predicted to have a mean momentum of 2.4 MeV whereas the electrons that penetrate the LBE target will have lost approximately 3 MeV. Most of the positrons have a momentum beetween one and three MeV. A system to transport these electrons would need to cover this momentum range to avoid appreciable loss. A solenoidal magnetic field was chosen that surround the LBD target.
|
Optimal Solenoidal Field
Beam pipe heating from scattered electrons that deposit energy in the beam pipe was estimated using GEANT4. A set of stainless steel windows were added to the 2mm thick LBE target for this simulation.
Beam Pipe heating
The energy deposited by electrons scattered into a 3.48 diameter stainless steel beam pipe (1.65 mm thick) from a PbBi target as a function of a uniform Solenoidal magnetic field.
The histogram is binned in 100 (10 cm) bin widths. The surface area becomes
To convert From Mev/ e- to kW/cm^2 assuming a current of 1mA (10^-3 C/s) you
 |

|

|
Solenoid Description
A 10 MeV electron beam with a radius of 0.5 cm was incident on a 2 mm thick PbBi target. The target is positioned at Z = -901 mm.
| Element | dimension |
| Inner beam pipe radius | 1.74 cm |
| Inner beam pipe thickness | 0.165 cm |
| water jacket thickness | 0.457 cm |
| outer beam pipe radius | 2.362 cm |
| outer beam pipe thickness | 0.165 cm |
| Solenoid inner radius | 2.527 cm |
| Solenoid outer radius | 4.406 cm |