Difference between revisions of "Tamar AnalysisChapt"
| Line 95: | Line 95: | ||
|- | |- | ||
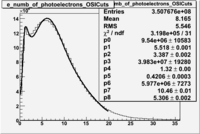
| [[Image:electrons_nphe_without_cuts_all_data_with_fits.gif|200px|thumb|The number of photoelectrons without cuts]] | | [[Image:electrons_nphe_without_cuts_all_data_with_fits.gif|200px|thumb|The number of photoelectrons without cuts]] | ||
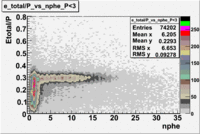
| − | ||[[Image:e_total_vs_nphe_momentum_cut_file_dst27070.gif|200px]] | + | ||[[Image:e_total_vs_nphe_momentum_cut_file_dst27070.gif|200px|thumb|The total energy deposited into the Calorimeter vs The Number of Photoelectrons]] |
| − | |||
| − | |||
|} | |} | ||
| Line 106: | Line 104: | ||
where <math>\beta c</math> is the particle's velocity and n - index of refraction of the medium. The charged particle in time t travels <math>\beta c t</math> distance, while the electromagnetic waves - <math>\frac{c}{n}t</math>. For a medium with given index of refraction n there is a threshold velocity <math>\beta_{thr}=\frac{1}{n}</math>, below <math>\beta_{thr}</math> no radiation can take place. This process may be used to observe the passage of charged particles in a detector which can measure the produced photons.<br> | where <math>\beta c</math> is the particle's velocity and n - index of refraction of the medium. The charged particle in time t travels <math>\beta c t</math> distance, while the electromagnetic waves - <math>\frac{c}{n}t</math>. For a medium with given index of refraction n there is a threshold velocity <math>\beta_{thr}=\frac{1}{n}</math>, below <math>\beta_{thr}</math> no radiation can take place. This process may be used to observe the passage of charged particles in a detector which can measure the produced photons.<br> | ||
| − | The number of photons produced per unit path length of a particle with charge <math>Ze</math> and per unit energy interval of the photons is proportional to the sine of the Cherenkov angle | + | The number of photons produced per unit path length of a particle with charge <math>Ze</math> and per unit energy interval of the photons is proportional to the sine of the Cherenkov angle<ref name="Nakamure"> Nakamure, K., ''et al.''. (2010). The Review of Particle Physics. Particle Data Group. J. Phys. G '''37''', 075021.</ref> <br> |
<math>\frac{d^2 N}{dEdx}=\frac{\alpha z^2}{\hbar c}sin ^2 \theta_c=\frac{\alpha z^2}{\hbar c}[1-\frac{1}{\beta^2 n^2 (E)}]</math><br> | <math>\frac{d^2 N}{dEdx}=\frac{\alpha z^2}{\hbar c}sin ^2 \theta_c=\frac{\alpha z^2}{\hbar c}[1-\frac{1}{\beta^2 n^2 (E)}]</math><br> | ||
| Line 120: | Line 118: | ||
The gas used in the CLAS Cerenkov counter is perfluorobutane <math>C_4 F_{10}</math> with index of refraction equal to 1.00153. The number of photoelectrons emitted by electrons is about 13. On the other hand, calculations show that the number of photons produced by the negatively charged pions in the Cherenkov detector is approximately 2. | The gas used in the CLAS Cerenkov counter is perfluorobutane <math>C_4 F_{10}</math> with index of refraction equal to 1.00153. The number of photoelectrons emitted by electrons is about 13. On the other hand, calculations show that the number of photons produced by the negatively charged pions in the Cherenkov detector is approximately 2. | ||
| − | [[Image:e_momentum_vs_numb_of_photoelectrons_27095_theory.gif|200px]][[Image:pi_momentum_vs_numb_of_photons_27095_theory.gif|200px]] | + | [[Image:e_momentum_vs_numb_of_photoelectrons_27095_theory.gif|200px|thumb|The number of photoelectrons for Electrons, Theoretical Calculation]] ]][[Image:pi_momentum_vs_numb_of_photons_27095_theory.gif|200px|thumb|The number of photoelectrons for Pions, Theoretical Calculation]] |
Geometrical cuts on the location of the particle at the entrance to the cerenkov detector and time matching cuts were applied to reduce the pion contamination. For each CLAS Cherenkov detector segment the following cut has been applied | Geometrical cuts on the location of the particle at the entrance to the cerenkov detector and time matching cuts were applied to reduce the pion contamination. For each CLAS Cherenkov detector segment the following cut has been applied | ||
| Line 128: | Line 126: | ||
where <math>\theta_p</math> represents the measured polar angle in projective plane for each electron event. Cherenkov counter projective plane is an imaginary plane behind the Cherenkov detector where cherenkov radiation would arrive in the case if it moved the same distance from emission point to PMT, without reflections in the mirror system, <math>{\theta_p}^{center}</math> - the polar angle from the CLAS center to the image of Cherenkov counter segment center and <math>{\theta_p}^{offset}</math> - the shift in the segment center position. In addition to geometrical cut timing cut has been applied in order to perform time matching between Cherenkov counter and time of flight system. | where <math>\theta_p</math> represents the measured polar angle in projective plane for each electron event. Cherenkov counter projective plane is an imaginary plane behind the Cherenkov detector where cherenkov radiation would arrive in the case if it moved the same distance from emission point to PMT, without reflections in the mirror system, <math>{\theta_p}^{center}</math> - the polar angle from the CLAS center to the image of Cherenkov counter segment center and <math>{\theta_p}^{offset}</math> - the shift in the segment center position. In addition to geometrical cut timing cut has been applied in order to perform time matching between Cherenkov counter and time of flight system. | ||
| − | The pion contamination in electron sample was estimated by fitting the number of photoelectron distribution with two Gaussian distributions convoluted with a Landau distribution, which is presented below: | + | The pion contamination in electron sample was estimated by fitting the number of photoelectron distribution with two Gaussian distributions convoluted with a Landau distribution, which is presented below<ref name="Lanczos"> Lanczos, C. (1964). A precision approximation of the Gamma function. SIAM Journal of Numerical Analysis, B1 '''86'''. </ref>: |
;<math>N_{pe}= p_0 e^{-0.5 \left (\frac{x-p_1}{p_2} \right )^2} + p4\frac{1}{1-\left(\frac{x-p5}{p6}\right )} + p_6 e^{-0.5 \left (\frac{x-p_7}{p_8} \right )^2}</math> | ;<math>N_{pe}= p_0 e^{-0.5 \left (\frac{x-p_1}{p_2} \right )^2} + p4\frac{1}{1-\left(\frac{x-p5}{p6}\right )} + p_6 e^{-0.5 \left (\frac{x-p_7}{p_8} \right )^2}</math> | ||
| Line 140: | Line 138: | ||
| [[Image:electrons_nphe_without_cuts_all_data_with_fits.gif|200px|thumb|The number of photoelectrons without cuts]] || [[Image:electrons_nphe_with_OSIcuts_all_data_with_fits.gif|200px|thumb|The number of photoelectrons with OSI cuts]] | | [[Image:electrons_nphe_without_cuts_all_data_with_fits.gif|200px|thumb|The number of photoelectrons without cuts]] || [[Image:electrons_nphe_with_OSIcuts_all_data_with_fits.gif|200px|thumb|The number of photoelectrons with OSI cuts]] | ||
|} | |} | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
==Notes== | ==Notes== | ||
Revision as of 21:46, 22 February 2011
Introduction
THIS CHAPTER IS NOT READY< PLEASE READ OTHER THREE CHAPTERS.
This chapter will describe the techniques to calculate semi inclusive double spin asymmetries for the following reactions and for NH3 and ND3 polarized targets respectively. The goal of this work is the extraction of the ratio of polarized to unpolarized quark distribution functions using double spin asymmetries.
There are couple of main steps in the data analysis, which will be discussed bellow: 1) the data selection, 2) background elimination, 3) the target and beam polarization, 4) the detector acceptance >???????
The CLAS Data Selection
The data files from the EG1b run were chosen for this analysis. During the run 2.2 GeV, 4.2 GeV and 5.7 GeV longitudinally polarized electron beam were used on the polarized frozen ammonia. This work will discuss the analysis of 4.2 GeV electron beam on hydrogen and deuteron targets.
Table ?? EG1b Runs used for Analysis.(Beam Energy, Target Type, Torus Current, Target and Beam Polarization)
The collected data during the experiment has been filtered by applying restrictions, which will be discussed in the Data Analysis chapter.
Beam Helicity and Charge Asymmetry
In double spin asymmetry measurements it is important to eliminate any source of false asymmetry, like charge asymmetry.
The helicity of the electron beam was flipped with a rate of 1 HZ and its original state was chosen at the injector in a pseudo-random way. The next state of the electron helicity, so called complement state, is always with opposite helicity. The diagram on Fig.?.? represents the helicity state.
Figure ??. The Helicity State
A one bit signal from the beam injector gives the helicity information, whereas a sync bit with a 2 HZ frequency is generated at the same time and is equal to the helicity flip time. However, the electron beam helicity sequence can be interrupted by the Data Acquisition System(DAQ), like leaving unpaired helicity states and dead time problems.
In order to eliminate the asymmetry caused by the DAQ, the Faraday cup was installed to measure the charge simultaneously with the helicity state flip. The stored data files include accumulated charge value for each helicity state.
Data Analysis
Radiative Corrections
Analysis chapter is not ready, Please start reading Experimental setup, theory and CEBAF. Those are the chapters that i finished, and would like them corrected. Ill rewrite the chapter below, it is not argent and include the diagrams.
In double spin asymmetry analysis the electron nucleon scattering process is given as an one photon exchange event, so called the Born approximation. In reality, there are multiple photon effects during the experiments. These high order processes, also called radiative effects, can be calculated and used to correct the cross section data.
There are two types of radiative corrections, internal and external. Internal radiative corrections describe the contributions which took place during the lepton-hadron interaction. In first order approximation they include vertex photon exchange, self energy and vacuum polarization.<ref name="RadiativeCorrections"> Nucleon Form Factors. In Scholarpedia, from http://www.scholarpedia.org/article/Nucleon_Form_factors#History </ref>
On the other hand, the external radiative corrections account for the Bremsstrahlung by the incoming and scattered electron and by the recoiling target nucleon.
One of the major advantages of the double polarization experiments is the minimum contribution from the radiative corrections. For the ~5.6 GeV incident electron beam data the radiative corrections are less than 5%<ref>http://www.jlab.org/Hall-B/secure/eg1/EG2000/fersch/QUALITY_CHECKS/file_quality/runinfo.txt</ref>. Due to negligible contributions from the radiative corrections they are not included in the double spin asymmetry analysis.
EC CUTS
During the EG1b experiment, the electromagnetic calorimeter was used to separate electrons from the pions. The energy deposition into the electromagnetic calorimeter is different for electrons and pions.
Electrons interact with the matter , producing electromagnetic showers and releasing the energy into the matter. The deposited energy into matter is linearly proportional to the momentum of the electrons. On the other hand , pions are minimum ionizing particles(MIP), losing energy with the rate of approximately .
Electromagnetic calorimeter(EC) consists of 39 of 10 thick scintillator and ~2 mm thick lead. After passing through the calorimeter detector pions lose about 0.08 GeV energy independent their momentum. The energy loss by the electrons is linearly proportional to the momentum of the electron. Pions produce the constant signal in the calorimeter around 0.08 GeV. In order to eliminate misidentified pions from the electron sample, following cut has been applied:
where p represents particle momentum and - inner part of the calorimeter.
Electromagnetic calorimeter contains 13 layers of lead-scintillator sandwich. Each set of 13 layers are subdivided into 5 inner and 8 outer layers. Since the energy loss of pions is related to the detector thickness the correlation can be established between the energy deposition into the inner and outer layers of the detector:
which gives the following cut for the energy deposition into the outer layer of the calorimeter:
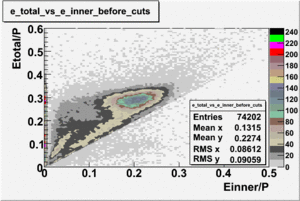
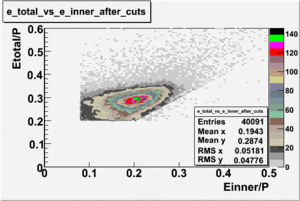
Figure ?.?. vs before and after EC cuts (, for EC inner - ).
Cherenkov Counter Cut
The Cherenkov counter has been used to separate electrons from the background negatively charged pions. These negative pions are produced when lepton goes at polar angle close to zero and is not measured by the detector. The number of photoelectron distribution measured in the cherenkov detector and the energy deposition dependence on number of photoelectrons are shown in Figure ?.?. One can see, that a single photoelectron peak is caused by the misidentified pions as electrons.
When the velocity of a charged particle is greater than the local phase velocity of light or when it enters a medium with different optical properties the charged particle will emit photons. The Cherenkov light is emitted under a constant angle - the angle of Cherenkov radiation relative to the particle's direction. It can be shown geometrically that the cosine of the Cherenkov radiation angle is anti-proportional to the velocity of the charged particle
where is the particle's velocity and n - index of refraction of the medium. The charged particle in time t travels distance, while the electromagnetic waves - . For a medium with given index of refraction n there is a threshold velocity , below no radiation can take place. This process may be used to observe the passage of charged particles in a detector which can measure the produced photons.
The number of photons produced per unit path length of a particle with charge and per unit energy interval of the photons is proportional to the sine of the Cherenkov angle<ref name="Nakamure"> Nakamure, K., et al.. (2010). The Review of Particle Physics. Particle Data Group. J. Phys. G 37, 075021.</ref>
after deriving the Taylor expansion of our function and considering only the first two terms, we get
The gas used in the CLAS Cerenkov counter is perfluorobutane with index of refraction equal to 1.00153. The number of photoelectrons emitted by electrons is about 13. On the other hand, calculations show that the number of photons produced by the negatively charged pions in the Cherenkov detector is approximately 2.
]]
Geometrical cuts on the location of the particle at the entrance to the cerenkov detector and time matching cuts were applied to reduce the pion contamination. For each CLAS Cherenkov detector segment the following cut has been applied
where represents the measured polar angle in projective plane for each electron event. Cherenkov counter projective plane is an imaginary plane behind the Cherenkov detector where cherenkov radiation would arrive in the case if it moved the same distance from emission point to PMT, without reflections in the mirror system, - the polar angle from the CLAS center to the image of Cherenkov counter segment center and - the shift in the segment center position. In addition to geometrical cut timing cut has been applied in order to perform time matching between Cherenkov counter and time of flight system.
The pion contamination in electron sample was estimated by fitting the number of photoelectron distribution with two Gaussian distributions convoluted with a Landau distribution, which is presented below<ref name="Lanczos"> Lanczos, C. (1964). A precision approximation of the Gamma function. SIAM Journal of Numerical Analysis, B1 86. </ref>:
After applying cuts the pion contamination reduces from? % to ? %.
| No cuts | OSI Cuts |
Notes
<references/>
[Go Back]