|
|
| Line 50: |
Line 50: |
| | | | |
| | ===Appendix=== | | ===Appendix=== |
| − |
| |
| − | {| border="1" cellspacing="0" style="text-align: center; width: 500px; height: 500px;"
| |
| − | ! scope="col" width="50" | bremsstrahlung for Ti
| |
| − | ! scope="col" width="50" | bremsstrahlung for Al
| |
| − | |-
| |
| − | |[[File:bremss44MeV.png | 000 px]] ||[File:Al 44MeV.png | 400 px]]
| |
| − | |}
| |
| − |
| |
| − | ====bremsstrahlung for Ti====
| |
| | | | |
| | [[File:bremss44MeV.png | 600 px]] | | [[File:bremss44MeV.png | 600 px]] |
| − | | + | [[File:Al 44MeV.png.png | 600 px]] |
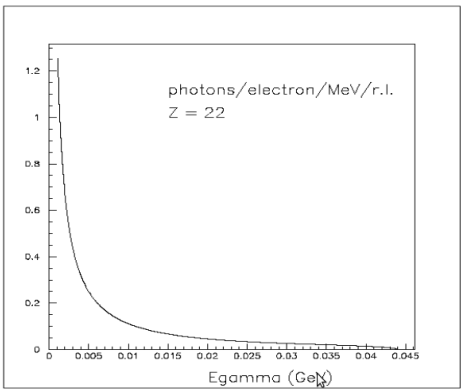
| − | in (10,20) MeV region we have about '''0.1 photons/electrons/MeV/r.l'''
| |
| − | | |
| − | ====bremsstrahlung for Al====
| |
| − | | |
| − | [[File:Aluminum-44MeV.png | 600 px]] | |
| | | | |
| | in (10,20) MeV region we have about '''0.1 photons/electrons/MeV/r.l''' | | in (10,20) MeV region we have about '''0.1 photons/electrons/MeV/r.l''' |
Revision as of 04:47, 2 February 2011
Go Back
LINAC parameters used in calculations
1) pulse width 50 ps
2) pulse current 50 A
3) repetition rate 300 Hz
4) energy 44 MeV
Number of electrons/sec on radiator
[math] 50\ \frac{C}{sec} \times \frac{1\cdot e^-}{1.6\cdot 10^{-19}\ C} \times 50\ \mbox{ps} \times 300\ \mbox{Hz} = 0.47 \cdot 10^{13}\ \frac{e^-}{sec}[/math]
Number of photons/sec out of radiator
1/2 mil of Ti
- [math]\sigma_{brems}=0.1\ \mbox{photons/electrons/MeV/r.l}[/math]
- [math]\mbox{r.l.(Ti)} = 3.59\ \mbox{cm}[/math]
- [math]\mbox{radiator}\ \mbox{thickness} = 12.5\ \mu m[/math]
[math]\frac{12.5\ \mu m}{3.59\ cm} = 3.48 \cdot 10^{-4}\ r.l.[/math]
[math]0.47 \cdot 10^{13}\ \frac{e^-}{sec} \times 0.1\ \frac{\gamma 's}{(e^- \cdot MeV \cdot r.l.)} \times 3.48 \cdot 10^{-4}\ r.l. \times 10\ MeV =1.63 \cdot 10^{9} \frac{\gamma}{sec}[/math]
Alex factor is 6.85 %
[math]1.63 \cdot 10^{9} \frac{\gamma}{sec} \cdot 6.85\ % = 1.12 \cdot 10^{8} \frac{\gamma}{sec}[/math]
1/2 mil of Al
- [math]\sigma_{brems}=xxx\ \mbox{photons/electrons/MeV/r.l}[/math]
- [math]\mbox{r.l.(Al)} = 8.89\ \mbox{cm}[/math]
- [math]\mbox{radiator}\ \mbox{thickness} = 12.5\ \mu m[/math]
[math]\frac{12.5\ \mu m}{8.89\ cm} = 1.41 \cdot 10^{-4}\ r.l.[/math]
[math]0.47 \cdot 10^{13}\ \frac{e^-}{sec} \times xxx\ \frac{\gamma 's}{(e^- \cdot MeV \cdot r.l.)} \times 1.41 \cdot 10^{-4}\ r.l. \times 10\ MeV =xxx \cdot 10^{9} \frac{\gamma}{sec}[/math]
Alex factor is 6.85 %
[math]xxx \cdot 10^{9} \frac{\gamma}{sec} \cdot 6.85\ % = xxx \cdot 10^{8} \frac{\gamma}{sec}[/math]
Conversion factor from Ti to Al
All my following calculation for pair production rate are based on (1/2) mil of Ti radiator. If we want to recalculate for (1/2) mil of Al converter we need to use the conversion factor:
[math]\frac{aaa}{1.12} = ddd [/math]
Appendix
 600 px
600 px
in (10,20) MeV region we have about 0.1 photons/electrons/MeV/r.l
Pair production rate
out of Al converter
[math]\sigma_{pairs} = 0.5\ \frac{\mbox{barns}}{\mbox{atom}}[/math]
[math]l = 3.0\ \mu m[/math] (by varying width we can vary the yield)
[math]N_{Al} = \frac{2.70\ \frac{g}{cm^3} \times 6.02 \cdot 10^{23}\ \frac{atoms}{mol} \times 3.0\ \mu m} {26.98\ \frac{g}{mol}} = 1.81 \cdot 10^{23}\ \frac{\mbox{atoms}}{m^2}[/math]
[math]\frac {1.12 \cdot 10^{8}\ \frac{\gamma}{sec} \times \sigma_{pairs} \times N_{Al}} {f} = 3.38\ \frac{\mbox{pairs}}{\mbox{pulse}} [/math]
through 1 m of air
Assume air consists entirely from Nitrogen:
[math]\sigma_{pairs}\ (\mbox{Nitrogen}) = 0.15\ \frac{\mbox{barns}}{\mbox{atom}}[/math]
[math]l = 1.0\ \mbox{m}[/math]
[math]N_{\mbox{Nitrogen}} = \frac{0.00125\ \frac{g}{cm^3} \times 6.02 \cdot 10^{23}\ \frac{atoms}{mol} \times 1.0\ \mbox{m}} {14.01\ \frac{g}{mol}} = 5.37 \cdot 10^{25}\ \frac{\mbox{atoms}}{m^2}[/math]
[math]\frac {1.12 \cdot 10^{8}\ \frac{\gamma}{sec} \times \sigma_{\mbox{pairs}} \times N_{\mbox{Nitrogen}}} {f} = 300\ \frac{\mbox{pairs}}{\mbox{pulse}} [/math]
Appendix
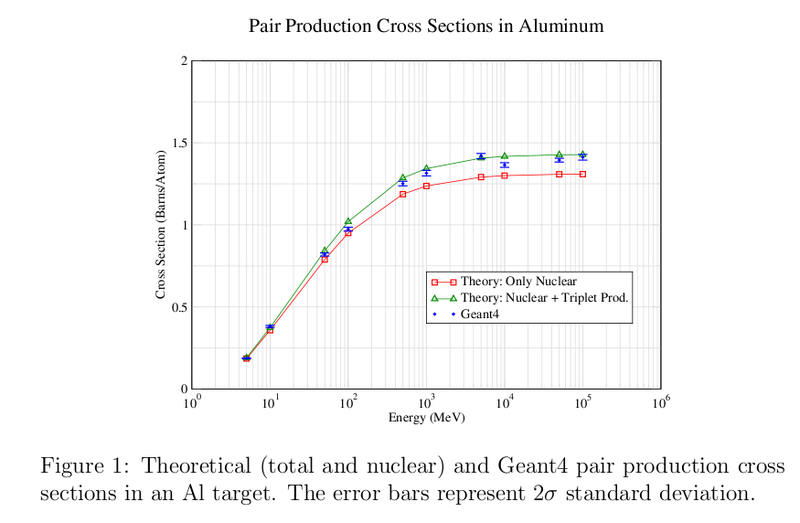
pair production cross sections in an Al target
Ref. Geant4 and Theoretical Pair Production Cross Sections for 1 MeV - 100 GeV photons in Aluminum. Vakho Makarashvili, December 18, 2007
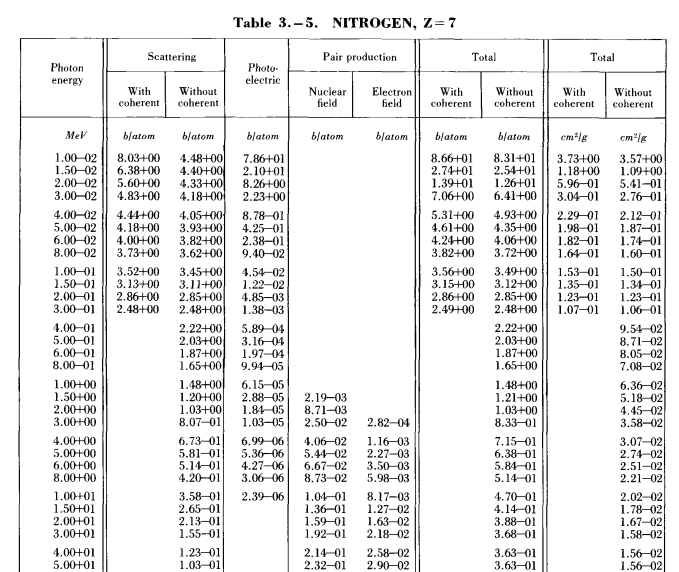
pair production cross sections in a Nitrogen
Ref. Photon Cross Section, Attenuation Coefficients, and Energy Absorption Coefficients From 10 keV to 100 Gev. J.H.Hubbell. Center for Radiation Research.National Bureau of Standards. Washington, D.C. 20234
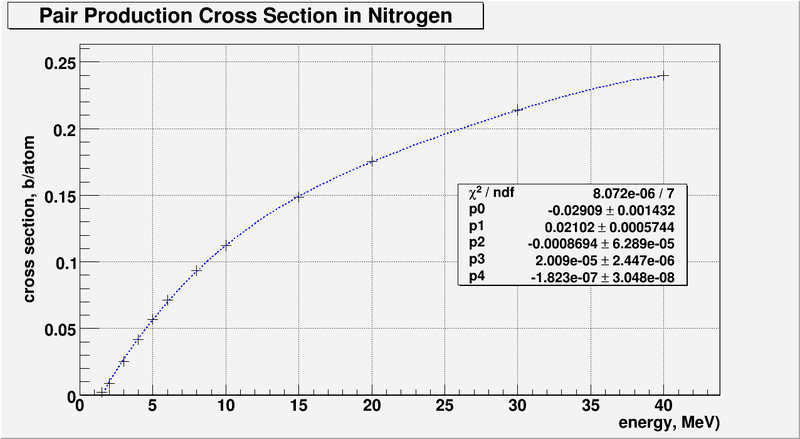
Here I just plotted the table above for pair production cross section in (0, 40) MeV energy region
Go Back



