Difference between revisions of "New 4-momentum quantities"
Jump to navigation
Jump to search
(Created page with "=New 4-Momentum Quantities= Working in just the CM frame, we can form new 4-Momentum Vectors comprised of 4-Momenta in this frame, with <center><math>{\mathbf P_1^*}- {\mathbf P…") |
|||
| Line 20: | Line 20: | ||
and the fact that the length of these 4-Momentum Vectors are invariant, | and the fact that the length of these 4-Momentum Vectors are invariant, | ||
| − | <center><math>\left({\mathbf P_1^*}- {\mathbf P_1^{'*}}\right)^2=\left({\mathbf P_1^*}^2-2{\mathbf P_1^*}\cdot {\mathbf P_1^{'*}}+ {\mathbf P_1^{'*}}\right)= \left( \begin{matrix}E_1^*-E_1^{'*}\\ p_{1(x)}^*-p_{1(x)}^{'*} \\ p_{1(y)}^*-p_{1(y)}^{'*} \\ p_{1(z)}^*-p_{1(z)}^{'*}\end{matrix} \right)^2=\left({\mathbf P_a^*}\right)^2</math></center> | + | <center><math>\left({\mathbf P_1^*}- {\mathbf P_1^{'*}}\right)^2=\left({\mathbf P_1^*}^2-2{\mathbf P_1^*}\cdot {\mathbf P_1^{'*}}+ {\mathbf P_1^{'*}}^2 \right)= \left( \begin{matrix}E_1^*-E_1^{'*}\\ p_{1(x)}^*-p_{1(x)}^{'*} \\ p_{1(y)}^*-p_{1(y)}^{'*} \\ p_{1(z)}^*-p_{1(z)}^{'*}\end{matrix} \right)^2=\left({\mathbf P_a^*}\right)^2</math></center> |
| − | <center><math>\left({\mathbf P_1^*}- {\mathbf P_2^{'*}}\right)^2=\left({\mathbf P_1^*}^2-2{\mathbf P_1^*}\cdot {\mathbf P_2^{'*}}+ {\mathbf P_2^{'*}}\right)= \left( \begin{matrix}E_1^*-E_2^{'*}\\ p_{1(x)}^*-p_{2(x)}^{'*} \\ p_{1(y)}^*-p_{2(y)}^{'*} \\ p_{1(z)}^*-p_{2(z)}^{'*}\end{matrix} \right)^2=\left({\mathbf P_b^*}\right)^2</math></center> | + | <center><math>\left({\mathbf P_1^*}- {\mathbf P_2^{'*}}\right)^2=\left({\mathbf P_1^*}^2-2{\mathbf P_1^*}\cdot {\mathbf P_2^{'*}}+ {\mathbf P_2^{'*}}^2 \right)= \left( \begin{matrix}E_1^*-E_2^{'*}\\ p_{1(x)}^*-p_{2(x)}^{'*} \\ p_{1(y)}^*-p_{2(y)}^{'*} \\ p_{1(z)}^*-p_{2(z)}^{'*}\end{matrix} \right)^2=\left({\mathbf P_b^*}\right)^2</math></center> |
| − | <center><math>\left({\mathbf P_2^*}- {\mathbf P_1^{'*}}\right)^2=\left({\mathbf P_2^*}^2-2{\mathbf P_2^*}\cdot {\mathbf P_1^{'*}}+ {\mathbf P_1^{'*}}\right)= \left( \begin{matrix}E_2^*-E_1^{'*}\\ p_{2(x)}^*-p_{1(x)}^{'*} \\ p_{2(y)}^*-p_{1(y)}^{'*} \\ p_{2(z)}^*-p_{1(z)}^{'*}\end{matrix} \right)^2=\left({\mathbf P_c^*}\right)^2</math></center> | + | <center><math>\left({\mathbf P_2^*}- {\mathbf P_1^{'*}}\right)^2=\left({\mathbf P_2^*}^2-2{\mathbf P_2^*}\cdot {\mathbf P_1^{'*}}+ {\mathbf P_1^{'*}}^2 \right)= \left( \begin{matrix}E_2^*-E_1^{'*}\\ p_{2(x)}^*-p_{1(x)}^{'*} \\ p_{2(y)}^*-p_{1(y)}^{'*} \\ p_{2(z)}^*-p_{1(z)}^{'*}\end{matrix} \right)^2=\left({\mathbf P_c^*}\right)^2</math></center> |
| − | <center><math>\left({\mathbf P_2^*}- {\mathbf P_2^{'*}}\right)^2=\left({\mathbf P_2^*}^2-2{\mathbf P_2^*}\cdot {\mathbf P_2^{'*}}+ {\mathbf P_2^{'*}}\right)= \left( \begin{matrix}E_2^*-E_2^{'*}\\ p_{2(x)}^*-p_{2(x)}^{'*} \\ p_{2(y)}^*-p_{2(y)}^{'*} \\ p_{2(z)}^*-p_{2(z)}^{'*}\end{matrix} \right)^2=\left({\mathbf P_d^*}\right)^2</math></center> | + | <center><math>\left({\mathbf P_2^*}- {\mathbf P_2^{'*}}\right)^2=\left({\mathbf P_2^*}^2-2{\mathbf P_2^*}\cdot {\mathbf P_2^{'*}}+ {\mathbf P_2^{'*}}^2 \right)= \left( \begin{matrix}E_2^*-E_2^{'*}\\ p_{2(x)}^*-p_{2(x)}^{'*} \\ p_{2(y)}^*-p_{2(y)}^{'*} \\ p_{2(z)}^*-p_{2(z)}^{'*}\end{matrix} \right)^2=\left({\mathbf P_d^*}\right)^2</math></center> |
Using the fact that the scalar product of a 4-momenta with itself is invariant, | Using the fact that the scalar product of a 4-momenta with itself is invariant, | ||
Revision as of 09:48, 2 June 2017
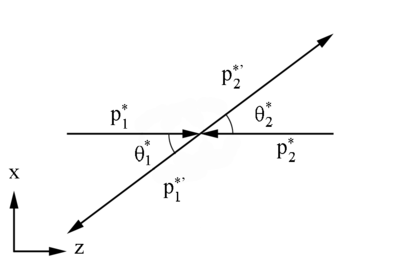
New 4-Momentum Quantities
Working in just the CM frame, we can form new 4-Momentum Vectors comprised of 4-Momenta in this frame, with
Using the algebraic fact
and the fact that the length of these 4-Momentum Vectors are invariant,
Using the fact that the scalar product of a 4-momenta with itself is invariant,
We can simiplify the expressions
Finding the cross terms,
Using the fact that in the CM frame,

Since this is an ellastic collision between identical particles, Energy is conserved,
Lastly as shown earlier,
We can further simplify