Determining wire-phi correspondance

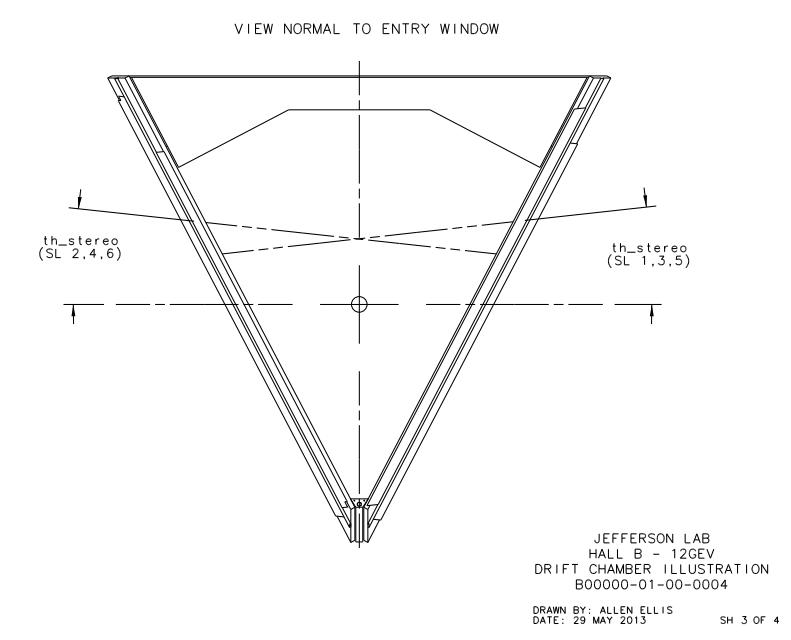
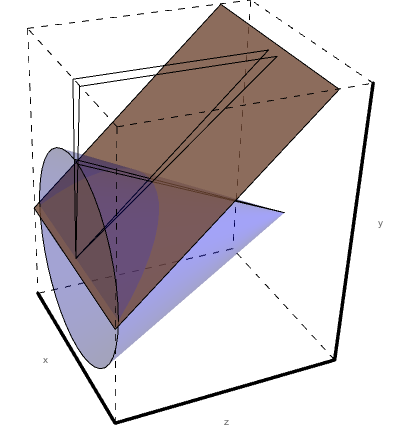
Using Mathematica, we can produce a 3D rendering of how the sectors for Level 1 would have to interact with a steady angle theta with respect to the beam line, as angle phi is rotated through 360 degrees.

Looking just at sector 1, we can see that the intersection of level 1 and the cone of constant angle theta forms a conic section.

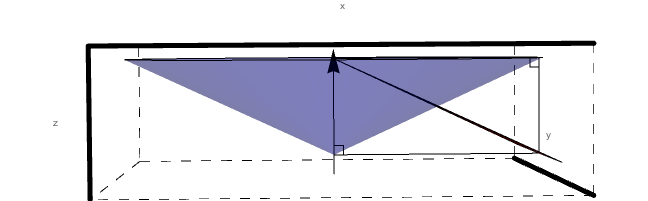
Following the rules of conic sections we know that the eccentricity of the conic is given by:

[math]\displaystyle{ e=\frac{\sin \beta}{\sin\alpha} }[/math]

Where β is the angle of the plane, and α is the slant of the cone.

If the conic is an circle, e=0

If the conic is an parabola, e=1

If the conic is an ellipse, [math]\displaystyle{ e=\sqrt{1-\frac{b^2}{a^2}} }[/math]

For ellipses centered at (j,k):

[math]\displaystyle{ \frac{(x-j)^2}{a^2}+\frac{(y-k)^2}{b^2}=1 }[/math]

where

a = major radius (= 1/2 length major axis) b = minor radius (= 1/2 length minor axis)

For a parabola:

[math]\displaystyle{ 4px = y^2 }[/math]

where

p = distance from vertex to focus (or directrix)