Difference between revisions of "Positrons Using The HRRL"
| (6 intermediate revisions by the same user not shown) | |||
| Line 23: | Line 23: | ||
Reference serkan thesis | Reference serkan thesis | ||
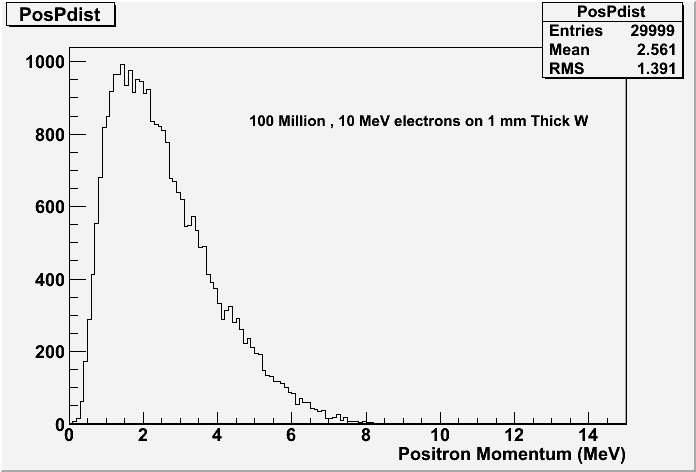
| + | GEANT4 simulation of 100 Million , 10 MeV electrons hitting a 0.8 mm thick Tungsten target at an angle of 45 degrees. | ||
| + | |||
| + | [[File:HRRL_PosPdist_10MeV1mmW_71912.png]] | ||
| + | |||
| + | |||
| + | GEANT4 predicts about 1 , 2 MeV positron for every 10,000 electrons. To get a single positron in a pulse you need a peak current of at least | ||
| + | :<math>\frac{10^5 \times 1.6 \times 10^{-19} Coul }{300 \times 10^{-9}} = 50 </math>nA | ||
| + | |||
| + | ;collection and detection efficiency would need to be 100% for this to happen. | ||
===Electron Momentum Distributions=== | ===Electron Momentum Distributions=== | ||
| Line 30: | Line 39: | ||
The electrons which penetrate a 1 mm Tungsten foil have the momentum distribution below | The electrons which penetrate a 1 mm Tungsten foil have the momentum distribution below | ||
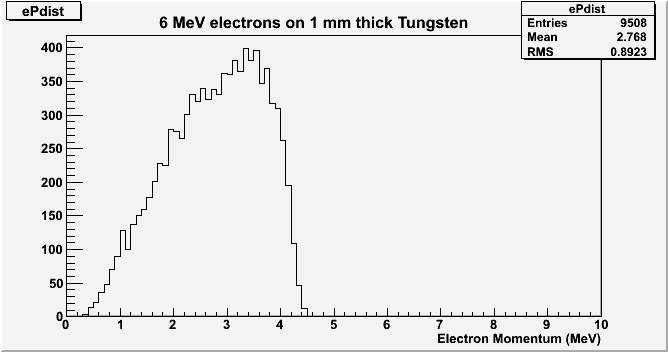
| − | + | Accelerating 100,000 6 MeV electrons onto a 1 mm thick Tungsten target | |
[[File:HRRL_6MeVePdist_71812.png]] | [[File:HRRL_6MeVePdist_71812.png]] | ||
| Line 37: | Line 46: | ||
| − | + | Accelerating 100,000 10 MeV electrons onto a 1 mm thick Tungsten target | |
[[File:HRRL_10MeVePdist_71812.png]] | [[File:HRRL_10MeVePdist_71812.png]] | ||
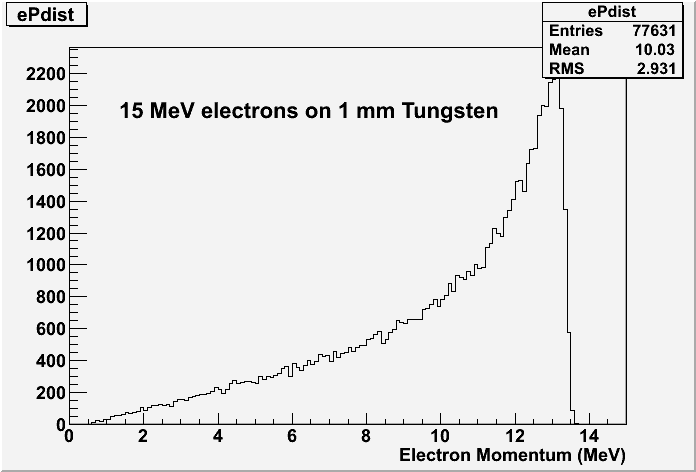
| − | + | Accelerating 100,000 15 MeV electrons onto a 1 mm thick Tungsten target | |
[[File:HRRL_15MeVePdist_71812.png]] | [[File:HRRL_15MeVePdist_71812.png]] | ||
Latest revision as of 14:32, 19 July 2012
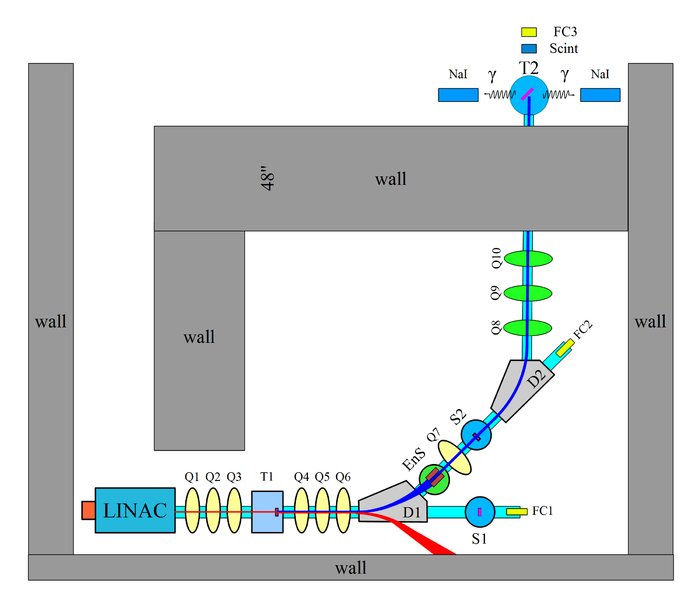
The purpose of this research is to evaluate the veracity of a positron beam line using the quad triplet method to collect positrons produced when a 10 MeV electron beam impinges on a 1.25 mm thick Tungsten target.
Positron Beam Properties
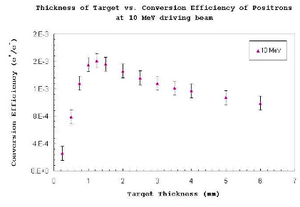
Tungsten thickness
The optimal Tungsten target thickness to produce positrons using a 10 MeV incident electron beam appears to be 1.25 mm.
Reference Serkan Thesis
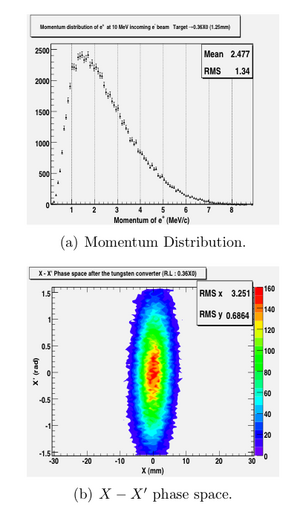
Positron Momentum and Phase space distribution
Assuming a 10 meV electron beam having a gaussian spot size with a = 3mm.
We may want the achromat to have a 10% or less energy spread to measure the above distribution but we will also wan to open the slits to maximize positron current. This may mean accepting an energy spread of 30%.
Reference serkan thesis
GEANT4 simulation of 100 Million , 10 MeV electrons hitting a 0.8 mm thick Tungsten target at an angle of 45 degrees.
GEANT4 predicts about 1 , 2 MeV positron for every 10,000 electrons. To get a single positron in a pulse you need a peak current of at least
- nA
- collection and detection efficiency would need to be 100% for this to happen.
Electron Momentum Distributions
According to GEANT4
The electrons which penetrate a 1 mm Tungsten foil have the momentum distribution below
Accelerating 100,000 6 MeV electrons onto a 1 mm thick Tungsten target
If the incident beam is 100 mA then you might get a current of 100*400/100000 = 0.4 mA of 3 MeV electrons.
Accelerating 100,000 10 MeV electrons onto a 1 mm thick Tungsten target
Accelerating 100,000 15 MeV electrons onto a 1 mm thick Tungsten target
Measured HRRL emittance
HRRL beam line