Difference between revisions of "Niowave 11-2015"

From New IAC Wiki
Jump to navigation Jump to search
 
(3 intermediate revisions by the same user not shown)
Line 1: Line 1:
−
A 10 MeV electron beam with a radius of 0.5 cm was incident on a 2 mm thick PbBi target.  The target is positioned at Z = -902 mm. 
 
 
Niowave Positron Project Progress for November 2015
 
Niowave Positron Project Progress for November 2015
  
  
 
The next step in the positron project, after determining the PbBi thickness for maximum positron production, was to estimate the heat that would be deposited from the primary electron beam on the beam pipe.  Electrons scattered by the PbBi target will intersect a beam pipe used to transport the desired positrons.  Electrons traversing the beam pipe can deposit energy that will heat the beam pipe.  The goal of this simulation was to estimate the amount of the deposited energy to determine if there is sufficient cooling to prevent a beam pipe breach.  Below is a preliminary design of the beam pipe dimensions.
 
The next step in the positron project, after determining the PbBi thickness for maximum positron production, was to estimate the heat that would be deposited from the primary electron beam on the beam pipe.  Electrons scattered by the PbBi target will intersect a beam pipe used to transport the desired positrons.  Electrons traversing the beam pipe can deposit energy that will heat the beam pipe.  The goal of this simulation was to estimate the amount of the deposited energy to determine if there is sufficient cooling to prevent a beam pipe breach.  Below is a preliminary design of the beam pipe dimensions.
−
 
−
 
  
  
Line 29: Line 26:
 
|}
 
|}
  
−
A comparison between the prediction from MCNPX and GEANT4 was once again performed.
+
A comparison between the prediction from MCNPX and GEANT4 was once again performed.
 +
A 10 MeV electron beam with a radius of 0.5 cm was incident on a 2 mm thick PbBi target.  The target is positioned at Z = -902 mm. 
 
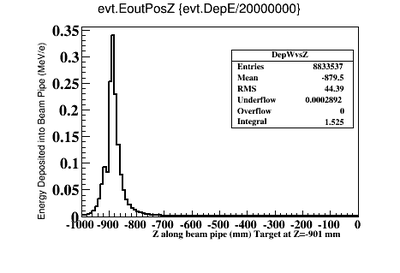
As shown in the figure below, GEANT4 predicts a peak energy deposition of 0.35 MeV/e while MCNPX predicts about 0.38 MeV/e.  GEANT4 predicts a total of <math>3.08\times 10^7</math> MeV (the integral adds up the energy in each 1cm bin) of energy will be deposited in  
 
As shown in the figure below, GEANT4 predicts a peak energy deposition of 0.35 MeV/e while MCNPX predicts about 0.38 MeV/e.  GEANT4 predicts a total of <math>3.08\times 10^7</math> MeV (the integral adds up the energy in each 1cm bin) of energy will be deposited in  
−
a 1m long beam pipe surrounding a 2 mm thick PbBi target located at Z=-902 mm when 20 million electrons impinge the target.   
+
a 1m long beam pipe surrounding a 2 mm thick PbBi target located at Z=-902 mm when 20 million electrons impinge the target.  The next step is to repeat his calculation in the presence of a solenoid surrounding the target.
 
 
 
{| border="1"
 
{| border="1"
−
| [[File:BeamPipeDepEmev_34.8_082915.png |200px]] || [[File:MCNPXBeamPipeDepEmev_34.8_090315.png |200px]]  
+
| [[File:BeamPipeDepEmev_34.8_082915.png |400px]] || [[File:MCNPXBeamPipeDepEmev_34.8_090315.png |400px]]  
 
|+ Energy deposited (MeV) in a 1 m long 3.48 cm diameter beam pipe surrounding a 2 mm target located at Z=-900 mm.
 
|+ Energy deposited (MeV) in a 1 m long 3.48 cm diameter beam pipe surrounding a 2 mm target located at Z=-900 mm.
−
|}
 
−
 
−
 
−
 
−
 
−
 
−
The energy deposited by electrons scattered into a 1.65 mm thick stainless steel beam pipe from the PbBi target as a function of a uniform solenoidal magnetic field was investigated for a 3.48 diameter stainless steel beam pipe.  The simulation program GEANT4 was used to determine the amount of energy an electron lost in the stainless steel beam pipe.  The beam pipe surface was divided into unit squares that were a centimeter in length and the energy deposited by a sample of 5 million electrons was summed for each unit square.  The left side figure below shows a predicted peak energy deposition of 0.35 MeV/e^- in the 1.65 mm thick stainless steel beam pipe per 10 MeV electron impinging the 2 mm thick LBE target that has been sandwiched between two 0.25 mm thick stainless steel windows. 
 
−
 
−
The energy deposited in the beam pipe can be converted to an average heat load if a average beam current is assumed.
 
−
Under the assumption of a 1mA (10^-3 C/s) average beam current, the deposited energy (E_{dep}) per cm^2 per electron can be converted to the deposited power (P) per area using the equation
 
−
 
−
<math>P= E_{dep} \left( \frac{\mbox{MeV}}{\mbox{cm}^2 \mbox{e}^-}\right) \times \left( \frac{ \mbox{e}^-}{1.6 \times 10^{-19}\mbox{C}}  \right ) \times \left( \frac{1 \times 10^{-3} \mbox{C}}{\mbox{s}} \right )  \times \left( \frac{1.6 \times 10^{-13}\mbox{W} \cdot \mbox{ s}}{\mbox{MeV} }\right ).</math>
 
−
 
−
In other words, the energy deposited in units of keV/cm^2/e by a 1 mA electron beam is equivalent to a heat load in the units of Watts per cm^2.
 
−
 
−
<math>\left( \frac{\mbox{keV}}{\mbox{cm}^2 \mbox{e}^-}\right) = \left( \frac{\mbox{W} }{\mbox{cm}^2 } \right )</math>
 
−
 
−
Based on this observation the peak heat load, as shown in the figure below on the right, for a 1 mA electron beam is 35 W/cm^2.  The electrons will begin to intersect a 3.48 diameter beam pipe less when the solenoidal magnetic field strength increases beyond one Tesla.  A solenoidal field between two and four Tesla would substantially reduce the electron heat load on the beam pipe and place the positrons in a tight helical orbit for transportation.
 
−
 
−
 
−
{| border="1"
 
−
| [[File:BeamPipeDepEmev-vs-B.png |200px]] || [[File:BeamPipeDepPower-vs-B.png |200px]] 
 
−
|+ Energy deposited (MeV) along a 1 m long beam pipe of stainless steel 1.65 mm thick.
 
 
|}
 
|}

Latest revision as of 15:20, 13 April 2016

Niowave Positron Project Progress for November 2015


The next step in the positron project, after determining the PbBi thickness for maximum positron production, was to estimate the heat that would be deposited from the primary electron beam on the beam pipe. Electrons scattered by the PbBi target will intersect a beam pipe used to transport the desired positrons. Electrons traversing the beam pipe can deposit energy that will heat the beam pipe. The goal of this simulation was to estimate the amount of the deposited energy to determine if there is sufficient cooling to prevent a beam pipe breach. Below is a preliminary design of the beam pipe dimensions.


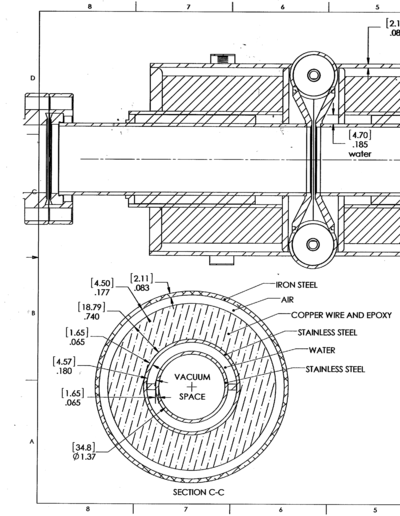
TF Niowave SolenoidDesign 9-3-15.png
Element dimension
Inner beam pipe radius 1.74 cm
Inner beam pipe thickness 0.165 cm
water jacket thickness 0.457 cm
outer beam pipe radius 2.362 cm
outer beam pipe thickness 0.165 cm
Solenoid inner radius 2.527 cm
Solenoid outer radius 4.406 cm

A comparison between the prediction from MCNPX and GEANT4 was once again performed. A 10 MeV electron beam with a radius of 0.5 cm was incident on a 2 mm thick PbBi target. The target is positioned at Z = -902 mm. As shown in the figure below, GEANT4 predicts a peak energy deposition of 0.35 MeV/e while MCNPX predicts about 0.38 MeV/e. GEANT4 predicts a total of [math]3.08\times 10^7[/math] MeV (the integral adds up the energy in each 1cm bin) of energy will be deposited in a 1m long beam pipe surrounding a 2 mm thick PbBi target located at Z=-902 mm when 20 million electrons impinge the target. The next step is to repeat his calculation in the presence of a solenoid surrounding the target.

BeamPipeDepEmev 34.8 082915.png MCNPXBeamPipeDepEmev 34.8 090315.png
Energy deposited (MeV) in a 1 m long 3.48 cm diameter beam pipe surrounding a 2 mm target located at Z=-900 mm.
Retrieved from "https://wiki.iac.isu.edu/index.php?title=Niowave_11-2015&oldid=105745"