Forest UCM MiNF

Mechanics in Noninertial Reference Frames

Linearly accelerating reference frames

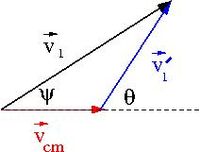
Let [math]\mathcal S_0[/math] represent an inertial reference frame and \mathcal S represent an noninertial reference frame with acceleration [math]\vec A[/math] relative to [math]\mathcal S_0[/math].

Ball thrown straight up

Consider the motion of a ball thrown straight up as viewed from [math]\mathcal S[/math].

Using a Galilean transformation (not a relativistic Lorentz transformation)

At some instant in time the velocities add like

[math]\dot {\vec {r}_0} = \dot {\vec r}+ \vec V[/math]

where

[math]\vec V[/math] = velocity of moving frame [math]\mathcal S[/math] with respect to [math]\mathcal S_0[/math] at some instant in time

[math]\Rightarrow \dot {\vec r} = \dot {\vec {r}_0} - \vec V[/math]

taking derivative with respect to time

[math]\Rightarrow \ddot {\vec r} = \ddot {\vec {r}_0} - \dot \vec V= \ddot {\vec {r}_0} - \vec A [/math]

[math]\Rightarrow m\ddot {\vec r} = m\ddot {\vec {r}_0} - m \vec A= \vec F - m\vec A= \vec F - \vec {F}_{\mbox {inertial}}[/math]

where

[math]\vec {F}_{\mbox {inertial}} = m \vec A \equiv[/math] inertial force

in your noninertial frame, the ball appears to have a force causing it to accelerate in the [math]\vec A[/math] direction.

The inertial force may also be referred to as a fictional force

an example is the "fictional" centrifugal force for rotational acceleration.

The observer in a noninertial reference frame will feel these frictional forces as if they are real but they are really a consequence of your accelerating reference frame

example

A force pushes you back into your seat when your Jet airplane takes off

you slam on the brakes and hit your head on the car's dashboard

Forest_Ugrad_ClassicalMechanics