Difference between revisions of "Relative efficiency"

From New IAC Wiki
Jump to navigation Jump to search
 
(120 intermediate revisions by the same user not shown)
Line 8: Line 8:
  
  
−
'''Rletive efficiency obtained from the 2n opening angle w/ uncorrelated neutrons'''
+
==Rletive efficiency obtained from the 2n opening angle w/ uncorrelated neutrons==
  
 
The result of simulation of 2n openeing angle obtained by different neutron detectors (each neutron hit different detector) having y-resolution is presented below. The source of neutrons was isotropic.
 
The result of simulation of 2n openeing angle obtained by different neutron detectors (each neutron hit different detector) having y-resolution is presented below. The source of neutrons was isotropic.
Line 24: Line 24:
 
| Point isotropic n-source w/o material || Volume isotropic n-source + DU material
 
| Point isotropic n-source w/o material || Volume isotropic n-source + DU material
 
|}
 
|}
−
 
  
 
The result of simulation of 2n openeing angle obtained by the same neutron detectors (2 neutrons hit same detector) having y-resolution is presented below:
 
The result of simulation of 2n openeing angle obtained by the same neutron detectors (2 neutrons hit same detector) having y-resolution is presented below:
Line 34: Line 33:
 
[[File:relative_effcy_diff_pulses.png || 800px]]
 
[[File:relative_effcy_diff_pulses.png || 800px]]
  
−
'''Relative efficiency of the detecting system obtained using experimental data only (all runs)'''
+
==Relative efficiency of the detecting system obtained using experimental data only (all runs)==
  
 
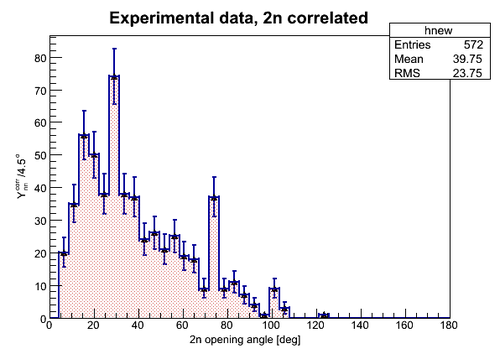
In this part the experimantal data are presented for the cases when (1) the 2n opening angle was measured for neutrons correlated and (2) the 2n opening angle was measured for neutrons uncorrelated (neutrons from separate pulses). In the figure below the experimental data on the 2n opening angle measured for the case of correlated neutrons is presented where the statistical error bar width is equal to <math>\pm \sqrt{N_{bin}}</math> along y-axis and 4.5 deg along x-axis (the bin width). The total number of triggering pulses during the experiment was Nexp_pulses=2.469256E+7.
 
In this part the experimantal data are presented for the cases when (1) the 2n opening angle was measured for neutrons correlated and (2) the 2n opening angle was measured for neutrons uncorrelated (neutrons from separate pulses). In the figure below the experimental data on the 2n opening angle measured for the case of correlated neutrons is presented where the statistical error bar width is equal to <math>\pm \sqrt{N_{bin}}</math> along y-axis and 4.5 deg along x-axis (the bin width). The total number of triggering pulses during the experiment was Nexp_pulses=2.469256E+7.
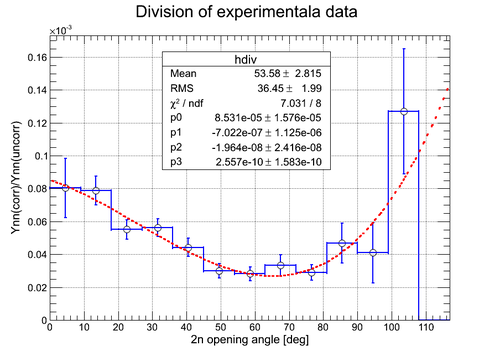
Line 48: Line 47:
 
[[File:corr_over_uncorr_fit.png || 500px]]
 
[[File:corr_over_uncorr_fit.png || 500px]]
  
−
'''Normalization'''
+
==Normalization==
  
 
Data table 1.
 
Data table 1.
Line 54: Line 53:
 
{| class="wikitable"
 
{| class="wikitable"
 
! style="text-align: center;" | run#
 
! style="text-align: center;" | run#
−
! style="text-align: center;" | Total # of events per run
+
! style="text-align: center;" | Total # of pulses per run
 
|-
 
|-
 
| style="text-align: center;" | run4118
 
| style="text-align: center;" | run4118
Line 131: Line 130:
 
| style="text-align: center; background: red; color: white" | 24692561
 
| style="text-align: center; background: red; color: white" | 24692561
 
|}
 
|}
 +
 +
Normalization factor is 24692561.
 +
 +
[[File:2n_corr_opAngle_normalized.png | 500px]]
  
 
Data table 2. Uncorrelated neutron pairs.
 
Data table 2. Uncorrelated neutron pairs.
Line 189: Line 192:
 
| style="text-align: center;" | 177560268
 
| style="text-align: center;" | 177560268
 
| style="text-align: center;" | 64360835399566
 
| style="text-align: center;" | 64360835399566
−
|-
+
|-Y_{nn}^{corr}
 
| style="text-align: center;" | run4154
 
| style="text-align: center;" | run4154
 
| style="text-align: center;" | 1695297
 
| style="text-align: center;" | 1695297
Line 261: Line 264:
 
|-
 
|-
 
| style="text-align: center;" | totals
 
| style="text-align: center;" | totals
−
| style="text-align: center;" | 36068732
+
| style="text-align: center;background: black; color: white" | 36068732
 
| style="text-align: center;background: green; color: white" | 542970453
 
| style="text-align: center;background: green; color: white" | 542970453
 
| style="text-align: center;background: blue; color: white" | 734498220663898
 
| style="text-align: center;background: blue; color: white" | 734498220663898
 
|}
 
|}
 +
 +
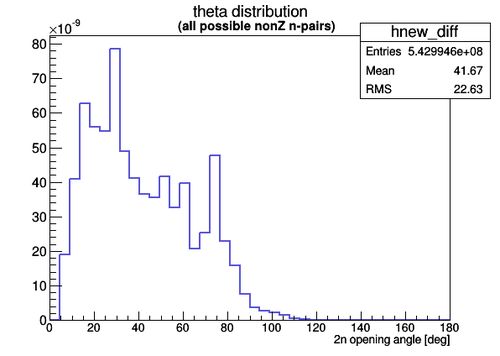
In the case if all possible non zero 2n pairs are considered the shape then the unncorrelated 2n opening angle distribution normalized looks like (the normalization factor is the total number of all possible n-pairs 734498220663898):
 +
 +
[[File:2n_op_ang_all_nonZpairs.png | 500px]]
 +
 +
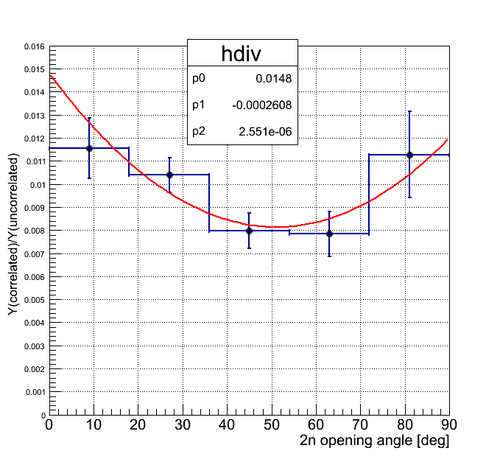
The division of normalized experimental data fir the case of correlared 2n pairs and uncorrelated 2n pairs (all non zero n pairs are taken into account) gives the following 2n angle distribution:
 +
 +
[[File:division_norm_exp_2ndata2.png | 500px]]
 +
 +
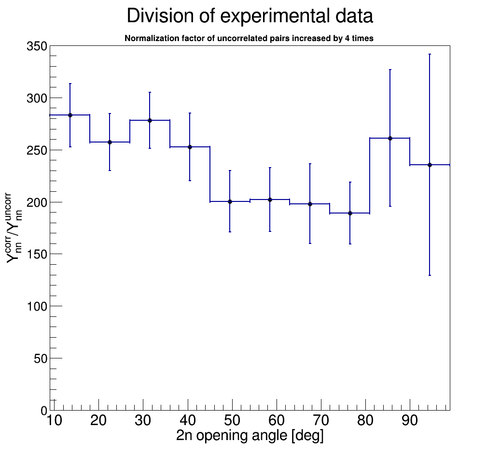
If the normalization factor of uncorrelated neutron pairs increased by 4 then the 2n opening angle distribution looks like:
 +
 +
[[File:division_4xNorm_exp_2ndata.png | 500px]]
 +
 +
==Y(80 n-pairs)/Y(tot # of n-pairs)==
 +
 +
{| cellpadding="2" style="border: 1px solid darkgray;"
 +
! width="400" | 80 uncorrelated neutron pairs uniformly distributed
 +
! width="400" | All possible uncorrelated n-pairs
 +
|- border="0"
 +
| [[File:exp_data_2n_uncorrelated_Err2.png|500px|Region 1]]
 +
| [[File:ytot2.png|500px|Region 2]]
 +
|- align="center"
 +
| 80 n-pairs algorithm || All possible combinations
 +
|}
 +
 +
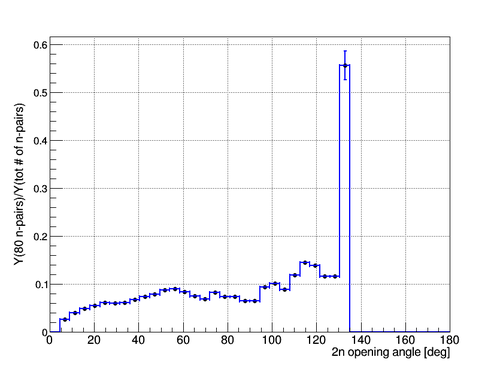
The result of division of Y(80 n-pairs) over Y(tot # of n-pairs) is presented below
 +
 +
[[File:y80_over_ytot.png | 500px]]
 +
 +
==Relative efficiency by random pairing neutrons from different pulses==
 +
 +
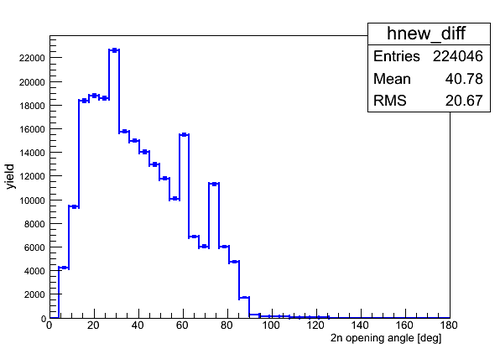
2n opening angle distribution obtained by random sampling of neutron pairs (without repetition of a neutrons taken once) is presented below:
 +
 +
[[File:yuncorr_shuffle.png | 500px]]
 +
 +
The relative efficiency obtained as a result of division of 2n opening angle distribution in the case of correlated neutron pairs by 2n opening angle distribution obtained by random sampling of uncorrelated neutron pairs is presented below:
 +
 +
[[File:ycorr_over_yuncorr_shuffle.png | 500px ]]
 +
 +
==Relative efficiency by pairing neutrons from different pulses (ascending event #)==
 +
 +
Another algorithm of neutron pairing was used to obtain the relative efficiency of the detecting system. Every run data file was sorted such that the event number order was ascending (see [https://wiki.iac.isu.edu/index.php/ASCII_data_files], second column in the table) and two neighboring neutrons from different detectors and different events were used to make a pair and the opening angle was calculated.
 +
 +
The normalization procedure of the opening angle distribution in the case of different pulses was the following
 +
 +
[[File:run_summary_norm.pdf]]
 +
 +
After going through the data one more time there were found some suspicious events. They were filtered out from the data. Now the range of 2n opening angles matches the range of the angles obtained via geant simulation for the current experimental setup (see the top section on the current page).
 +
 +
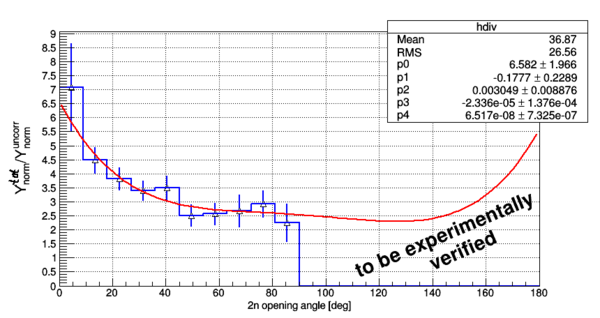
The fit on the picture below shows one of the possible trends which the experimental data may follow and is needed to be experimentally proved.
 +
 +
Over the y-axis it is plotted normalized ratio <math>(Y^{corr}+Y^{uncorr})/Y^{uncorr} = Y^{tot}/Y^{uncorr}</math>.
 +
 +
[[File:Ycoor_ovr_Yuncorr_norm_cleaned3.png | 600px]]
 +
 +
==The ratio of the integrated yields==
 +
 +
The ratio of the normalized integrated yields of correlated + uncorrelated neutrons per pulse <math>Y^{\text{tot}}=2.2E-5</math>  and calculated yield of uncorrelated neutrons per pulse <math>Y^{\text{2n/fisson}}=6.49E-6</math> gives the following number <math>\frac{Y^{\text{tot}}}{Y^{\text{2 fisson}}}=3.4</math>. If we want to get the ratio of pure yield of correlated neutrons <math>Y^{\text{1n/fission}}</math> over the yield of uncorrelated neutrons <math>Y^{\text{2n/fisson}}</math> we should take the difference <math>Y^{\text{1n/fission}}=Y^{\text{tot}}-Y^{\text{2n/fisson}}</math> and then take the ratio <math>\frac{Y^{\text{1n/fission}}}{Y^{\text{2n/fisson}}} = \frac{(2.2E-5)-(6.49E-6)}{6.49E-6}=2.4</math>
 +
 +
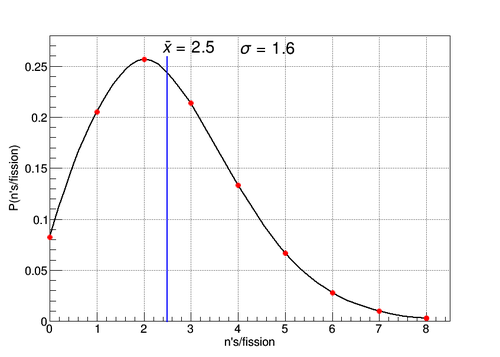
Poisson distribution <math>P(n)=\frac{\bar{n}^n  e^{-\bar{n}}}{n!}=\frac{(2.5)^ne^{-2.5}}{n!}</math> of the number of fission neutrons per fission event if the average number of neutrons emitted in fission event is 2.5 neutrons.
 +
 +
[[File:poisson_2_5n_ave.png | 500px]]
 +
 +
'''Bin-by-bin subtraction'''
 +
 +
 +
Now let's plot the ratio <math>(Y^{tot}-Y^{uncorr})/Y^{uncorr}=Y^{corr}/Y^{uncorr}</math>. The error propagation is going to be the following:
 +
 +
<math>\frac{Y^{tot}-Y^{uncorr}}{Y^{uncorr}} = \frac{(a \pm \delta a) - (b \pm \delta b)}{(b \pm \delta b)}</math>
 +
 +
<math>=\frac{(a-b) \pm (\sqrt{\delta a^2 + \delta b^2})}{b \pm \delta b}</math>
 +
 +
<math>=\frac{a-b}{b} \pm Err(\frac{Y^{tot}-Y^{uncorr}}{Y^{uncorr}})</math>
 +
 +
<math>Err(\frac{Y^{tot}-Y^{uncorr}}{Y^{uncorr}}) = \frac{a-b}{b} \sqrt{\frac{\delta a^2 + \delta b^2}{(a-b)^2}+(\frac{\delta b}{b})^2}</math>
 +
 +
The plot of the ratio of yield of pure correlated neutrons over the yield of pure uncorrelated neutrons looks like
 +
 +
[[File:Pure_corrY_over_uncorrY2.png | 600px]]

Latest revision as of 15:33, 22 September 2014

Some papers

File:Nn correlation extraction.pdf


File:Ynn vs Dnn.pdf


Rletive efficiency obtained from the 2n opening angle w/ uncorrelated neutrons

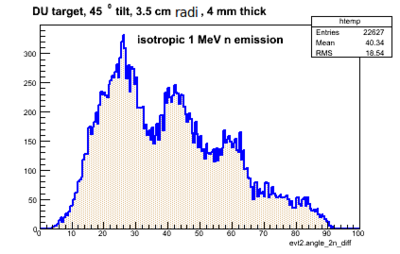
The result of simulation of 2n openeing angle obtained by different neutron detectors (each neutron hit different detector) having y-resolution is presented below. The source of neutrons was isotropic.



Empty target, [math]10^8[/math] events sampled DU target, [math]10^8[/math] events sampled
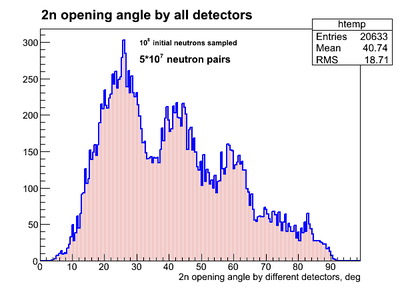
Region 1 Region 2
Point isotropic n-source w/o material Volume isotropic n-source + DU material

The result of simulation of 2n openeing angle obtained by the same neutron detectors (2 neutrons hit same detector) having y-resolution is presented below:

2n op angle all sameDets.png

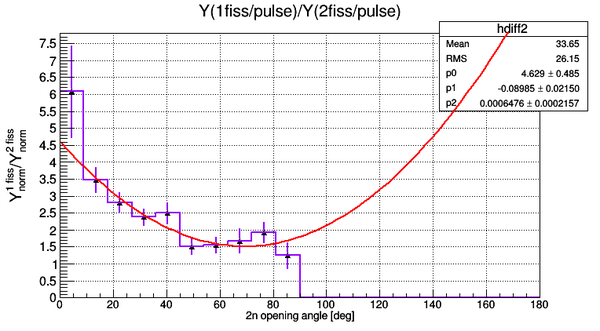
In the experiment our expereimental setup did not have y-resolution across the surface of the neutron detectors. So it was necessary to simulate the 2n opening angle for the neutron detectors w/o y-resolution. The result of the simulation of 2n opening angle (the 2n angles detected by all detectors are superimposed) for the case where thre was no y-resolution is going to be noted as [math]D_{nn}[/math]. The experimental data obtained from run 4172 (DU target) on 2n opening angle for uncorrelated neutrons (i.e. in the case of isotropic source of neutrons) from different pulses is going to be noted as [math]Y_{nn}[/math]. The relative efficiency is defined as [math]\epsilon=Y_{nn}/D_{nn}[/math] and it is plotted below as a result of bib-by-bin division of [math]Y_{nn}[/math] over [math]D_{nn}[/math].

Relative effcy diff pulses.png

Relative efficiency of the detecting system obtained using experimental data only (all runs)

In this part the experimantal data are presented for the cases when (1) the 2n opening angle was measured for neutrons correlated and (2) the 2n opening angle was measured for neutrons uncorrelated (neutrons from separate pulses). In the figure below the experimental data on the 2n opening angle measured for the case of correlated neutrons is presented where the statistical error bar width is equal to [math]\pm \sqrt{N_{bin}}[/math] along y-axis and 4.5 deg along x-axis (the bin width). The total number of triggering pulses during the experiment was Nexp_pulses=2.469256E+7.

Exp data 2n correlated Err.png

The experimental data on the 2n opening angle measured for the case of uncorrelated neutrons is presented in the next figure. 80 neutrons from different pulses were taken uniformly to reproduce 2n opening angle for the case of uncorrelated neutrons. The total number of uncorrelated neutron pairs was Npair_uncorr = 3.608397E+7. The width of the error bars is smaller then the size of the markers.

Exp data 2n uncorrelated Err2.png

The result of the division of the two histograms ([math]Y_{nn}^{correlated}/Y_{nn}^{uncorrelated}[/math]) obtained for the described two cases is shown below. In order to obtain the resulting histogram as a division of the previous two histograms we need to do the division on the bin-by-bin basis as [math]\frac{a \pm \delta a}{b \pm \delta b}[/math], where [math]a[/math] and [math]b[/math] are the contents of the corresponding bins and [math]\delta a[/math] and [math]\delta b[/math] are the coresponding statistical errors for the bin contents [math]a[/math] and [math]b[/math]. The error bars were obtained by the propagation in the following way: [math]\delta Err = \frac{a}{b}\sqrt{(\frac{\delta a}{a})^2+(\frac{\delta b}{b})^2}[/math]. The resulting histogram wa normalized to the ratio of the total number of pulses to the total number of uncorrelated neutron pairs considered Norm = Nexp_pulses/Npair_uncorr = 2.469256E+7/3.608397E+7 . It should be noted that the total number of uncorrelated neutron pairs can be varied by changing the number of corresponding combinations and the number of pulses involved into the 2n opening angle reproduction, so the normalization may change.

Corr over uncorr fit.png

Normalization

Data table 1.

run# Total # of pulses per run
run4118 1668482
run4119 2135901
run4133 1704331
run4134 1732490
run4135 1603604
run4137 800699
run4144 1645667
run4145 1544605
run4146 510826
run4153 1750657
run4154 1631436
run4155 1062048
run4166 214600
run4169 191592
run4170 429974
run4171 1141113
run4172 650734
run4173 354992
run4174 953831
run4175 299086
run4176 144774
run4177 225877
run4203 659050
run4204 1636192
totals 24692561

Normalization factor is 24692561.

2n corr opAngle normalized.png

Data table 2. Uncorrelated neutron pairs.

run# 80 puls pairs all nonZ pairs total # of pairs
run4118 1949796 35168980 58460510939894
run4119 2470399 57115623 95803579611944
run4133 1741760 30145143 60999663129029
run4134 1832885 33384324 63031990015343
run4135 1727993 28580648 54002495269034
run4137 833189 7099684 13463513489481
run4144 1770693 26603021 56872651957830
run4145 1592222 20562899 50101929186175
run4146 491705 2157292 5479817982802
run4153 8753312 177560268 64360835399566
run4154 1695297 26345381 55893286150871
run4155 1182325 12257834 23686887361551
run4166 174390 226636 967120869210
run4169 175558 235498 770861409690
run4170 319678 1008712 3882439488990
run4171 1415700 19159461 27344940440490
run4172 623978 3412116 8892563188751
run4173 395573 1327163 2646413182232
run4174 894440 5765727 19105685150123
run4175 255450 619705 1878507428108
run4176 110058 119818 440152774689
run4177 976415 1721476 1071433552715
run4203 3003073 23564022 9121298818219
run4204 1682843 28829022 56219643867161
totals 36068732 542970453 734498220663898

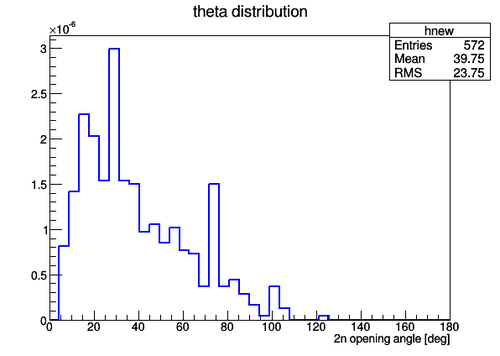
In the case if all possible non zero 2n pairs are considered the shape then the unncorrelated 2n opening angle distribution normalized looks like (the normalization factor is the total number of all possible n-pairs 734498220663898):

2n op ang all nonZpairs.png

The division of normalized experimental data fir the case of correlared 2n pairs and uncorrelated 2n pairs (all non zero n pairs are taken into account) gives the following 2n angle distribution:

Division norm exp 2ndata2.png

If the normalization factor of uncorrelated neutron pairs increased by 4 then the 2n opening angle distribution looks like:

Division 4xNorm exp 2ndata.png

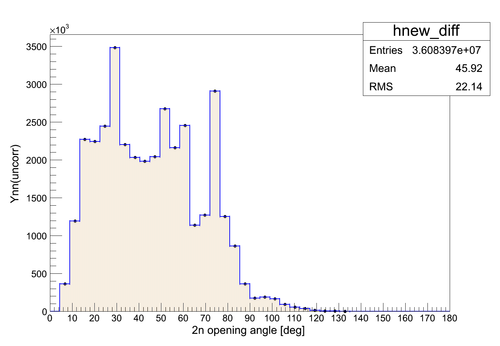
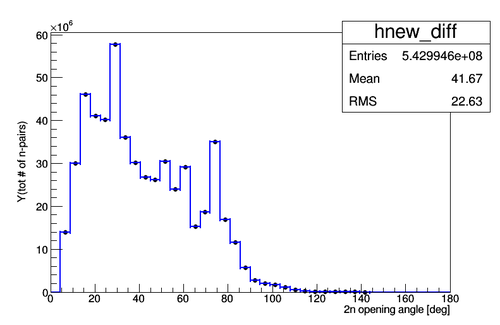
Y(80 n-pairs)/Y(tot # of n-pairs)

80 uncorrelated neutron pairs uniformly distributed All possible uncorrelated n-pairs
Region 1 Region 2
80 n-pairs algorithm All possible combinations

The result of division of Y(80 n-pairs) over Y(tot # of n-pairs) is presented below

Y80 over ytot.png

Relative efficiency by random pairing neutrons from different pulses

2n opening angle distribution obtained by random sampling of neutron pairs (without repetition of a neutrons taken once) is presented below:

Yuncorr shuffle.png

The relative efficiency obtained as a result of division of 2n opening angle distribution in the case of correlated neutron pairs by 2n opening angle distribution obtained by random sampling of uncorrelated neutron pairs is presented below:

Ycorr over yuncorr shuffle.png

Relative efficiency by pairing neutrons from different pulses (ascending event #)

Another algorithm of neutron pairing was used to obtain the relative efficiency of the detecting system. Every run data file was sorted such that the event number order was ascending (see [1], second column in the table) and two neighboring neutrons from different detectors and different events were used to make a pair and the opening angle was calculated.

The normalization procedure of the opening angle distribution in the case of different pulses was the following

File:Run summary norm.pdf

After going through the data one more time there were found some suspicious events. They were filtered out from the data. Now the range of 2n opening angles matches the range of the angles obtained via geant simulation for the current experimental setup (see the top section on the current page).

The fit on the picture below shows one of the possible trends which the experimental data may follow and is needed to be experimentally proved.

Over the y-axis it is plotted normalized ratio [math](Y^{corr}+Y^{uncorr})/Y^{uncorr} = Y^{tot}/Y^{uncorr}[/math].

Ycoor ovr Yuncorr norm cleaned3.png

The ratio of the integrated yields

The ratio of the normalized integrated yields of correlated + uncorrelated neutrons per pulse [math]Y^{\text{tot}}=2.2E-5[/math] and calculated yield of uncorrelated neutrons per pulse [math]Y^{\text{2n/fisson}}=6.49E-6[/math] gives the following number [math]\frac{Y^{\text{tot}}}{Y^{\text{2 fisson}}}=3.4[/math]. If we want to get the ratio of pure yield of correlated neutrons [math]Y^{\text{1n/fission}}[/math] over the yield of uncorrelated neutrons [math]Y^{\text{2n/fisson}}[/math] we should take the difference [math]Y^{\text{1n/fission}}=Y^{\text{tot}}-Y^{\text{2n/fisson}}[/math] and then take the ratio [math]\frac{Y^{\text{1n/fission}}}{Y^{\text{2n/fisson}}} = \frac{(2.2E-5)-(6.49E-6)}{6.49E-6}=2.4[/math]

Poisson distribution [math]P(n)=\frac{\bar{n}^n e^{-\bar{n}}}{n!}=\frac{(2.5)^ne^{-2.5}}{n!}[/math] of the number of fission neutrons per fission event if the average number of neutrons emitted in fission event is 2.5 neutrons.

Poisson 2 5n ave.png

Bin-by-bin subtraction


Now let's plot the ratio [math](Y^{tot}-Y^{uncorr})/Y^{uncorr}=Y^{corr}/Y^{uncorr}[/math]. The error propagation is going to be the following:

[math]\frac{Y^{tot}-Y^{uncorr}}{Y^{uncorr}} = \frac{(a \pm \delta a) - (b \pm \delta b)}{(b \pm \delta b)}[/math]

[math]=\frac{(a-b) \pm (\sqrt{\delta a^2 + \delta b^2})}{b \pm \delta b}[/math]

[math]=\frac{a-b}{b} \pm Err(\frac{Y^{tot}-Y^{uncorr}}{Y^{uncorr}})[/math]

[math]Err(\frac{Y^{tot}-Y^{uncorr}}{Y^{uncorr}}) = \frac{a-b}{b} \sqrt{\frac{\delta a^2 + \delta b^2}{(a-b)^2}+(\frac{\delta b}{b})^2}[/math]

The plot of the ratio of yield of pure correlated neutrons over the yield of pure uncorrelated neutrons looks like

Pure corrY over uncorrY2.png

Retrieved from "https://wiki.iac.isu.edu/index.php?title=Relative_efficiency&oldid=94975"