Difference between revisions of "Forest UCM NLM AtwoodMachine"
Jump to navigation
Jump to search
| (3 intermediate revisions by the same user not shown) | |||
| Line 107: | Line 107: | ||
1 & -m_1 & m_1 \\ | 1 & -m_1 & m_1 \\ | ||
1 & m_2 & m_2 \\ | 1 & m_2 & m_2 \\ | ||
| − | 2 & 0 & -m_3 \end{array} \right) | + | 2 & 0 & -m_3 \end{array} \right)\left( \begin{array}{ccc} |
| + | T_1 \\ | ||
| + | a_r \\ | ||
| + | a_3 \end{array} \right) = \left( \begin{array}{ccc} | ||
| + | m_1 g \\ | ||
| + | m_2 g \\ | ||
| + | m_3 g\end{array} \right) | ||
</math> | </math> | ||
| + | |||
| + | Cramer's Rule: | ||
| + | |||
| + | |||
| + | :<math>a_3 = \frac{\left| \begin{array}{ccc} | ||
| + | 1 & -m_1 & m_1g \\ | ||
| + | 1 & m_2 & m_2g \\ | ||
| + | 2 & 0 & m_3g \end{array} \right|}{\left| \begin{array}{ccc} | ||
| + | 1 & -m_1 & m_1 \\ | ||
| + | 1 & m_2 & m_2 \\ | ||
| + | 2 & 0 & -m_3 \end{array} \right|}</math> | ||
| + | |||
[[Forest_UCM_NLM#Atwoods_Machine]] | [[Forest_UCM_NLM#Atwoods_Machine]] | ||
Latest revision as of 12:52, 22 August 2014
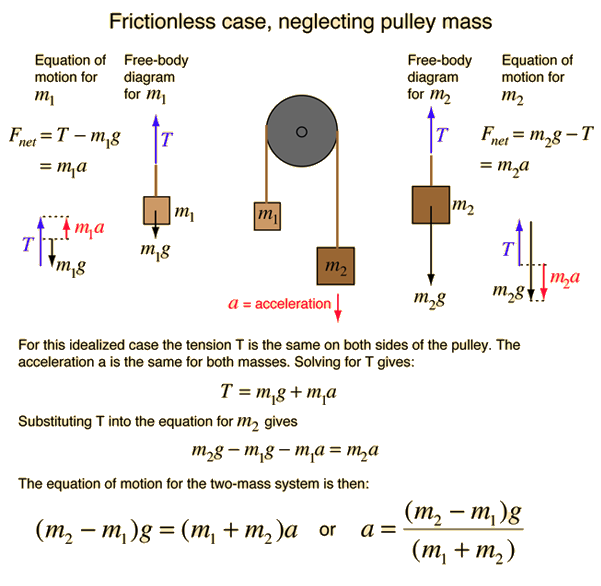
Simple Atwood's machine
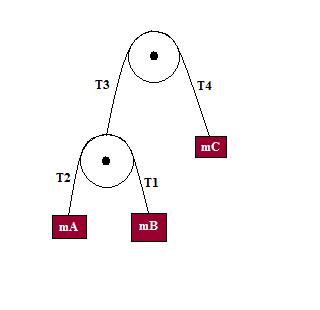
Double Atwood's machine
The problem
Determine the acceleration of each mass in the above picture.
Step 1: Identify the system
- Each block is a separate system with two external forces; a gravitational force and the rope tension.
Step 2: Choose a suitable coordinate system
- A coordinate system with one axis that defines the posive direction as up is one possible orientation.
Step 3: Draw the Free Body Diagram
Step 4: Define the Force vectors using the above coordinate system
since the system is one dimensional I will omit the vector notation
- Tension in the rope attached to mass
- Tension in the rope attached to mass
- Tension in the rope attached to mass
- = force of gravity on each mass or or
Step 5: Use Newton's second law
- for mass 1
- for mass 2
- for mass 3
If we know the mass of all the objects in the system then we are left with three unkown Tensions and three unknown acceleratios. In total we currently have 6 unkowns and 3 equations.
Using Newton's third law we know that reducing the unkowns to 5.
- We need 2 more equations!
External Forces on Lower pulley
Consider the external forces acting on the MASSLESS lower pulley
Now we have 4 unkwons and 3 equations
relative acceleration
let
- acceleration of with respect to the lower pulley
assuming that is moving upwards with respect to the earth
- : acceleration of lower pully as well as
similarly
- : if is accelerating upwards then is accelerating downwards
3 equations and 3 unknowns
Solutions
solving the above system of equations leads to the solutions
- Matrix method solution ( are the unkowns)
Cramer's Rule: