Difference between revisions of "Forest UCM NLM"
Jump to navigation
Jump to search
| Line 26: | Line 26: | ||
Velocity: | Velocity: | ||
| − | \vec{v} = \frac{d \vec{r}}{dt} = \frac{d x}{dt}\hat{i} + x\frac{d \hat{i}}{dt} + … = | + | \vec{v} = <math>\frac{d \vec{r}}{dt}</math> = <math>\frac{d x}{dt}\hat{i} + x\frac{d \hat{i}}{dt} + …</math> = |
===Spherical=== | ===Spherical=== | ||
Revision as of 18:27, 12 June 2014
Newton's Laws of Motion
Limits of Classical Mechanic
Classical Mechanics is the formulations of physics developed by Newton (1642-1727), Lagrange(1736-1813), and Hamilton(1805-1865).
It may be used to describe the motion of objects which are not moving at high speeds (0.1) nor are microscopically small ( ).
The laws are formulated in terms of space, time, mass, and force:
Space and Time
Space
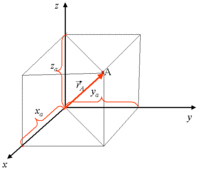
Cartesian, Spherical, and Cylindrical coordinate systems are commonly used to describe three-dimensional space.
Cartesian
Vector Notation convention:
Position:
Velocity: \vec{v} = = =