Difference between revisions of "D2O bank"
| Line 47: | Line 47: | ||
[[File:weighted_D2_xsection.png | 600 px]] | [[File:weighted_D2_xsection.png | 600 px]] | ||
| − | As an example of the efficiency calculation let's find the efficiency of Det M: | + | As an example of the efficiency calculation let's find the efficiency of Det M in terms of the known absolute efficiency of Det E: |
| − | <math>\ | + | <math>\frac{\epsilon_E}{\epsilon_M} = \frac{N_n^E \cdot Area_E}{N_n^M \cdot Area_M}</math> |
Revision as of 15:24, 17 April 2013
Relative photon flux
Relative photon flux obtained during the experiment using D2O target.
Normalization?
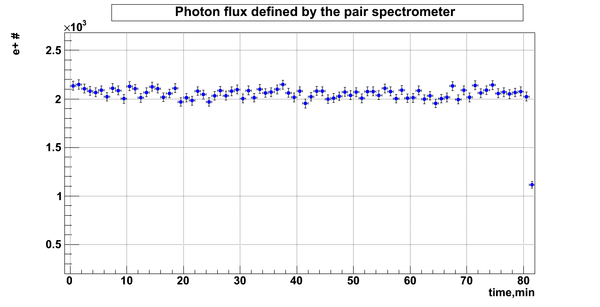
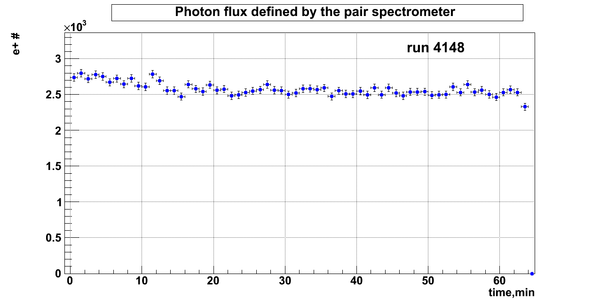
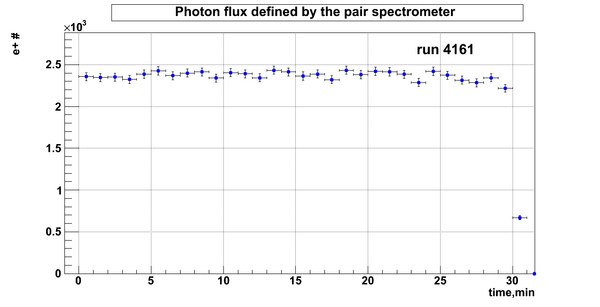
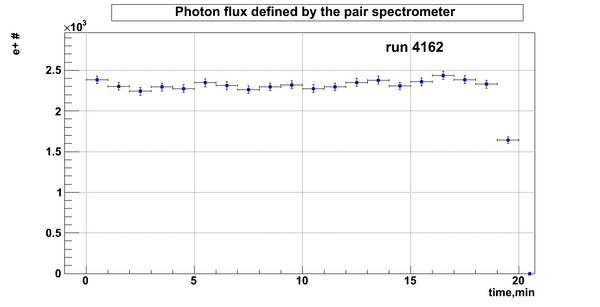
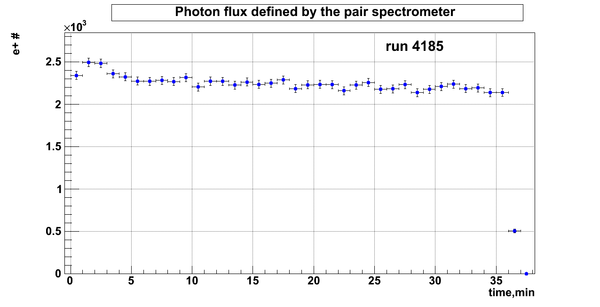
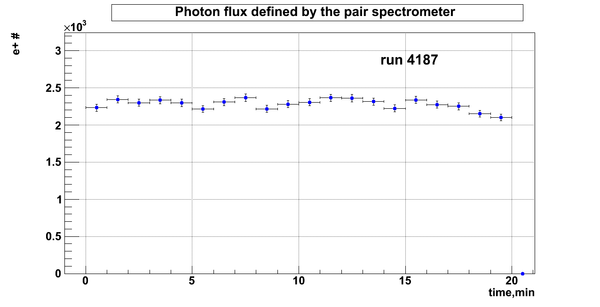
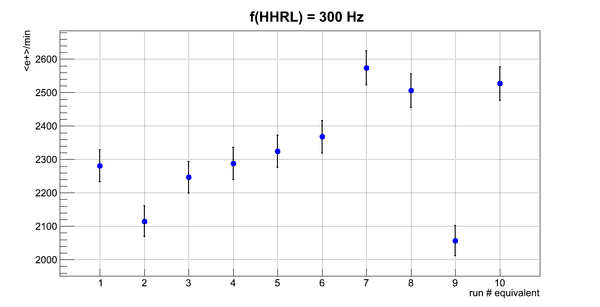
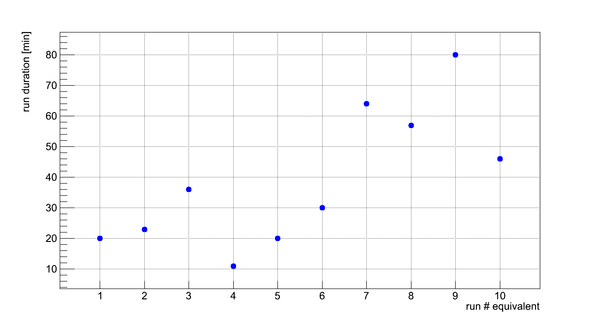
Flux fluctuation over the runs:
run # equivalent 1 corresponds to run 4187, 2 - 4186, 3 - 4185, 4 - 4164, 5 - 4162, 6 - 4161, 7 - 4148, 8 - 4138, 9 - 4126, 10 - 4111.
The thing is that the pair spectrometer is sensitive to the low energy background which may be present in the beam (e- beam finite size and, hence, scraping) so the value of the flux may be affected by low energy component. This thing may not be reflected in the number of neutrons detected by the neutron detectors. So, it is arguable that the pair spectrometer can be used for the flux normalization procedure. One has to investigate the energy spectra of the positrons detected.
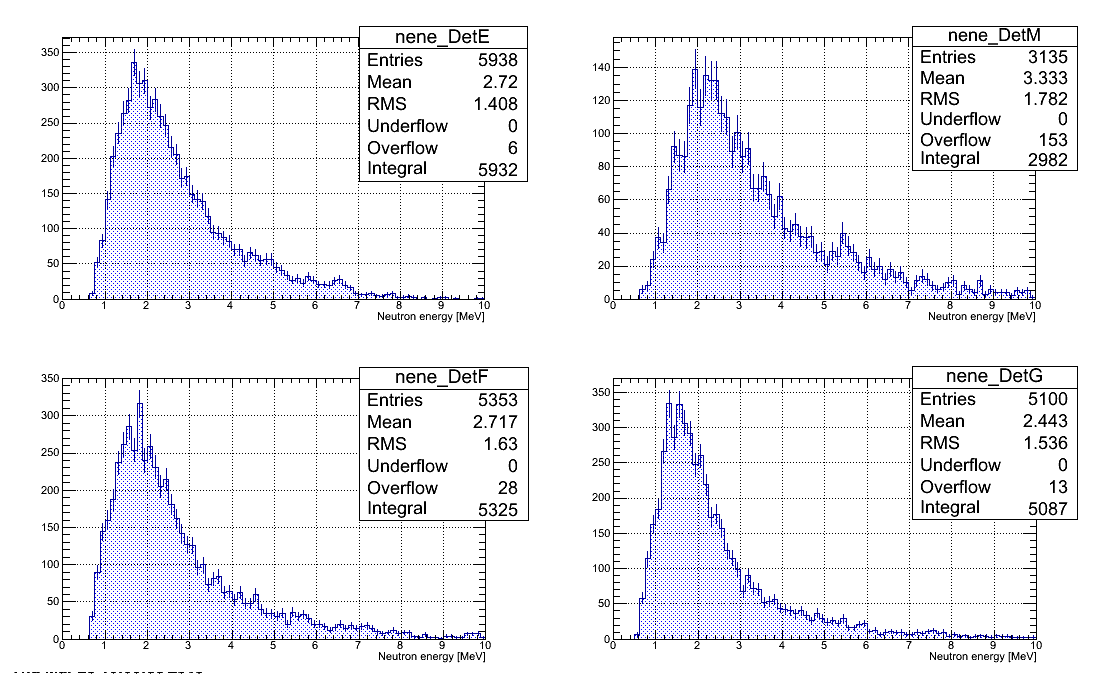
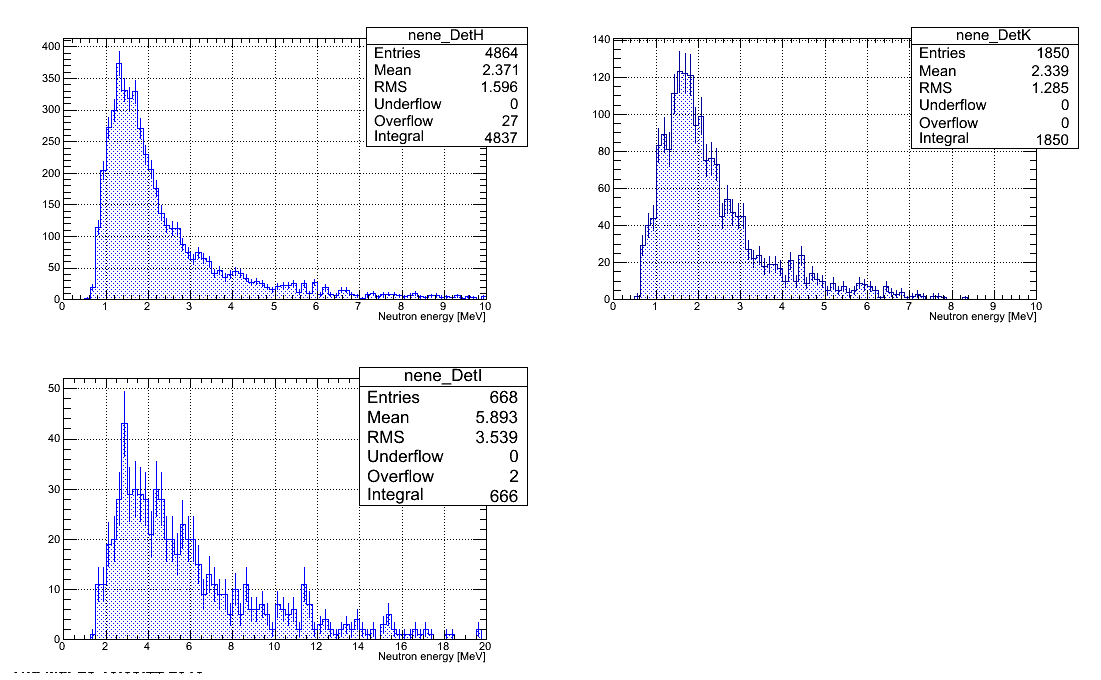
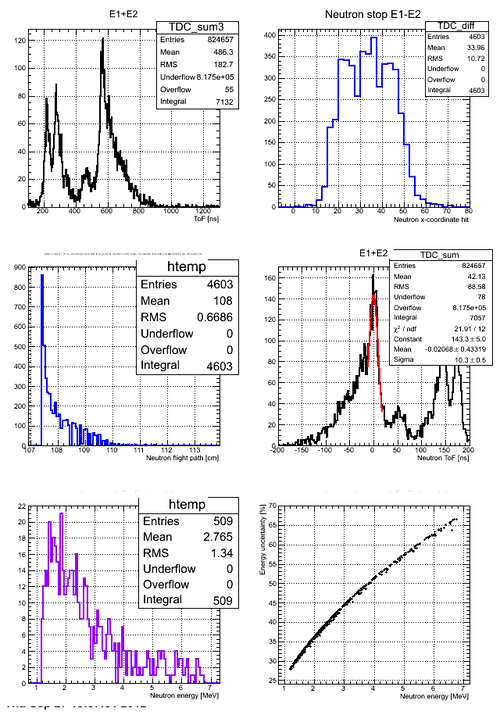
Neutron energy spectra
Neutron energy spectra restored from all the runs with D2O target are plotted below. Statistical error bars only presented. All the histograms have same number of channels.
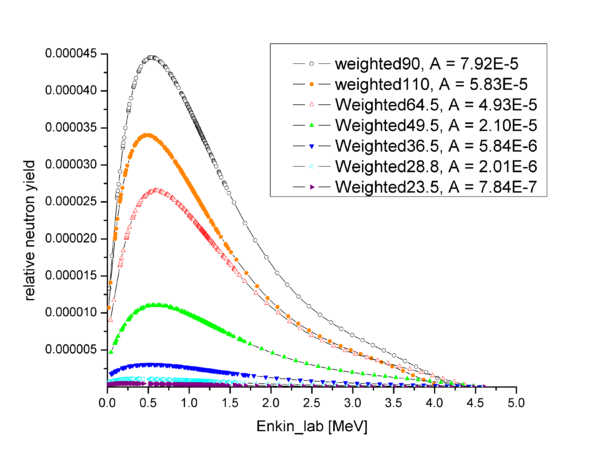
The relative neutron yield obtained by weighting the D2 photodisintegration cross section by bremsstahlung photon flux and solid angle of each of the detector is plotted below as a function of the neutron kinetic energy recalculated from the photon energy using simple kinematics:
As an example of the efficiency calculation let's find the efficiency of Det M in terms of the known absolute efficiency of Det E:
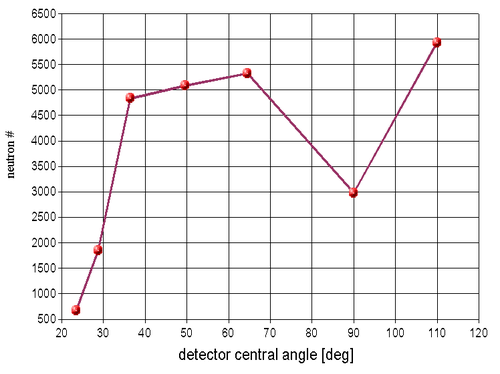
Neutron number vs neutron detector central angle is plotted below:
Energy uncertainty issue
Statistical errors on the number of neutrons per energy bin are not bad, however, big uncertainties in energy come due to the wide width of the photon peak: