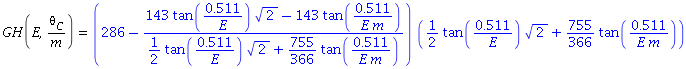Difference between revisions of "Minimum accelerator energy to run experiment"

From New IAC Wiki
Jump to navigation Jump to search
(Created page with '[http://wiki.iac.isu.edu/index.php/PhotoFission_with_Polarized_Photons_from_HRRL Go Back] =Minimum accelerator energy to run experiment= ==condition 1: fitting the collimator s...')
 
 
(69 intermediate revisions by one other user not shown)
Line 1: Line 1:
−
[http://wiki.iac.isu.edu/index.php/PhotoFission_with_Polarized_Photons_from_HRRL Go Back]
+
[https://wiki.iac.isu.edu/index.php/Roman_calculation Go Back]
  
−
=Minimum accelerator energy to run experiment=
+
=general setup=
  
−
==condition 1: fitting the collimator size into the hole==
+
[[File:minimum_energy_condition.png]]
  
−
[[File:min_energy.png|800px]]
+
= fitting the collimator size into the hole through the concrete wall=
  
−
The minimum energy of accelerator (MeV) is limited by fitting the collimator size <math>r_2</math> into the hole R = 8.73 cm:
+
I can express the distance <math>A_1D_1</math> as function of collimator size <math>\Theta_C/m</math> and electron beam energy E:
 +
 +
[[File:formula_cond11.png]]
  
−
<math>x_2 + r_2 = R</math>
+
To fit the collimator size into the hole through the concrete wall with radius R = 8.73 cm we need to solve equation:
  
−
1) Assuming the collimator diameter is <math>\Theta_C</math>:
+
<math>A_1D_1(E,\ \Theta_C/m) = 8.73\ cm</math>
  
−
<math>\frac{1}{\sqrt{2}}\ (286+183)\ \tan\left(\frac{0.511}{E_{min}}\right) +
+
1) some solutions of this equation for different collimator sizes m are:
−
      \frac{1}{2}\ (286+183)\ \tan\left(\frac{0.511}{E_{min}}\right) = 8.73 \Rightarrow E_{min} = 33.1\ MeV  </math>
 
  
−
2) Assuming the collimator diameter is <math>\Theta_C/2</math>:
+
<math>m = 1 \Rightarrow E_{min} = 33.1\ MeV  </math><br>
 +
<math>m = 2 \Rightarrow E_{min} = 26.3\ MeV  </math><br>
 +
<math>m = 4 \Rightarrow E_{min} = 22.8\ MeV  </math><br>
  
−
<math>\frac{1}{\sqrt{2}}\ (286+183)\ \tan\left(\frac{0.511}{E_{min}}\right) +
+
2) in general for arbitrary collimator size m the solutions are:
−
      \frac{1}{2}\ (286+183)\ \tan\left(\frac{1}{2}\ \frac{0.511}{E_{min}}\right) = 8.73 \Rightarrow E_{min} = 26.3\ MeV  </math>
 
  
−
3) Assuming the collimator diameter is <math>\Theta_C/4</math>:
+
[[File:energy_condition1.jpeg]]
  
−
<math>\frac{1}{\sqrt{2}}\ (286+183)\ \tan\left(\frac{0.511}{E_{min}}\right) +
+
All energies above this line is good to run experiment for condition above
−
      \frac{1}{2}\ (286+183)\ \tan\left(\frac{1}{4}\ \frac{0.511}{E_{min}}\right) = 8.73 \Rightarrow E_{min} = 22.8\ MeV  </math>
 
  
−
4) for arbitrary collimator size <math>\Theta_C/2</math>:
+
=critical collimator line condition=
  
−
[[File:plot_energy_collimatorsize.jpeg]]
+
Also I can express the distance <math>GH</math> as function of collimator size <math>\Theta_C/m</math> and electron beam energy E:
 +
 +
[[File:formula_cond21.png]]
 +
 +
If I would like that nothing hitting the 4" box to go through the collimator I need to solve equation:
  
−
All energy above this line is good to run experiment
+
<math>GH(E,\ \Theta_C/m) = 5.08\ cm</math>
  
−
==condition 2: F1A = 286 cm==
+
1) some solutions of this equation for different collimator sizes m are:
  
−
1) assuming the collimator diameter is <math>\Theta_C</math>
+
<math>m = 1 \Rightarrow E_{min} = 73.7\ MeV  </math><br>
 +
<math>m = 2 \Rightarrow E_{min} = 36.9\ MeV  </math><br>
 +
<math>m = 4 \Rightarrow E_{min} = 18.4\ MeV  </math><br>
  
−
<math> E_{min} = 73.7\ MeV  </math>
+
2) in general for arbitrary collimator size m the solutions are:
  
−
2) assuming the collimator diameter is <math>\Theta_C/2</math>
+
[[File:energy_condition2.jpeg]]
  
−
<math> E_{min} = 36.9\ MeV  </math>
+
All energies above this line is good to run experiment for condition above
  
−
3) assuming the collimator diameter is <math>\Theta_C/4</math>
+
=both solutions are together=
  
−
<math> E_{min} = 18.4\ MeV  </math>
+
[[File:energy_condition12.jpeg]]
  
−
4) for arbitrary collimator size <math>\Theta_C/m</math>:
+
All energies above this lines is good to run experiment for both conditions above
  
−
[[File:plot_energy_F1A.jpeg]]
 
−
 
−
All energy above this line is good to run experiment
 
−
 
−
==both conditions above are together==
 
−
 
−
[[File:plot_energy_bothcondition.jpeg]]
 
−
 
−
All energy above this lines is good to run experiment
 
−
 
−
[http://wiki.iac.isu.edu/index.php/PhotoFission_with_Polarized_Photons_from_HRRL Go Back]
 
−
 
−
=Minimum accelerator energy to run experiment=
 
−
 
−
==condition 1: fitting the collimator size into the hole==
 
−
 
−
[[File:min_energy.png|800px]]
 
−
 
−
The minimum energy of accelerator (MeV) is limited by fitting the collimator size <math>r_2</math> into the hole R = 8.73 cm:
 
−
 
−
<math>x_2 + r_2 = R</math>
 
−
 
−
1) Assuming the collimator diameter is <math>\Theta_C</math>:
 
−
 
−
<math>\frac{1}{\sqrt{2}}\ (286+183)\ \tan\left(\frac{0.511}{E_{min}}\right) +
 
−
      \frac{1}{2}\ (286+183)\ \tan\left(\frac{0.511}{E_{min}}\right) = 8.73 \Rightarrow E_{min} = 33.1\ MeV  </math>
 
−
 
−
2) Assuming the collimator diameter is <math>\Theta_C/2</math>:
 
−
 
−
<math>\frac{1}{\sqrt{2}}\ (286+183)\ \tan\left(\frac{0.511}{E_{min}}\right) +
 
−
      \frac{1}{2}\ (286+183)\ \tan\left(\frac{1}{2}\ \frac{0.511}{E_{min}}\right) = 8.73 \Rightarrow E_{min} = 26.3\ MeV  </math>
 
−
 
−
3) Assuming the collimator diameter is <math>\Theta_C/4</math>:
 
−
 
−
<math>\frac{1}{\sqrt{2}}\ (286+183)\ \tan\left(\frac{0.511}{E_{min}}\right) +
 
−
      \frac{1}{2}\ (286+183)\ \tan\left(\frac{1}{4}\ \frac{0.511}{E_{min}}\right) = 8.73 \Rightarrow E_{min} = 22.8\ MeV  </math>
 
−
 
−
4) for arbitrary collimator size <math>\Theta_C/2</math>:
 
−
 
−
[[File:plot_energy_collimatorsize.jpeg]]
 
−
 
−
All energy above this line is good to run experiment
 
−
 
−
==condition 2: F1A = 286 cm==
 
−
 
−
1) assuming the collimator diameter is <math>\Theta_C</math>
 
−
 
−
<math> E_{min} = 73.7\ MeV  </math>
 
−
 
−
2) assuming the collimator diameter is <math>\Theta_C/2</math>
 
−
 
−
<math> E_{min} = 36.9\ MeV  </math>
 
−
 
−
3) assuming the collimator diameter is <math>\Theta_C/4</math>
 
−
 
−
<math> E_{min} = 18.4\ MeV  </math>
 
−
 
−
4) for arbitrary collimator size <math>\Theta_C/m</math>:
 
−
 
−
[[File:plot_energy_F1A.jpeg]]
 
−
 
−
All energy above this line is good to run experiment
 
−
 
−
==both conditions above are together==
 
−
 
−
[[File:plot_energy_bothcondition.jpeg]]
 
−
 
−
All energy above this lines is good to run experiment
 
  
  
 
[http://wiki.iac.isu.edu/index.php/PhotoFission_with_Polarized_Photons_from_HRRL Go Back]
 
[http://wiki.iac.isu.edu/index.php/PhotoFission_with_Polarized_Photons_from_HRRL Go Back]

Latest revision as of 19:03, 24 May 2012

Go Back

general setup

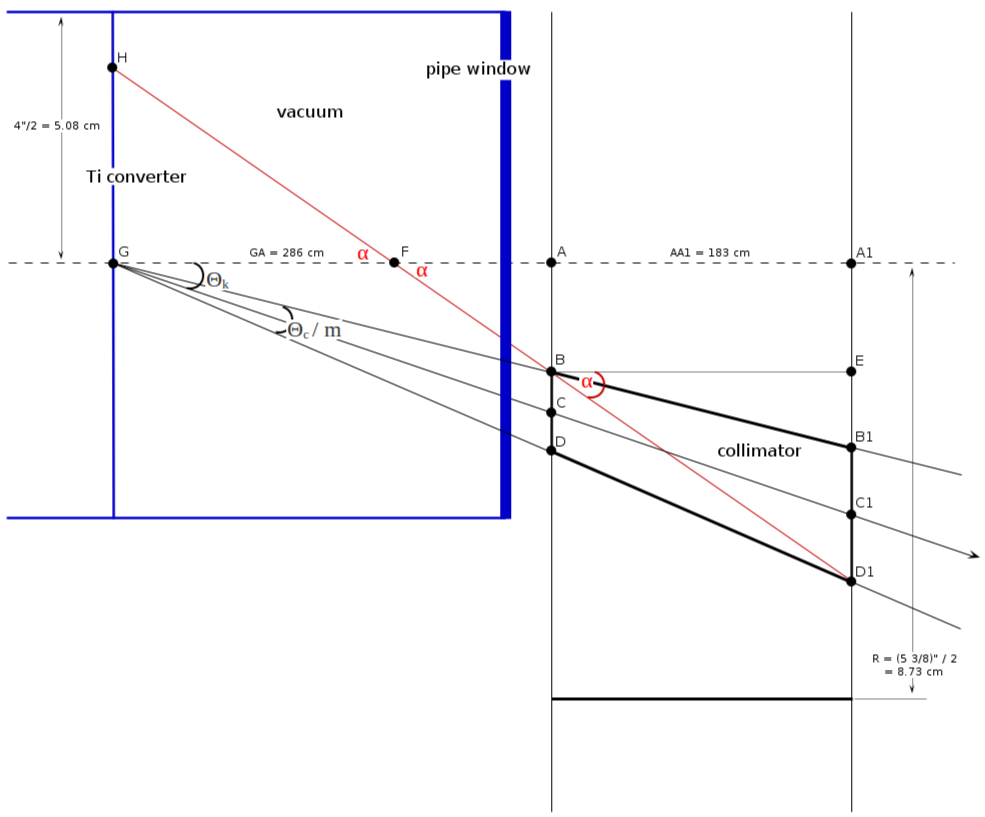
Minimum energy condition.png

fitting the collimator size into the hole through the concrete wall

I can express the distance [math]A_1D_1[/math] as function of collimator size [math]\Theta_C/m[/math] and electron beam energy E: 

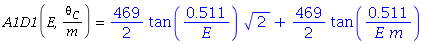
Formula cond11.png

To fit the collimator size into the hole through the concrete wall with radius R = 8.73 cm we need to solve equation: 

[math]A_1D_1(E,\ \Theta_C/m) = 8.73\ cm[/math]

1) some solutions of this equation for different collimator sizes m are: 

[math]m = 1 \Rightarrow E_{min} = 33.1\ MeV  [/math]

[math]m = 2 \Rightarrow E_{min} = 26.3\ MeV  [/math]

[math]m = 4 \Rightarrow E_{min} = 22.8\ MeV  [/math]

2) in general for arbitrary collimator size m the solutions are:

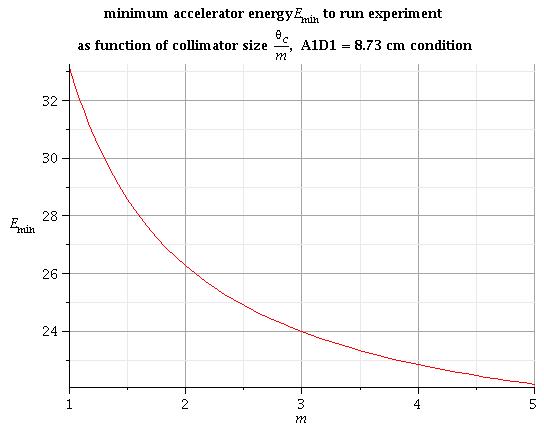
Energy condition1.jpeg

All energies above this line is good to run experiment for condition above

critical collimator line condition

Also I can express the distance [math]GH[/math] as function of collimator size [math]\Theta_C/m[/math] and electron beam energy E: 

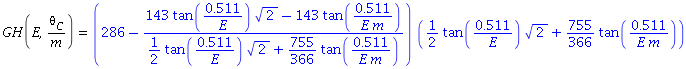
Formula cond21.png

If I would like that nothing hitting the 4" box to go through the collimator I need to solve equation: 

[math]GH(E,\ \Theta_C/m) = 5.08\ cm[/math]

1) some solutions of this equation for different collimator sizes m are: 

[math]m = 1 \Rightarrow E_{min} = 73.7\ MeV  [/math]

[math]m = 2 \Rightarrow E_{min} = 36.9\ MeV  [/math]

[math]m = 4 \Rightarrow E_{min} = 18.4\ MeV  [/math]

2) in general for arbitrary collimator size m the solutions are:

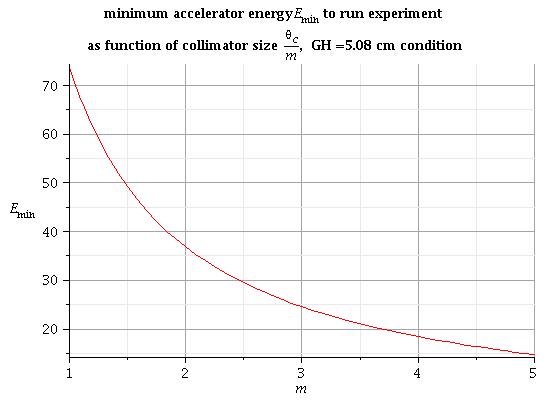
Energy condition2.jpeg

All energies above this line is good to run experiment for condition above

both solutions are together

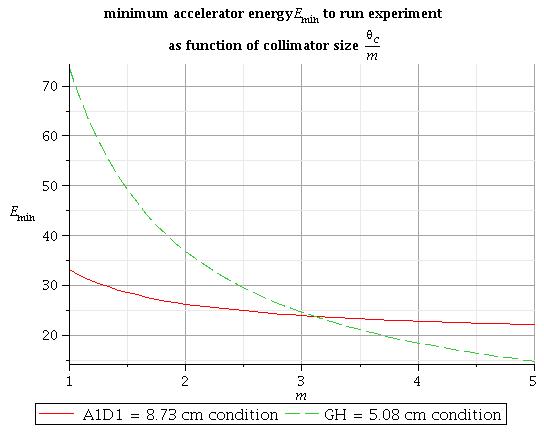
Energy condition12.jpeg

All energies above this lines is good to run experiment for both conditions above


Go Back

Retrieved from "https://wiki.iac.isu.edu/index.php?title=Minimum_accelerator_energy_to_run_experiment&oldid=74568"