Difference between revisions of "Anisotropic n's vs. isotropic ones"
| Line 74: | Line 74: | ||
[[File:U235.sigmaflux.01.png | 1000px]] | [[File:U235.sigmaflux.01.png | 1000px]] | ||
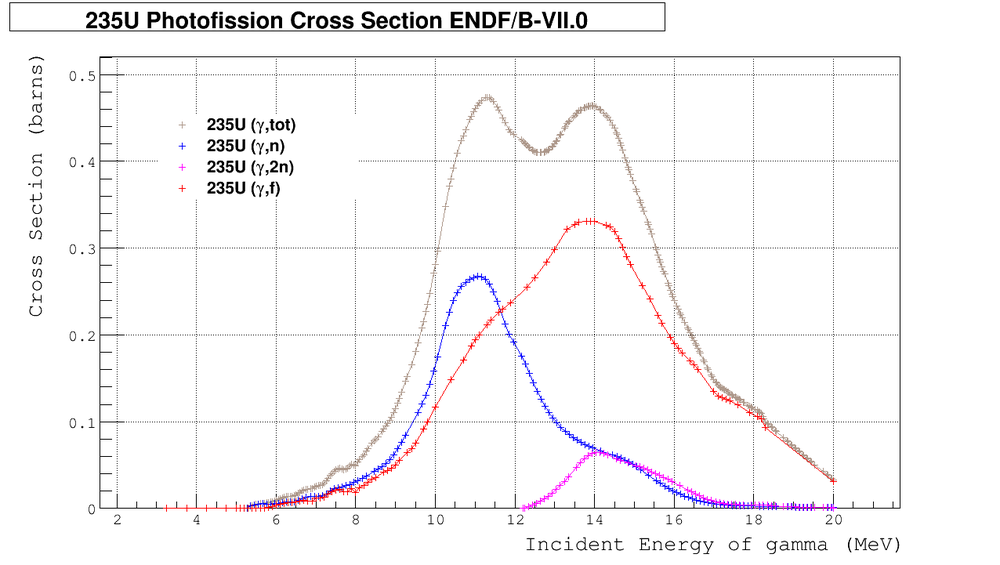
| − | From the plot above | + | From the plot above we can expect significant number of isotropic neutrons from (<math>\gamma</math>,n) and (<math>\gamma</math>,2n) channels. So the effect of washing out the anisotropy by isotropic neutrons should be |
==Let's count isotropic and anisotropic neutrons== | ==Let's count isotropic and anisotropic neutrons== | ||
Revision as of 01:29, 27 June 2011
Motivation: there are a lot of source of isotropic neutrons=
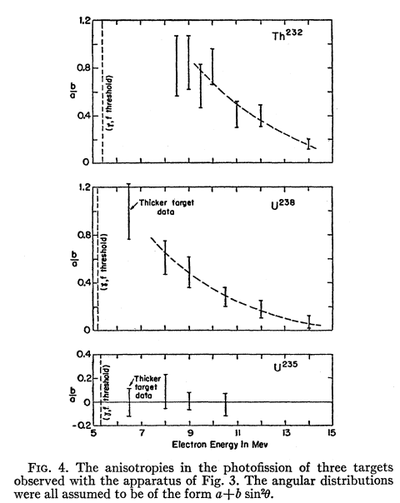
1. Winhold and Halpern, Phys.Rev. 103 4, 990 (1956).
- The observation were consistent with the assumption that
- anisotropic fission is due solely to photons with-in about
- 3 MeV of the fission threshold".
- The photons in the giant resonance region were found
- to produce essentially isotropic fission
2. A lot of other papers.
3. Isotropic neutrons from (,n) channel and (,2n) channel
Anisotropic n's vs. isotropic ones
Say, we have only anisotropic neutrons with 1.25 calculated ratio:
The calculated asymmetry would be:
Now, say, we have extra 100 isotropic neutrons for each detector:
The total number of neutrons become:
So the calculated asymmetry would be:
So if we would have about the same number of isotropic and anisotropic neutrons that would be reduced the expectation values of asymmetry almost in two times!!!.
We need to count isotropic and anisotropic neutrons to estimate the effect of washing out the anisotropy by isotropic neutrons.
Source of anisotropic neutrons:
- (,f) channel due to photons with-in about 3 MeV of the fission threshold
Source of isotropic neutrons:
- (,f) channel due to photons out of 3 MeV of the fission threshold
- (,n) channel.
- (,2n) channel.
U Photo-fission Cross Section
From the plot above we can expect significant number of isotropic neutrons from (,n) and (,2n) channels. So the effect of washing out the anisotropy by isotropic neutrons should be
Let's count isotropic and anisotropic neutrons
Assuming conservatively:
Source of anisotropic neutrons:
- (,f) channel for all incident gammas. In reality the number of anisotropic neutrons will reduce with increasing the energy of gammas.
Source of isotropic neutrons:
- (,n) channel
- (,2n) channel
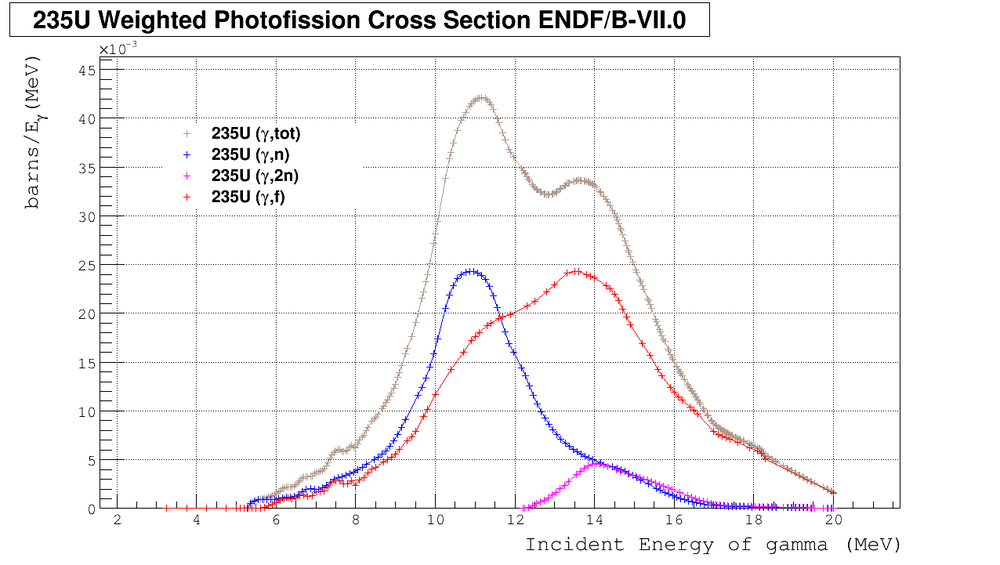
We can calculate the relative number of neutrons from different channels using formulas:
Using root to approximate sigma's by appropriate polynomials and using Maple to estimate integrals I found: