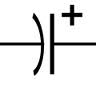Difference between revisions of "TF EIM Chapt2"
| Line 76: | Line 76: | ||
[[File:TF_EIM_AV_VoltageSymbol.png| 200 px]] | [[File:TF_EIM_AV_VoltageSymbol.png| 200 px]] | ||
| − | + | A mathematical form is for an AC voltage source is: | |
:<math>V = V_0 \sin(\omega t)</math> | :<math>V = V_0 \sin(\omega t)</math> | ||
Revision as of 20:53, 23 January 2011
Alternating Current (AC)
Thus far we have discussed direct current circuits which were built from a battery (constant voltage supply).
Another type of circuit is one in which the current is driven in an alternating fashion. Typically the current is increased so some positive maximum value, then decreased until it passes through zero and reverses direction until it reaches another maximum value and then it is decreased again, passing though zero once more on its way to a positive maximum value.
Definitions
- frequency (, )
- The frequency is number of complete cycles which occur in 1 sec (cycles/sec = Hz)
- Angular frequency = = radians/sec
- Period
- The period is the time to complete one cycle = 1/
- Amplitude
- The change in the current from zero to its most positive value
- peak-to-peak
- The amount the current changes from its largest positive value to its most negative.
- phase
- The time a current takes to reaches its maximum value compared to another alternating current
- Note
- The above definitions can be used for voltage as easily as current.
Mathematical description
Trigonometric
- Note
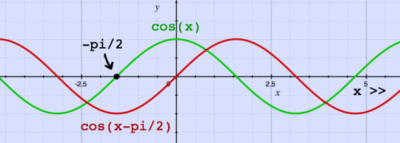
- A 180 degree ( radian) phase shift will put the above cosine wave completely out of sync with the original one such that if we add the two signals together the net current would be zero.
I_{RMS}
With this functional form for the alternating current we can now calculate another property, the RMS or root mean square.
The RMS quantifies an average fluctuation of the alternating current such that
where T represent the time interval over which the average is calculated ()
- if T is infinite or an integer number of cycles
Power
The instantaneous power dissipated in a resister by an alternating current is given as
Average power
Plane waves
Another mathematical expression for an alternating current uses complex variables
Expressing the current in this form will be useful later
Voltage sources of AC current
The circuit symbol for an emf used to drive alternating currents is given below
A mathematical form is for an AC voltage source is:
Capacitors
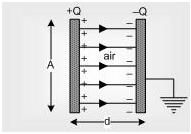
A capacitor is a device used to store charge in a circuit. The simplest capacitor is built from two conducting plates placed close to each other.
- Noteice
- The potential is constant over the face of the capacitor
The symbol for a capacitor in a circuit is usually.
or
dielectric symbol
dielectric capacitors can be shorted out. Put the positive probe from the voltmeter on the positive side of the electrolytic capacitor, the capacitor should be good if you measure a large resistance otherwise a shorted electrolytic capacitor (small resistance) is a bad one.
Capacitor Properties
- Measures ability to store charge
- : Double Q Doubles V
- SI units = Farrads = Coul/Volt
- Magnitude depends on the elements geometry (conductor area, separation of conductors, ...)
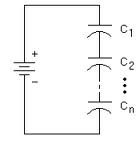
Capacitors in Parallel and Series
Series
Capacitors in Series add like resistors in Parallel.(Capacitance decreases when hooked up in series)
- Note
- The same amount of charge is pushed onto each capacitor
For capacitors in series you can apply the loop theorem for the above case and the one where all the capacitors are replaced by a single capacitor.
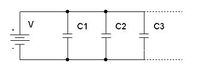
Parallel
Capacitors in parallel add like resistors in Series (Capacitance increases when hooked up in parallel)
If you were to replace all capacitors with an equivalent capacitance (C_{eq}) then the charge driven onto the equivalent capacitance would be equal to the sum of the charge driven onto each capacitor.
- ...
- ...
- ...
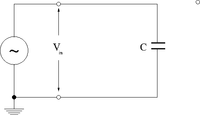
Charging/Discharging Capacitor circuit
If
then applying loop theorem lead to
- Notice that the current is 90 degrees out of phase with the driving voltage.
- Note
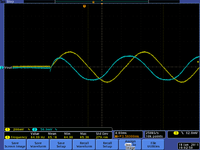
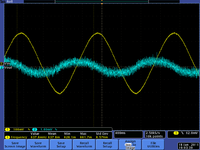
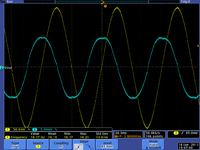
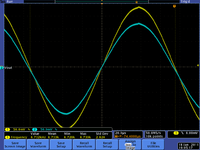
- The current increases faster than the voltage. Below are a few scope pictures measuring the voltage coming out of the voltage source and the voltage on the ground side of the capacitor (the capacitor is grounded through the scope). This is actually a high pass filter because of the internal resistance of the scope used to measure .
| 1 Hz | 16Hz | kHz |
Reactance: Resistive nature of capacitance
To illustrate the resistive nature of capacitors, let's write the driving voltage in using complex notation
The notation
- : Euler's equation
- The real part of a complex variable.
The current is written as
where
- Effective impedance of a Capacitor
- Observe
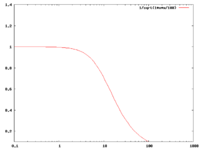
- At high frequencies the effective impedance (X_C) is small so the driving voltage should pass right through.
- At low frequencies the effective impedance (X_C) is large so the driving voltage will be severely attenuated.
- Capacitors block Alternating Currents so you can reduce ripples on DC voltages.
- You could measure the capacitance by measuring for several frequencies
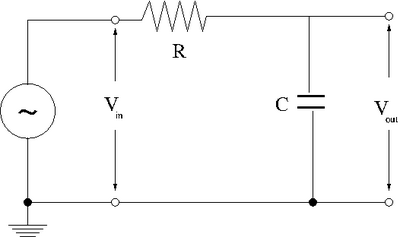
RC circuit
Now add a resistor in series with the capacitor in the above capacitor charging circuit.
Quick Solution
Applying the loop theorem using the reactance of the capacitor instead of Q/C
- the capacitor can be added in series with the other resistor
It looks like the voltage divider from the resistance section
To evaluate where and
Let
- break point (cut off ) frequency
then
- Note
- The RC circuit is another way to measure the capacitance by measureing -vs- and do a linear extrapolation to determine the break point frequency.
Phasor Diagram
Phasor diagrams are a technique used to illustrate the phase difference between the input and output voltages.
Returning to the loop theorem for the low pass RC filter
We can write the voltage as a complex variable using a coordinate system in which the "x-axis" represents real numbers and the "y-axis" represents the complex components. This is analogous to expressing 2-D vectors.
Below is a figure representing the complex plane.
exact solution
Again apply the loop theorem
or
- First order non-homogeneous linear differential equation
First solve homogeneous part
- First order homogeneous linear differential equation
The above is integratable
This gives you the general solution for the homogeneous problem
In this case it represents the solution for a discharging capacitor.
Trial solution method for Non-Homogeneous Solution
A common and more general technique to solving such a differential equation is to use the solution from the discharging equation (the homogeneous diff. eq.) as a trial solution by inserting constants.
You then substitute this trial solution into the differential equation and apply boundary conditions to determine the coefficients.
To determine the other coefficient you apply the boundary condition that q = 0 when t=0