Difference between revisions of "Geometry (25 MeV LINAC exit port)"
Jump to navigation
Jump to search
| Line 7: | Line 7: | ||
The minimum energy of accelerator (MeV) is limited by fitting the collimator (<math>r_2</math>) into the hole (<math>R</math>) | The minimum energy of accelerator (MeV) is limited by fitting the collimator (<math>r_2</math>) into the hole (<math>R</math>) | ||
| − | Assuming the collimator | + | Assuming the collimator diameter is <math>\Theta_C/2</math> |
| − | <math>x_2 + r_2 = R</math><br> | + | <math>x_2 + r_2 = R \Rightarow</math><br> |
<math>\frac{1}{\sqrt{2}}\ (286+183)\ \tan\left(\frac{0.511}{E}\right) + | <math>\frac{1}{\sqrt{2}}\ (286+183)\ \tan\left(\frac{0.511}{E}\right) + | ||
\frac{1}{2}\ (286+183)\ \tan\left(\frac{1}{2}\ \frac{0.511}{E}\right) = R </math> | \frac{1}{2}\ (286+183)\ \tan\left(\frac{1}{2}\ \frac{0.511}{E}\right) = R </math> | ||
Revision as of 03:06, 13 June 2010
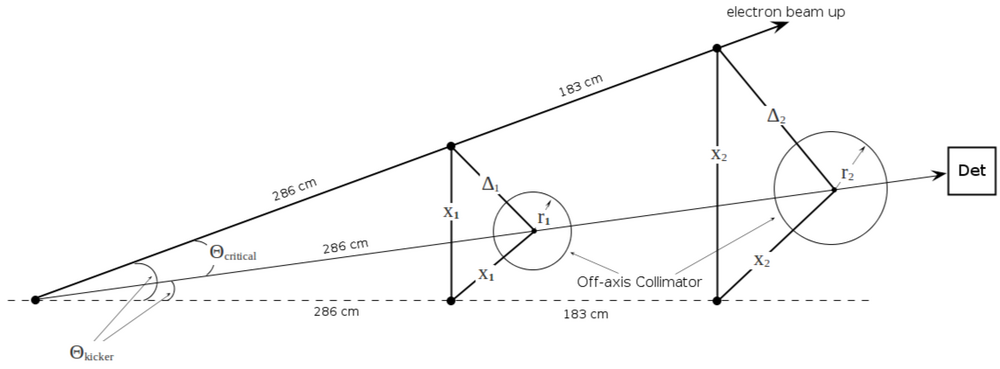
Minimum accelerator energy to run experiment
The minimum energy of accelerator (MeV) is limited by fitting the collimator () into the hole ()
Assuming the collimator diameter is
25 MeV geometry
critical angle
kicker angle
geometry ()
collimator center position
(wall 1)
(wall 2)
collimator diameter
(wall 1)
(wall 2)
collimator critical angle
:
minimal distance from the wall ()
geometry ()
collimator center position
(wall 1)
(wall 2)
collimator diameter
(wall 1)
(wall 2)
collimator critical angle
:
minimal distance from the wall ()
Funny pictures...
how it looks 1 (, box 3"x4" and then pipe 4")
File:Vacuum pipe collimator .png
how it looks 2 (, box 3"x4" and then pipe 4")
File:Vacuum pipe collimator .png