Difference between revisions of "Conversion TDC to Energy"

From New IAC Wiki
Jump to navigation Jump to search
 
(118 intermediate revisions by the same user not shown)
Line 1: Line 1:
−
=Calculation=
+
[http://wiki.iac.isu.edu/index.php/PhotoFission_with_Polarized_Photons_from_HRRL Go Back]
  
−
t<sub>n</sub>: Neutron time of flight
 
  
−
t<sub>ɣ</sub>: Gamma time of flight
+
= Method 1:  =
 +
 
 +
==Calculation==
 +
 
 +
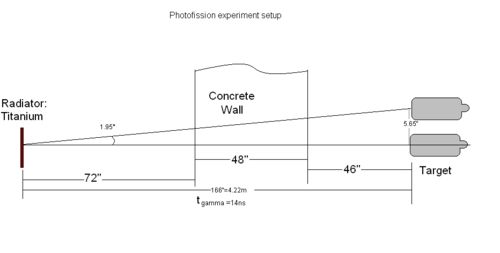
<font size="4">When electron beam hit the radiator, TDC start to count. It will take gamma 14 ns to reach the target. Then after some time <math>t_{n1}</math>, neutron arrive to the detectors.
 +
 
 +
Let's call the the total time from electron beam hit the radiator to neutron to be detected is <math>t_n</math>. So,
 +
 
 +
<math>t_n = t_{n1} + 14
 +
 
 +
            = \frac{d}{v} + 14    ns</math>
 +
 
 +
where d is the distance between detector and target, v is speed of neutron. So,
 +
 
 +
<math>v=\frac{d}{(t-14)*10^{-9}} m/s </math>
 +
 
 +
where should be in the unit of nano second.
 +
 
 +
[[Image:Setup.jpg|500px|right]]
 +
 
 +
 
 +
 
 +
 
 +
<math>\beta_n=\frac{v}{c}</math>
 +
 
 +
<math>\gamma_n=\sqrt{\frac{1}{1-\beta^2}}</math>
 +
 
 +
<math>E_{tot}=M_n\gamma_nc^2</math>
 +
 
 +
<math>E_k=E_{tot}-M_n c^2</math>
 +
 
 +
==Data==
 +
 
 +
For data go to link:
 +
 
 +
http://inca.iac.isu.edu/~setiniyaz/photofis/Method1/
 +
 
 +
or click on:
 +
 
 +
[[Image:method 1 polarized.pdf]]
 +
 
 +
[[Image:method 1 unpolarized top.pdf]]
 +
 
 +
[[Image:method 1 unpolarized side.pdf]]
 +
 
 +
= Method 2: Using time difference in two peaks =
 +
 
 +
==Calculation==
 +
 
 +
If we assume gamma flash is coming from target, then by time difference in gamma peak and neutron peak, we can tell the energies in neutrons.
 +
 
 +
<math>t_n</math>: Neutron time of flight
 +
 
 +
<math>t_{\gamma}</math>: Gamma time of flight
  
 
d: Distance between detector and target.  
 
d: Distance between detector and target.  
  
−
d for polarized case: d<sub>pol</sub> = 91.25 inches = 231.775 cm.
+
d for polarized case: <math>d_{pol}</math> = 91.25 inches = 231.775 cm.
  
−
d for unpolarized top detector: d<sub>unpol_top</sub>=96.25 inches = 244.745 cm.
+
d for unpolarized top detector: <math>d_{unpol top}</math>=96.25 inches = 244.745 cm.
  
−
d for unpolarized side detector: d<sub>unpol_side</sub>=91.39 inches = 232.131 cm.
+
d for unpolarized side detector: <math>d_{unpol side}</math>=91.39 inches = 232.131 cm.
  
  
  
−
t<sub>n</sub>-t<sub>ɣ</sub>=d/v-d/c=d(1/v-1/c)
+
<math>t_n-t_{\gamma}=\frac{d}{v}-\frac{d}{c}=d(\frac{1}{v}-\frac{1}{c}</math>)
  
−
=> <math>v=\frac{1}{\frac{1}{c}}</math>
+
=> <math>v=\frac{1}{\frac{t_n-t_{\gamma}}{d}+\frac{1}{c}}</math>
  
  
Line 24: Line 76:
 
<math>\gamma_n=\sqrt{\frac{1}{1-\beta^2}}</math>
 
<math>\gamma_n=\sqrt{\frac{1}{1-\beta^2}}</math>
  
−
E<sub>tot</sub>=M<sub>n</sub>ɣ<sub>n</sub>c<sup>2</sup>
+
<font size="4"><math>E_{tot}=M_n\gamma_nc^2</math>
  
−
E<sub>k</sub>=E<sub>tot</sub>-M<sub>n</sub>c<sup>2</sup>
+
<font size="4"><math>E_k</math>=<math>E_{tot}-M_n c^2</math>
  
−
=Data=
+
=<font size="4"><math>M_n c^2</math>{1- <math>\sqrt{\frac{1}{1-(\frac{1}{1+\frac{c}{d}(t_n-t_{\gamma})})^2}}</math>}
 +
 
 +
=939.5656*{1- <math>\sqrt{\frac{1}{1-(\frac{1}{1+\frac{c}{d}(t_n-t_{\gamma})})^2}}</math>}  (MeV)
 +
 
 +
Where d is geven above.
 +
 
 +
==Data==
  
 
For data go to Link below:
 
For data go to Link below:
  
−
[http://inca.iac.isu.edu/~setiniyaz/photofis/TDCtoMeV/]
+
http://inca.iac.isu.edu/~setiniyaz/photofis/TDCtoMeV/
 +
 
 +
or click on:
 +
 
 +
[[Image:method 2 polarized.pdf]]
 +
 
 +
[[Image:method 2 unpolarized top.pdf]]
 +
 
 +
[[Image:method 2 unpolarized side.pdf]]
 +
 
 +
===Energy vs Channel===
 +
 
 +
We calibrated ADC [[http://www.iac.isu.edu/mediawiki/index.php/TDC_Calibration]] and using those data we can tell energy of neutron by its relative channel number difference from gamma peak.
 +
 
 +
 
 +
There a two scale for ADC. One is 2000 ns, the other one is 5000ns.
 +
 
 +
For 2000 ns scale:
 +
 
 +
[[Image:method 2 polarized 2000 channels.pdf]]
 +
 
 +
 
 +
For 5000 ns scale:
 +
 
 +
[[Image:method 2 polarized 5000 channels.pdf]]
 +
 
 +
==Example Analysis==
 +
 
 +
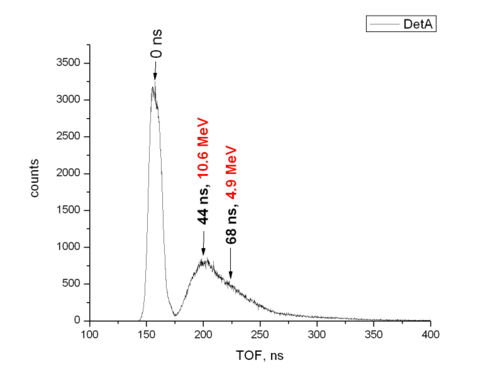
[[Image:Energy_time.jpg | 500px]]
 +
 
 +
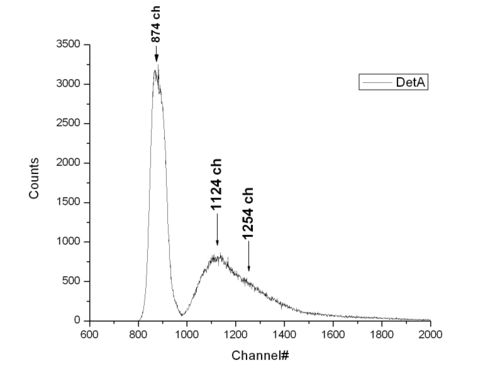
[[Image:Detector_A_CHannels.jpg |500px]]
 +
 
 +
Channel difference in two peaks is about 260 channels. This corresponds to 47 ns, which is 9.5 MeV neutron kinetic energy.
 +
 
 +
4.9 MeV neutrons come 68 ns later, which corresponds to 380 channels difference.
 +
 
 +
[http://wiki.iac.isu.edu/index.php/PhotoFission_with_Polarized_Photons_from_HRRL go back]

Latest revision as of 06:18, 5 February 2009

Go Back


Method 1:

Calculation

When electron beam hit the radiator, TDC start to count. It will take gamma 14 ns to reach the target. Then after some time [math]t_{n1}[/math], neutron arrive to the detectors.

Let's call the the total time from electron beam hit the radiator to neutron to be detected is [math]t_n[/math]. So,

[math]t_n = t_{n1} + 14 = \frac{d}{v} + 14 ns[/math]

where d is the distance between detector and target, v is speed of neutron. So,

[math]v=\frac{d}{(t-14)*10^{-9}} m/s [/math]

where should be in the unit of nano second.

Setup.jpg



[math]\beta_n=\frac{v}{c}[/math]

[math]\gamma_n=\sqrt{\frac{1}{1-\beta^2}}[/math]

[math]E_{tot}=M_n\gamma_nc^2[/math]

[math]E_k=E_{tot}-M_n c^2[/math]

Data

For data go to link:

http://inca.iac.isu.edu/~setiniyaz/photofis/Method1/

or click on:

File:Method 1 polarized.pdf

File:Method 1 unpolarized top.pdf

File:Method 1 unpolarized side.pdf

Method 2: Using time difference in two peaks

Calculation

If we assume gamma flash is coming from target, then by time difference in gamma peak and neutron peak, we can tell the energies in neutrons.

[math]t_n[/math]: Neutron time of flight

[math]t_{\gamma}[/math]: Gamma time of flight

d: Distance between detector and target.

d for polarized case: [math]d_{pol}[/math] = 91.25 inches = 231.775 cm.

d for unpolarized top detector: [math]d_{unpol top}[/math]=96.25 inches = 244.745 cm.

d for unpolarized side detector: [math]d_{unpol side}[/math]=91.39 inches = 232.131 cm.


[math]t_n-t_{\gamma}=\frac{d}{v}-\frac{d}{c}=d(\frac{1}{v}-\frac{1}{c}[/math])

=> [math]v=\frac{1}{\frac{t_n-t_{\gamma}}{d}+\frac{1}{c}}[/math]


[math]\beta_n=\frac{v}{c}[/math]

[math]\gamma_n=\sqrt{\frac{1}{1-\beta^2}}[/math]

[math]E_{tot}=M_n\gamma_nc^2[/math]

[math]E_k[/math]=[math]E_{tot}-M_n c^2[/math]

=[math]M_n c^2[/math]{1- [math]\sqrt{\frac{1}{1-(\frac{1}{1+\frac{c}{d}(t_n-t_{\gamma})})^2}}[/math]}

=939.5656*{1- [math]\sqrt{\frac{1}{1-(\frac{1}{1+\frac{c}{d}(t_n-t_{\gamma})})^2}}[/math]} (MeV)

Where d is geven above.

Data

For data go to Link below:

http://inca.iac.isu.edu/~setiniyaz/photofis/TDCtoMeV/

or click on:

File:Method 2 polarized.pdf

File:Method 2 unpolarized top.pdf

File:Method 2 unpolarized side.pdf

Energy vs Channel

We calibrated ADC [[1]] and using those data we can tell energy of neutron by its relative channel number difference from gamma peak.


There a two scale for ADC. One is 2000 ns, the other one is 5000ns.

For 2000 ns scale:

File:Method 2 polarized 2000 channels.pdf


For 5000 ns scale:

File:Method 2 polarized 5000 channels.pdf

Example Analysis

Energy time.jpg

Detector A CHannels.jpg

Channel difference in two peaks is about 260 channels. This corresponds to 47 ns, which is 9.5 MeV neutron kinetic energy.

4.9 MeV neutrons come 68 ns later, which corresponds to 380 channels difference.

go back

Retrieved from "https://wiki.iac.isu.edu/index.php?title=Conversion_TDC_to_Energy&oldid=37207"