Difference between revisions of "LB Thesis SNR"
Jump to navigation
Jump to search
| Line 8: | Line 8: | ||
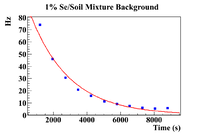
The clearest exponential behavior is seen in the 1% sample. The background rate dropped by 62% between the first and second measurements (<math> \Delta t </math> = 7.5 minutes). This indicated that considering the background to be constant from <math> t_{Beam Off} </math> until <math> t_{Measure} </math>, which was an average of 23.3 <math> \pm </math> 2.67 minutes would not be sufficient. Since this was the case, an exponential fit was attempted on the 1% mixture data to find the half life of the background (even though this is still just an estimate). | The clearest exponential behavior is seen in the 1% sample. The background rate dropped by 62% between the first and second measurements (<math> \Delta t </math> = 7.5 minutes). This indicated that considering the background to be constant from <math> t_{Beam Off} </math> until <math> t_{Measure} </math>, which was an average of 23.3 <math> \pm </math> 2.67 minutes would not be sufficient. Since this was the case, an exponential fit was attempted on the 1% mixture data to find the half life of the background (even though this is still just an estimate). | ||
| + | |||
| + | [[File:LB 170047 Background HLPlot Expo.png|200px]] | ||
| + | [[File:LB 170048 Background HLPlot Expo.png|200px]] | ||
[[File:LB 170049 Background HLPlot Expo.png|200px]] | [[File:LB 170049 Background HLPlot Expo.png|200px]] | ||
Revision as of 23:23, 24 June 2018
Background Analysis
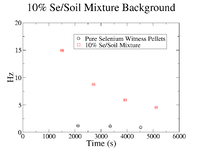
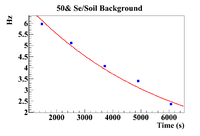
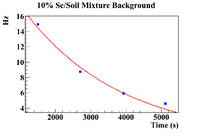
In order to find the SNR at , the background was analyzed to check if it remained constant from until . First, the background was plotted for each concentration of Se/Soil and was overlaid with the the background in the pure sample for each respective target.
The clearest exponential behavior is seen in the 1% sample. The background rate dropped by 62% between the first and second measurements ( = 7.5 minutes). This indicated that considering the background to be constant from until , which was an average of 23.3 2.67 minutes would not be sufficient. Since this was the case, an exponential fit was attempted on the 1% mixture data to find the half life of the background (even though this is still just an estimate).