Difference between revisions of "Relativistic Frames of Reference"
(Created page with "From the Galilean description of motion for a frame of reference moving relative to another frame considered stationary we know that <center><math>t=t'</math></center> <center><…") |
|||
| (76 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
| + | <center><math> \underline{\textbf{Navigation} }</math> | ||
| + | |||
| + | [[Uniform_distribution_in_Energy_and_Theta_LUND_files|<math>\vartriangleleft </math>]] | ||
| + | [[VanWasshenova_Thesis#Moller_Scattering|<math>\triangle </math>]] | ||
| + | [[Relativistic_Units|<math>\vartriangleright </math>]] | ||
| + | |||
| + | </center> | ||
| + | |||
| + | =Relativistic Frames of Reference= | ||
| + | |||
| + | |||
From the Galilean description of motion for a frame of reference moving relative to another frame considered stationary we know that | From the Galilean description of motion for a frame of reference moving relative to another frame considered stationary we know that | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | <center>[[File:GalileanFrames.png]]</center> | + | |
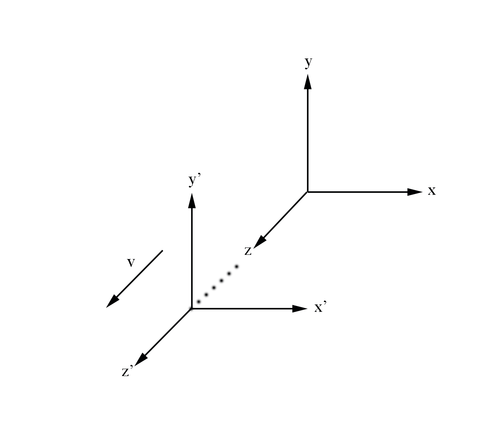
| + | <center>[[File:GalileanFrames.png|thumb|center|500px|alt=Galilean Frames of Reference|'''Figure 2.1:''' Primed reference frame moving in the z direction with velocity v. ]]</center> | ||
| + | |||
| + | |||
| + | In the rest frame of v=0 | ||
| + | |||
| + | <center><math>v=0 \Rightarrow \begin{cases} | ||
| + | t= t' \\ | ||
| + | |||
| + | x=x' \\ | ||
| + | |||
| + | y=y' \\ | ||
| + | |||
| + | z=z'+vt' | ||
| + | \end{cases}</math></center> | ||
| + | |||
| + | While conversely, from the rest frame of v'=0 | ||
| + | |||
| + | <center><math>v'=0 \Rightarrow \begin{cases} | ||
| + | t'= t \\ | ||
| + | |||
| + | x'=x \\ | ||
| + | |||
| + | y'=y \\ | ||
| + | |||
| + | z'=z-vt | ||
| + | \end{cases}</math></center> | ||
| + | |||
| + | |||
| + | |||
| + | |||
| + | |||
| + | Using Einstein's Theory of Relativity, we know that the speed of light is a constant, c, for all reference frames. In the unprimed frame, from the definition of speed: | ||
| + | |||
| + | |||
| + | <center><math>speed=\frac{\Delta Distance}{\Delta Time}</math></center> | ||
| + | |||
| + | |||
| + | <center><math>c=\frac{\Delta d}{\Delta t}</math></center> | ||
| + | |||
| + | |||
| + | where | ||
| + | |||
| + | <center><math>c=3\times 10^8\ m/s</math></center> | ||
| + | |||
| + | Using the distance equation in a Cartesian coordinate system, the equation for the speed of light becomes | ||
| + | |||
| + | |||
| + | <center><math>c=\frac{\sqrt{\Delta x^2+\Delta y^2+\Delta z^2}}{\Delta t}</math></center> | ||
| + | |||
| + | |||
| + | Following the postulate of Special Relativity, this implies for the primed frame | ||
| + | |||
| + | |||
| + | <center><math>c=\frac{\sqrt{\Delta x^{'2}+\Delta y^{'2}+\Delta z^{'2}}}{\Delta t}</math></center> | ||
| + | |||
| + | |||
| + | |||
| + | |||
| + | We can rewrite this as | ||
| + | |||
| + | |||
| + | |||
| + | <center><math>\frac{\Delta x^{'2}+\Delta y^{'2}+\Delta z^{'2}}{\Delta t^{'2}}= c^2=\frac{\Delta x^2+\Delta y^2+\Delta z^2}{\Delta t^2}</math></center> | ||
| + | |||
| + | |||
| + | This is possible since the ratios of distance to time are multiples of the same base, i.e. the square of the speed of light <math>(\frac{3\times 10^8\ m}{s})^2</math>. Therefore for the relative change in the time in one frame, the distance must change by the same factor to maintain the same constant. With this we can write | ||
| + | |||
| + | |||
| + | <center><math>c^2 \Delta t^{'2}=\Delta x^{'2}+\Delta y^{'2}+\Delta z^{'2}\ \ \ \ \ c^2 \Delta t^{2}=\Delta x^2+\Delta y^2+\Delta z^2</math></center> | ||
| + | |||
| + | |||
| + | |||
| + | |||
| + | <center><math>\Rightarrow c^2 \Delta t^{'2}-\Delta x^{'2}-\Delta y^{'2}-\Delta z^{'2}= c^2 \Delta t^{2}-\Delta x^2-\Delta y^2-\Delta z^2</math></center> | ||
| + | |||
| + | |||
| + | |||
| + | This quantity is known as the time space interval <math>ds^2</math> when the change is infinitesimal | ||
| + | |||
| + | |||
| + | <center><math>ds^2\equiv c^2 dt^{'2}-dx^{'2}-dy^{'2}-dz^{'2}= c^2 dt^{2}-dx^2-dy^2-dz^2</math></center> | ||
| + | |||
| + | |||
| + | Since the speed of light is a constant for all frames of reference, this allows the space time interval to also be invariant for inertial frames. | ||
| + | |||
| + | <center><math>ds^2\equiv c^2 dt^{'2}-dx^{'2}-dy^{'2}-dz^{'2}= c^2 dt^{2}-dx^2-dy^2-dz^2</math></center> | ||
| + | |||
| + | |||
| + | <center><math>ds^2\equiv c^2 dt^{'2}-dr^{'2}= c^2 dt^{2}-dr^2</math></center> | ||
| + | |||
| + | |||
| + | <center><math>ds^2\equiv (c^2 -v^{'2})dt^{'2}= (c^2 -v^2)dt^{2}</math></center> | ||
| + | |||
| + | |||
| + | From the rest frame of v'=0 | ||
| + | |||
| + | |||
| + | <center><math>ds^2\equiv c^2 dt^{'2}= (c^2 -v^2)dt^{2}</math></center> | ||
| + | |||
| + | |||
| + | <center><math>\Rightarrow dt^{'2}= (1-\frac{v^2}{c^2 })dt^{2}</math></center> | ||
| + | |||
| + | |||
| + | |||
| + | <center><math>\Rightarrow \begin{cases} | ||
| + | dt'= \sqrt{1-\frac{v^2}{c^2 }}dt=\frac{1}{\gamma} dt\\ | ||
| + | \\ | ||
| + | dt= \frac{1}{\sqrt{1-\frac{v^2}{c^2 }}}dt'=\gamma dt' | ||
| + | \end{cases}</math></center> | ||
| + | |||
| + | |||
| + | <center><math>\Rightarrow \begin{cases} | ||
| + | t'=\frac{1}{\gamma} t\\ | ||
| + | \\ | ||
| + | t=\gamma t' | ||
| + | \end{cases}</math></center> | ||
| + | |||
| + | |||
| + | |||
| + | Assuming motion is only along the z direction | ||
| + | |||
| + | |||
| + | <center><math>z \equiv ct\ \ \ \ z'\equiv ct'</math></center> | ||
| + | |||
| + | |||
| + | |||
| + | |||
| + | Substituting these changes into the Galilean transformations | ||
| + | |||
| + | |||
| + | <center><math>\underline{\textbf{Galilean\ Transformations}}\quad \underline{\textbf{Lorentz\ Transformations}}</math></center> | ||
| + | |||
| + | <center><math> | ||
| + | v=0\Rightarrow | ||
| + | \begin{cases} | ||
| + | t= t' \\ | ||
| + | |||
| + | x=x' \\ | ||
| + | |||
| + | y=y' \\ | ||
| + | |||
| + | z=z'+vt' | ||
| + | \end{cases} | ||
| + | \qquad \Rightarrow \qquad \quad | ||
| + | \begin{cases} | ||
| + | t=\gamma (t'+vz'/c^2) \\ | ||
| + | |||
| + | x=x' \\ | ||
| + | |||
| + | y=y' \\ | ||
| + | |||
| + | z=\gamma (z'+vt') | ||
| + | \end{cases} | ||
| + | </math></center> | ||
| + | |||
| + | |||
| + | <center><math>v'=0\Rightarrow | ||
| + | \begin{cases} | ||
| + | t'= t' \\ | ||
| + | |||
| + | x'=x' \\ | ||
| + | |||
| + | y'=y' \\ | ||
| + | |||
| + | z'=z-vt | ||
| + | \end{cases} | ||
| + | \qquad \Rightarrow \qquad \quad | ||
| + | \begin{cases} | ||
| + | t'=\gamma (t-vz/c^2) \\ | ||
| + | |||
| + | x'=x' \\ | ||
| + | |||
| + | y'=y' \\ | ||
| + | |||
| + | z'=\gamma (z-vt) | ||
| + | \end{cases} | ||
| + | </math></center> | ||
| + | ---- | ||
| + | |||
| + | |||
| + | |||
| + | <center><math> \underline{\textbf{Navigation} }</math> | ||
| + | |||
| + | [[Uniform_distribution_in_Energy_and_Theta_LUND_files|<math>\vartriangleleft </math>]] | ||
| + | [[VanWasshenova_Thesis#Moller_Scattering|<math>\triangle </math>]] | ||
| + | [[Relativistic_Units|<math>\vartriangleright </math>]] | ||
| + | |||
| + | </center> | ||
Latest revision as of 18:45, 15 May 2018
Relativistic Frames of Reference
From the Galilean description of motion for a frame of reference moving relative to another frame considered stationary we know that
In the rest frame of v=0
While conversely, from the rest frame of v'=0
Using Einstein's Theory of Relativity, we know that the speed of light is a constant, c, for all reference frames. In the unprimed frame, from the definition of speed:
where
Using the distance equation in a Cartesian coordinate system, the equation for the speed of light becomes
Following the postulate of Special Relativity, this implies for the primed frame
We can rewrite this as
This is possible since the ratios of distance to time are multiples of the same base, i.e. the square of the speed of light . Therefore for the relative change in the time in one frame, the distance must change by the same factor to maintain the same constant. With this we can write
This quantity is known as the time space interval when the change is infinitesimal
Since the speed of light is a constant for all frames of reference, this allows the space time interval to also be invariant for inertial frames.
From the rest frame of v'=0
Assuming motion is only along the z direction
Substituting these changes into the Galilean transformations