Difference between revisions of "LB Thesis Thin Window Analysis"
| Line 3: | Line 3: | ||
==Thin Window Histograms== | ==Thin Window Histograms== | ||
| − | Since the resolution of Detector A at the IAC is about 1 keV, the window in which the signal is viewed should have a width of 2 (whether you plot channel number or energy along the horizontal axis) | + | Since the resolution of Detector A at the IAC is about 1 keV, the window in which the signal is viewed should have a width of 2 (whether you plot channel number or energy along the horizontal axis). The signals were fit with a function of the form |
<math> f(x) = C + Ae^{\frac{(x-\overline{x})^2}{2 \sigma^2}} </math> | <math> f(x) = C + Ae^{\frac{(x-\overline{x})^2}{2 \sigma^2}} </math> | ||
| − | + | ||
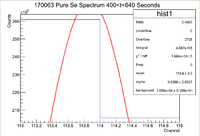
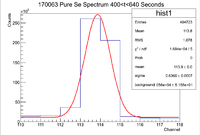
| + | Here, the constant value is to assess the value for the background. The background must be removed from the signal in order to have a more accurate analysis as it is not a part of the signal that we are interested in. Below are sample plots of the original signal and the signal viewed in a thin 2 channel window: | ||
[[File:170063 PureSe ThinWindow 400 640Sec.png|200px]] | [[File:170063 PureSe ThinWindow 400 640Sec.png|200px]] | ||
| + | |||
| + | |||
| + | [[File:170063 PureSe 400 640Sec OGWindow.png|200px]] | ||
This signal corresponds to the 103 keV line of Se-81. I plotted channel number instead of energy to maintain integer bins and avoid a picket fencing effect in the signal. | This signal corresponds to the 103 keV line of Se-81. I plotted channel number instead of energy to maintain integer bins and avoid a picket fencing effect in the signal. | ||
| + | |||
| + | In looking at the thin window, the total number of counts within the window is given by the "Integral" value in the statistics box, which I will denote as I. | ||
| + | |||
| + | <math> Stats Box Integral = I </math> | ||
Revision as of 16:19, 29 November 2017
This will be a detailed explanation of how to trace the isotope of interest's activity back to its original value. The sample of interest here is the oven ash sample with inventory number 170063.
Thin Window Histograms
Since the resolution of Detector A at the IAC is about 1 keV, the window in which the signal is viewed should have a width of 2 (whether you plot channel number or energy along the horizontal axis). The signals were fit with a function of the form
Here, the constant value is to assess the value for the background. The background must be removed from the signal in order to have a more accurate analysis as it is not a part of the signal that we are interested in. Below are sample plots of the original signal and the signal viewed in a thin 2 channel window:
This signal corresponds to the 103 keV line of Se-81. I plotted channel number instead of energy to maintain integer bins and avoid a picket fencing effect in the signal.
In looking at the thin window, the total number of counts within the window is given by the "Integral" value in the statistics box, which I will denote as I.