Difference between revisions of "GEANT Moller Simulations Comparison"
Jump to navigation
Jump to search
(Created page with "https://wiki.iac.isu.edu/index.php/Converting_to_barns https://wiki.iac.isu.edu/index.php/Check_Differential_Cross-Section Converting the number of electrons scattered per angl…") |
|||
| Line 59: | Line 59: | ||
<center><math>t=1.3\times 10^{-5}\ s</math></center> | <center><math>t=1.3\times 10^{-5}\ s</math></center> | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
Revision as of 20:28, 30 December 2016
https://wiki.iac.isu.edu/index.php/Converting_to_barns
https://wiki.iac.isu.edu/index.php/Check_Differential_Cross-Section
Converting the number of electrons scattered per angle theta to barns, we can use the relation
If the time is taken to be the same for the amount scattered as for the amount incident (the time simulated), this can be viewed as the probability of one incident electron producing a Moller event.
While this expression has no explicit dependancies on energy, the ratio is a function of the energy, as well as the physical makeup of the target.
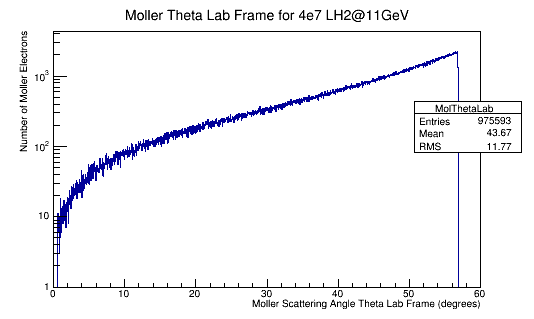
This gives, for LH2:
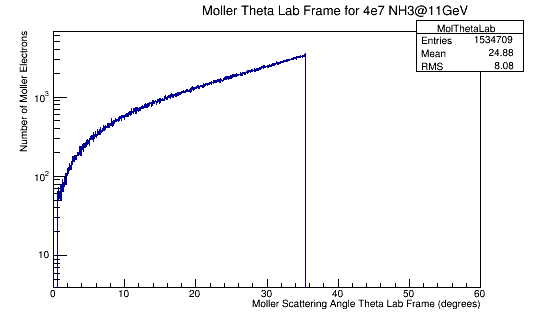
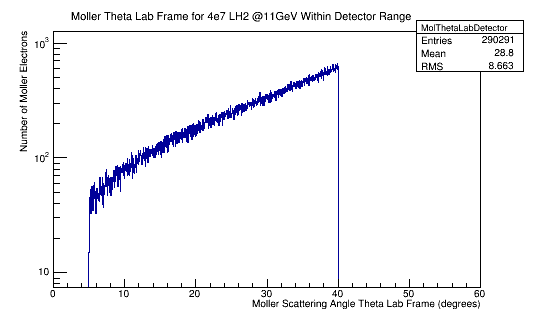
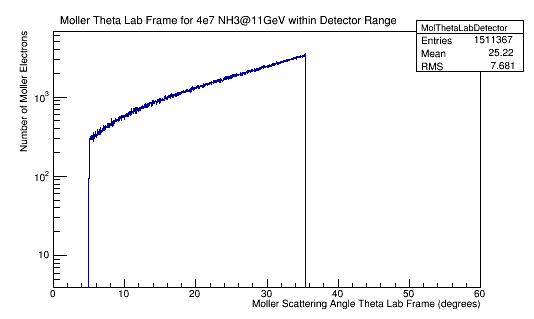
From earlier simulations for random angle Phi, we know that the full range of Theta is limited depending on the target material.




For a Luminosity of