|
|
| Line 16: |
Line 16: |
| | | | |
| | =Transforming Cross Section Between Frames= | | =Transforming Cross Section Between Frames= |
| | + | ==Showing Equality== |
| | Transforming the cross section between two different frames of reference has the condition that the quantity must be equal in both frames. This is due to the fact that | | Transforming the cross section between two different frames of reference has the condition that the quantity must be equal in both frames. This is due to the fact that |
| | | | |
| Line 73: |
Line 74: |
| | | | |
| | | | |
| − | | + | ==Using Chain Rule== |
| | We can use the chain rule to find the transformation term on the right hand side: | | We can use the chain rule to find the transformation term on the right hand side: |
| | | | |
| Line 162: |
Line 163: |
| | | | |
| | <center><math>\frac{\partial p^*_z}{\partial p_z}=-\beta \gamma \frac{p_z}{E}+\frac{\gamma\, E}{E}=\frac{E^*}{E}</math></center> | | <center><math>\frac{\partial p^*_z}{\partial p_z}=-\beta \gamma \frac{p_z}{E}+\frac{\gamma\, E}{E}=\frac{E^*}{E}</math></center> |
| | + | |
| | + | ==Final Expression== |
Revision as of 17:49, 3 February 2016
Scattering Cross Section
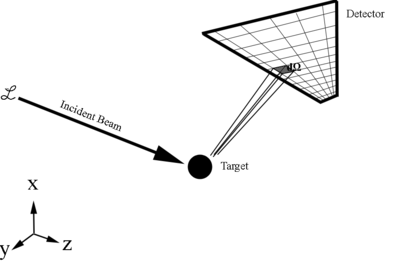
[math]\frac{d\sigma}{d\Omega} = \frac{\left(\frac{number\ of\ particles\ scattered/second}{d\Omega}\right)}{\left(\frac{number\ of\ incoming\ particles/second}{cm^2}\right)}=\frac{dN}{\mathcal L\, d\Omega} =differential\ scattering\ cross\ section[/math]
[math]where\ d\Omega=\sin{\theta}\,d\theta\,d\phi[/math]
[math]\Rightarrow \sigma=\int\limits_{\theta=0}^{\pi} \int\limits_{\phi=0}^{2\pi} \left(\frac{d\sigma}{d\Omega}\right)\ \sin{\theta}\,d\theta\,d\phi =\frac{N}{\mathcal L}\equiv total\ scattering\ cross\ section[/math]
Since this is just a ratio of detected particles to total particles, this gives the cross section as a relative probablity of a scattering, or reaction, to occur.
Transforming Cross Section Between Frames
Showing Equality
Transforming the cross section between two different frames of reference has the condition that the quantity must be equal in both frames. This is due to the fact that
[math]\sigma=\frac{N}{\mathcal L}=constant\ number[/math]
This makes the total cross section a Lorentz invariant in that it is not effected by any relativistic transformations.
[math]\therefore\ \sigma_{CM}=\sigma_{Lab}[/math]
This implies that the number of particles going into the solid-angle element d ΩLab and having a momentum between pLab and pLab+dpLabbe the same as the number going into the corresponding solid-angle element dΩCM and having a corresponding momentum between pCM and pCM+dpCM
[math]\frac{\partial ^2\sigma_{Lab}}{\partial p_{Lab}\,\partial \Omega_{Lab}}dp_{Lab}\,d\Omega_{Lab}=\frac{\partial ^2\sigma_{CM}}{\partial p_{CM}\,\partial \Omega_{CM}}dp_{CM}\, d\Omega_{CM}[/math]
[math]where\ d\Omega=\sin{\theta}\,d\theta\,d\phi[/math]
Expressing this in terms of the solid angle components,
[math]\frac{\partial ^2\sigma_{Lab}}{\partial p_{Lab}\,\partial \Omega_{Lab}}\partial p_{Lab}\,\sin{\theta_{Lab}}\,d\theta_{Lab}\,d\phi_{Lab}=\frac{d^2\sigma_{CM}}{dp_{CM}\, d\Omega_{CM}}dp_{CM}\, \sin{\theta_{CM}}\,d\theta_{CM}\,d\phi_{CM}[/math]
As shown earlier,
[math]\phi_{Lab}=\phi_{CM}[/math]
Thus,
[math]\Rightarrow\ d\phi_{Lab}=d\phi_{CM}[/math]
Simplify our expression for the cross section gives:
[math]\frac{\partial ^2\sigma_{Lab}}{\partial p_{Lab}\,\partial \Omega_{Lab}} dp_{Lab}\,\sin{\theta_{Lab}}\,d\theta_{Lab}=\frac{\partial ^2\sigma_{CM}}{\partial p_{CM}\, \partial \Omega_{CM}}dp_{CM}\, \sin{\theta_{CM}}\,d\theta_{CM}[/math]
We can use the fact that
[math]\sin{\theta}\ d\theta=d(\cos{\theta})[/math]
To give
[math]\frac{\partial ^2\sigma_{Lab}}{\partial p_{Lab}\,\partial \Omega_{Lab}} dp_{Lab}\,d(\cos{\theta_{Lab}})=\frac{\partial ^2\sigma_{CM}}{\partial p_{CM}\, \partial \Omega_{CM}}dp_{CM}\, d(\cos{\theta_{CM}})[/math]
[math]\frac{\partial ^2\sigma_{Lab}}{\partial p_{Lab}\,\partial \Omega_{Lab}} =\frac{\partial ^2\sigma_{CM}}{\partial p_{CM}\, \partial \Omega_{CM}} \frac{dp_{CM}\,d(\cos{\theta_{CM}})}{dp_{Lab}\,d(\cos{\theta_{Lab}})}[/math]
[math]\frac{\partial ^2\sigma_{Lab}}{\partial p_{Lab}\,\partial \Omega_{Lab}} =\frac{\partial ^2\sigma_{CM}}{\partial p_{CM}\, \partial \Omega_{CM}} \frac{\partial (p_{CM}\,\cos{\theta_{CM})}}{\partial (p_{Lab}\,\cos{\theta_{Lab}})}[/math]
Using Chain Rule
We can use the chain rule to find the transformation term on the right hand side:
[math]\frac{\partial (p^*\, \cos{\theta^*)}}{\partial (p^*\, \theta^*\, \phi^*)} \frac{\partial (p^*\, \theta^*\, \phi^*)}{\partial (p^*_x\, p^*_y\, p^*_z)} \frac{\partial (p^*_x\, p^*_y\, p^*_z)}{\partial (p_x\, p_y\, p_z)} \frac{\partial (p_x\, p_y\, p_z)}{\partial (p\, \theta\, \phi)} \frac{\partial (p\, \theta\, \phi)}{\partial (p\, \cos{\theta})}=\frac{\partial (p^*\, \cos{\theta^*})}{\partial (p\, \cos{\theta})}[/math]
[math]\frac{\partial (p^*\, \cos{\theta^*})} {\partial (p^*\, \theta^*\phi^*)}=\frac{d p^*\, \sin{\theta^*} \, d \theta^* \, d \phi^*}{d p^*\, d \theta^*\, d \phi^*}=\sin{\theta^*}[/math]
Similarly,
[math]\frac{\partial (p\, \theta\, \phi)}{\partial (p\, \cos{\theta})}=\frac{1}{\sin{\theta}}[/math]
Using the conversion of cartesian to spherical coordinates we know:
[math]\begin{cases}
p_x=p\, \sin{\theta}\, \cos{\phi} \\
p_y=p\, \sin{\theta}\, \sin{\phi} \\
p_z=p\, \cos{\theta}
\end{cases}[/math]
and the fact that as was shown earlier, that
[math]\begin{cases}
p^*_x=p_x \\
p^*_y=p_y \\
\phi^*=\phi
\end{cases}[/math]
This allows us to express the term:
[math]\frac{\partial (p^*\, \theta^*\, \phi^*)}{\partial (p^*_x\, p^*_y\, p^*_z)}=\biggl[\frac{\partial (p^*_x\, p^*_y\, p^*_z)}{\partial (p^*\, \theta^*\, \phi^*)}\biggr]^{-1}=\biggl[\frac{\partial (p^*\, \sin{\theta^*}\, \cos{\phi^*}p^*\, \sin{\theta^*}\, \sin{\phi^*}p^*\, \cos{\theta^*})}{\partial p^*\, \partial \theta^*\, \partial \phi^*}\biggr]^{-1}[/math]
[math]\frac{\partial (p^*\, \theta^*\, \phi^*)}{\partial (p^*_x\, p^*_y\, p^*_z)}=\biggl[ \frac{d (p^{*-1})\, d(\cos{\theta^{*}}^{-1})} {d p^*\, d\theta^*}\biggr]=\frac{1}{p^{*2}\sin{\theta^*}}[/math]
Again, similarly
[math]\frac{\partial (p_x\, p_y\, p_z)}{\partial (p\, \theta\, \phi)}=p^2\, \sin{\theta}[/math]
To find the middle component in the chain rule expansion,
[math]\left( \begin{matrix} E^* \\ p^*_x \\ p^*_y \\ p^*_z\end{matrix} \right)=\left(\begin{matrix}\gamma & 0 & 0 & -\beta \gamma\\0 & 1 & 0 & 0 \\ 0 & 0 & 1 &0 \\ -\beta\gamma & 0 & 0 & \gamma \end{matrix} \right) . \left( \begin{matrix}E\\ p_x \\ p_y\\ p_z\end{matrix} \right)[/math]
which gives,
[math]\Longrightarrow\begin{cases}
E^*=\gamma E-\beta \gamma^* p_z \\
p^*_z=-\beta \gamma\, E+\gamma\, p_z
\end{cases}[/math]
[math]\frac{\partial (p^*_x\, p^*_y\, p^*_z)}{\partial (p_x\, p_y\, p_z)}=\frac{\partial p^*_z}{\partial p_z}=\frac{\partial (-\beta \gamma\, E+\gamma\, p_z)}{\partial p_z}=-\beta \gamma \,\frac{\partial E}{\partial p_z}+\gamma[/math]
We can use the relativistic definition of the total Energy,
[math]E=\sqrt{p^2+m^2}=\sqrt{p_x^2+p_y^2+p_z^2+m^2}[/math]
[math]\Rightarrow \frac{\partial p^*_z}{\partial p_z}=\frac{\sqrt {p_x^2+p_y^2+p_z^2+m^2}} {\partial p_z}=\frac{p_z}{\sqrt {p_x^2+p_y^2+p_z^2+m^2}}=\frac{p_z}{E}[/math]
[math]\frac{\partial p^*_z}{\partial p_z}=-\beta \gamma \frac{\partial E}{\partial p_z}+\gamma=-\beta \gamma \frac{p_z}{E}+\gamma[/math]
Then using the fact that
[math]E^*\equiv \gamma E-\beta \gamma\, p_z [/math]
[math]\frac{\partial p^*_z}{\partial p_z}=-\beta \gamma \frac{p_z}{E}+\frac{\gamma\, E}{E}=\frac{E^*}{E}[/math]
Final Expression
